Chapter 2.1, Problem 35E

a.

To determine

Construct a frequency bar graph for each city.

Answer to Problem 35E

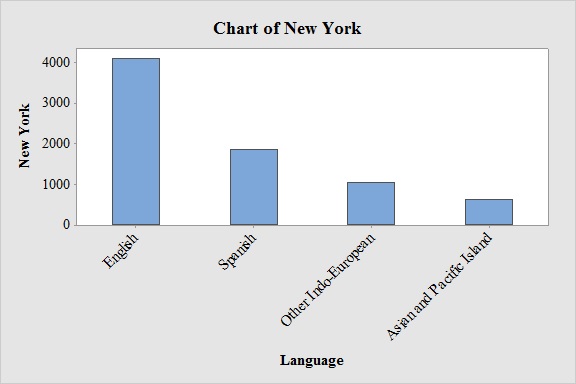
Output obtained from MINITAB software for New York is:

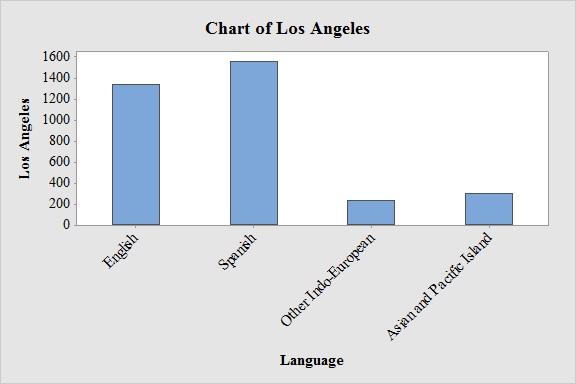
Output obtained from MINITAB software for Los Angeles is:

Explanation of Solution

Calculation:

The given information is a table representing the number of households categorized by the language spoken at home, for the cities of New York and Los Angeles in a recent year.

Software procedure:

• Step by step procedure to draw the bar chart for each city using MINITAB software.
• Choose Graph > Bar Chart.
• From Bars represent, choose unique values from table.
• Choose Simple.
• Click OK.
• In Graph variables, enter the column of New York and Los Angeles.
• In Categorical variables, enter the column of Language.
• Click OK

Observation:

From the bar graphs, it can be seen that the most frequently spoken language at home in New York and Los Angeles are English and Spanish respectively.

b.

To determine

Construct a frequency bar graph for the total.

Answer to Problem 35E

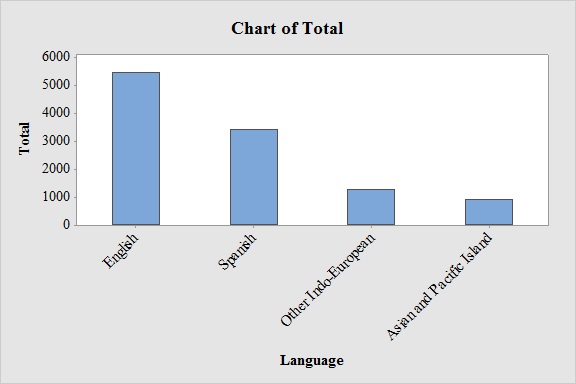
Output obtained from MINITAB software for Total is:

Explanation of Solution

Calculation:

Software procedure:

• Step by step procedure to draw the bar chart for each city using MINITAB software.
• Choose Graph > Bar Chart.
• From Bars represent, choose unique values from table.
• Choose Simple.
• Click OK.
• In Graph variables, enter the column of Total.
• In Categorical variables, enter the column of Language.
• Click OK

Observation:

From the bar graphs, it can be seen that the most frequently spoken language at home in both New York and Los Angeles is English.

c.

To determine

Construct a relative frequency bar graph for each city.

Answer to Problem 35E

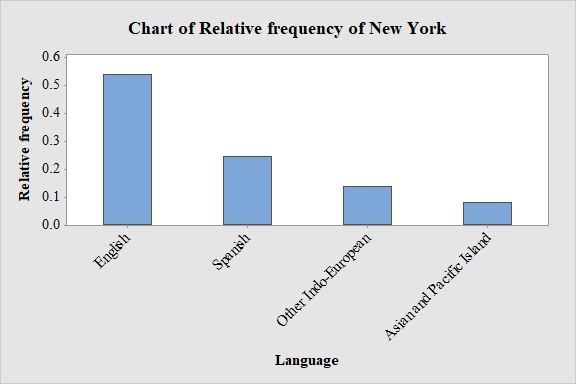
Output obtained from MINITAB software for New York is:

Output obtained from MINITAB software for Los Angeles is:

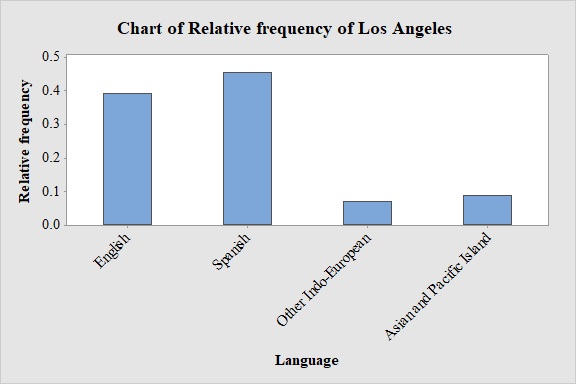
Explanation of Solution

Calculation:

Relative frequency for New York:

The general formula for the relative frequency is,

$\text{Relative}\text{Frequency = }\frac{\text{Frequency}}{\text{Total frequency}}$

Therefore,

$\begin{array}{c}\text{Relative}\text{Frequency}\text{for English}=\frac{4,098}{7,623}\\ =0.538\end{array}$

Similarly, the relative frequencies for New York is obtained below:

Language	New York	Relative Frequency
English	4,098	$\frac{4,098}{7,623}=0.538$
Spanish	1,870	$\frac{1,870}{7,623}=0.245$
Other Indo-European	1,037	$\frac{1,037}{7,623}=0.136$
Asian and Pacific Island	618	$\frac{618}{7,623}=0.081$

Software procedure:

• Step by step procedure to draw the Bar chart for each city using MINITAB software.
• Choose Graph > Bar Chart.
• From Bars represent, choose unique values from table.
• Choose Simple.
• Click OK.
• In Graph variables, enter the column of Relative Frequency of New York
• In Categorical variables, enter the column of Language.
• Click OK

Observation:

From the graph, it can be seen that most probable spoken language at home in New York is English.

Relative frequency for Los Angeles:

$\begin{array}{c}\text{Relative}\text{Frequency}\text{for English}=\frac{1,339}{3,432}\\ =0.390\end{array}$

Similarly, the relative frequencies for Los Angeles is obtained below:

Language	Los Angeles	Relative Frequency
English	1,339	$\frac{1,339}{3,432}=0.390$
Spanish	1,555	$\frac{1,555}{3,432}=0.453$
Other Indo-European	237	$\frac{237}{3,432}=0.069$
Asian and Pacific Island	301	$\frac{301}{3,432}=0.088$

Software procedure:

• Step by step procedure to draw the Bar chart for Los Angeles using MINITAB software.
• Choose Graph > Bar Chart.
• From Bars represent, choose unique values from table.
• Choose Simple.
• Click OK.
• In Graph variables, enter the column of Relative Frequency of Los Angeles.
• In Categorical variables, enter the column of Language.
• Click OK

Observation:

From the graph, it can be seen that most probable spoken language at home in Los Angeles is Spanish.

d.

To determine

Construct a relative frequency bar graph for the total.

Answer to Problem 35E

Output obtained from MINITAB software for Total is:

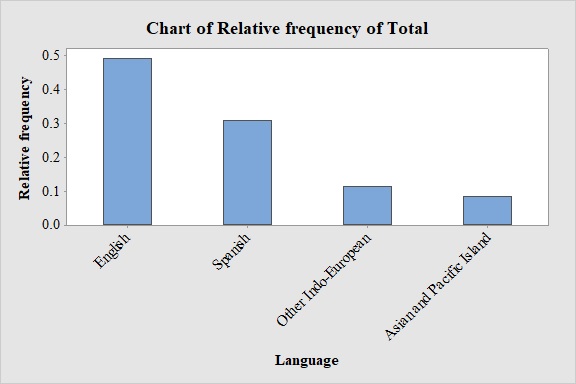
Explanation of Solution

Calculation:

Relative frequency for total:

The general formula for the relative frequency is,

$\text{Relative}\text{Frequency = }\frac{\text{Frequency}}{\text{Total frequency}}$

Therefore,

$\begin{array}{c}\text{Relative}\text{Frequency}\text{for English}=\frac{5,437}{11,055}\\ =0.492\end{array}$

Similarly, the relative frequencies for the total are obtained below:

Language	Total	Relative Frequency
English	5,437	$\frac{5,437}{11,055}=0.492$
Spanish	3,425	$\frac{3,425}{11,055}=0.310$
Other Indo-European	1,274	$\frac{1,274}{11,055}=0.115$
Asian and Pacific Island	919	$\frac{919}{11,055}=0.083$

Software procedure:

• Step by step procedure to draw the Bar chart for total using MINITAB software.
• Choose Graph > Bar Chart.
• From Bars represent, choose unique values from table.
• Choose Simple.
• Click OK.
• In Graph variables, enter the column of Relative frequency of total.
• In Categorical variables, enter the column of Language.
• Click OK

Observation:

From the graph, it can be seen that most probable spoken language at home in both New York and Los Angeles is English.

e.

To determine

Explain the reason behind the heights of the bars for the frequency bar graph for the total are equal to the sums of the heights for the individual cities.

Explanation of Solution

The total frequency represents the numbers of households in both cities combined. Therefore, the total frequency is the sum of the frequencies for New York and Los Angeles.

f.

To determine

Explain the reason behind the heights of the bars for the relative frequency bar graph for the total are not equal to the sums of the heights for the individual cities.

Explanation of Solution

The relative frequency is the frequency divided by total frequency. The frequencies and total frequencies are different for each cities. Therefore, the relative frequency bar graph for the total are not equal to the sums of the heights for the individual cities.