
Concept explainers
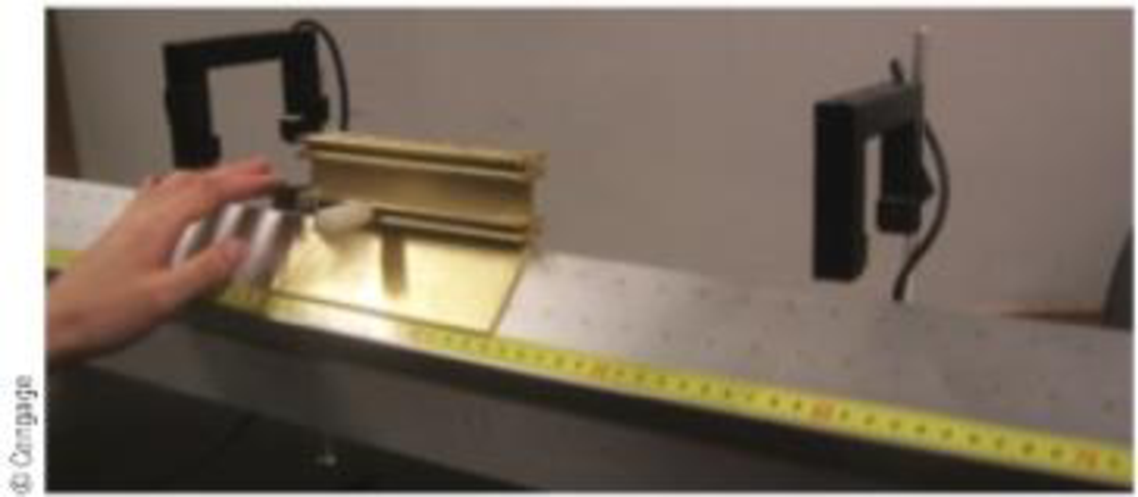
A glider of length ℓ moves through a stationary photogate on an air track. A photogate (Fig. P2.19) is a device that measures the time interval Δtd during which the glider blocks a beam of infrared light passing across the photogate. The ratio vd = ℓ/Δtd is the average velocity of the glider over this part of its motion. Suppose the glider moves with constant acceleration. (a) Argue for or against the idea that vd is equal to the instantaneous velocity of the glider when it is halfway through the photogate in space. (b) Argue for or against the idea that vd is equal to the instantaneous velocity of the glider when it is halfway through the photogate in time.
Figure P2.19
Want to see the full answer?
Check out a sample textbook solution
Chapter 2 Solutions
Bundle: Physics for Scientists and Engineers with Modern Physics, Loose-leaf Version, 9th + WebAssign Printed Access Card, Multi-Term
- 2. A projectile is shot from a launcher at an angle 0,, with an initial velocity magnitude vo, from a point even with a tabletop. The projectile hits an apple atop a child's noggin (see Figure 1). The apple is a height y above the tabletop, and a horizontal distance x from the launcher. Set this up as a formal problem, and solve for x. That is, determine an expression for x in terms of only v₁, o,y and g. Actually, this is quite a long expression. So, if you want, you can determine an expression for x in terms of v., 0., and time t, and determine another expression for timet (in terms of v., 0., y and g) that you will solve and then substitute the value of t into the expression for x. Your final equation(s) will be called Equation 3 (and Equation 4).arrow_forward4.56 ... CALC An object of mass m is at rest in equilibrium at the origin. At t = 0 a new force F(t) is applied that has components Fx(t) = k₁ + k₂y Fy(t) = k3t where k₁, k2, and k3 are constants. Calculate the position (1) and veloc- ity (t) vectors as functions of time.arrow_forward4.14 ⚫ A 2.75 kg cat moves in a straight line (the x-axis). Figure E4.14 shows a graph of the x- component of this cat's velocity as a function of time. (a) Find the maximum net force on this cat. When does this force occur? (b) When is the net force on the cat equal to zero? (c) What is the net force at time 8.5 s? Figure E4.14 V₁ (m/s) 12.0 10.0 8.0 6.0 4.0 2.0 0 t(s) 2.0 4.0 6.0 8.0 10.0arrow_forward
- 4.36 ... CP An advertisement claims that a particular automobile can "stop on a dime." What net force would be necessary to stop a 850 kg automobile traveling initially at 45.0 km/h in a distance equal to the di- ameter of a dime, 1.8 cm?arrow_forward4.46 The two blocks in Fig. P4.46 are connected by a heavy uniform rope with a mass of 4.00 kg. An up- ward force of 200 N is applied as shown. (a) Draw three free-body diagrams: one for the 6.00 kg block, one for B the 4.00 kg rope, and another one for the 5.00 kg block. For each force, indicate what object exerts that force. (b) What is the acceleration of the system? (c) What is the tension at the top of the heavy rope? (d) What is the tension at the midpoint of the rope? Figure P4.46 F= 200 N 4.00 kg 6.00 kg 5.00 kgarrow_forward4.35 ⚫ Two adults and a child want to push a wheeled cart in the direc- tion marked x in Fig. P4.35 (next page). The two adults push with hori- zontal forces F and F as shown. (a) Find the magnitude and direction of the smallest force that the child should exert. Ignore the effects of friction. (b) If the child exerts the minimum force found in part (a), the cart ac- celerates at 2.0 m/s² in the +x-direction. What is the weight of the cart? Figure P4.35 F₁ = 100 N 60° 30° F2 = 140 Narrow_forward
- 4.21 ⚫ BIO World-class sprinters can accelerate out of the starting blocks with an acceleration that is nearly horizontal and has magnitude 15 m/s². How much horizontal force must a 55 kg sprinter exert on the starting blocks to produce this acceleration? Which object exerts the force that propels the sprinter: the blocks or the sprinter herself?arrow_forwardNo chatgpt pls will upvotearrow_forwardPlease don't use Chatgpt will upvote and give handwritten solutionarrow_forward
- The kinetic energy of a pendulum is greatest Question 20Select one: a. at the top of its swing. b. when its potential energy is greatest. c. at the bottom of its swing. d. when its total energy is greatest.arrow_forwardPart a-D plarrow_forwardThe figure (Figure 1) shows representations of six thermodynamic states of the same ideal gas sample. Figure 1 of 1 Part A ■Review | Constants Rank the states on the basis of the pressure of the gas sample at each state. Rank pressure from highest to lowest. To rank items as equivalent, overlap them. ▸ View Available Hint(s) highest 0 ☐ ☐ ☐ ☐ ☐ ☐ Reset Help B F A D E The correct ranking cannot be determined. Submit Previous Answers × Incorrect; Try Again; 4 attempts remaining Provide Feedback lowest Next >arrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill





