Fundamentals of Physics Extended
10th Edition
ISBN: 9781118230725
Author: David Halliday, Robert Resnick, Jearl Walker
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 15, Problem 8P
What is the phase constant for the harmonic oscillator with the position function x(t) given in Fig. 15-30 if the position function has the form x = xm cos(ωt + ϕ)? The vertical axis scale is set by xs = 6.0 cm.
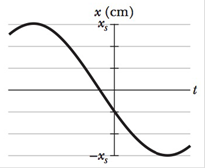
Figure 15-30 Problem 8.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Your RL circuit has a characteristic time constant of 19.5 ns, and a resistance of 4.60 MQ.
(a) What is the inductance (in H) of the circuit?
0.00897
× H
(b) What resistance (in MQ) should you use (instead of the 4.60 MQ resistor) to obtain a 1.00 ns time constant, perhaps needed for quick response in an oscilloscope?
8.97
* ΜΩ
Your RL circuit has a characteristic time constant of 19.5 ns, and a resistance of 4.60 MQ.
(a) What is the inductance (in H) of the circuit?
H
(b) What resistance (in MQ) should you use (instead of the 4.60 MQ resistor) to obtain a 1.00 ns time constant, perhaps needed for quick response in an oscilloscope?
ΜΩ
At a distance of 0.212 cm from the center of a charged conducting sphere with radius 0.100cm, the electric field is 485 N/C . What is the electric field 0.598 cm from the center of the sphere? At a distance of 0.196 cmcm from the axis of a very long charged conducting cylinder with radius 0.100cm, the electric field is 485 N/C . What is the electric field 0.620 cm from the axis of the cylinder? At a distance of 0.202 cm from a large uniform sheet of charge, the electric field is 485 N/C . What is the electric field 1.21 cm from the sheet?
Chapter 15 Solutions
Fundamentals of Physics Extended
Ch. 15 - Which of the following describe for the SHM of...Ch. 15 - The velocity vt of a particle undergoing SHM is...Ch. 15 - The acceleration at of a particle undergoing SHM...Ch. 15 - Which of the following relationships between the...Ch. 15 - You are to complete Fig. 15-22a so that it is a...Ch. 15 - You are to complete Fig. 15-23a so that it is a...Ch. 15 - Figure 15-24 shows the xt curves for three...Ch. 15 - Figure 15-25 shows plots of the kinetic energy K...Ch. 15 - Figure 15-26 shows three physical pendulums...Ch. 15 - You are to build the oscillation transfer device...
Ch. 15 - In Fig. 15-28, a springblock system is put into...Ch. 15 - Figure 15-29 gives, for three situations, the...Ch. 15 - An object undergoing simple harmonic motion takes...Ch. 15 - A 0.12 kg body undergoes simple harmonic motion of...Ch. 15 - What is the maximum acceleration of a platform...Ch. 15 - An automobile can be considered to be mounted on...Ch. 15 - SSM In an electric shaver, the blade moves back...Ch. 15 - A particle with a mass of 1.00 1020 kg is...Ch. 15 - SSM A loudspeaker produces a musical sound by...Ch. 15 - What is the phase constant for the harmonic...Ch. 15 - The position function x = 6.0 m cos3 rad/st /3...Ch. 15 - An oscillating blockspring system takes 0.75 s to...Ch. 15 - In Fig. 15-31, two identical springs of spring...Ch. 15 - What is the phase constant for the harmonic...Ch. 15 - SSM An oscillator consists of a block of mass...Ch. 15 - A simple harmonic oscillator consists of a block...Ch. 15 - SSM Two particles oscillate in simple harmonic...Ch. 15 - Two particles execute simple harmonic motion of...Ch. 15 - ILW An oscillator consists of a block attached to...Ch. 15 - GO At a certain harbor, the tides cause the ocean...Ch. 15 - A block rides on a piston a squat cylindrical...Ch. 15 - GO Figure 15-33a is a partial graph of the...Ch. 15 - ILW In Fig. 15-31, two springs are attached to a...Ch. 15 - GO Figure 15-34 shows block 1 of mass 0.200 kg...Ch. 15 - SSM WWW A block is on a horizontal surface a shake...Ch. 15 - In Fig. 15-35, two springs are joined and...Ch. 15 - GO In Fig. 15-36, a block weighing 14.0 N, which...Ch. 15 - GO In Fig. 15-37, two blocks m = 1.8 kg and M = 10...Ch. 15 - SSM When the displacement in SHM is one-half the...Ch. 15 - Figure 15-38 gives the one-dimensional potential...Ch. 15 - SSM Find the mechanical energy of a blockspring...Ch. 15 - An oscillating blockspring system has a mechanical...Ch. 15 - ILW A 5.00 kg object on a horizontal frictionless...Ch. 15 - Figure 15-39 shows the kinetic energy K of a...Ch. 15 - GO A block of mass M = 5.4 kg, at rest on a...Ch. 15 - GO In Fig. 15-41, block 2 of mass 2.0 kg...Ch. 15 - A 10 g particle undergoes SHM with an amplitude of...Ch. 15 - If the phase angle for a blockspring system in SHM...Ch. 15 - GO A massless spring hangs from the ceiling with a...Ch. 15 - A 95 kg solid sphere with a 15 cm radius is...Ch. 15 - SSM WWW The balance wheel of an old-fashioned...Ch. 15 - ILW A physical pendulum consists of a meter stick...Ch. 15 - SSM In Fig. 15-42, the pendulum consists of a...Ch. 15 - Suppose that a simple pendulum consists of a small...Ch. 15 - a If the physical pendulum of Fig. 15-13 and the...Ch. 15 - A physical pendulum consists of two meter-long...Ch. 15 - A performer seated on a trapeze is swinging back...Ch. 15 - A physical pendulum has a center of oscillation at...Ch. 15 - In Fig. 15-44, a physical pendulum consists of a...Ch. 15 - GO A rectangular block, with face lengths a = 35...Ch. 15 - GO The angle of the pendulum of Fig. 15-11b is...Ch. 15 - Prob. 50PCh. 15 - GO In Fig. 15-46, a stick of length L = 1.85 m...Ch. 15 - GO The 3.00 kg cube in Fig. 15-47 has edge lengths...Ch. 15 - SSM ILW In the overhead view of Fig. 15-48, a long...Ch. 15 - Prob. 54PCh. 15 - GO A pendulum is formed by pivoting a long thin...Ch. 15 - In Fig. 15-50: a 2.50 kg disk of diameter D = 42.0...Ch. 15 - The amplitude of a lightly damped oscillator...Ch. 15 - For the damped oscillator system shown in Fig....Ch. 15 - SSM WWW For the damped oscillator system shown in...Ch. 15 - The suspension system of a 2000 kg automobile sags...Ch. 15 - For Eq. 15-45, suppose the amplitude xm is given...Ch. 15 - Hanging from a horizontal beam are nine simple...Ch. 15 - A. 1000 kg car carrying four 82 kg people travels...Ch. 15 - Although California is known for earthquakes, is...Ch. 15 - A loudspeaker diaphragm is oscillating in simple...Ch. 15 - A uniform spring with k = 8600 N/m is cut into...Ch. 15 - GO In Fig. 15-51, three 10, 000 kg ore cars are...Ch. 15 - A 2.00 kg block hangs from a spring. A 300 g body...Ch. 15 - SSM In the engine of a locomotive, a cylindrical...Ch. 15 - GO A wheel is free to rotate about its fixed axle....Ch. 15 - A 50.0 g stone is attached to the bottom of a...Ch. 15 - A uniform circular disk: whose radius R is 12.6 cm...Ch. 15 - SSM A vertical spring stretches 9.6 cm when a 1.3...Ch. 15 - A massless spring with spring constant 19 N/m...Ch. 15 - A 4.00 kg block is suspended from a spring with k...Ch. 15 - A 55.0 g block oscillates in SHM on the end of a...Ch. 15 - Figure 15-53 gives the position of a 20 g block...Ch. 15 - Figure 15-53 gives the position xt of a block...Ch. 15 - Figure 15-54 shows the kinetic energy K of a...Ch. 15 - A block is in SHM on the end of a spring, with...Ch. 15 - A simple harmonic oscillator consists of a 0.50 kg...Ch. 15 - A simple pendulum of length 20 cm and mass 5.0 g...Ch. 15 - The scale of a spring balance that reads from 0 to...Ch. 15 - A 0.10 kg block oscillates back and forth along a...Ch. 15 - The end point of a spring oscillates with a period...Ch. 15 - The tip of one prong of a tuning fork undergoes...Ch. 15 - Prob. 87PCh. 15 - A block weighing 20 N oscillates at one end of a...Ch. 15 - A 3.0 kg particle is in simple harmonic motion in...Ch. 15 - A particle executes linear SHM with frequency 0.25...Ch. 15 - SSM What is the frequency of a simple pendulum 2.0...Ch. 15 - A grandfather clock has a pendulum that consists...Ch. 15 - A 4.00 kg block hangs from a spring, extending it...Ch. 15 - What is the phase constant for SMH with at given...Ch. 15 - An engineer has an odd-shaped 10 kg object and...Ch. 15 - A spider can tell when its web has captured, say,...Ch. 15 - A torsion pendulum consists of a metal disk with a...Ch. 15 - When a 20 N can is hung from the bottom of a...Ch. 15 - For a simple pendulum, find the angular amplitude...Ch. 15 - In Fig. 15-59, a solid cylinder attached to a...Ch. 15 - SSM A 1.2 kg block sliding on a horizontal...Ch. 15 - A simple harmonic oscillator consists of an 0.80...Ch. 15 - A block sliding on a horizontal frictionless...Ch. 15 - A damped harmonic oscillator consists of a block m...Ch. 15 - A block weighing 10.0 N is attached to the lower...Ch. 15 - A simple harmonic oscillator consists of a block...Ch. 15 - The vibration frequencies of atoms in solids at...Ch. 15 - Figure 15-61 shows that if we hang a block on the...Ch. 15 - The physical pendulum in Fig. 15-62 has two...Ch. 15 - A common device for entertaining a toddler is a...Ch. 15 - A 2.0 kg block executes SHM while attached to a...Ch. 15 - In Fig. 15-64, a 2500 kg demolition ball swings...Ch. 15 - The center of oscillation of a physical pendulum...Ch. 15 - A hypothetical large slingshot is stretched 2.30 m...Ch. 15 - What is the length of a simple pendulum whose full...Ch. 15 - A 2.0 kg block is attached to the end of a spring...
Additional Science Textbook Solutions
Find more solutions based on key concepts
16. Explain some of the reasons why the human species has been able to expand in number and distribution to a g...
Campbell Biology: Concepts & Connections (9th Edition)
8. A human maintaining a vegan diet (containing no animal products) would be a:
a. producer
b. primary consume...
Human Biology: Concepts and Current Issues (8th Edition)
12.1 Give the IUPAC name for each of the following:
a. CH3-CH2-OH
b.
c.
d.
Chemistry: An Introduction to General, Organic, and Biological Chemistry (13th Edition)
Explain all answers clearly, with complete sentences and proper essay structure if needed. An asterisk (*) desi...
Cosmic Perspective Fundamentals
SCIENTIFIC INQUIRY DRAW IT As a consequence of size alone, larger organisms tend to have larger brains than sm...
Campbell Biology (11th Edition)
9. FIGURE EX1.9 shows five points of a motion diagram. Use Tactics Box 1.3 to find the average acceleration vec...
Physics for Scientists and Engineers: A Strategic Approach, Vol. 1 (Chs 1-21) (4th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- A hollow, conducting sphere with an outer radius of 0.260 m and an inner radius of 0.200 m has a uniform surface charge density of +6.67 × 10−6 C/m2. A charge of -0.800 μC is now introduced into the cavity inside the sphere. What is the new charge density on the outside of the sphere? Calculate the strength of the electric field just outside the sphere. What is the electric flux through a spherical surface just inside the inner surface of the sphere?arrow_forwardA point charge of -3.00 μC is located in the center of a spherical cavity of radius 6.60 cm inside an insulating spherical charged solid. The charge density in the solid is 7.35 × 10−4 C/m3. Calculate the magnitude of the electric field inside the solid at a distance of 9.10 cm from the center of the cavity. Find the direction of this electric field.arrow_forwardAn infinitely long conducting cylindrical rod with a positive charge λ per unit length is surrounded by a conducting cylindrical shell (which is also infinitely long) with a charge per unit length of −2λ and radius r1, as shown in the figure. What is E(r), the radial component of the electric field between the rod and cylindrical shell as a function of the distance r from the axis of the cylindrical rod? Express your answer in terms of λ, r, and ϵ0, the permittivity of free space. What is σinner, the surface charge density (charge per unit area) on the inner surface of the conducting shell? What is σouterσouter, the surface charge density on the outside of the conducting shell? (Recall from the problem statement that the conducting shell has a total charge per unit length given by −2λ.) What is the radial component of the electric field, E(r), outside the shell?arrow_forward
- A very long conducting tube (hollow cylinder) has inner radius aa and outer radius b. It carries charge per unit length +α, where αα is a positive constant with units of C/m. A line of charge lies along the axis of the tube. The line of charge has charge per unit length +α. Calculate the electric field in terms of α and the distance r from the axis of the tube for r<a. Calculate the electric field in terms of α and the distance rr from the axis of the tube for a<r<b. Calculate the electric field in terms of αα and the distance r from the axis of the tube for r>b. What is the charge per unit length on the inner surface of the tube? What is the charge per unit length on the outer surface of the tube?arrow_forwardTwo small insulating spheres with radius 9.00×10−2 m are separated by a large center-to-center distance of 0.545 m . One sphere is negatively charged, with net charge -1.75 μC , and the other sphere is positively charged, with net charge 3.70 μC . The charge is uniformly distributed within the volume of each sphere. What is the magnitude E of the electric field midway between the spheres? Take the permittivity of free space to be ϵ0 = 8.85×10−12 C2/(N⋅m2) . What is the direction of the electric field midway between the spheres?arrow_forwardA conducting spherical shell with inner radius aa and outer radius bb has a positive point charge Q located at its center. The total charge on the shell is -3Q, and it is insulated from its surroundings. Derive the expression for the electric field magnitude in terms of the distance r from the center for the region r<a. Express your answer in terms of some or all of the variables Q, a, b, and appropriate constants. Derive the expression for the electric field magnitude in terms of the distance rr from the center for the region a<r<b. Derive the expression for the electric field magnitude in terms of the distance rr from the center for the region r>b. What is the surface charge density on the inner surface of the conducting shell? What is the surface charge density on the outer surface of the conducting shell?arrow_forward
- A small sphere with a mass of 3.00×10−3 g and carrying a charge of 4.80×10−8 C hangs from a thread near a very large, charged insulating sheet, as shown in the figure (Figure 1). The charge density on the sheet is −2.20×10−9 C/m2 . Find the angle of the thread.arrow_forwardA small conducting spherical shell with inner radius aa and outer radius bb is concentric with a larger conducting spherical shell with inner radius c and outer radius d (Figure 1). The inner shell has total charge +2q, and the outer shell has charge −2q. Calculate the magnitude of the electric field in terms of q and the distance rr from the common center of the two shells for r<a. Calculate the magnitude of the electric field for a<r<b. Calculate the magnitude of the electric field for b<r<c.arrow_forwardA cube has sides of length L = 0.800 m . It is placed with one corner at the origin as shown in the figure. The electric field is not uniform but is given by E→=αxi^+βzk^, where α=−3.90 and β= 7.10. What is the sum of the flux through the surface S5 and S6? What is the sum of the flux through the surface S2 and S4? Find the total electric charge inside the cube.arrow_forward
- In the figure, a proton is projected horizontally midway between two parallel plates that are separated by 0.6 cm. The electrical field due to the plates has magnitude 450000 N/C between the plates away from the edges. If the plates are 3 cm long, find the minimum speed of the proton if it just misses the lower plate as it emerges from the field.arrow_forwardA point charge of magnitude q is at the center of a cube with sides of length L. What is the electric flux Φ through each of the six faces of the cube? What would be the flux Φ1 through a face of the cube if its sides were of length L1? Please explain everything.arrow_forwardIf a 1/2 inch diameter drill bit spins at 3000 rotations per minute, how fast is the outer edge moving as it contacts a piece of metal while drilling a machine part?arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Modern PhysicsPhysicsISBN:9781111794378Author:Raymond A. Serway, Clement J. Moses, Curt A. MoyerPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
Classical Dynamics of Particles and Systems
Physics
ISBN:9780534408961
Author:Stephen T. Thornton, Jerry B. Marion
Publisher:Cengage Learning
University Physics Volume 1
Physics
ISBN:9781938168277
Author:William Moebs, Samuel J. Ling, Jeff Sanny
Publisher:OpenStax - Rice University
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Modern Physics
Physics
ISBN:9781111794378
Author:Raymond A. Serway, Clement J. Moses, Curt A. Moyer
Publisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...
Physics
ISBN:9781305116399
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
What Are Sound Wave Properties? | Physics in Motion; Author: GPB Education;https://www.youtube.com/watch?v=GW6_U553sK8;License: Standard YouTube License, CC-BY