McDougal Littell Jurgensen Geometry: Student Edition Geometry

5th Edition

ISBN: 9780395977279

Author: Ray C. Jurgensen, Richard G. Brown, John W. Jurgensen

Publisher: Houghton Mifflin Company College Division

See similar textbooks

Concept explainers

Statements and Logic

Logic is the analysis of general patterns of thoughts without regard to any specific sense of context.

Videos

Question

Chapter 1.5, Problem 18WE

(a)

To determine

To check: The postulate that guarantees the existence of the plane ABC, ABD, ACD, BCD .

(a)

Expert Solution

Answer to Problem 18WE

It is the postulate 7.

Explanation of Solution

Given information:

Consider the statements below and associated figure

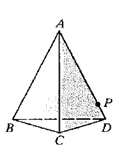
Points A , B , C and D are four non coplanar points

McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 1.5, Problem 18WE , additional homework tip 1

Formula used:

Through any 3 non collinear points there is exactly one plane

Calculation:

From Postulate 7, through any 3 non collinear points there is exactly one plane. From the figure, points A , B , C and D are non collinear points, therefore from above argument there will be exactly one plane through points A,B,C and A,B,D and A,C,D and B,C,D

Hence, the answer is postulate 7.

Conclusion:

It is the postulate 7.

(b)

To determine

To describe:The ruler postulate that guarantees the existence of point P between A and D .

(b)

Expert Solution

Explanation of Solution

Given information:

Consider the statements below and associated figure

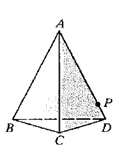
Points A, B, C and D are four non coplanar points

McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 1.5, Problem 18WE , additional homework tip 2

Formula used:

Through any 3 non collinear points there is exactly one plane

Calculation:

By Ruler Postulate, the distance between any two points equals the absolute value of the difference of coordinates. If P is between A and D , with the respective coordinates y, x , and z and assuming x < y < z

By the ruler postulate,

AP=|y−x|PD=|z−y|AD=|z−x|

But y-x is positive because x < y. By similar argument, z - y and z - x are positive.

Therefore,

AP+PD=y−x+z−y =z−xAP+PD=AD

Therefore, the assumption of P is between A and D is correct.

Hence, the answer is P between A and D is proved by ruler postulate.

Conclusion:

The answer is P between A and D is proved by ruler postulate.

(c)

To determine

To write:The postulate that guarantees the existence of BCP.

(c)

Expert Solution

Answer to Problem 18WE

The answer is postulate 7.

Explanation of Solution

Given information:

Consider the statements below and associated figure

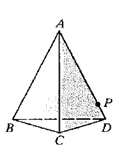
Points A, B, C and D are four non coplanar points.

McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 1.5, Problem 18WE , additional homework tip 3

Formula used:

Through any 3 non collinear points there is exactly one plane

Calculation:

From Postulate 7, through any 3 non collinear points there is exactly one plane. From the figure, points B, C and P are non collinear points therefore from above argument there will be exactly one plane through points B, C, P

Hence, the answer is postulate 7.

Conclusion:

The answer is postulate 7.

(d)

To determine

To show:how there are infinite number of planes through BC¯

(d)

Expert Solution

Answer to Problem 18WE

The infinite number of point on AD¯ to form 3 non-collinear points with B and C and postulate 7 guarantees the existence of a unique plane through each set of the three points.

Explanation of Solution

Given information:

Consider the statements below and associated figure

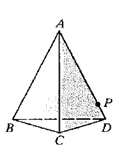
Points A, B, C and D are four non coplanar points

McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 1.5, Problem 18WE , additional homework tip 4

Formula used:

Through any 3 non collinear points there is exactly one plane

Calculation:

From the definition, a line segment consists of infinite number of points. This implies, there are an infinite number of points on AD¯ . Therefore, there are an infinite number of non collinear points to match up with B and C.

From Postulate 7, through any 3 non collinear points there is exactly one plane which implies each set of the three points forms a unique plane.

Hence, the answer to is infinite number of point on AD¯ to form 3 non-collinear points with B and C and postulate 7 guarantees the existence of a unique plane through each set of the three points.

Conclusion:

The infinite number of point on AD¯ to form 3 non-collinear points with B and C and postulate 7 guarantees the existence of a unique plane through each set of the three points.

Chapter 1.5, Problem 17WE

Chapter 1.5, Problem 19WE

Chapter 1 Solutions

McDougal Littell Jurgensen Geometry: Student Edition Geometry

Ch. 1.1 - Prob. 1CE Ch. 1.1 - Prob. 2CE Ch. 1.1 - Prob. 3CE Ch. 1.1 - Prob. 4CE Ch. 1.1 - Prob. 5CE Ch. 1.1 - Prob. 6CE Ch. 1.1 - Prob. 7CE Ch. 1.1 - Prob. 8CE Ch. 1.1 - Prob. 1WE Ch. 1.1 - Prob. 2WE

Ch. 1.1 - Prob. 3WE Ch. 1.1 - Prob. 4WE Ch. 1.1 - Prob. 5WE Ch. 1.1 - Prob. 6WE Ch. 1.1 - Prob. 7WE Ch. 1.1 - Prob. 8WE Ch. 1.1 - Prob. 9WE Ch. 1.1 - Prob. 10WE Ch. 1.2 - Prob. 1CE Ch. 1.2 - Prob. 2CE Ch. 1.2 - Prob. 3CE Ch. 1.2 - Prob. 4CE Ch. 1.2 - Prob. 5CE Ch. 1.2 - Prob. 6CE Ch. 1.2 - Prob. 7CE Ch. 1.2 - Prob. 8CE Ch. 1.2 - Prob. 9CE Ch. 1.2 - Prob. 10CE Ch. 1.2 - Prob. 11CE Ch. 1.2 - Prob. 12CE Ch. 1.2 - Prob. 13CE Ch. 1.2 - Prob. 14CE Ch. 1.2 - Prob. 15CE Ch. 1.2 - Prob. 16CE Ch. 1.2 - Prob. 17CE Ch. 1.2 - Prob. 18CE Ch. 1.2 - Prob. 19CE Ch. 1.2 - Prob. 20CE Ch. 1.2 - Prob. 1WE Ch. 1.2 - Prob. 2WE Ch. 1.2 - Prob. 3WE Ch. 1.2 - Prob. 4WE Ch. 1.2 - Prob. 5WE Ch. 1.2 - Prob. 6WE Ch. 1.2 - Prob. 7WE Ch. 1.2 - Prob. 8WE Ch. 1.2 - Prob. 9WE Ch. 1.2 - Prob. 10WE Ch. 1.2 - Prob. 11WE Ch. 1.2 - Prob. 12WE Ch. 1.2 - Prob. 13WE Ch. 1.2 - Prob. 14WE Ch. 1.2 - Prob. 15WE Ch. 1.2 - Prob. 16WE Ch. 1.2 - Prob. 17WE Ch. 1.2 - Prob. 18WE Ch. 1.2 - Prob. 19WE Ch. 1.2 - Prob. 20WE Ch. 1.2 - Prob. 21WE Ch. 1.2 - Prob. 22WE Ch. 1.2 - Prob. 23WE Ch. 1.2 - Prob. 24WE Ch. 1.2 - Prob. 25WE Ch. 1.2 - Prob. 26WE Ch. 1.2 - Prob. 27WE Ch. 1.2 - Prob. 28WE Ch. 1.2 - Prob. 29WE Ch. 1.2 - Prob. 30WE Ch. 1.2 - Prob. 31WE Ch. 1.2 - Prob. 32WE Ch. 1.2 - Prob. 33WE Ch. 1.2 - Prob. 34WE Ch. 1.2 - Prob. 35WE Ch. 1.2 - Prob. 36WE Ch. 1.2 - Prob. 1ST1 Ch. 1.2 - Prob. 2ST1 Ch. 1.2 - Prob. 3ST1 Ch. 1.2 - Prob. 4ST1 Ch. 1.2 - Prob. 5ST1 Ch. 1.2 - Prob. 6ST1 Ch. 1.2 - Prob. 7ST1 Ch. 1.2 - Prob. 1AR Ch. 1.2 - Prob. 2AR Ch. 1.2 - Prob. 3AR Ch. 1.2 - Prob. 4AR Ch. 1.2 - Prob. 5AR Ch. 1.2 - Prob. 6AR Ch. 1.2 - Prob. 7AR Ch. 1.2 - Prob. 8AR Ch. 1.2 - Prob. 9AR Ch. 1.2 - Prob. 10AR Ch. 1.2 - Prob. 11AR Ch. 1.2 - Prob. 12AR Ch. 1.2 - Prob. 13AR Ch. 1.2 - Prob. 14AR Ch. 1.2 - Prob. 15AR Ch. 1.2 - Prob. 16AR Ch. 1.2 - Prob. 17AR Ch. 1.2 - Prob. 18AR Ch. 1.2 - Prob. 19AR Ch. 1.2 - Prob. 20AR Ch. 1.2 - Prob. 21AR Ch. 1.2 - Prob. 22AR Ch. 1.2 - Prob. 23AR Ch. 1.2 - Prob. 24AR Ch. 1.2 - Prob. 25AR Ch. 1.2 - Prob. 26AR Ch. 1.2 - Prob. 27AR Ch. 1.2 - Prob. 28AR Ch. 1.2 - Prob. 29AR Ch. 1.2 - Prob. 30AR Ch. 1.2 - Prob. 31AR Ch. 1.2 - Prob. 32AR Ch. 1.2 - Prob. 33AR Ch. 1.2 - Prob. 34AR Ch. 1.2 - Prob. 35AR Ch. 1.2 - Prob. 36AR Ch. 1.2 - Prob. 37AR Ch. 1.2 - Prob. 38AR Ch. 1.2 - Prob. 39AR Ch. 1.2 - Prob. 40AR Ch. 1.2 - Prob. 41AR Ch. 1.2 - Prob. 42AR Ch. 1.2 - Prob. 43AR Ch. 1.2 - Prob. 44AR Ch. 1.2 - Prob. 45AR Ch. 1.2 - Prob. 46AR Ch. 1.3 - Prob. 1CE Ch. 1.3 - Prob. 2CE Ch. 1.3 - Prob. 3CE Ch. 1.3 - Prob. 4CE Ch. 1.3 - Prob. 5CE Ch. 1.3 - Prob. 6CE Ch. 1.3 - Prob. 7CE Ch. 1.3 - Prob. 8CE Ch. 1.3 - Prob. 9CE Ch. 1.3 - Prob. 10CE Ch. 1.3 - Prob. 11CE Ch. 1.3 - Prob. 12CE Ch. 1.3 - Prob. 13CE Ch. 1.3 - Prob. 14CE Ch. 1.3 - Prob. 15CE Ch. 1.3 - Prob. 16CE Ch. 1.3 - Prob. 17CE Ch. 1.3 - Prob. 18CE Ch. 1.3 - Prob. 19CE Ch. 1.3 - Prob. 20CE Ch. 1.3 - Prob. 21CE Ch. 1.3 - Prob. 22CE Ch. 1.3 - Prob. 23CE Ch. 1.3 - Prob. 24CE Ch. 1.3 - Prob. 25CE Ch. 1.3 - Prob. 26CE Ch. 1.3 - Prob. 1WE Ch. 1.3 - Prob. 2WE Ch. 1.3 - Prob. 3WE Ch. 1.3 - Prob. 4WE Ch. 1.3 - Prob. 5WE Ch. 1.3 - Prob. 6WE Ch. 1.3 - Prob. 7WE Ch. 1.3 - Prob. 8WE Ch. 1.3 - Prob. 9WE Ch. 1.3 - Prob. 10WE Ch. 1.3 - Prob. 11WE Ch. 1.3 - Prob. 12WE Ch. 1.3 - Prob. 13WE Ch. 1.3 - Prob. 14WE Ch. 1.3 - Prob. 15WE Ch. 1.3 - Prob. 16WE Ch. 1.3 - Prob. 17WE Ch. 1.3 - Prob. 18WE Ch. 1.3 - Prob. 19WE Ch. 1.3 - Prob. 20WE Ch. 1.3 - Prob. 21WE Ch. 1.3 - Prob. 22WE Ch. 1.3 - Prob. 23WE Ch. 1.3 - Prob. 24WE Ch. 1.3 - Prob. 25WE Ch. 1.3 - Prob. 26WE Ch. 1.3 - Prob. 27WE Ch. 1.3 - Prob. 28WE Ch. 1.3 - Prob. 29WE Ch. 1.3 - Prob. 30WE Ch. 1.3 - Prob. 31WE Ch. 1.3 - Prob. 32WE Ch. 1.3 - Prob. 33WE Ch. 1.3 - Prob. 34WE Ch. 1.3 - Prob. 35WE Ch. 1.3 - Prob. 36WE Ch. 1.3 - Prob. 37WE Ch. 1.3 - Prob. 38WE Ch. 1.3 - Prob. 39WE Ch. 1.3 - Prob. 40WE Ch. 1.3 - Prob. 41WE Ch. 1.3 - Prob. 42WE Ch. 1.3 - Prob. 43WE Ch. 1.3 - Prob. 44WE Ch. 1.3 - Prob. 45WE Ch. 1.3 - Prob. 46WE Ch. 1.3 - Prob. 47WE Ch. 1.3 - Prob. 48WE Ch. 1.4 - Prob. 1CE Ch. 1.4 - Prob. 2CE Ch. 1.4 - Prob. 3CE Ch. 1.4 - Prob. 4CE Ch. 1.4 - Prob. 5CE Ch. 1.4 - Prob. 6CE Ch. 1.4 - Prob. 7CE Ch. 1.4 - Prob. 8CE Ch. 1.4 - Prob. 9CE Ch. 1.4 - Prob. 10CE Ch. 1.4 - Prob. 11CE Ch. 1.4 - Prob. 12CE Ch. 1.4 - Prob. 13CE Ch. 1.4 - Prob. 14CE Ch. 1.4 - Prob. 15CE Ch. 1.4 - Prob. 16CE Ch. 1.4 - Prob. 17CE Ch. 1.4 - Prob. 18CE Ch. 1.4 - Prob. 19CE Ch. 1.4 - Prob. 20CE Ch. 1.4 - Prob. 21CE Ch. 1.4 - Prob. 22CE Ch. 1.4 - Prob. 23CE Ch. 1.4 - Prob. 24CE Ch. 1.4 - Prob. 25CE Ch. 1.4 - Prob. 26CE Ch. 1.4 - Prob. 27CE Ch. 1.4 - Prob. 28CE Ch. 1.4 - Prob. 29CE Ch. 1.4 - Prob. 30CE Ch. 1.4 - Prob. 31CE Ch. 1.4 - Prob. 32CE Ch. 1.4 - Prob. 33CE Ch. 1.4 - Prob. 34CE Ch. 1.4 - Prob. 35CE Ch. 1.4 - Prob. 36CE Ch. 1.4 - Prob. 37CE Ch. 1.4 - Prob. 38CE Ch. 1.4 - Prob. 1WE Ch. 1.4 - Prob. 2WE Ch. 1.4 - Prob. 3WE Ch. 1.4 - Prob. 4WE Ch. 1.4 - Prob. 5WE Ch. 1.4 - Prob. 6WE Ch. 1.4 - Prob. 7WE Ch. 1.4 - Prob. 8WE Ch. 1.4 - Prob. 9WE Ch. 1.4 - Prob. 10WE Ch. 1.4 - Prob. 11WE Ch. 1.4 - Prob. 12WE Ch. 1.4 - Prob. 13WE Ch. 1.4 - Prob. 14WE Ch. 1.4 - Prob. 15WE Ch. 1.4 - Prob. 16WE Ch. 1.4 - Prob. 17WE Ch. 1.4 - Prob. 18WE Ch. 1.4 - Prob. 19WE Ch. 1.4 - Prob. 20WE Ch. 1.4 - Prob. 21WE Ch. 1.4 - Prob. 22WE Ch. 1.4 - Prob. 23WE Ch. 1.4 - Prob. 24WE Ch. 1.4 - Prob. 25WE Ch. 1.4 - Prob. 26WE Ch. 1.4 - Prob. 27WE Ch. 1.4 - Prob. 28WE Ch. 1.4 - Prob. 29WE Ch. 1.4 - Prob. 30WE Ch. 1.4 - Prob. 31WE Ch. 1.4 - Prob. 32WE Ch. 1.4 - Prob. 33WE Ch. 1.4 - Prob. 34WE Ch. 1.4 - Prob. 35WE Ch. 1.4 - Prob. 36WE Ch. 1.5 - Prob. 1CE Ch. 1.5 - Prob. 2CE Ch. 1.5 - Prob. 3CE Ch. 1.5 - Prob. 4CE Ch. 1.5 - Prob. 5CE Ch. 1.5 - Prob. 6CE Ch. 1.5 - Prob. 7CE Ch. 1.5 - Prob. 8CE Ch. 1.5 - Prob. 9CE Ch. 1.5 - Prob. 10CE Ch. 1.5 - Prob. 11CE Ch. 1.5 - Prob. 12CE Ch. 1.5 - Prob. 13CE Ch. 1.5 - Prob. 14CE Ch. 1.5 - Prob. 15CE Ch. 1.5 - Prob. 16CE Ch. 1.5 - Prob. 1WE Ch. 1.5 - Prob. 2WE Ch. 1.5 - Prob. 3WE Ch. 1.5 - Prob. 4WE Ch. 1.5 - Prob. 5WE Ch. 1.5 - Prob. 6WE Ch. 1.5 - Prob. 7WE Ch. 1.5 - Prob. 8WE Ch. 1.5 - Prob. 9WE Ch. 1.5 - Prob. 10WE Ch. 1.5 - Prob. 11WE Ch. 1.5 - Prob. 12WE Ch. 1.5 - Prob. 13WE Ch. 1.5 - Prob. 14WE Ch. 1.5 - Prob. 15WE Ch. 1.5 - Prob. 16WE Ch. 1.5 - Prob. 17WE Ch. 1.5 - Prob. 18WE Ch. 1.5 - Prob. 19WE Ch. 1.5 - Prob. 20WE Ch. 1.5 - Prob. 1E Ch. 1.5 - Prob. 2E Ch. 1.5 - Prob. 3E Ch. 1.5 - Prob. 4E Ch. 1.5 - Prob. 5E Ch. 1.5 - Prob. 6E Ch. 1.5 - Prob. 1ST2 Ch. 1.5 - Prob. 2ST2 Ch. 1.5 - Prob. 3ST2 Ch. 1.5 - Prob. 4ST2 Ch. 1.5 - Prob. 5ST2 Ch. 1.5 - Prob. 6ST2 Ch. 1.5 - Prob. 7ST2 Ch. 1.5 - Prob. 8ST2 Ch. 1.5 - Prob. 9ST2 Ch. 1.5 - Prob. 10ST2 Ch. 1.5 - Prob. 11ST2 Ch. 1.5 - Prob. 12ST2 Ch. 1 - Prob. 1CR Ch. 1 - Prob. 2CR Ch. 1 - Prob. 3CR Ch. 1 - Prob. 4CR Ch. 1 - Prob. 5CR Ch. 1 - Prob. 6CR Ch. 1 - Prob. 7CR Ch. 1 - Prob. 8CR Ch. 1 - Prob. 9CR Ch. 1 - Prob. 10CR Ch. 1 - Prob. 11CR Ch. 1 - Prob. 12CR Ch. 1 - Prob. 13CR Ch. 1 - Prob. 14CR Ch. 1 - Prob. 15CR Ch. 1 - Prob. 16CR Ch. 1 - Prob. 17CR Ch. 1 - Prob. 18CR Ch. 1 - Prob. 19CR Ch. 1 - Prob. 20CR Ch. 1 - Prob. 1CT Ch. 1 - Prob. 2CT Ch. 1 - Prob. 3CT Ch. 1 - Prob. 4CT Ch. 1 - Prob. 5CT Ch. 1 - Prob. 6CT Ch. 1 - Prob. 7CT Ch. 1 - Prob. 8CT Ch. 1 - Prob. 9CT Ch. 1 - Prob. 10CT Ch. 1 - Prob. 11CT Ch. 1 - Prob. 12CT Ch. 1 - Prob. 13CT Ch. 1 - Prob. 14CT Ch. 1 - Prob. 15CT Ch. 1 - Prob. 16CT Ch. 1 - Prob. 17CT Ch. 1 - Prob. 18CT Ch. 1 - Prob. 19CT Ch. 1 - Prob. 20CT Ch. 1 - Prob. 21CT Ch. 1 - Prob. 22CT Ch. 1 - Prob. 23CT Ch. 1 - Prob. 24CT

Additional Math Textbook Solutions

Find more solutions based on key concepts

The blank in the given statement.

Pre-Algebra Student Edition

4. Notation What does the notation z? indicate?

Elementary Statistics (13th Edition)

In Exercises 13–16, find the margin of error for the values of c, ?, and n. 13. c = 0.95, ? = 5.2, n = 30

Elementary Statistics: Picturing the World (7th Edition)

The four flaws in the given survey.

Elementary Statistics

CHECK POINT 1 Write a word description of the set L = {a, b, c, d, e, f}.

Thinking Mathematically (6th Edition)

Each of Exercises 1–6 gives a formula for the nth term an of a sequence {an}. Find the values of a1 a2, a3 and ...

University Calculus: Early Transcendentals (4th Edition)

Knowledge Booster

$Geometry$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, geometry and related others by exploring similar questions and additional content below.