McDougal Littell Jurgensen Geometry: Student Edition Geometry

5th Edition

ISBN: 9780395977279

Author: Ray C. Jurgensen, Richard G. Brown, John W. Jurgensen

Publisher: Houghton Mifflin Company College Division

See similar textbooks

Concept explainers

Limits

When it comes to calculus, limits are considered to be a very important topic of discussion. The main importance of limit lies in expressing the value of a function as it gets closer to a certain value. Also, limits can help you to understand other topic…

Videos

Question

Chapter 1.1, Problem 3WE

To determine

To sketch: a line which shows a set of points equidistant from F and T as shown in the figure below.

Expert Solution

Explanation of Solution

Given information: Points F,T and P are shown as below in the diagram and a sketch has to be made of a line that specifies a set of points which are equidistant from F and T .

McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 1.1, Problem 3WE , additional homework tip 2

Sketch: Take a centimeter ruler and find a point midway between F and T and mark it. Now draw a line through that point perpendicular to the line joining F and T, which is the required line.

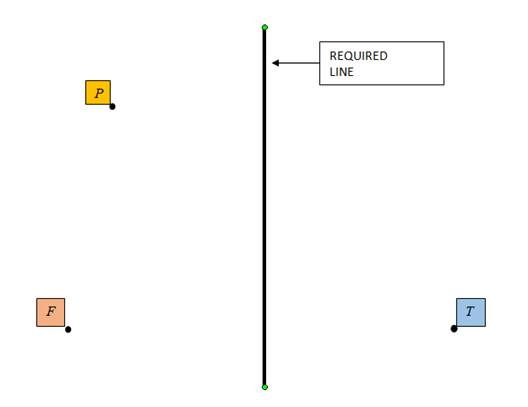
McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 1.1, Problem 3WE , additional homework tip 3

Interpretation: The required line is equidistant from points F and T which can be checked using a centimeter ruler.

To determine

To sketch: a circle with radius 2 cm with center at P either by compass or freehand as shown in the figure below.

Expert Solution

Explanation of Solution

Given information: Points F, T and P are shown as below in the diagram and a sketch has to be made of a circle whose radius is 2 cm and the center is at P as shown below.

McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 1.1, Problem 3WE , additional homework tip 5

Sketch: Use a ruler to set the distance from the point of the compass needle to the pencil's lead at 2 cm. Place the compass needle at P and rotate it about 360 degrees. The required object in the plane is a circle of radius 2 cm.

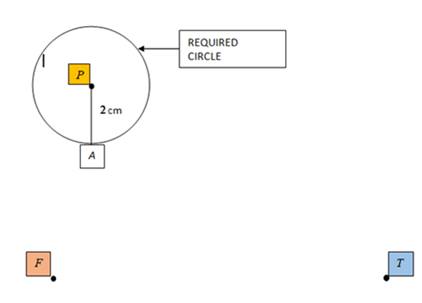
McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 1.1, Problem 3WE , additional homework tip 6

Interpretation: The circle so formed has a radius of 2 cm which can be checked by a centimeter ruler.

To determine

To sketch: the points 2 cm from P which are equidistant from F and T as shown in the figure below,

Expert Solution

Explanation of Solution

Given information: Points F,T and P are shown as below in the diagram; it is to be found that how many points are equidistant from F and T and are also 2 cm away from P.

McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 1.1, Problem 3WE , additional homework tip 8

Graph: Take a centimeter ruler and find a point midway between F and T and mark it. Now draw a line through that point perpendicular to the line joining F and T. Use a ruler to set the distance from the point of the compass needle to the pencil's lead at 2 cm. Place the compass needle at P and rotate it about 360 degrees. The required object in the plane is a circle of radius 2 cm. But it does not cross the line joining F and T even once.

So there are no point of intersection obtained as a result.

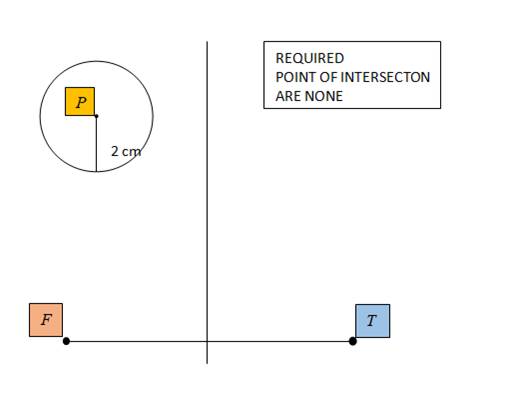
McDougal Littell Jurgensen Geometry: Student Edition Geometry, Chapter 1.1, Problem 3WE , additional homework tip 9

Interpretation: There are no points which are equidistant from F and T and 2 cm away from P.

Chapter 1.1, Problem 2WE

Chapter 1.1, Problem 4WE

Chapter 1 Solutions

McDougal Littell Jurgensen Geometry: Student Edition Geometry

Ch. 1.1 - Prob. 1CE Ch. 1.1 - Prob. 2CE Ch. 1.1 - Prob. 3CE Ch. 1.1 - Prob. 4CE Ch. 1.1 - Prob. 5CE Ch. 1.1 - Prob. 6CE Ch. 1.1 - Prob. 7CE Ch. 1.1 - Prob. 8CE Ch. 1.1 - Prob. 1WE Ch. 1.1 - Prob. 2WE

Ch. 1.1 - Prob. 3WE Ch. 1.1 - Prob. 4WE Ch. 1.1 - Prob. 5WE Ch. 1.1 - Prob. 6WE Ch. 1.1 - Prob. 7WE Ch. 1.1 - Prob. 8WE Ch. 1.1 - Prob. 9WE Ch. 1.1 - Prob. 10WE Ch. 1.2 - Prob. 1CE Ch. 1.2 - Prob. 2CE Ch. 1.2 - Prob. 3CE Ch. 1.2 - Prob. 4CE Ch. 1.2 - Prob. 5CE Ch. 1.2 - Prob. 6CE Ch. 1.2 - Prob. 7CE Ch. 1.2 - Prob. 8CE Ch. 1.2 - Prob. 9CE Ch. 1.2 - Prob. 10CE Ch. 1.2 - Prob. 11CE Ch. 1.2 - Prob. 12CE Ch. 1.2 - Prob. 13CE Ch. 1.2 - Prob. 14CE Ch. 1.2 - Prob. 15CE Ch. 1.2 - Prob. 16CE Ch. 1.2 - Prob. 17CE Ch. 1.2 - Prob. 18CE Ch. 1.2 - Prob. 19CE Ch. 1.2 - Prob. 20CE Ch. 1.2 - Prob. 1WE Ch. 1.2 - Prob. 2WE Ch. 1.2 - Prob. 3WE Ch. 1.2 - Prob. 4WE Ch. 1.2 - Prob. 5WE Ch. 1.2 - Prob. 6WE Ch. 1.2 - Prob. 7WE Ch. 1.2 - Prob. 8WE Ch. 1.2 - Prob. 9WE Ch. 1.2 - Prob. 10WE Ch. 1.2 - Prob. 11WE Ch. 1.2 - Prob. 12WE Ch. 1.2 - Prob. 13WE Ch. 1.2 - Prob. 14WE Ch. 1.2 - Prob. 15WE Ch. 1.2 - Prob. 16WE Ch. 1.2 - Prob. 17WE Ch. 1.2 - Prob. 18WE Ch. 1.2 - Prob. 19WE Ch. 1.2 - Prob. 20WE Ch. 1.2 - Prob. 21WE Ch. 1.2 - Prob. 22WE Ch. 1.2 - Prob. 23WE Ch. 1.2 - Prob. 24WE Ch. 1.2 - Prob. 25WE Ch. 1.2 - Prob. 26WE Ch. 1.2 - Prob. 27WE Ch. 1.2 - Prob. 28WE Ch. 1.2 - Prob. 29WE Ch. 1.2 - Prob. 30WE Ch. 1.2 - Prob. 31WE Ch. 1.2 - Prob. 32WE Ch. 1.2 - Prob. 33WE Ch. 1.2 - Prob. 34WE Ch. 1.2 - Prob. 35WE Ch. 1.2 - Prob. 36WE Ch. 1.2 - Prob. 1ST1 Ch. 1.2 - Prob. 2ST1 Ch. 1.2 - Prob. 3ST1 Ch. 1.2 - Prob. 4ST1 Ch. 1.2 - Prob. 5ST1 Ch. 1.2 - Prob. 6ST1 Ch. 1.2 - Prob. 7ST1 Ch. 1.2 - Prob. 1AR Ch. 1.2 - Prob. 2AR Ch. 1.2 - Prob. 3AR Ch. 1.2 - Prob. 4AR Ch. 1.2 - Prob. 5AR Ch. 1.2 - Prob. 6AR Ch. 1.2 - Prob. 7AR Ch. 1.2 - Prob. 8AR Ch. 1.2 - Prob. 9AR Ch. 1.2 - Prob. 10AR Ch. 1.2 - Prob. 11AR Ch. 1.2 - Prob. 12AR Ch. 1.2 - Prob. 13AR Ch. 1.2 - Prob. 14AR Ch. 1.2 - Prob. 15AR Ch. 1.2 - Prob. 16AR Ch. 1.2 - Prob. 17AR Ch. 1.2 - Prob. 18AR Ch. 1.2 - Prob. 19AR Ch. 1.2 - Prob. 20AR Ch. 1.2 - Prob. 21AR Ch. 1.2 - Prob. 22AR Ch. 1.2 - Prob. 23AR Ch. 1.2 - Prob. 24AR Ch. 1.2 - Prob. 25AR Ch. 1.2 - Prob. 26AR Ch. 1.2 - Prob. 27AR Ch. 1.2 - Prob. 28AR Ch. 1.2 - Prob. 29AR Ch. 1.2 - Prob. 30AR Ch. 1.2 - Prob. 31AR Ch. 1.2 - Prob. 32AR Ch. 1.2 - Prob. 33AR Ch. 1.2 - Prob. 34AR Ch. 1.2 - Prob. 35AR Ch. 1.2 - Prob. 36AR Ch. 1.2 - Prob. 37AR Ch. 1.2 - Prob. 38AR Ch. 1.2 - Prob. 39AR Ch. 1.2 - Prob. 40AR Ch. 1.2 - Prob. 41AR Ch. 1.2 - Prob. 42AR Ch. 1.2 - Prob. 43AR Ch. 1.2 - Prob. 44AR Ch. 1.2 - Prob. 45AR Ch. 1.2 - Prob. 46AR Ch. 1.3 - Prob. 1CE Ch. 1.3 - Prob. 2CE Ch. 1.3 - Prob. 3CE Ch. 1.3 - Prob. 4CE Ch. 1.3 - Prob. 5CE Ch. 1.3 - Prob. 6CE Ch. 1.3 - Prob. 7CE Ch. 1.3 - Prob. 8CE Ch. 1.3 - Prob. 9CE Ch. 1.3 - Prob. 10CE Ch. 1.3 - Prob. 11CE Ch. 1.3 - Prob. 12CE Ch. 1.3 - Prob. 13CE Ch. 1.3 - Prob. 14CE Ch. 1.3 - Prob. 15CE Ch. 1.3 - Prob. 16CE Ch. 1.3 - Prob. 17CE Ch. 1.3 - Prob. 18CE Ch. 1.3 - Prob. 19CE Ch. 1.3 - Prob. 20CE Ch. 1.3 - Prob. 21CE Ch. 1.3 - Prob. 22CE Ch. 1.3 - Prob. 23CE Ch. 1.3 - Prob. 24CE Ch. 1.3 - Prob. 25CE Ch. 1.3 - Prob. 26CE Ch. 1.3 - Prob. 1WE Ch. 1.3 - Prob. 2WE Ch. 1.3 - Prob. 3WE Ch. 1.3 - Prob. 4WE Ch. 1.3 - Prob. 5WE Ch. 1.3 - Prob. 6WE Ch. 1.3 - Prob. 7WE Ch. 1.3 - Prob. 8WE Ch. 1.3 - Prob. 9WE Ch. 1.3 - Prob. 10WE Ch. 1.3 - Prob. 11WE Ch. 1.3 - Prob. 12WE Ch. 1.3 - Prob. 13WE Ch. 1.3 - Prob. 14WE Ch. 1.3 - Prob. 15WE Ch. 1.3 - Prob. 16WE Ch. 1.3 - Prob. 17WE Ch. 1.3 - Prob. 18WE Ch. 1.3 - Prob. 19WE Ch. 1.3 - Prob. 20WE Ch. 1.3 - Prob. 21WE Ch. 1.3 - Prob. 22WE Ch. 1.3 - Prob. 23WE Ch. 1.3 - Prob. 24WE Ch. 1.3 - Prob. 25WE Ch. 1.3 - Prob. 26WE Ch. 1.3 - Prob. 27WE Ch. 1.3 - Prob. 28WE Ch. 1.3 - Prob. 29WE Ch. 1.3 - Prob. 30WE Ch. 1.3 - Prob. 31WE Ch. 1.3 - Prob. 32WE Ch. 1.3 - Prob. 33WE Ch. 1.3 - Prob. 34WE Ch. 1.3 - Prob. 35WE Ch. 1.3 - Prob. 36WE Ch. 1.3 - Prob. 37WE Ch. 1.3 - Prob. 38WE Ch. 1.3 - Prob. 39WE Ch. 1.3 - Prob. 40WE Ch. 1.3 - Prob. 41WE Ch. 1.3 - Prob. 42WE Ch. 1.3 - Prob. 43WE Ch. 1.3 - Prob. 44WE Ch. 1.3 - Prob. 45WE Ch. 1.3 - Prob. 46WE Ch. 1.3 - Prob. 47WE Ch. 1.3 - Prob. 48WE Ch. 1.4 - Prob. 1CE Ch. 1.4 - Prob. 2CE Ch. 1.4 - Prob. 3CE Ch. 1.4 - Prob. 4CE Ch. 1.4 - Prob. 5CE Ch. 1.4 - Prob. 6CE Ch. 1.4 - Prob. 7CE Ch. 1.4 - Prob. 8CE Ch. 1.4 - Prob. 9CE Ch. 1.4 - Prob. 10CE Ch. 1.4 - Prob. 11CE Ch. 1.4 - Prob. 12CE Ch. 1.4 - Prob. 13CE Ch. 1.4 - Prob. 14CE Ch. 1.4 - Prob. 15CE Ch. 1.4 - Prob. 16CE Ch. 1.4 - Prob. 17CE Ch. 1.4 - Prob. 18CE Ch. 1.4 - Prob. 19CE Ch. 1.4 - Prob. 20CE Ch. 1.4 - Prob. 21CE Ch. 1.4 - Prob. 22CE Ch. 1.4 - Prob. 23CE Ch. 1.4 - Prob. 24CE Ch. 1.4 - Prob. 25CE Ch. 1.4 - Prob. 26CE Ch. 1.4 - Prob. 27CE Ch. 1.4 - Prob. 28CE Ch. 1.4 - Prob. 29CE Ch. 1.4 - Prob. 30CE Ch. 1.4 - Prob. 31CE Ch. 1.4 - Prob. 32CE Ch. 1.4 - Prob. 33CE Ch. 1.4 - Prob. 34CE Ch. 1.4 - Prob. 35CE Ch. 1.4 - Prob. 36CE Ch. 1.4 - Prob. 37CE Ch. 1.4 - Prob. 38CE Ch. 1.4 - Prob. 1WE Ch. 1.4 - Prob. 2WE Ch. 1.4 - Prob. 3WE Ch. 1.4 - Prob. 4WE Ch. 1.4 - Prob. 5WE Ch. 1.4 - Prob. 6WE Ch. 1.4 - Prob. 7WE Ch. 1.4 - Prob. 8WE Ch. 1.4 - Prob. 9WE Ch. 1.4 - Prob. 10WE Ch. 1.4 - Prob. 11WE Ch. 1.4 - Prob. 12WE Ch. 1.4 - Prob. 13WE Ch. 1.4 - Prob. 14WE Ch. 1.4 - Prob. 15WE Ch. 1.4 - Prob. 16WE Ch. 1.4 - Prob. 17WE Ch. 1.4 - Prob. 18WE Ch. 1.4 - Prob. 19WE Ch. 1.4 - Prob. 20WE Ch. 1.4 - Prob. 21WE Ch. 1.4 - Prob. 22WE Ch. 1.4 - Prob. 23WE Ch. 1.4 - Prob. 24WE Ch. 1.4 - Prob. 25WE Ch. 1.4 - Prob. 26WE Ch. 1.4 - Prob. 27WE Ch. 1.4 - Prob. 28WE Ch. 1.4 - Prob. 29WE Ch. 1.4 - Prob. 30WE Ch. 1.4 - Prob. 31WE Ch. 1.4 - Prob. 32WE Ch. 1.4 - Prob. 33WE Ch. 1.4 - Prob. 34WE Ch. 1.4 - Prob. 35WE Ch. 1.4 - Prob. 36WE Ch. 1.5 - Prob. 1CE Ch. 1.5 - Prob. 2CE Ch. 1.5 - Prob. 3CE Ch. 1.5 - Prob. 4CE Ch. 1.5 - Prob. 5CE Ch. 1.5 - Prob. 6CE Ch. 1.5 - Prob. 7CE Ch. 1.5 - Prob. 8CE Ch. 1.5 - Prob. 9CE Ch. 1.5 - Prob. 10CE Ch. 1.5 - Prob. 11CE Ch. 1.5 - Prob. 12CE Ch. 1.5 - Prob. 13CE Ch. 1.5 - Prob. 14CE Ch. 1.5 - Prob. 15CE Ch. 1.5 - Prob. 16CE Ch. 1.5 - Prob. 1WE Ch. 1.5 - Prob. 2WE Ch. 1.5 - Prob. 3WE Ch. 1.5 - Prob. 4WE Ch. 1.5 - Prob. 5WE Ch. 1.5 - Prob. 6WE Ch. 1.5 - Prob. 7WE Ch. 1.5 - Prob. 8WE Ch. 1.5 - Prob. 9WE Ch. 1.5 - Prob. 10WE Ch. 1.5 - Prob. 11WE Ch. 1.5 - Prob. 12WE Ch. 1.5 - Prob. 13WE Ch. 1.5 - Prob. 14WE Ch. 1.5 - Prob. 15WE Ch. 1.5 - Prob. 16WE Ch. 1.5 - Prob. 17WE Ch. 1.5 - Prob. 18WE Ch. 1.5 - Prob. 19WE Ch. 1.5 - Prob. 20WE Ch. 1.5 - Prob. 1E Ch. 1.5 - Prob. 2E Ch. 1.5 - Prob. 3E Ch. 1.5 - Prob. 4E Ch. 1.5 - Prob. 5E Ch. 1.5 - Prob. 6E Ch. 1.5 - Prob. 1ST2 Ch. 1.5 - Prob. 2ST2 Ch. 1.5 - Prob. 3ST2 Ch. 1.5 - Prob. 4ST2 Ch. 1.5 - Prob. 5ST2 Ch. 1.5 - Prob. 6ST2 Ch. 1.5 - Prob. 7ST2 Ch. 1.5 - Prob. 8ST2 Ch. 1.5 - Prob. 9ST2 Ch. 1.5 - Prob. 10ST2 Ch. 1.5 - Prob. 11ST2 Ch. 1.5 - Prob. 12ST2 Ch. 1 - Prob. 1CR Ch. 1 - Prob. 2CR Ch. 1 - Prob. 3CR Ch. 1 - Prob. 4CR Ch. 1 - Prob. 5CR Ch. 1 - Prob. 6CR Ch. 1 - Prob. 7CR Ch. 1 - Prob. 8CR Ch. 1 - Prob. 9CR Ch. 1 - Prob. 10CR Ch. 1 - Prob. 11CR Ch. 1 - Prob. 12CR Ch. 1 - Prob. 13CR Ch. 1 - Prob. 14CR Ch. 1 - Prob. 15CR Ch. 1 - Prob. 16CR Ch. 1 - Prob. 17CR Ch. 1 - Prob. 18CR Ch. 1 - Prob. 19CR Ch. 1 - Prob. 20CR Ch. 1 - Prob. 1CT Ch. 1 - Prob. 2CT Ch. 1 - Prob. 3CT Ch. 1 - Prob. 4CT Ch. 1 - Prob. 5CT Ch. 1 - Prob. 6CT Ch. 1 - Prob. 7CT Ch. 1 - Prob. 8CT Ch. 1 - Prob. 9CT Ch. 1 - Prob. 10CT Ch. 1 - Prob. 11CT Ch. 1 - Prob. 12CT Ch. 1 - Prob. 13CT Ch. 1 - Prob. 14CT Ch. 1 - Prob. 15CT Ch. 1 - Prob. 16CT Ch. 1 - Prob. 17CT Ch. 1 - Prob. 18CT Ch. 1 - Prob. 19CT Ch. 1 - Prob. 20CT Ch. 1 - Prob. 21CT Ch. 1 - Prob. 22CT Ch. 1 - Prob. 23CT Ch. 1 - Prob. 24CT

Additional Math Textbook Solutions

Find more solutions based on key concepts

Constructing and Graphing Discrete Probability Distributions In Exercises 19 and 20, (a) construct a probabilit...

Elementary Statistics: Picturing the World (7th Edition)

CHECK POINT I Express as a percent.

Thinking Mathematically (6th Edition)

What is the domain and the range of y=secx ?

Precalculus

8. Accuracy of Fast Food Drive-Through Orders In a study of Burger King drive-through orders, it was found that...

Elementary Statistics (13th Edition)

In Exercises 5-36, express all probabilities as fractions. 23. Combination Lock The typical combination lock us...

Elementary Statistics

Find the point-slope form of the line passing through the given points. Use the first point as (x1, .y1). Plot ...

College Algebra with Modeling & Visualization (5th Edition)

Knowledge Booster

$Geometry$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, geometry and related others by exploring similar questions and additional content below.

Recommended textbooks for you

Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning

Elementary Geometry For College Students, 7e

Geometry

ISBN:9781337614085

Author:Alexander, Daniel C.; Koeberlein, Geralyn M.

Publisher:Cengage,

Elementary Geometry for College Students

Geometry

ISBN:9781285195698

Author:Daniel C. Alexander, Geralyn M. Koeberlein

Publisher:Cengage Learning

SEE MORE TEXTBOOKS

Limits and Continuity; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=9brk313DjV8;License: Standard YouTube License, CC-BY