Applied Statics and Strength of Materials (6th Edition)

6th Edition

ISBN: 9780133840544

Author: George F. Limbrunner, Craig D'Allaird, Leonard Spiegel

Publisher: PEARSON

See similar textbooks

Concept explainers

Slope and Deflection

The slope in a deflected beam is described as an angle in radians made by the tangent of the section with the original axis of the beam. The deflection at any point on the axis of the beam is defined as the lateral displacement of the point before and af…

Videos

Textbook Question

Chapter 15, Problem 15.35P

For Problems 15.31 through 15.43, use the moment-area method.

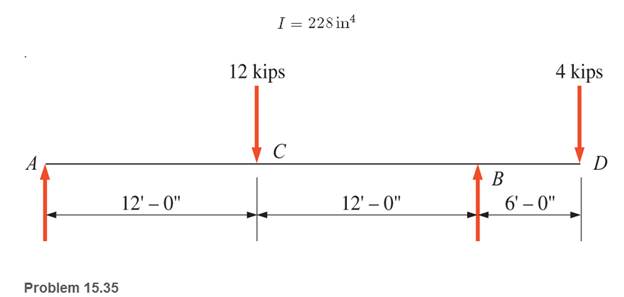
15.35 A structural steel wide-flange section is loaded as shown. Calculate the maximum deflection between the supports and the deflection of the free end. Assume that I = 228 in 4

Chapter 15, Problem 15.35P, For Problems 15.31 through 15.43, use the moment-area method. 15.35 A structural steel wide-flange

Expert Solution & Answer

Want to see the full answer?

Check out a sample textbook solution

See solution

Chapter 15, Problem 15.34P

Chapter 15, Problem 15.36P

Students have asked these similar questions

On a formatted bond paper, copy and solve the problem. Show your neat and detailed solution. Use three (3) decimal places for your answers and enclosed it in a box. Compute the deflection and slope at a section 8 ft from the wall for the beam shown in the figure using Double Integration Method. Assume that E = 28 x 103 psi and 1= 30.75 1200 lb in4. A 800 lb/ft -8 ft -8 ft B

For the beam and loading shown, use discontinuity functions to compute: (a) the deflection VA of the beam at A, and (b) the deflection Vmidspan of the beam at midspan (i.e., x = 2.45 m). Assume a constant value of El = 1270 kN-m² for the beam; M₁ = 9 kN-m, wo = 19.8 kN/m, LAB = 1.1 m, LBc = 2.7 m. MA A Answer: (a) VA = (b) Vmid i LAB i Wo B LBC mm. mm.

(use EI constant for whole span). A 10-meter-span, propped beam (fixed at the left support and roller at right support), with a uniformly distributed load from left support to six meters to the right, with a magnitude of six kilonewton per lineal meter, a downward concentrated load at the midspan. Solve the reactions at the fixed support and roller support, slope and deflection at the roller support, using Area Moment Method. Use the concentrated load as 24 kN.

Chapter 15 Solutions

Applied Statics and Strength of Materials (6th Edition)

Ch. 15 - A 14 in.-diameter aluminum rod is bent into a...Ch. 15 - 15.2 Calculate the maximum bending stress produced...Ch. 15 - A 500 -mm-long steel bar having a cross section of...Ch. 15 - 15.4 An aluminum wire has a diameter of in....Ch. 15 - 15.5 A -in.-wide by in.-thick board is bent to a...Ch. 15 - 15.6 A Douglas fir beam is in. wide and in. deep....Ch. 15 - Prob. 15.7P Ch. 15 - For Problems 15.7 through 15.14, use the formula...Ch. 15 - For Problems 15.7 through 15.14, use the formula...Ch. 15 - For Problems 15.7 through 15.14, use the formula...

Knowledge Booster

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.

For Problems 15.31 through 15.43, use the moment-area method . 15.35 A structural steel wide-flange section is loaded as shown. Calculate the maximum deflection between the supports and the deflection of the free end. Assume that I = 228 in 4

Concept explainers

Videos

Want to see the full answer?

Chapter 15 Solutions