Chapter 12.7, Problem 23HP

To determine

To draw: A cone with the given surface area.

Answer to Problem 23HP

The surface area of the cone is approximately $113.04unit{s}^2$ .

Therefore the surface area lies between 100 and 150 square units.

Explanation of Solution

Given:

Surface area is between 100 and 150 square units.

Calculation:

Consider the figure.

  

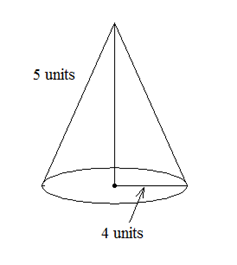
Consider a cone whose radius is 4 units and the slant height of the cone is 5 units.

The objective is to find the surface area of the cone.

The lateral area L of a cone is given by the formula

  $L=\pi rl$

Where r is the radius of the circle and l is the slant height the cone.

The surface area S of a cone is given by the formula

  $S=L+B$

WhereL is the lateral surface area and B is the area of the base that is circle.

First find the lateral area of the cone.

Put r =4 and l=5 in the lateral area formula to find the lateral area of the cone.

Thus,

  $\begin{array}{l}L=\pi rl\\ =\pi \cdot 4\cdot 5\\ =20\pi \\ \approx 20\left(3.14\right)\left[\text{Since }\pi \approx 3.14\right]\\ =62.8\end{array}$

Therefore, the lateral area of the cone is approximately $62.8unit{s}^2$ .

Next find the surface area of the cone.

First find the area B ofthe cone. The area B of the base is given by

  $\begin{array}{l}B=\pi r^2\\ =\pi {\left(4\right)}^2\\ =16\pi \\ \approx 16\left(3.14\right)\left[Since\pi \approx 3.14\right]\\ =50.24\end{array}$

Put $B\approx 50.24$ and $L\approx 62.8$ in the surface area formula to find the surface area of the cone.

  $\begin{array}{l}S=L+B\\ \approx 62.8+50.24\\ =113.04\end{array}$

Therefore, the surface area of the cone is approximately $113.04unit{s}^2$ . Therefore the surface area lies between 100 and 150 square units.

Conclusion:

Therefore, the surface area of the cone is approximately $113.04unit{s}^2$ . Therefore the surface area lies between 100 and 150 square units.