Chapter 11.8, Problem 19AYU

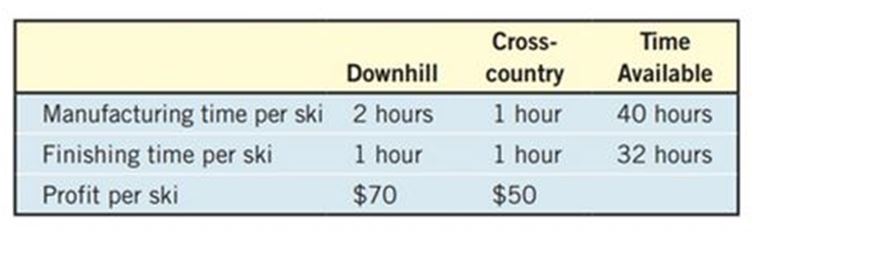
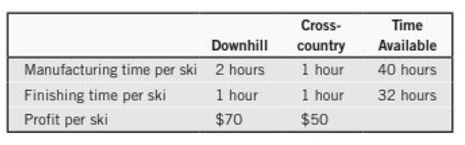
Maximizing Profit A manufacturer of skis produces two types: downhill and cross-country. Use the following table to determine how many of each kind of ski should be produced to achieve a maximum profit. What is the maximum profit? What would the maximum profit be if the time available for manufacturing were increased to 48 hours?

To determine

To solve: The given linear programming problem.

Answer to Problem 19AYU

Solution:

1. The maximum profit is 1760, when producing 8 downhill and 24 cross country skis.

2. The maximum profit is 1920, when producing 16 downhill and 16 cross country skis.

Explanation of Solution

Given:

Calculation:

Step 1:

Begin by assigning symbols for the two variables.

$x=$ Number of downhill skis produced.

$y=$ Number of cross country skis produced.

If $P$ is the profit, then

$P=70x+50y$

Step 2:

The goal is to maximize $P$ subject to certain constraints on $x$ and $y$. Because $x$ and $y$ represents number of skis produced, the only meaningful values of $x$ and $y$ are non-negative.

Therefore, $x\ge 0,y\ge 0$.

Time available for manufacturing and finishing also given. From that, we get

$2x+y\le 40$

$x+y\le 32$

Therefore, the linear programming problem may be stated as

$\text{Maximize} P=70x+50y$

Subject to

$x\ge 0$

$y\ge 0$

$2x+y\le 40$

$x+y\le 32$

Step 3:

The graph of the constraints is illustrated in the figure below.

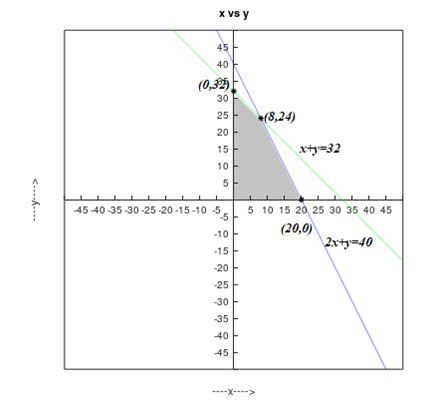
The below table lists the corner points and the corresponding values of objective function.

Corner points are	Value of objective function
$\left(20,0\right)$	1400
$\left(0,32\right)$	1600
$\left(8,24\right)$	1760

From the table,

1. The maximum profit is 1760, when producing 8 downhill and 24 cross country skis.

2. If time available for manufacturing were increased to 48 hrs, the linear programming problem becomes,

Maximize $P=70x+50y$

Subject to

$x\ge 0$

$y\ge 0$

$2x+y\le 48$

$x+y\le 32$

The graph of the constraints is illustrated in the figure below.

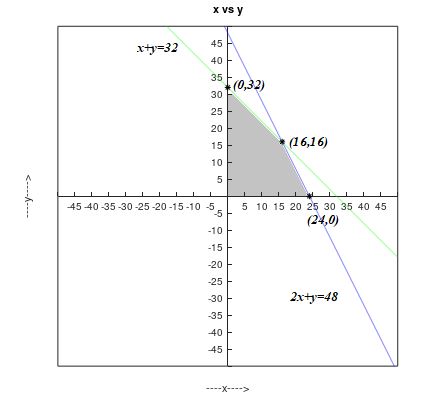
The below table lists the corner points and the corresponding values of objective function.

Corner points are	Value of objective function
$\left(24,0\right)$	1680
$\left(0,32\right)$	1600
$\left(16,16\right)$	1920

The maximum profit is 1920, when producing 16 downhill and 16 cross country skis.