Chapter 11, Problem 52P

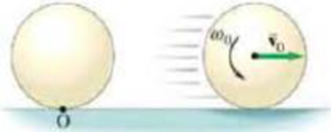
(III) On a level billiards table a cue ball, initially at rest at point O on the table, is struck so that it leaves the cue stick with a center-of-mass speed v₀ and a “reverse” spin of angular speed ω₀ (sec Fig. 11–40). A kinetic friction force acts on the ball as it initially skids across the table. (a) Explain why the ball’s angular momentum is conserved about point O. (b) Using conservation of angular momentum, find the critical angular speed ω_C, such that, if ω₀ = ω_C, kinetic friction will bring the ball to a complete (as opposed to momentary) stop. (c) If ω₀ is 10% smaller than ω_C, i.e., ω₀ = 0.90 ω_C, determine the ball’s CM velocity v_CM when it starts to roll without slipping. (d) If ω₀ is 10% larger than ω_C, i.e., ω₀ = 1.10 ω_C, determine the ball’s CM velocity v_CM when it starts to roll without slipping. [Hint: The ball possesses two types of angular momentum, the first due to the linear speed v_CM of its CM relative to point O, the second due to the spin at angular velocity ω about its own CM. The ball’s total L about O is the sum of these two angular momenta.]

FIGURE 11–40

Problem 52.