
Concept explainers
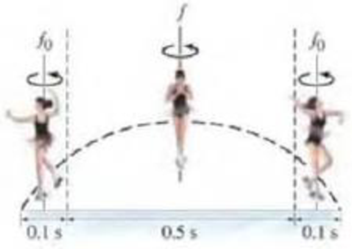
Competitive ice skaters commonly perform single, double, and triple axel jumps in which they rotate
Want to see the full answer?
Check out a sample textbook solution
Chapter 11 Solutions
Physics for Scientists and Engineers with Modern Physics
Additional Science Textbook Solutions
Concepts of Genetics (12th Edition)
Genetic Analysis: An Integrated Approach (3rd Edition)
Campbell Biology (11th Edition)
Chemistry: An Introduction to General, Organic, and Biological Chemistry (13th Edition)
Physics for Scientists and Engineers: A Strategic Approach, Vol. 1 (Chs 1-21) (4th Edition)
Organic Chemistry (8th Edition)
- A shot putter releases a shot at 13 m/s at an angle of 42 degrees to the horizontal and from a height of 1.83 m above the ground. (Note: For each question draw a diagram to show the vector/s. Show all the step and provide units in the answers. Provide answer to 2 decimal places unless stated otherwise.) Calculate and answer all parts. Only use equations PROVIDED:arrow_forward"looks" like a particle.) ...32 GO In Fig. 22-55, positive charge q = 7.81 pC is spread uni- formly along a thin nonconducting rod of length L = 14.5 cm. What are the (a) magnitude and (b) direction (relative to the positive direction of the x axis) of the electric field produced at point P, at distance R = 6.00 cm from the rod along its perpendicular bisector? R y Р + + + + + + + + +-× L Figure 22-55 Problem 32.arrow_forward1) A horizontal wire carrying current I in +x direction on the x-axis from x=0 to x=2 2) A vertical wire carrying current I upward at along the x=2 line from y=0 to y=8 3) A diagonal straight wire started at the origin and it ends at y=8 x=2 carrying a current in SE direction ( diagonally downward); y=4x In a regional magnetic field that is given in vector notation by B = ( y i - x j )/(x^2+y^2+25) As components Bx = (y+1)/x^2+y^2+25) By = (1- x )/(x^2+y^2+25) Find the integral expression for the net force for each branch carrying 5 ampere current.arrow_forward
- An electric power station that operates at 30 KV and uses a 15:1 set step-up ideal transformer is producing 400MW (Mega-Watt) of power that is to be sent to a big city with only 2.0% loss. What which is located 270 km away is the resistance of the Two wires that are being used? 52arrow_forwardSlink, from Toy Story, is a slinky dog whose middle section is a giant spring with a spring constant of 10.9 N/m. Woody, who has a mass of 0.412 kg, grabs onto the tail end of Slink and steps off the bed (as shown in figure A) with no initial velocity and reaches the floor right as his velocity hits zero again (as shown in figure C).arrow_forwardThe character Min Min from Arms was a DLC character added to Super Smash Bros. Min Min’s arms are large springs, with a spring constant of 8.53 ⋅ 10^3 N/m, which she uses to punch and fling away her opponents. Min Min pushes her spring arm against Steve, who is not moving, compressing it 1.20 m as shown in figure A. Steve has a mass of 81.6 kg. Assuming she uses only the spring to launch Steve, how fast is Steve moving when the spring is no longer compressed? As Steve goes flying away he goes over the edge of the level, as shown in figure C. What is the magnitude of Steve’s velocity when he is 2.00 m below where he started?arrow_forward
- Calculate the energy needed to melt 50 g of 0°C icearrow_forwardTwo very long line charges are set up along lines that areparallel to the z-axis, so they set up Electric fields strictly in the xy plane. One goes throughthe x-axis at x = −0.40 m and has charge a density λ1 = +12.0 μC/m, the other goesthrough the x-axis at x = +0.40 m has charge density λ2 = −8.0 μC/m.A. Find the Electric field at point A: (0.40, 0.80) (distances in meters). Give answersin unit vector notation and draw a graph of the x-y plane with the E-fields you justfound.B. Find a point on the x-axis at which the total E-field is 0.arrow_forwardIn order to increase the amount of exercise in her daily routine, Tara decides to walk up the four flights of stairs to her car instead of taking the elevator. Each of the steps she takes are 18.0 cm high, and there are 12 steps per flight. (a) If Tara has a mass of 77.0 kg, what is the change in the gravitational potential energy of the Tara-Earth system (in J) when she reaches her car? ] (b) If the human body burns 1.5 Calories (6.28 x 10³ J) for each ten steps climbed, how much energy (in J) has Tara burned during her climb? ] (c) How does the energy she burned compare to the change in the gravitational potential energy of the system? Eburned Δυarrow_forward
- A 4.40 kg steel ball is dropped onto a copper plate from a height of 10.0 m. If the ball leaves a dent 2.75 mm deep, what is the average force exerted by the plate on the ball during the impact? Narrow_forwardA block of mass m = 7.00 kg is released from rest from point and slides on the frictionless track shown in the figure below. (Assume h₂ = 7.80 m.) a m ha 3.20 m 2.00 m i (a) Determine the block's speed at points ® and point B ©. m/s m/s point (b) Determine the net work done by the gravitational force on the block as it moves from point J A to pointarrow_forwardA 1.10 x 10²-g particle is released from rest at point A on the inside of a smooth hemispherical bowl of radius R R B 2R/3 (a) Calculate its gravitational potential energy at A relative to B. ] (b) Calculate its kinetic energy at B. ] (c) Calculate its speed at B. m/s (d) Calculate its potential energy at C relative to B. J (e) Calculate its kinetic energy at C. ] = 26.5 cm (figure below).arrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





