Bundle: Principles of Physics: A Calculus-Based Text, 5th + WebAssign Printed Access Card for Serway/Jewett's Principles of Physics: A Calculus-Based Text, 5th Edition, Multi-Term
5th Edition
ISBN: 9781133422013
Author: Raymond A. Serway; John W. Jewett
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 11, Problem 21P
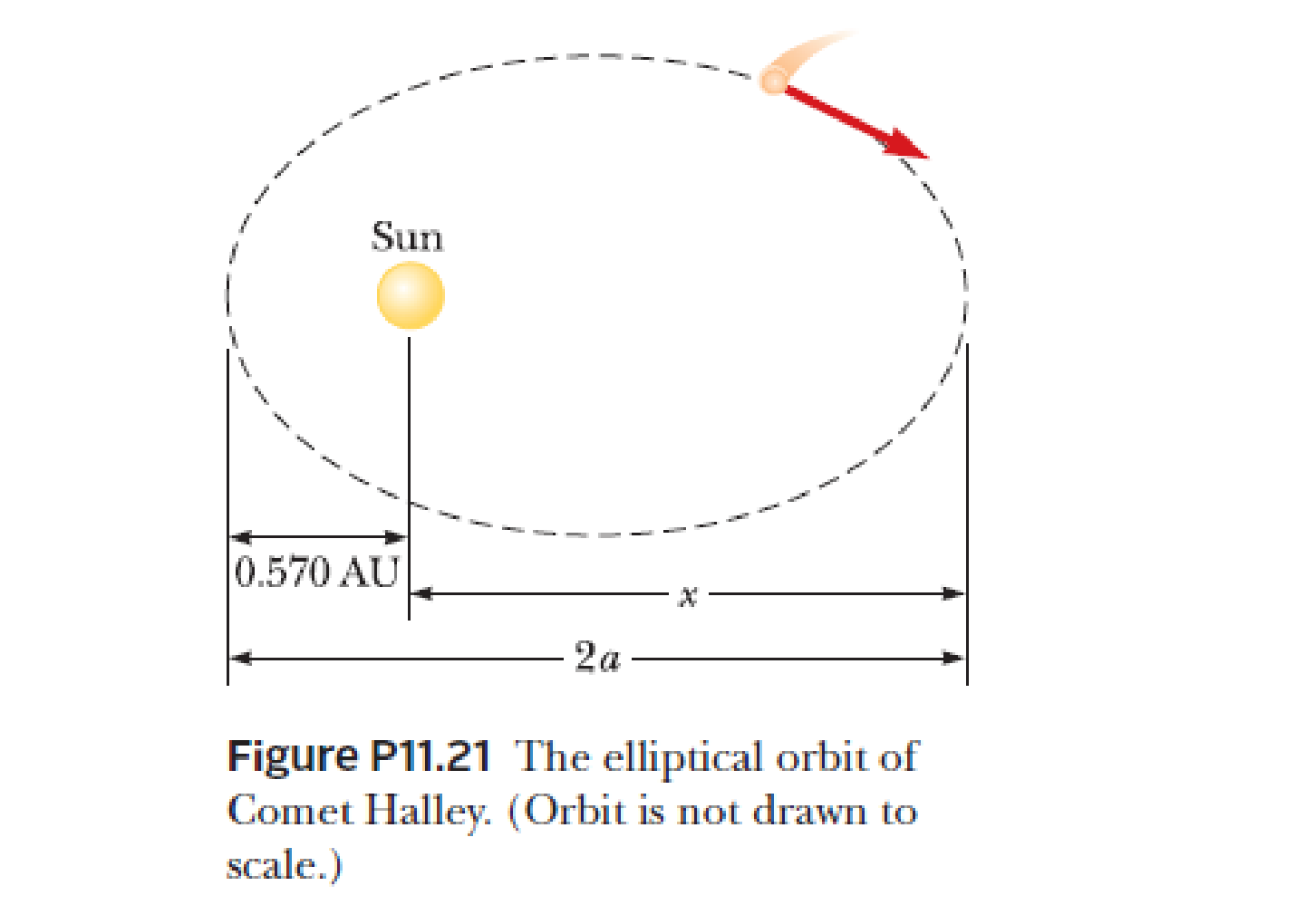
Comet Halley (Fig. P11.21) approaches the Sun to within 0.570 AU, and its orbital period is 75.6 yr. (AU is the symbol for astronomical unit, where 1 AU = 1.50 × 1011 m is the mean Earth–Sun distance.) How far from the Sun will Halley’s comet travel before it starts its return journey?
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
A comet (see the figure below) approaches the Sun to within 0.570 AU, and its orbital period is 79.6 yr. (AU is the symbol for astronomical unit, where 1 AU = 1.50 x 1011 m is the mean Earth-Sun distance.) How far from the Sun will the comet travel before it starts its return journey?
(a)
Jupiter's third-largest natural satellite, Io, follows an orbit with a semimajor axis of 422,000 km (4.22 ✕ 105 km) and a period of 1.77 Earth days (PIo = 1.77 d). To use Kepler's Third Law, we first must convert Io's orbital semimajor axis to astronomical units. One AU equals 150 million km (1 AU = 1.50 ✕ 108 km). Convert Io's a value to AU and record the result.
aIo = AU
(b)
One Earth year is about 365 days. Convert Io's orbital period to Earth years and record the result.
PIo = yr
(c)
Use the Kepler's Third Law Calculator to calculate Jupiter's mass in solar units. Record the result.
MJup(Io) = MSun
(d)
Based on this result, Jupiter's mass is about that of the Sun.
Jupiter has a similar fraction of the Sun's volume. The two objects therefore have rather similar density! In fact, Jupiter has a fairly similar composition as well: most of its mass is in the form of hydrogen and helium.
According to Lunar Laser Ranging experiment the average distance LM from the Earth to the Moon is approximately 3.82 x 105 km. The Moon orbits the
Earth and completes one revolution relative to the stars in approximately 27.5 days (a sidereal month).
Calculate the orbital velocity of the Moon in m/s.
Chapter 11 Solutions
Bundle: Principles of Physics: A Calculus-Based Text, 5th + WebAssign Printed Access Card for Serway/Jewett's Principles of Physics: A Calculus-Based Text, 5th Edition, Multi-Term
Ch. 11.1 - A planet has two moons of equal mass. Moon 1 is in...Ch. 11.3 - An asteroid is in a highly eccentric elliptical...Ch. 11.4 - Prob. 11.3QQCh. 11.5 - Prob. 11.4QQCh. 11 - Prob. 1OQCh. 11 - The gravitational force exerted on an astronaut on...Ch. 11 - Prob. 3OQCh. 11 - Prob. 4OQCh. 11 - A system consists of five particles. How many...Ch. 11 - Suppose the gravitational acceleration at the...
Ch. 11 - Prob. 7OQCh. 11 - Prob. 8OQCh. 11 - Prob. 9OQCh. 11 - Rank the following quantities of energy from...Ch. 11 - Prob. 11OQCh. 11 - Prob. 12OQCh. 11 - Prob. 13OQCh. 11 - Prob. 14OQCh. 11 - Prob. 1CQCh. 11 - Prob. 2CQCh. 11 - Prob. 3CQCh. 11 - Prob. 4CQCh. 11 - Prob. 5CQCh. 11 - Prob. 6CQCh. 11 - Prob. 7CQCh. 11 - Prob. 8CQCh. 11 - In his 1798 experiment, Cavendish was said to have...Ch. 11 - Prob. 1PCh. 11 - Prob. 2PCh. 11 - A 200-kg object and a 500-kg object are separated...Ch. 11 - Prob. 4PCh. 11 - Prob. 5PCh. 11 - Prob. 6PCh. 11 - Prob. 7PCh. 11 - Prob. 8PCh. 11 - Prob. 9PCh. 11 - Prob. 10PCh. 11 - A spacecraft in the shape of a long cylinder has a...Ch. 11 - (a) Compute the vector gravitational field at a...Ch. 11 - Prob. 13PCh. 11 - Two planets X and Y travel counterclockwise in...Ch. 11 - Prob. 15PCh. 11 - Prob. 16PCh. 11 - Prob. 17PCh. 11 - Prob. 18PCh. 11 - Plasketts binary system consists of two stars that...Ch. 11 - As thermonuclear fusion proceeds in its core, the...Ch. 11 - Comet Halley (Fig. P11.21) approaches the Sun to...Ch. 11 - Prob. 22PCh. 11 - Prob. 23PCh. 11 - Prob. 24PCh. 11 - Prob. 25PCh. 11 - A space probe is fired as a projectile from the...Ch. 11 - Prob. 27PCh. 11 - Prob. 28PCh. 11 - Prob. 29PCh. 11 - Prob. 30PCh. 11 - Prob. 31PCh. 11 - Prob. 32PCh. 11 - Prob. 33PCh. 11 - Prob. 34PCh. 11 - Prob. 35PCh. 11 - Prob. 36PCh. 11 - Prob. 37PCh. 11 - Prob. 38PCh. 11 - Prob. 39PCh. 11 - Prob. 40PCh. 11 - Prob. 41PCh. 11 - Prob. 42PCh. 11 - Prob. 43PCh. 11 - Prob. 44PCh. 11 - Prob. 45PCh. 11 - Prob. 46PCh. 11 - Let gM represent the difference in the...Ch. 11 - Prob. 48PCh. 11 - Prob. 49PCh. 11 - Two stars of masses M and m, separated by a...Ch. 11 - Prob. 51PCh. 11 - Prob. 52PCh. 11 - Prob. 53PCh. 11 - Prob. 54PCh. 11 - Prob. 55PCh. 11 - Prob. 56PCh. 11 - Prob. 57PCh. 11 - Prob. 58PCh. 11 - Prob. 59PCh. 11 - Prob. 60PCh. 11 - Prob. 61PCh. 11 - Prob. 62PCh. 11 - Prob. 63PCh. 11 - Prob. 64PCh. 11 - Prob. 65P
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- The mass of Mars is 6.42 × 10^23 kg. Its moon Phobos is 9.378 x 10^6 meters away from Mars, with a mass of 1.06 × 10^16 kg and a period of 7.66 hours. It's moon Deimos has a mass of 1.4762x10^15 kg and a period of 30.3 hours. a) Use Kepler's 3rd law to determine the orbital distance between Mars and Deimos? b) What is the tangential velocity of Phobos, using the formula v (tangential) = sqrt (G x m(central)/ r)? c) What is the gravitational force of attraction between Mars and Phobos.arrow_forwardA planet of mass 5.8 x 1024 kg orbits a star of mass 1.6 x 1030 kg in a circular orbit 10 x 1011 m from its center. Calculate the period of the orbit, in Earth years. Use G = 6.7 x 10-11 N m2/ kg2 and that one Earth year is 3.15 x 107 s. (Please answer to the fourth decimal place - i.e 14.3225)arrow_forwardA planet with mass 9.69x1023 kg orbits a star with mass 9.21x1030 kg. The orbit is circular, and the distance from the planet to the sun is 225x106 km. What is the length of a year on this planet? Give your answer in earth years (1 earth year = 31,557,600 seconds).arrow_forward
- According to Lunar Laser Ranging experiment the average distance LM from the Earth to the Moon is approximately 3.92 x 105 km. The Moon orbits the Earth and completes one revolution relative to the stars in approximately 27.5 days (a sidereal month). Calculate the orbital velocity of the Moon in m/s. Answer: Choose...arrow_forwardOn the surface of the Moon an astronaut has a weight of Fg = 190 N. The radius of the Moon is Rm = 1.74 × 106 m, the gravitational constant is G = 6.67 × 10-11 N (m/kg)2 and the mass of the Moon is Mm = 7.35 × 1022 kg. Calculate the mass of the astronaut, m, in kilograms.arrow_forwardAccording to Lunar Laser Ranging experiments the average distance L M from the Earth to the Moon is approximately 3.85 × 105 km. The Moon orbits the Earth and completes one revolution in approximately 27.5 days (a sidereal month). Calculate mass of the Eartharrow_forward
- A new planet is discovered orbiting a distant star. Observations have confirmed that the planet has a circular orbit with a radius of 12 AU and takes 117 days to orbit the star. Determine the mass of the star. State your answer with appropriate mks units. [NOTE: AU ..stands.for...astronomical unit". It is the average distance between Earth & the Sun. 1 AU≈ 1.496 x 1011 m.] Enter a number with units. I be quite large and your calculator will display the answer as a power of 10. If, as an example, your answer was 8.54 x 1056, you would type "8.54e56" into the answer box (remember to state your units with your answer).]arrow_forwardTo model a moon in the solar system, consider a sphere with radius R and uniform mass density p. Let gm = the acceleration due to gravity on the surface of the sphere. Calculate gm for these values of R and p: R = 2.0×106 m; p= 2.7x103 kg/m^3; (in m/s^2) OA: OB: 1.509 2.007 OC: 2.669 OD: 3.549 OE: OF: 4.721 6.279 OG: 8.351 OH: 1.111x101arrow_forwardAccording to Lunar Laser Ranging experiments the average distance L M from the Earth to the Moon is approximately 3.85 X 105 km. The Moon orbits the Earth and completes one revolution in approximately 27.5 days (a sidereal month). Calculate the mass of the Earth and provide your answer in units of 1024 kg. For example, if your answer is 2.7×1024 enter 2.7.arrow_forward
- The semimajor axis of Mars orbit is about 1.52 astronomical units (au), where an au is the Earth's average distance from the Sun, meaning the semimajor axis of Earth's orbit is 1 au. To go from Earth to Mars and use the least energy from rocket fuel, the orbit has a semimajor axis of 1.26 au and an eccentricity of about 0.21. Starting at Earth's orbit, to follow this path we give the spacecraft an orbital velocity of 40 km/s. Which of the following describes this best? a. It arrives at Mars orbit at the same moment that Mars is there, and must speed up to go into an orbit next to Mars or else drop back into perihelion (closest to the Sun) at Earth's orbit. b. It arrives at Mars orbit at the same moment that Mars is there, and must slow down to go into an orbit next to Mars or else drop back into perihelion (closest to the Sun) at Earth's orbit. c. It flys past Mars on its trajectory unless it is braked by accelerating toward the Sun. It which leaves Earth when Mars is nearly…arrow_forwardThe solar system has a planet with an orbital period T1b=1.51d and an orbital radius of R1b=1.6456x10^6km. Another planet in the system has an orbital radius of R1f=5.5352x10^6 km. Calculate its orbital period in days.arrow_forwardThe semimajor axis of Mars orbit is about 1.52 astronomical units (au), where an au is the Earth's average distance from the Sun, meaning the semimajor axis of Earth's orbit is 1 au. To go from Earth to Mars and use the least energy from rocket fuel, the orbit has a semimajor axis of 1.26 au and an eccentricity of about 0.21. Starting at Earth's orbit, to follow this path we give the spacecraft an orbital velocity of 40 km/s. Which of the following describes this best? It arrives at Mars orbit at the same moment that Mars is there, and must speed up to go into an orbit next to Mars or else drop back into perihelion (closest to the Sun) at Earth's orbit. It arrives at Mars orbit at the same moment that Mars is there, and must slow down to go into an orbit next to Mars or else drop back into perihelion (closest to the Sun) at Earth's orbit. It flys past Mars on its trajectory unless it is braked by accelerating toward the Sun. It which leaves Earth when…arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Kepler's Three Laws Explained; Author: PhysicsHigh;https://www.youtube.com/watch?v=kyR6EO_RMKE;License: Standard YouTube License, CC-BY