Bundle: Mechanics Of Materials, Loose-leaf Version, 9th + Mindtap Engineering, 1 Term (6 Months) Printed Access Card
9th Edition
ISBN: 9781337594318
Author: Barry J. Goodno; James M. Gere
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 10, Problem 10.4.2P
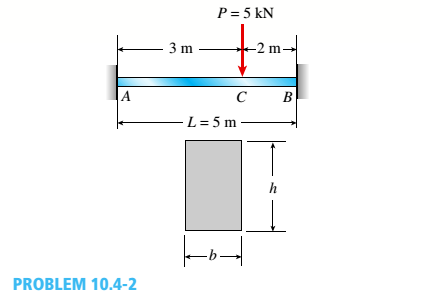
A fixed-end beam AB carries point load P acting at point C. The beam has a rectangular cross section (b = 75 mm, h = 150 mm). Calculate the reactions of the beam and the displacement at point C. Assume that E = 190 GPa.
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
Problem For the beam shown in Figure
A. Find the reaction at support B (RB)
B. Find the reaction at support D (RD)
Home Work:
1. Determine the maximum deflection d in a simply supported beam of length L
carrying a uniformly distributed load of intensity w, applied over its entire length.
2. For the beam loaded as shown in the Figure, compute the moment of area of the
M diagrams between the reactions about both the left and the right reaction. (Hint:
Draw the moment diagram by parts from right to left).
500 N
2 m
1 m
1 m
400 N/m
B
R1
R2
Please solve according to
the exporters of a typical
solution.
1
M
E*I
3. Moment Diagram by Parts
but
ds = pd0
M
de
M
ds
E*I
1
The construction of moment diagram by parts depends on two basic principles:
1) The resultant bending moment at any section caused by any load system is the
algebraic sum of the bending moment at that section caused by each load acting
separately.
= de =
%3D
E*I
ds
but
ds = dx
(Flat curve)
M
Σ
. de =
-) M. =
MR
M =
E * I
1
(M * dx
EML,EMR : Sum of the moment caused by all the forces to the left and right
section respectively.
.. 0 =
E * I
2)…
Home Work:
1. Determine the maximum deflection d in a simply supported beam of length L
carrying a uniformly distributed load of intensity w, applied over its entire length.
2. For the beam loaded as shown in the Figure, compute the moment of area of the
M diagrams between the reactions about both the left and the right reaction. (Hint:
Draw the moment diagram by parts from right to left).
500 N
2 m
1 m
1 m
400 N/m
R1
R2
Please solve according to
the exporters of a typical
solution.
1
E*I
3. Moment Diagram by Parts
but
ds = pd0
M
de
M
ds
E*I
1
The construction of moment diagram by parts depends on two basic principles:
1) The resultant bending moment at any section caused by any load system is the
= de =
%3D
E*I
ds
algebraic sum of the bending moment at that section caused by each load acting
separately.
but
ds = dx
(Flat curve)
M
=) M2 =
Σ
= OP **
E * I
MR
1
[M * dx
EM,EMR : Sum of the moment caused by all the forces to the left and right
section respectively.
:. 0 =
E * I
2) The…
Chapter 10 Solutions
Bundle: Mechanics Of Materials, Loose-leaf Version, 9th + Mindtap Engineering, 1 Term (6 Months) Printed Access Card
Ch. 10 - A propped cantilever steel beam is constructed...Ch. 10 - A fixed-end b earn is subjected to a point load at...Ch. 10 - A propped cantilever beam AB of a length L is...Ch. 10 - A fixed-end beam AB of a length L supports a...Ch. 10 - A cantilever beam AB of a length L has a fixed...Ch. 10 - A cantilever beam of a length L and loaded by a...Ch. 10 - A cantilever beam has a length L and is loaded by...Ch. 10 - A propped cantilever beam of a length L is loaded...Ch. 10 - A propped cantilever beam of a length L is loaded...Ch. 10 - A fixed-end beam of a length L is loaded by a...
Ch. 10 - A fixed-end b earn of a length L is loaded by a...Ch. 10 - A fixed-end beam of a length L is loaded by...Ch. 10 - A counterclockwise moment M0acts at the midpoint...Ch. 10 - A propped cantilever beam of a length L is loaded...Ch. 10 - A propped cantilever beam is subjected to uniform...Ch. 10 - Repeat Problem 10.3-15 using L = 3.5 m, max = 3...Ch. 10 - A two-span, continuous wood girder (E = 1700 ksi)...Ch. 10 - A fixed-end beam AB carries point load P acting at...Ch. 10 - A fixed-end beam AB supports a uniform load of...Ch. 10 - -4-4 A cantilever beam is supported at B by cable...Ch. 10 - A propped cantilever beam AB of a length L carries...Ch. 10 - A beam with a sliding support at B is loaded by a...Ch. 10 - A propped cantilever beam of a length 2L with a...Ch. 10 - The continuous frame ABC has a pin support at /l,...Ch. 10 - The continuous frame ABC has a pin support at A,...Ch. 10 - Beam AB has a pin support at A and a roller...Ch. 10 - The continuous frame ABCD has a pin support at B:...Ch. 10 - Two flat beams AB and CD, lying in horizontal...Ch. 10 - -4-13 A propped cantilever beam of a length 2L is...Ch. 10 - A propped cantilever beam of a length 2L is loaded...Ch. 10 - Determine the fixed-end moments (MAand MB) and...Ch. 10 - A continuous beam ABC wit h two unequal spans, one...Ch. 10 - Beam ABC is fixed at support A and rests (at point...Ch. 10 - A propped cantilever beam has flexural rigidity EI...Ch. 10 - A triangularly distributed 1oad with a maximum...Ch. 10 - A fixed-end beam is loaded by a uniform load q =...Ch. 10 - Uniform load q = 10 lb/ft acts over part of the...Ch. 10 - A propped cantilever beam with a length L = 4 m is...Ch. 10 - A cant i levé r b ea m i s supported by a tie rod...Ch. 10 - The figure shows a nonprismatic, propped...Ch. 10 - A beam ABC is fixed at end A and supported by beam...Ch. 10 - A three-span continuous beam A BCD with three...Ch. 10 - A beam rests on supports at A and B and is loaded...Ch. 10 - A propped cantilever beam is subjected to two...Ch. 10 - A propped cantilever beam is loaded by a...Ch. 10 - A fixed-end beam AB of a length L is subjected to...Ch. 10 - A temporary wood flume serving as a channel for...Ch. 10 - Two identical, simply supported beams AB and CD...Ch. 10 - The cantilever beam AB shown in the figure is an...Ch. 10 - The beam AB shown in the figure is simply...Ch. 10 - The continuous frame ABC has a fixed support at A,...Ch. 10 - The continuous frame ABC has a pinned support at...Ch. 10 - A wide-flange beam ABC rests on three identical...Ch. 10 - A fixed-end beam AB of a length L is subjected to...Ch. 10 - A beam supporting a uniform load of intensity q...Ch. 10 - A thin steel beam AB used in conjunction with an...Ch. 10 - Find an expression for required moment MA(in terms...Ch. 10 - Repeat Problem 10.4-41 for the loading shown in...Ch. 10 - A propped cantilever beam is loaded by two...Ch. 10 - A cable CD of a length H is attached to the third...Ch. 10 - A propped cantilever beam, fixed at the left-hand...Ch. 10 - Solve t he preceding problem by integrating the...Ch. 10 - A two-span beam with spans of lengths L and L/3 is...Ch. 10 - Solve the preceding problem by integrating the...Ch. 10 - Assume that the deflected shape of a beam AB with...Ch. 10 - (a) A simple beam AB with length L and height h...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- A fixed-end beam AB of a length L is subjected to a uniform load of intensity q acting over the middle region of the beam (sec figure). Obtain a formula for the fixed-end moments MAand MBin terms of the load q, the length L, and the length h of the loaded part of the beam. Plot a graph of the fixed-end moment MAversus the length b of the loaded part of the beam. For convenience, plot the graph in the following nondimensional form: MAqL2/l2versusbL with the ratio b/L varying between its extreme values of 0 and 1. (c) For the special case in which ù = h = L/3, draw the shear-force and bending-moment diagrams for the beam, labeling all critical ordinates.arrow_forwardCantilever beam AB carries an upward uniform load of intensity q1from x = 0 to L/2 (see Fig. a) and a downward uniform load of intensity q from x = L/2 to L. Find q1in terms of q if the resulting moment at A is zero. Draw V and M diagrams for the case of both q and qtas applied loadings. Repeat part (a) for the case of an upward triangularly distributed load with peak intensity q0(see Fig. b). For part (b), find q0, instead of q1arrow_forwardDraw the shear-force and bending-moment diagrams for a cantilever beam AB acted upon by two different load cases. A distributed load with linear variation and maximum intensity q0(see figure part a). A distributed load with parabolic variation and maximum intensity q0(see figure part b).arrow_forward
- 3. Two beams are supported as shown in the diagram below, each 150mm x 200mm x 6 meters. Beam CD is a cantilever beam carrying a uniformly distributed load of 6 KN/m freely supported on beam AB. Beam AB is freely supported on each ends. E = 13.8 GPa for both beam. Neglect the weight of the beam. a. Compute the reaction at D. b. Compute the deflection at D. c. Compute the bending stress of beam CD. 6 ka lm бт 6m 6marrow_forwardA fixed-end beam AB carries point load Pacting at point C. The beam has a rectangular crosssection (b = 75 mm, h = 150 mm). Calculate the reactionsof the beam and the displacement at point C.Assume that E 5190 GPa.arrow_forward3 The ratio of the reactions RA and RB of a simply supported beam shown in * below figure is 5 kN 2kN/m 3 kN A. 2 m - 2 m 2 m - 2 m Barrow_forward
- Find the moment support reaction about axis y for the beam shown in the picturearrow_forward2. For the simply supported beam shown below, a. Find reactions at A and B 60KN A b. Sketch the VM diagrams АА Im 40kN/m 120 kNm B 3m Im Imarrow_forwardSHOW THE FOLLOWING: 1. COMPUTATION OF REACTIONS 2. SHEAR AND MOMENT DIAGRAM 3. MAXIMUM BENDING MOMENT @ THE LOACTION OF THE MAXIMUM DEFLECTION 4. VALUE OF THE MAXIMUM DEFLECTION (A/B). A beam 7 m long is simply supported at its ends and loaded as follows: 120 kN at 1 m from one end A, 20kN at 4 m from A and 60 kN at 5 m from A. Calculate the position and magnitude of the maximum deflection. The second moment of area of the beam section is 400 x 10-m* and E for the beam material is 210 GN/m².arrow_forward
- A beam, which is fixed at one end, is acted upon by a distributed load (the curve is a continuous cubic curve) as shown in the figure. Calculate the reactions at the support. Include proper signs (with right, up and counterclockwise assumed to be positive for X, Y and the moment) 300 N/m y = kx3 %3D 300 N/m 4 m 3 m Ry (in N) = ? R,(in N) = ? M* (in N) =?arrow_forwardA beam with a solid homogeneous rectangular section is simply supported at A and B. A concentrated load F= 275 kilonewtons (kN) acts at point C where distance L1 (A to C) = 3.75 metres (m) and distance L2 (C to B)= 1.25 metres (m). The dimensions of the rectangular section of the beam are breadth, b=45 mm and depth d= 205 mm. Calculate the second moment of area for rectangular section of the beam about its centroidal x-axis (Ixxcentroid). Give your answer in mm4 and rounded to nearest mm4. 0000arrow_forwardThe overhanging beam, as shown in the Figure below, is supported by a pin at A. Identify the support reactions at A only. 8 m 400 N B 800 N·m 4 m 5 marrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Solids: Lesson 53 - Slope and Deflection of Beams Intro; Author: Jeff Hanson;https://www.youtube.com/watch?v=I7lTq68JRmY;License: Standard YouTube License, CC-BY