Principles of Heat Transfer (Activate Learning with these NEW titles from Engineering!)
8th Edition
ISBN: 9781305387102
Author: Kreith, Frank; Manglik, Raj M.
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 1, Problem 1.80P
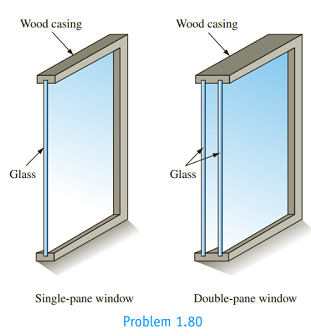
Describe and compare the modes of heat loss through the single-pane and double-pane window assemblies shown in the sketch below.
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
The 150-lb skater passes point A with a speed of 6 ft/s.
(Figure 1)
Determine his speed when he reaches point B. Neglect friction.
Determine the normal force exerted on him by the track at this point.
25 ft
B
= 4x
A
20 ft
x
A virtual experiment is designed to determine the effect of friction on the timing and speed
of packages being delivered to a conveyor belt and the normal force applied to the tube.
A package is held and then let go at the edge of a circular shaped tube of radius R = 5m.
The particle at the bottom will transfer to the conveyor belt, as shown below.
Run the simulations for μ = 0, 0.1, 0.2, 0.3, 0.4, 0.5, 0.6 and determine the time and speed at
which the package is delivered to the conveyor belt. In addition, determine the maximum
normal force and its location along the path as measured by angle 0.
Submit in hardcopy form:
(0) Free Body Diagram, equations underneath, derivations
(a) Your MATLAB mfile
(b) A table listing the values in 5 columns:
μ, T (time of transfer), V (speed of transfer), 0 (angle of max N), Nmax (max N)
(c) Based on your results, explain in one sentence what you think will happen to the
package if the friction is increased even further, e.g. μ = 0.8.
NOTE: The ODE is…
Patm = 1 bar
Piston
m = 50 kg
5 g of Air
T₁ = 600 K
P₁ = 3 bar
Stops
A 9.75 x 10-3 m²
FIGURE P3.88
Chapter 1 Solutions
Principles of Heat Transfer (Activate Learning with these NEW titles from Engineering!)
Ch. 1 - 1.1 On a cold winter day, the outer surface of a...Ch. 1 - 1.2 The weight of the insulation in a spacecraft...Ch. 1 - 1.3 A furnace wall is to be constructed of brick...Ch. 1 - 1.4 To measure thermal conductivity, two similar...Ch. 1 - To determine the thermal conductivity of a...Ch. 1 - A square silicon chip 7mm7mm in size and 0.5-mm...Ch. 1 - A cooling system is to be designed for a food...Ch. 1 - 1.80 Describe and compare the modes of heat loss...Ch. 1 - Heat is transferred at a rate of 0.1 kW through...Ch. 1 - 1.10 A heat flux meter at the outer (cold) wall of...
Ch. 1 - 1.11 Calculate the heat loss through a glass...Ch. 1 - 1.12 A wall with a thickness is made of a...Ch. 1 - 1.13 If the outer air temperature in Problem is...Ch. 1 - Using Table 1.4 as a guide, prepare a similar...Ch. 1 - 1.15 A thermocouple (0.8-mm-diameter wire) used to...Ch. 1 - Water at a temperature of 77C is to be evaporated...Ch. 1 - The heat transfer rate from hot air by convection...Ch. 1 - The heat transfer coefficient for a gas flowing...Ch. 1 - 1.19 A cryogenic fluid is stored in a...Ch. 1 - A high-speed computer is located in a...Ch. 1 - 1.21 In an experimental set up in a laboratory, a...Ch. 1 - 1.22 In order to prevent frostbite to skiers on...Ch. 1 - Using the information in Problem 1.22, estimate...Ch. 1 - Two large parallel plates with surface conditions...Ch. 1 - 1.25 A spherical vessel, 0.3 m in diameter, is...Ch. 1 - 1.26 Repeat Problem 1.25 but assume that the...Ch. 1 - Determine the rate of radiant heat emission in...Ch. 1 - 1.28 The sun has a radius of and approximates a...Ch. 1 - 1.29 A spherical interplanetary probe with a 30-cm...Ch. 1 - A spherical communications satellite, 2 m in...Ch. 1 - A long wire 0.7 mm in diameter with an emissivity...Ch. 1 - Wearing layers of clothing in cold weather is...Ch. 1 - A section of a composite wall with the dimensions...Ch. 1 - A section of a composite wall with the dimensions...Ch. 1 - Repeat Problem 1.35 but assume that instead of...Ch. 1 - 1.37 Mild steel nails were driven through a solid...Ch. 1 - Prob. 1.38PCh. 1 - 1.39 On a cold winter day, the outside wall of a...Ch. 1 - As a designer working for a major electric...Ch. 1 - 1.41 A heat exchanger wall consists of a copper...Ch. 1 - 1.43 A simple solar heater consists of a flat...Ch. 1 - A composite refrigerator wall is composed of 5 cm...Ch. 1 - An electronic device that internally generates 600...Ch. 1 - 1.47 A flat roof is modeled as a flat plate...Ch. 1 - A horizontal, 3-mm-thick flat-copper plate, 1-m...Ch. 1 - 1.49 A small oven with a surface area of is...Ch. 1 - A steam pipe 200 mm in diameter passes through a...Ch. 1 - 1.51 The inner wall of a rocket motor combustion...Ch. 1 - 1.52 A flat roof of a house absorbs a solar...Ch. 1 - Determine the power requirement of a soldering...Ch. 1 - 1.54 The soldering iron tip in Problem 1.53...Ch. 1 - Prob. 1.55PCh. 1 - A pipe carrying superheated steam in a basement at...Ch. 1 - Draw the thermal circuit for heat transfer through...Ch. 1 - 1.60 Two electric resistance heaters with a 20 cm...Ch. 1 - 1.63 Liquid oxygen (LOX) for the space shuttle is...Ch. 1 - The interior wall of a large, commercial walk-in...Ch. 1 - 1.67 In beauty salons and in homes, a ubiquitous...Ch. 1 - The heat transfer coefficient between a surface...Ch. 1 - The thermal conductivity of fibreglass insulation...Ch. 1 - 1.71 The thermal conductivity of silver at 212°F...Ch. 1 - 1.72 An ice chest (see sketch) is to constructed...Ch. 1 - Estimate the R-values for a 5-cm-thick fiberglass...Ch. 1 - A manufacturer in the United States wants to sell...Ch. 1 - Referring to Problem 1.74, how many kilograms of...Ch. 1 - 1.76 Explain a fundamental characteristic that...Ch. 1 - 1.77 Explain each in your own words. (a) What is...Ch. 1 - What are the important modes of heat transfer for...Ch. 1 - 1.79 Consider the cooling of (a) a personal...Ch. 1 - Describe and compare the modes of heat loss...Ch. 1 - A person wearing a heavy parka is standing in a...Ch. 1 - Discuss the modes of heat transfer that determine...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- Assume a Space Launch System (Figure 1(a)) that is approximated as a cantilever undamped single degree of freedom (SDOF) system with a mass at its free end (Figure 1(b)). The cantilever is assumed to be massless. Assume a wind load that is approximated with a concentrated harmonic forcing function p(t) = posin(ωt) acting on the mass. The known properties of the SDOF and the applied forcing function are given below. • Mass of SDOF: m =120 kip/g • Acceleration of gravity: g = 386 in/sec2 • Bending sectional stiffness of SDOF: EI = 1015 lbf×in2 • Height of SDOF: h = 2000 inches • Amplitude of forcing function: po = 6 kip • Forcing frequency: f = 8 Harrow_forwardAssume a Space Launch System (Figure 1(a)) that is approximated as a cantilever undamped single degree of freedom (SDOF) system with a mass at its free end (Figure 1(b)). The cantilever is assumed to be massless. Assume a wind load that is approximated with a concentrated harmonic forcing function p(t) = posin(ωt) acting on the mass. The known properties of the SDOF and the applied forcing function are given below. • Mass of SDOF: m =120 kip/g • Acceleration of gravity: g = 386 in/sec2 • Bending sectional stiffness of SDOF: EI = 1015 lbf×in2 • Height of SDOF: h = 2000 inches • Amplitude of forcing function: po = 6 kip • Forcing frequency: f = 8 Hz Figure 1: Single-degree-of-freedom system in Problem 1. Please compute the following considering the steady-state response of the SDOF system. Do not consider the transient response unless it is explicitly stated in the question. (a) The natural circular frequency and the natural period of the SDOF. (10 points) (b) The maximum displacement of…arrow_forwardAssume a Space Launch System (Figure 1(a)) that is approximated as a cantilever undamped single degree of freedom (SDOF) system with a mass at its free end (Figure 1(b)). The cantilever is assumed to be massless. Assume a wind load that is approximated with a concentrated harmonic forcing function p(t) = posin(ωt) acting on the mass. The known properties of the SDOF and the applied forcing function are given below. • Mass of SDOF: m =120 kip/g • Acceleration of gravity: g = 386 in/sec2 • Bending sectional stiffness of SDOF: EI = 1015 lbf×in2 • Height of SDOF: h = 2000 inches • Amplitude of forcing function: po = 6 kip • Forcing frequency: f = 8 Hz Figure 1: Single-degree-of-freedom system in Problem 1. Please compute the following considering the steady-state response of the SDOF system. Do not consider the transient response unless it is explicitly stated in the question. (a) The natural circular frequency and the natural period of the SDOF. (10 points) (b) The maximum displacement of…arrow_forward
- Please solve 13 * √(2675.16)² + (63.72 + 2255,03)² = 175x106 can you explain the process for getting d seperate thank youarrow_forwardIf the 300-kg drum has a center of mass at point G, determine the horizontal and vertical components of force acting at pin A and the reactions on the smooth pads C and D. The grip at B on member DAB resists both horizontal and vertical components of force at the rim of the drum. P 60 mm; 60 mm: 600 mm A E 30° B C 390 mm 100 mm D Garrow_forwardThe design of the gear-and-shaft system shown requires that steel shafts of the same diameter be used for both AB and CD. It is further required that the angle D through which end D of shaft CD rotates not exceed 1.5°. Knowing that G = 77.2 GPa, determine the required diameter of the shafts. 40 mm 400 mm 100 mm 600 mm T-1000 N-m Darrow_forward
- Assume a Space Launch System (Figure 1(a)) that is approximated as a cantilever undamped single degree of freedom (SDOF) system with a mass at its free end (Figure 1(b)). The cantilever is assumed to be massless. Assume a wind load that is approximated with a concentrated harmonic forcing function p(t) = posin(ωt) acting on the mass. The known properties of the SDOF and the applied forcing function are given below. • Mass of SDOF: m =120 kip/g • Acceleration of gravity: g = 386 in/sec2 • Bending sectional stiffness of SDOF: EI = 1015 lbf×in2 • Height of SDOF: h = 2000 inches • Amplitude of forcing function: po = 6 kip • Forcing frequency: f = 8 Hzarrow_forward13.44 The end of a cylindrical liquid cryogenic propellant tank in free space is to be protected from external (solar) radiation by placing a thin metallic shield in front of the tank. Assume the view factor Fts between the tank and the shield is unity; all surfaces are diffuse and gray, and the surroundings are at 0 K. Tank T₁ Shield, T T₁ = 100 K E1 Solar irradiation Gs ε₁ = ε₂ = 0.05 ε₁ = 0.10 Gs = 1250 W/m² E2 Find the temperature of the shield T, and the heat flux (W/m²) to the end of the tank.arrow_forwardquestion 664 thank youarrow_forward
- 13.38 Consider the attic of a home located in a hot climate. The floor of the attic is characterized by a width of L₁ = 8 m while the roof makes an angle of 0 = 30° from the horizontal direction, as shown in the schematic. The homeowner wishes to reduce the heat load to the home by adhering bright aluminum foil (ε = 0.07) onto the surfaces of the attic space. Prior to installation of the foil, the surfaces are of emissivity & = 0.90. Attic A2, 82, T2 0 = 30° A1, E1, T₁ 土 L₁ = 8 m (a) Consider installation on the bottom of the attic roof only. Determine the ratio of the radiation heat transfer after to before the installation of the foil. (b) Determine the ratio of the radiation heat transfer after to before installation if the foil is installed only on the top of the attic floor. (c) Determine the ratio of the radiation heat transfer if the foil is installed on both the roof bottom and the floor top.arrow_forward13.1 Determine F2 and F2 for the following configura- tions using the reciprocity theorem and other basic shape factor relations. Do not use tables or charts. (a) Small sphere of area A, under a concentric hemi- sphere of area A₂ = 3A₁ A₂ A1 (a) (b) Long duct. Also, what is F₁₂? A₂ Αν (b) (c) Long inclined plates (point B is directly above the center of A₁) B 100 mm A₂ - 220 mm (c) (d) Long cylinder lying on infinite plane + A₁ Az (d) (e) Hemisphere-disk arrangement -A₂, hemisphere, diameter D A₂ A₁, disk, diameter D/2 (e) (f) Long, open channel 1 m AA₂ 2 m (f) (g) Long cylinders with A₁ = 4A₁. Also, what is F₁₂? -D₁ A1 -A₂ -D2 (e) (h) Long, square rod in a long cylinder. Also, what is F22? w=D/5 18 A₁ -A2 (h) -Darrow_forward13.9 Determine the shape factor, F12, for the rectangles shown. 6 m 1 3 m 6 m 1 m 2 6 m 1 0.5 m 2 1 m (a) Perpendicular rectangles without a common edge. -1 m. (b) Parallel rectangles of unequal areas.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Principles of Heat Transfer (Activate Learning wi...Mechanical EngineeringISBN:9781305387102Author:Kreith, Frank; Manglik, Raj M.Publisher:Cengage Learning
Understanding Motor ControlsMechanical EngineeringISBN:9781337798686Author:Stephen L. HermanPublisher:Delmar Cengage Learning
Principles of Heat Transfer (Activate Learning wi...
Mechanical Engineering
ISBN:9781305387102
Author:Kreith, Frank; Manglik, Raj M.
Publisher:Cengage Learning
Understanding Motor Controls
Mechanical Engineering
ISBN:9781337798686
Author:Stephen L. Herman
Publisher:Delmar Cengage Learning
Understanding Conduction and the Heat Equation; Author: The Efficient Engineer;https://www.youtube.com/watch?v=6jQsLAqrZGQ;License: Standard youtube license