Operations Management: Sustainability and Supply Chain Management (12th Edition)
12th Edition
ISBN: 9780134130422
Author: Jay Heizer, Barry Render, Chuck Munson
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter A, Problem 1P
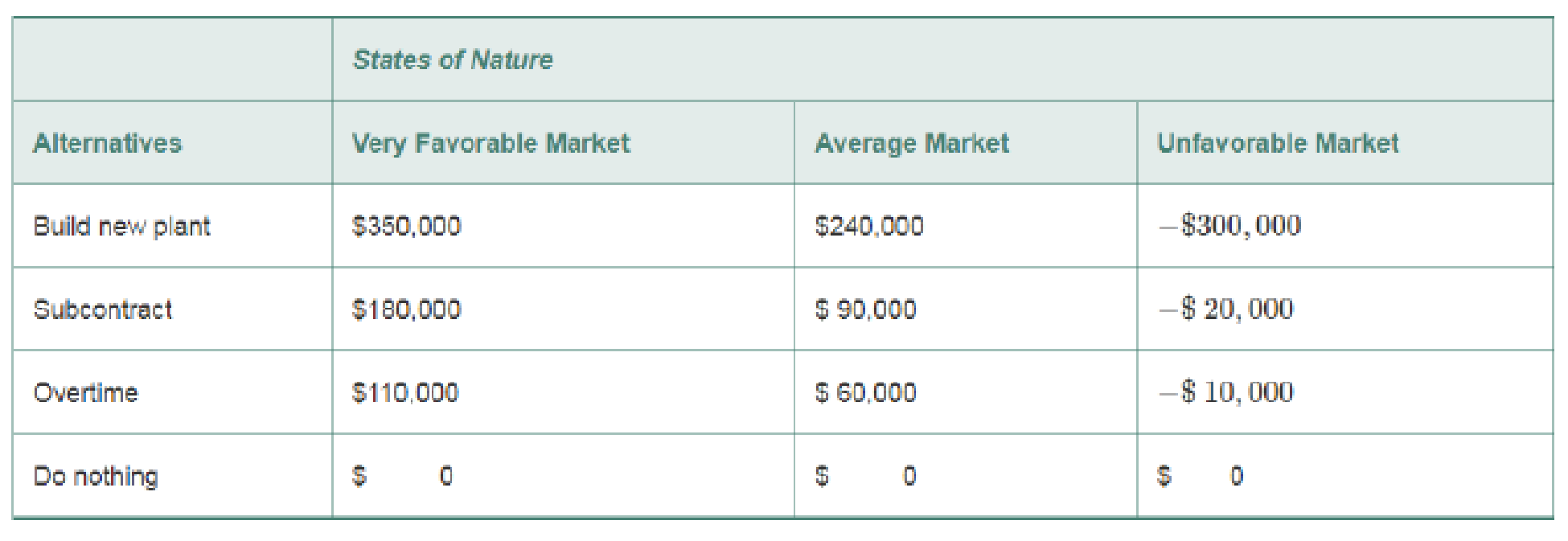
Given the following conditional value table, determine the appropriate decision under uncertainty using:
- a. Maximax
- b. Maximin
- c. Equally likely
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
3
Andrew Thomas, a sandwich vendor at Hard Rock Cafe's annual Rockfest, created a table of conditional values for the various alternatives (stocking decision) and
states of nature (size of crowd)
States of Nature (demand)
Average
$12,000
$9,000
$8,100
Big
Small
$22,000
-$2,000
Alternatives
Large Stock
Average Stock
Small Stock
$12,000
$6,000
$9,000
$4,000
The probabilities associated with the states of nature are 0.20 for a big demand, 0.45 for an average demand, and 0.35 for a small demand
a) The alternative that provides Andrew Thomas the greatest expected monetary value (EMV) is
Decision Making Under Uncertainty
In the Table, we apply the concept of Maximax choice, the Maxmin choice, and
the equally likely choice. Given the data/information in the Table, compute for the
row average. After that, determine the amount of the ROW AVERAGE that would
be adopted to be best alternative. The best alternative is the alternative with the
highest value of the row average. In this case, which option is more attractive?
Values in PhP
State of Nature
Favorable Unfavorable Maximum
Market
Minimum in
Row
Alternatives
Market
in Row
Row
Average
Project A
80,000.00
20,000.00 80,000.00
20,000.00
Project B
40,000.00
25,000.00 40,000.00
25,000.00
Project C
30,000.00 10,000.00
30,000.00 10,000.00
Project D (Do
Nothing)
Chapter A Solutions
Operations Management: Sustainability and Supply Chain Management (12th Edition)
Ch. A - Prob. 1DQCh. A - Prob. 2DQCh. A - Prob. 3DQCh. A - Prob. 4DQCh. A - Prob. 5DQCh. A - Question: 6. Explain how decision trees might be...Ch. A - Prob. 7DQCh. A - Prob. 8DQCh. A - Question 9. Identify the five steps in analyzing a...Ch. A - Prob. 10DQ
Ch. A - Question 11. The expected value criterion is...Ch. A - Question 12. When are decision trees most useful?Ch. A - Given the following conditional value table,...Ch. A - Prob. 2PCh. A - Prob. 3PCh. A - Jeffrey Helm owns a health and fitness center...Ch. A - Prob. 5PCh. A - Prob. 6PCh. A - Prob. 7PCh. A - Prob. 8PCh. A - Prob. 9PCh. A - Prob. 10PCh. A - The University of Miami bookstore stocks textbooks...Ch. A - Palmer Jam Company is a small manufacturer of...Ch. A - Prob. 13PCh. A - Prob. 14PCh. A - Prob. 21PCh. A - Prob. 22PCh. A - Prob. 23PCh. A - Prob. 24PCh. A - Prob. 25PCh. A - Prob. 26PCh. A - Philip Musa can build either a large video rental...Ch. A - Prob. 28PCh. A - Prob. 29PCh. A - Question A.24 On the opening page of Module A and...Ch. A - Question Warehouse Tenting at the Port of Miami...Ch. A - Question Warehouse Tenting at the Port of Miami...Ch. A - Question Warehouse Tenting at the Port of Miami...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, operations-management and related others by exploring similar questions and additional content below.Similar questions
- It costs a pharmaceutical company 75,000 to produce a 1000-pound batch of a drug. The average yield from a batch is unknown but the best case is 90% yield (that is, 900 pounds of good drug will be produced), the most likely case is 85% yield, and the worst case is 70% yield. The annual demand for the drug is unknown, with the best case being 20,000 pounds, the most likely case 17,500 pounds, and the worst case 10,000 pounds. The drug sells for 125 per pound and leftover amounts of the drug can be sold for 30 per pound. To maximize annual expected profit, how many batches of the drug should the company produce? You can assume that it will produce the batches only once, before demand for the drug is known.arrow_forwardPlay Things is developing a new Lady Gaga doll. The company has made the following assumptions: The doll will sell for a random number of years from 1 to 10. Each of these 10 possibilities is equally likely. At the beginning of year 1, the potential market for the doll is two million. The potential market grows by an average of 4% per year. The company is 95% sure that the growth in the potential market during any year will be between 2.5% and 5.5%. It uses a normal distribution to model this. The company believes its share of the potential market during year 1 will be at worst 30%, most likely 50%, and at best 60%. It uses a triangular distribution to model this. The variable cost of producing a doll during year 1 has a triangular distribution with parameters 15, 17, and 20. The current selling price is 45. Each year, the variable cost of producing the doll will increase by an amount that is triangularly distributed with parameters 2.5%, 3%, and 3.5%. You can assume that once this change is generated, it will be the same for each year. You can also assume that the company will change its selling price by the same percentage each year. The fixed cost of developing the doll (which is incurred right away, at time 0) has a triangular distribution with parameters 5 million, 7.5 million, and 12 million. Right now there is one competitor in the market. During each year that begins with four or fewer competitors, there is a 25% chance that a new competitor will enter the market. Year t sales (for t 1) are determined as follows. Suppose that at the end of year t 1, n competitors are present (including Play Things). Then during year t, a fraction 0.9 0.1n of the company's loyal customers (last year's purchasers) will buy a doll from Play Things this year, and a fraction 0.2 0.04n of customers currently in the market ho did not purchase a doll last year will purchase a doll from Play Things this year. Adding these two provides the mean sales for this year. Then the actual sales this year is normally distributed with this mean and standard deviation equal to 7.5% of the mean. a. Use @RISK to estimate the expected NPV of this project. b. Use the percentiles in @ RISKs output to find an interval such that you are 95% certain that the companys actual NPV will be within this interval.arrow_forwardAssume the demand for a companys drug Wozac during the current year is 50,000, and assume demand will grow at 5% a year. If the company builds a plant that can produce x units of Wozac per year, it will cost 16x. Each unit of Wozac is sold for 3. Each unit of Wozac produced incurs a variable production cost of 0.20. It costs 0.40 per year to operate a unit of capacity. Determine how large a Wozac plant the company should build to maximize its expected profit over the next 10 years.arrow_forward
- A new edition of a very popular textbook will be published a year from now. The publisher currently has 1000 copies on hand and is deciding whether to do another printing before the new edition comes out. The publisher estimates that demand for the book during the next year is governed by the probability distribution in the file P10_31.xlsx. A production run incurs a fixed cost of 15,000 plus a variable cost of 20 per book printed. Books are sold for 190 per book. Any demand that cannot be met incurs a penalty cost of 30 per book, due to loss of goodwill. Up to 1000 of any leftover books can be sold to Barnes and Noble for 45 per book. The publisher is interested in maximizing expected profit. The following print-run sizes are under consideration: 0 (no production run) to 16,000 in increments of 2000. What decision would you recommend? Use simulation with 1000 replications. For your optimal decision, the publisher can be 90% certain that the actual profit associated with remaining sales of the current edition will be between what two values?arrow_forwardThe Tinkan Company produces one-pound cans for the Canadian salmon industry. Each year the salmon spawn during a 24-hour period and must be canned immediately. Tinkan has the following agreement with the salmon industry. The company can deliver as many cans as it chooses. Then the salmon are caught. For each can by which Tinkan falls short of the salmon industrys needs, the company pays the industry a 2 penalty. Cans cost Tinkan 1 to produce and are sold by Tinkan for 2 per can. If any cans are left over, they are returned to Tinkan and the company reimburses the industry 2 for each extra can. These extra cans are put in storage for next year. Each year a can is held in storage, a carrying cost equal to 20% of the cans production cost is incurred. It is well known that the number of salmon harvested during a year is strongly related to the number of salmon harvested the previous year. In fact, using past data, Tinkan estimates that the harvest size in year t, Ht (measured in the number of cans required), is related to the harvest size in the previous year, Ht1, by the equation Ht = Ht1et where et is normally distributed with mean 1.02 and standard deviation 0.10. Tinkan plans to use the following production strategy. For some value of x, it produces enough cans at the beginning of year t to bring its inventory up to x+Ht, where Ht is the predicted harvest size in year t. Then it delivers these cans to the salmon industry. For example, if it uses x = 100,000, the predicted harvest size is 500,000 cans, and 80,000 cans are already in inventory, then Tinkan produces and delivers 520,000 cans. Given that the harvest size for the previous year was 550,000 cans, use simulation to help Tinkan develop a production strategy that maximizes its expected profit over the next 20 years. Assume that the company begins year 1 with an initial inventory of 300,000 cans.arrow_forwardThe following table is a payoff matrix associated with a farmer’s decision to purchase a pump for irrigation or to depend on the rains d) Draw up a regrets table and use it to determine which alternative will bechosen under the minimax regret decision criterion.(e) Estimate the expected value of perfect information (EVPI)arrow_forward
- Give proper and detailed explanationarrow_forwardAndrew Thomas, a sandwich vendor at hard rock café annual rockfest, created a table of conditional values for the various alternatives (stocking decision) and state of nature (size of crowd) Alternatives Large stock average stock small stock probabilities associated with states of nature are Big Big 35000 20000 10500 0.3 State of nature (demand) Average Small 15000 10000 8000 Average 0.5 determine the alternative that gives Andrew the greatest expected monetary value (EMV) compute the extpected value of perfect information (EVPI). Small -3000 5000 3000 0.2arrow_forwardAn electronics factory operates on four production lines: laptops, mobiles, desktop computers, smart boards, and there were three cases of demand (weak, medium, and high), and the matrix of returns was as follows: Laptops Mobiles Risk Desktop Computers Smart Boards High 40 45 35 30 Economic state Medium 25 15 30 20 1. The problem presented above is decision-making under Certainty Uncertainty Weak 10 5 -10 -5 Save All Answers Save and Submitarrow_forward
- Given the following conditional value table: States of Nature Very Favorable Average Unfavorable Alternatives Market Market Market Build new plant $250,000 $270,000 $180,000 $185,000 $50,000 $0 - $200,000 - $220,000 Subcontract $100,000 $0 Overtime - $12,000 Do Nothing $0 a) Using the decision making under uncertainty with the criterion of Maximax The appropriate decision will be The value of the return under this decision is $ b) Using the decision making under uncertainty with the criterion of Maximin The appropriate decision will be The value of the return under this decision is $ c) Using the decision making under uncertainty with the criterion of Equally Likely The appropriate decision will be The value of the return under this decision is $ (enter your answer as a whole number).arrow_forwardVery Favorable Average Market Unfavorable Alternatives Market Market Build new plant $250,000 $180.000 - S200,000 Subcontract $270.000 $185,000 - $220,000 Overtime S100.000 $50,000 - $12.000 Do Nothing SO SO $0 a) Using the decision making under uncertainty with the criterion of Maximax The appropriate decision will be The value of the return under this decision is $ b) Using the decision making under uncertainty with the criterion of Maximin The appropriate decision will be The value of the return under this decision is $ c) Using the decision making under uncertainty with the criterion of Equally Likely The appropriate decision will be The value of the return under this decision is $ (enter your answer as a whole number).arrow_forwardPlease do not give solution in image format thankuarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Practical Management ScienceOperations ManagementISBN:9781337406659Author:WINSTON, Wayne L.Publisher:Cengage,
Practical Management Science
Operations Management
ISBN:9781337406659
Author:WINSTON, Wayne L.
Publisher:Cengage,
Inventory Management | Concepts, Examples and Solved Problems; Author: Dr. Bharatendra Rai;https://www.youtube.com/watch?v=2n9NLZTIlz8;License: Standard YouTube License, CC-BY