Chapter 9.7, Problem 23WE

To determine

To calculate:the radius of the circle that contains the arc

Answer to Problem 23WE

The radius of the circle that contains the arc is 20.

Explanation of Solution

Given information:

The bridge’s span is 24 metres.

Midpoint of the arc is 4 meter higher than the endpoints.

Calculation:

Consider the image:

  

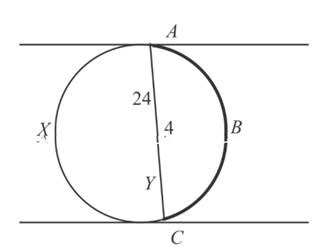
Arc $\widehat{ABC}$ is the bridge over the river with $B$ as the centre of arc $\widehat{ABC}$

The bridge’s height is 24 metres.

Therefore $AC=24$

The arc's midpoint is 4 m higher than the endpoints.

Therefore, $BY=4$

Also $Y$ is the midpoint of $AC$

Consider the following theorem

When two chords meet inside a circle, the segment product of one chord is equal to the segment product of the other chord.

  $XY\cdot YB=AY\cdot YC$

Since, $Y$ is the midpoint of $AC$

Therefore, $YB=4$

  $XY\cdot 4=12\cdot 12$

  $XY\cdot 4=144$

  $XY=36$

Now Solving the below:

  $\begin{array}{l}XB=XY+YB\\ =36+4\\ =40\end{array}$

Since, $XB$ is the diameter of the circle and radius is half the diameter

Therefore, the radius of the circle that contains the arc is 20.