
Concept explainers
To Find: The measure of arc by using trigonometry.
Answer to Problem 21WE
The measure of arc is approx.
Explanation of Solution
Given information:
The
Radius of the circle is 10 cm.
Consider the figure as below:
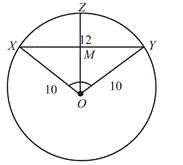
In above figure circle contain chords
Radius of the circle OX= OY=10
A line segment OM is a perpendicular segment from O to chord XY and it bisects NA XOY into two equal parts which means NA XOZ= NA YOZ .
In circle M is the midpoint of the chord XY which means
If
To find the measure of angle
Use trigonometric identity:
NA in form right triangle ΔXMO
Here perpendicular
Here,
But angle
This means the angle is:
Here measure of
If
According to theorem, In the same circle if two minor arc are congruent only when their central angles are congruent.
Therefore, measure of arc is approx. 74° .
Chapter 9 Solutions
McDougal Littell Jurgensen Geometry: Student Edition Geometry
Additional Math Textbook Solutions
Elementary Statistics (13th Edition)
University Calculus: Early Transcendentals (4th Edition)
Calculus: Early Transcendentals (2nd Edition)
College Algebra with Modeling & Visualization (5th Edition)
- Door 87.5in to 47 living 44.75 Closet 96in Window ISS.Sin 48in Train Table 96in 48in 132:2 Windowarrow_forward39 Two sides of one triangle are congruent to two sides of a second triangle, and the included angles are supplementary. The area of one triangle is 41. Can the area of the second triangle be found?arrow_forwardA parallelogram with an area of 211.41 m^2 hast a base Thatcher measures 24.3m. Find ist height.arrow_forward
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning

