MECHANICS OF MATERIALS
11th Edition
ISBN: 9780137605521
Author: HIBBELER
Publisher: RENT PEARS
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 9.4, Problem 8FP
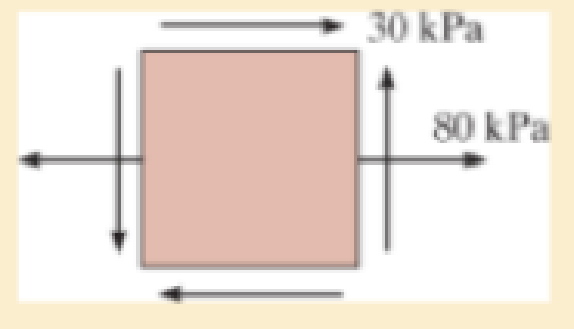
Also, find the corresponding orientation of the element with respect to the element shown.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Find the damping coefficient of system given below. Use k=20kN/m, c=100 N-s/m
and m=10kg.
122222
Je
0.3 m
0.3 m
0.6m
0.0402
1
b
0.056
C
0.556
PLEASE SOLVE STEP BY STEP WITHOUT ARTIFICIAL INTELLIGENCE OR CHATGPT
SOLVE BY HAND STEP BY STEP
PLEASE SOLVE STEP BY STEP WITHOUT ARTIFICIAL INTELLIGENCE OR CHATGPT
SOLVE BY HAND STEP BY STEP
Chapter 9 Solutions
MECHANICS OF MATERIALS
Ch. 9.3 - Determine the normal stress and shear stress...Ch. 9.3 - Determine the equivalent state of stress on an...Ch. 9.3 - Also, find the corresponding orientation of the...Ch. 9.3 - Determine the equivalent state of stress on an...Ch. 9.3 - Determine the maximum principal stress at point B.Ch. 9.3 - Determine the principal stress at point C.Ch. 9.3 - Determine the stress components acting on the...Ch. 9.3 - Solve Prob.99 using the stress transformation...Ch. 9.3 - Determine the stress components acting on the...Ch. 9.3 - Determine the equivalent state of stress on an...
Ch. 9.3 - The stress along two planes at a point is...Ch. 9.3 - The state of stress at a point in a member is...Ch. 9.3 - The wood beam is subjected to a load of 12 kN. If...Ch. 9.3 - The internal loadings at a section of the beam are...Ch. 9.3 - Solve Prob.925 for point B. 925. The internal...Ch. 9.3 - Solve Prob.925 for point C. 925. The internal...Ch. 9.3 - It is subjected to a torque of 12 kip in. and a...Ch. 9.3 - A paper tube is formed by rolling a cardboard...Ch. 9.3 - Solve Prob.931 for the normal stress acting...Ch. 9.3 - Determine the principal stresses in the...Ch. 9.3 - The shaft has a diameter d and is subjected to the...Ch. 9.4 - Use Mohrs circle to determine the normal stress...Ch. 9.4 - Also, find the corresponding orientation of the...Ch. 9.4 - Draw Mohrs circle and determine the principal...Ch. 9.4 - Determine the principal stresses at a point on the...Ch. 9.4 - Determine the principal stresses at point A on the...Ch. 9.4 - Point A is just below the flange.Ch. 9.4 - Mohrs circle for the state of stress is shown in...Ch. 9.4 - Determine (a) the principal stresses and (b) the...Ch. 9.4 - Determine the equivalent state of stress if an...Ch. 9.4 - Draw Mohrs circle that describes each of the...Ch. 9.4 - Draw Mohrs circle trial describes each of the...Ch. 9.4 - Determine (a) the principal stresses and (b) the...Ch. 9.4 - Determine (a) the principal stresses and (b) the...Ch. 9.4 - Draw Mohrs circle that describes each of the...Ch. 9.4 - The grains of wood in the board make an angle of...Ch. 9.4 - A spherical pressure vessel has an inner radius of...Ch. 9.4 - The cylindrical pressure vessel has an inner...Ch. 9.4 - If the box wrench is subjected to the 50 lb force,...Ch. 9.4 - If the box wrench is subjected to the 50-lb force,...Ch. 9.5 - Draw the three Mohrs circles that describe each of...Ch. 9.5 - Draw the three Mohrs circles that describe the...Ch. 9.5 - Determine the principal stresses and the absolute...Ch. 9.5 - The solid shaft is subjected to a torque, bending...Ch. 9.5 - The frame is subjected to a horizontal force and...Ch. 9 - Prob. 1RPCh. 9 - The steel pipe has an inner diameter of 2.75 in....Ch. 9 - Determine the equivalent state of stress If an...Ch. 9 - The crane is used to support the 350-lb load....Ch. 9 - Determine the equivalent state of stress on an...Ch. 9 - The propeller shaft of the tugboat is subjected to...Ch. 9 - Determine the principal stresses in the box beam...Ch. 9 - Determine (a) the principal stresses and (b) the...Ch. 9 - Determine the stress components acting on the...
Additional Engineering Textbook Solutions
Find more solutions based on key concepts
How is the hydrodynamic entry length defined for flow in a pipe? Is the entry length longer in laminar or turbu...
Fluid Mechanics: Fundamentals and Applications
17–1C A high-speed aircraft is cruising in still air. How does the temperature of air at the nose of the aircra...
Thermodynamics: An Engineering Approach
How are relationships between tables expressed in a relational database?
Modern Database Management
Why is the study of database technology important?
Database Concepts (8th Edition)
Write a summary list of the problem-solving steps identified in the chapter, using your own words.
BASIC BIOMECHANICS
What types of coolant are used in vehicles?
Automotive Technology: Principles, Diagnosis, And Service (6th Edition) (halderman Automotive Series)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- PLEASE SOLVE STEP BY STEP WITHOUT ARTIFICIAL INTELLIGENCE OR CHATGPT SOLVE BY HAND STEP BY STEParrow_forwardConsider the bar, shown in Figure 1 that undergoes axial displacement due to both a distributed load and a point force. The bar is of cross-sectional area A = 1.10-3 m², and has a modulus of elasticity E = 100 GPa. 1(x) = 5 kN/m x=0.0 x=2.0 2.0m 10 kN Figure 1: Bar domain with varying distributed forces. a) The general form of the governing equations describing the bar's displacement, u(x), is given by, d (AE du(x)) -) +1(x) = 0. d.x dx What are the accompanying boundary conditions for this bar? b) Using the mesh in Figure 2, form the basis functions associated with element 2 and write the FEM approximation over the element. 1 2 3 1 2 1m 1m Figure 2: Mesh of 2 elements. Elements are numbered with underlines. c) The general form of the element stiffness matrix system, with nodes indexed by i and j, is, AE Uj N;(x)l(x)dx – Ng(0)f(0) ¥ [4]}]{{}}={{{}\(\\+} + {N(2)f(2) = N (0)5() }, (1) 0, respectively. L = (2) where f(2) and f(0) denote the boundary forces at positions x 2 and x Evaluate…arrow_forwardanswer pleasearrow_forward
- amination) Question 1 Consider the bar, shown in Figure 1, that undergoes axial displacement due to both a distributed load and a point force. The bar is of cross-sectional area A = 1.103 m2, and has a modulus of elasticity E = 100 GPa. 1(x) = 5 kN/m 10 kN X x=0.0 x=2.0 2.0m Figure 1: Bar domain with varying distributed forces. a) The general form of the governing equations describing the bar's displacement, u(x), is given by, d (AE du(x)) + 1(x) = 0. dx dx What are the accompanying boundary conditions for this bar? MacBook Air a 会 DII F5 F6 F7 F8 80 F3 F4 0/ 20 [8 marksl 8 FOarrow_forwardAnswer Barrow_forwardfem helpUsing the mesh in Figure 2, form the basis functions associated with element 2 and write the FEMapproximation over the element.arrow_forward
- Answer carrow_forwardshow workingarrow_forwardCFD help Figure 3: Advection equation, solution for three different timesteps. Q1) Provide an explanation what conditions and numerical setup could explain the curves. Identify which of the three curves is the first, second and third timestep.arrow_forward
- answer pleasearrow_forwardFigure 3 shows the numerical solution of the advection equation for a scalar u along x at three consecutive timesteps. 1.0 0.8- 0.6 0.4- 0.2 0.0 00 -0.2 -0.4 -0.6- 3.0 3.5 4.0 4.5 5.0 5.5 6.0 6.5 Figure 3: Advection equation, solution for three different timesteps.arrow_forwardQuestion 2 Figure 3 shows the numerical solution of the advection equation for a scalar u along x at three consecutive timesteps. 1.0 0.8- 0.6- 0.4- 0.2- 0.0- -0.2- -0.4- -0.6 3.0 3.5 4.0 4.5 5.0 5.5 6.0 6.5 Figure 3: Advection equation, solution for three different timesteps. a) Provide an explanation what conditions and numerical setup could explain the curves. Identify which of the three curves is the first, second and third timestep. b) Consider explicit schemes with central and upwind discretisations. Explain how each of these candidate discretisations could produce the behaviour shown in Figure 3. c) Determine the CFL number that was used in the simulation for each of the candidate schemes for all possible updates. Assume that the timestep and mesh-width used are constant. Read the data to two digits of accuracy from Figure 4 shown at the end of the question, which is an enlarged version of Figure 3. Demonstrate your method and input data for one calculation, but then use a…arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
International Edition---engineering Mechanics: St...
Mechanical Engineering
ISBN:9781305501607
Author:Andrew Pytel And Jaan Kiusalaas
Publisher:CENGAGE L
Dislocations and Plastic Deformation; Author: LearnChemE;https://www.youtube.com/watch?v=cpvTwYAUeA8;License: Standard Youtube License