
A 15° wedge is forced under a 50-kg pipe as shown. The coefficient of static friction at all surfaces is 0.20. (a) Show that slipping will occur between the pipe and the vertical wall. (b) Determine the force P required to move the wedge.
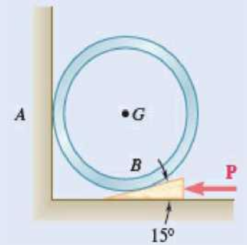
Fig. P8.64 and P8.65
(a)
Show that the slipping will occur between the pipe and the vertical wall.
Explanation of Solution
Given information:
The mass of the pipe is
The value of angle
The coefficient of static friction at all surfaces is
Calculation:
Find the weight (W) of the pipe using the relation.
Here, the acceleration due to gravity is g.
Consider the acceleration due to gravity is
Substitute 50 kg for m and
Show the free-body diagram of the pipe as in Figure 1.
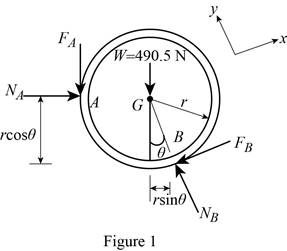
Find the friction force at point A using the relation.
Here, the normal force at point A is
Find the normal force at point A by taking moment about point B.
Substitute 490.5 N for W, 0.20 for
Find the friction force at point B
Substitute 490.5 N for W, 0.20 for
Find the normal force at point B
Substitute 490.5 N for W, 0.20 for
Find the maximum friction force at point B using the relation.
Substitute 0.20 for
The friction force at point B is less than the maximum friction force at point B.
Therefore, the slipping will not occur at point B and the slipping will occur between the pipe and the vertical wall.
(b)
Find the force P required to move the wedge.
Answer to Problem 8.64P
The force P required to move the wedge is
Explanation of Solution
Given information:
The mass of the pipe is
The value of angle
The coefficient of static friction at all surfaces is
Calculation:
Show the free-body diagram of the wedge as in Figure 2.
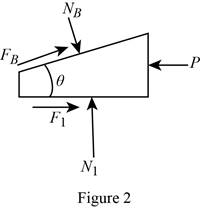
Find the normal force
Substitute 554.155 N for
Find the force P by resolving the horizontal component of forces.
Substitute 554.155 N for
Therefore, the force P required to move the wedge is
Want to see more full solutions like this?
Chapter 8 Solutions
VECTOR MECH...,STAT.+DYNA.(LL)-W/ACCESS
- Q1: For the following force system, find the moments with respect to axes x, y, and zarrow_forwardQ10) Body A weighs 600 lb contact with smooth surfaces at D and E. Determine the tension in the cord and the forces acting on C on member BD, also calculate the reaction at B and F. Cable 6' 3' wwwarrow_forwardHelp ارجو مساعدتي في حل هذا السؤالarrow_forward
- Q3: Find the resultant of the force system.arrow_forwardQuestion 1 A three-blade propeller of a diameter of 2 m has an activity factor AF of 200 and its ratio of static thrust coefficient to static torque coefficient is 10. The propeller's integrated lift coefficient is 0.3.arrow_forward(L=6847 mm, q = 5331 N/mm, M = 1408549 N.mm, and El = 8.6 x 1014 N. mm²) X A ΕΙ B L Y Marrow_forward
- Calculate the maximum shear stress Tmax at the selected element within the wall (Fig. Q3) if T = 26.7 KN.m, P = 23.6 MPa, t = 2.2 mm, R = 2 m. The following choices are provided in units of MPa and rounded to three decimal places. Select one: ○ 1.2681.818 O 2. 25745.455 O 3. 17163.636 O 4. 10727.273 ○ 5.5363.636arrow_forwardIf L-719.01 mm, = 7839.63 N/m³, the normal stress σ caused by self-weight at the location of the maximum normal stress in the bar can be calculated as (Please select the correct value of σ given in Pa and rounded to three decimal places.) Select one: ○ 1. 1409.193 2. 845.516 O 3. 11273.545 ○ 4.8455.159 ○ 5.4509.418 6. 2818.386 7.5636.772arrow_forwardTo calculate the rotation at Point B, a suitable virtual structure needs to be created. Which equation in the following choices most accurately represents the functional relationship between the bending moment, Mv2 ( Units: N.mm), of the virtual structure and the spatial coordinate x (Units: mm) if the applied unit virtual moment is clockwise? Select one: O 1. Mv2 1.000 O 2. Mv2=x+1.000 O 3. Mv2=x+0.000 4. Mv2 = -x-1.000 O 5. Mv2 -1.000 6. Mv2=-x+0.000arrow_forward
 International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
