Chapter 8, Problem 36P

8-33 to 8-36 The figure illustrates the non-permanent connection of a steel cylinder head to a grade 30 cast- iron pressure vessel using N bolts. A confined gasket seal has an effective sealing diameter D. The cylinder stores gas at a maximum pressure p_g. For the specifications given in the table for the specific problem assigned, select a suitable bolt length from the preferred sizes in Table A-17, then determine the yielding factor of safety n_p, the load factor n_L, and the joint separation factor n₀.

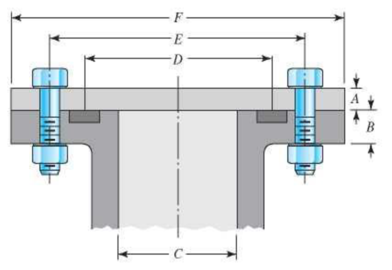
Problem Number	8-33	8-34	8-35	8-36
A	20 mm	$\frac{1}{2}$ in	20 mm	$\frac{3}{8}$ in
B	20 mm	$\frac{5}{8}$ in	25 mm	$\frac{1}{2}$ in
C	100 mm	3.5 in	0.8 m	3.25 in
D	150 mm	4.25 in	0.9 m	3.5 in
E	200 mm	6 in	1.0 m	5.5 in
F	300 mm	8 in	1.1 m	7 in
N	10	10	36	8
pg	6 MPa	1500 psi	550 kPa	1200 psi
Bolt grade	ISO 9.8	SAE 5	ISO 10.9	SAE 8
Bolt spec.	M12 × 1.75	$\frac{1}{2}$ in-13	M10 × 1.5	$\frac{7}{16}$ in-14

To determine

The yield factor of safety.

The load factor of safety.

The joint separation factor.

Answer to Problem 36P

The yield factor of safety is $1.28$.

The load factor of safety is $8.80$.

The joint separation factor is $10.9$.

Explanation of Solution

Write the expression of the length of the material squeeze between the bolt face and washer face.

$l=A+B$ (I)

Here the length of the material squeeze between the bolt face and washer face is $l$, and thickness of pressure vessel  inside the bolt is $A$, the thickness of the cylinder inside the bolt is $B$.

Write the expression for the length of the bolt.

$L\ge l+H$ (II)

Here the length of bolt is $L$, length of the material squeeze between the bolt face and washer face is $l$ and the thickness of the bolt is $H$.

Write the expression of the threaded length for hexagonal bolt.

$L_T=2d+0.25$ (III)

Here the threaded length is $L_T$.

Write the expression of the length of the unthreaded portion in grip.

$l_d=L-L_T$                                          (IV)

Here, the length of the unthreaded portion in the grip is $l_d$.

Write the expression of the length of the threaded portion in grip.

$l_t=l-l_d$                                                (V)

Here, the length of threaded portion in the grip is $l_t$.

Write the expression of the major area diameter.

$A_d=\frac{\pi }{4}{d}^2$ (VI)

Here the nominal diameter of the bolt is $d$.

Write the expression of the stiffness for the bolt.

$k_b=\frac{A_d{A}_t{E}_s}{A_d{l}_t+A_t{l}_d}$ . (VII)

Here the bolt stiffness is $k_b$, major area diameter of the fastener is $A_d$, tensile stress area is $A_t$, length of threaded portion is $l_t$, length of unthreaded portion is $l_d$ and young’s modulus of elasticity of $E_s$.

Write the expression of stiffness for the steel cylinder.

$k_1=\frac{0.5774\pi E_{s}d}{\ln \frac{\left(1.155t+D-d\right)\left(D+d\right)}{\left(1.155t+D+d\right)\left(D-d\right)}}$ (VIII)

Here the stiffness of the steel cylinder is $k_1$, young’s modulus for steel is $E_s$, effective sealing diameter of the gasket sealing is $D$, thickness of steel cylinder head is $t$.

Write the expression for the midpoint of the complete joint.

$t_o=\frac{A+B}{2}$ (IX)

Here, the midpoint of the joint is $t_o$, the thickness of the vessel inside the bolt if $A$ and thickness of the cylinder inside the bolt is $B$.

Write the expression of the thickness of the upper frustum.

$t_1=t_o-A$ . (X)

Here, the thickness of upper frustum of the gasket is $t_1$.

Write the expression for the effective sealing diameter of the gasket sealing in upper frustum.

$D_1=D+2A\tan \alpha$ (XI)

Here, the effective sealing diameter of upper frustum of the gasket sealing is $D_1$ semi-angle frustum of cone is $\alpha$.

Write the expression for the stiffness of the upper frustum of cast iron vessel.

$k_2=\frac{0.5774\pi E_{c}d}{\ln \frac{\left(1.155t_1+D_1-d\right)\left(D_1+d\right)}{\left(1.155t_1+D_1+d\right)\left(D_1-d\right)}}$ (XII)

Here, the stiffness of the cast-iron pressure vessel in the upper frustum is $k_2$ and the young’s modulus of cast iron is $E_c$.

Write the expression for the stiffness of the lower frustum of the cast iron vessel.

$k_3=\frac{0.5774\pi E_{c}d}{\ln \frac{\left(1.155t+D-d\right)\left(D+d\right)}{\left(1.155t+D+d\right)\left(D-d\right)}}$ (XIII)

Here, the stiffness of the cast-iron pressure vessel in the lower frustum is $k_3$.

Write the expression for the stiffness of the member or assembly.

$k_m={\left[\frac{1}{k_1}+\frac{1}{k_2}+\frac{1}{k_3}\right]}^{-1}$ (XIV)

Here, the stiffness of the member is $k_m$.

Write the expression of joint constant.

$C=\frac{k_b}{k_b+k_m}$ (XV)

Here the joint constant is $C$.

Write the expression of initial tension in the bolt.

$F_i=0.75A_t{S}_p$ (XVI)

Here the tensile stress area is $A_t$ and the proof strength of material of the bolt is $S_p$.

Write the expression of the effective area of the cylinder.

$A_g=\frac{\pi }{4}\left(C^2\right)$ (XVII)

Here, the effective area of the cylinder is $A_g$, diameter of cylinder is $C$.

Write the expression for the total force acting on the assembly.

$P_{total}=A_g{p}_g$ (XVIII)

Here, the total load acting on the assembly is $P_{total}$, stress on the cylinder sealing is $S_p$.

Write the expression for the load acting on each bolt.

$P=\frac{P_{total}}{N}$ (XIX)

Here, the number of bolt is $N$.

Write the expression for yield factor of safety.

$n_p=\frac{S_p{A}_t}{CP+F_i}$ (XX)

Here the overload factor of safety is $n_p$, initial tension on the bolt is $F_i$, joint constant is $C$ and the proof strength is $S_p$.

Write the expression of overload factor of safety.

$n_L=\frac{S_p{A}_t-F_i}{CP}$ (XXI)

Here the overload factor of safety is $n_L$.

Write the expression of joint separation factor of safety.

$n_o=\frac{F_i}{p\left(1-C\right)}$ . (XXII)

Here the factor of safety based on joint separation is $n_o$.

Conclusion:

Substitute $\frac{3}{8}\text{in}$ for $A$ and $\frac{1}{2}\text{in}$ for $B$ in the Equation (I)

$\begin{array}{c}l=\frac{3}{8}\text{in}+\frac{1}{2}\text{in}\\ \text{=}\left(0.375+0.5\right)\text{in}\\ =\text{0}\text{.875}\text{in}\end{array}$

Refer to Table $\text{A-31}$ “Dimensions of the hexagonal nut” to obtain $\frac{3}{8}\text{in}$ for $H$.

Substitute $0.875\text{in}$ for $l$ and $\frac{3}{8}\text{in}$ for $H$.Equation (II).

$\begin{array}{c}L\ge 0.875\text{in}+\frac{3}{8}\text{in}\\ L=0.875\text{in}+\text{0}\text{.375}\text{in}\\ =\text{1}\text{.25}\text{in}\end{array}$

Substitute $\frac{7}{16}\text{in}$ for $d$ in Equation (III).

$\begin{array}{c}{L}_T=2\times \frac{7}{16}\text{in}+0.25\\ =0.875\text{in}+0.25\\ =1.125\text{in}\end{array}$

Substitute $1.25\text{in}$ for $L$ and $1.125\text{in}$ for $L_T$ in Equation (IV).

$\begin{array}{c}{l}_d=1.25\text{in}-1.125\text{in}\\ \text{=}\left(1.25-1.125\right)\text{in}\\ =\text{0}\text{.125}\text{in}\end{array}$

Substitute $0.875\text{in}$ for $l$ and $0.125\text{in}$ for $l_d$ in Equation (V).

$\begin{array}{c}{l}_t=0.875\text{in}-0.125\text{in}\\ \text{=}\left(0.875-0.125\right)\text{in}\\ =\text{0}\text{.75}\text{in}\end{array}$

Substitute $0.5\text{in}$ for $d$ in Equation (VI).

$\begin{array}{c}{A}_d=\frac{\pi }{4}{\left(\frac{7}{16}\text{in}\right)}^2\\ =\left(\frac{3.14}{4}\right)\left(0.1914\right){\text{in}}^2\\ =0.15025{\text{in}}^2\\ \approx 0.1503{\text{in}}^2\end{array}$

Refer to Table $8.2$ “Diameter and Area of Unified Screw Threads UNC and UNF” to obtain the value of $A_t$ as $0.1063{\text{in}}^2$.

Refer to Table $8-8$ “Stiffness Parameter of Member Material” to obtain the value of $E_s$ as $30\text{Mpsi}$.

Substitute $0.1503{\text{in}}^2$ for $A_d$, $0.1063{\text{in}}^2$ for $A_t$, $0.75\text{in}$ for $l_t$, $0.125\text{in}$ for $l_d$ and $30\text{Mpsi}$ for $E_s$ in Equation (VII).

$\begin{array}{c}{k}_b=\frac{\left(0.1503{\text{in}}^2\right)\left(0.1063{\text{in}}^2\right)\left(30\text{Mpsi}\right)}{\left(0.1503{\text{in}}^2\right)\left(0.75\text{in}\right)+\left(0.1063{\text{in}}^2\right)\left(0.125\text{in}\right)}\\ =\frac{\left(0.1503{\text{in}}^2\right)\left(0.1063{\text{in}}^2\right)\left(30\text{Mpsi}\right)\left(\frac{\text{1}\text{Mlbf}/{\text{in}}^{\text{2}}}{\text{1}\text{Mpsi}}\right)}{\left(0.1503{\text{in}}^2\right)\left(0.75\text{in}\right)+\left(0.1063{\text{in}}^2\right)\left(0.125\text{in}\right)}\\ =\frac{0.47930\text{Mlbf}}{0.12601\text{in}}\\ \approx 3.804\text{Mlbf}/\text{in}\end{array}$

Substitute $30\text{Mpsi}$ for $E_s$, $0.375\text{in}$ for $t$, $\frac{7}{16}\text{in}$ for $d$ and $0.65625\text{in}$ for $D$ in Equation (VIII).

$\begin{array}{c}{k}_1=\frac{0.5774\pi \left(30\text{Mpsi}\right)\left(\frac{7}{16}\text{in}\right)}{\ln \frac{\left(1.155\left(0.375\text{in}\right)+0.65625\text{in}-\frac{7}{16}\text{in}\right)\left(0.65625\text{in}+\frac{7}{16}\text{in}\right)}{\left(1.155\left(0.375\text{in}\right)+0.65625\text{in}+\frac{7}{16}\text{in}\right)\left(0.65625\text{in}-\frac{7}{16}\text{in}\right)}}\\ =\frac{0.5774\left(3.14\right)\left(30\text{Mpsi}\right)\left(\frac{1\text{Mlbf}/{\text{in}}^{\text{2}}}{1\text{Mpsi}}\right)\left(0.4375\text{in}\right)}{\ln \frac{\left(1.155\left(0.375\text{in}\right)+0.65625\text{in}-0.4375\text{in}\right)\left(0.65625\text{in}+0.4375\text{in}\right)}{\left(1.155\left(0.375\text{in}\right)+0.65625\text{in}+0.4375\text{in}\right)\left(0.65625\text{in}-0.4375\text{in}\right)}}\\ =\frac{23.796\text{Mlbf}/\text{in}}{\ln \left(\frac{0.71298}{0.33400}\right)}\\ =\frac{23.796}{0.75832}\text{Mlbf}/\text{in}\end{array}$

$\begin{array}{c}{k}_1=\frac{23.796}{0.75832}\text{Mlbf}/\text{in}\\ =31.3797\text{Mlbf}/\text{in}\\ \approx 31.4\text{Mlbf}/\text{in}\end{array}$

Substitute $\frac{3}{8}\text{in}$ for $A$ and $\frac{1}{2}\text{in}$ for $B$ in the Equation (IX)

$\begin{array}{c}{t}_o=\frac{\frac{3}{8}\text{in}+\frac{1}{2}\text{in}}{2}\\ =\frac{0.375+0.5}{2}\text{in}\\ =0.4375\text{in}\end{array}$

Substitute $0.4375\text{in}$ for $t_o$ and $\frac{3}{8}\text{in}$ for $A$ in the Equation (X)

$\begin{array}{c}{t}_1=0.4375\text{in}-\frac{3}{8}\text{in}\\ =0.0625\text{in}\end{array}$

Substitute $0.65625\text{in}$ for $D$, $\frac{3}{8}\text{in}$ for $A$ and $30°$ for $\alpha$ in the Equation (XI)

$\begin{array}{c}{D}_1=0.65625\text{in}+2\left(\frac{3}{8}\text{in}\right)\tan \left(30°\right)\\ =\left(0.65625+\left(0.75\right)\left(0.57735\right)\right)\text{in}\\ \text{=1}\text{.0892}\text{in}\\ \approx \text{1}\text{.089}\text{in}\end{array}$

Refer to Table $8-8$ “Stiffness Parameter Of Member Material” obtaining the value of $E_c$ as $14.5\text{Mpsi}$.

Substitute $14.5\text{Mpsi}$ for $E_c$, $0.0625\text{in}$ for $t_1$, $\frac{7}{16}\text{in}$ for $d$ and $1.089\text{in}$ for $D_1$ in Equation (XII).

$\begin{array}{c}{k}_2=\frac{0.5774\pi \left(14.5\text{Mpsi}\right)\left(\frac{7}{16}\text{in}\right)}{\ln \frac{\left(1.155\left(0.0625\text{in}\right)+1.089\text{in}-\frac{7}{16}\text{in}\right)\left(1.089\text{in}+\frac{7}{16}\text{in}\right)}{\left(1.155\left(0.0625\text{in}\right)+1.089\text{in}+\frac{7}{16}\text{in}\right)\left(1.089\text{in}-\frac{7}{16}\text{in}\right)}}\\ =\left\{\frac{11.50144}{\ln \left(\frac{1.10470}{1.04154}\right)}\text{Mpsi}\cdot \text{in}\right\}\left(\frac{\text{Mlbf}/{\text{in}}^{\text{2}}}{\text{Mpsi}}\right)\\ =\frac{11.50144}{0.05886}\text{Mlbf}/\text{in}\\ =195.40\text{Mlbf}/\text{in}\end{array}$

Substitute $14.5\text{Mpsi}$ for $E_c$, $0.4375\text{in}$ for $t$, $0.4375\text{in}$ for $d$ and $0.65625\text{in}$ for $D$ in Equation (XIII).

$\begin{array}{c}{k}_3=\frac{0.5774\pi \left(14.5\text{Mpsi}\right)\left(\frac{7}{16}\text{in}\right)}{\ln \frac{\left(1.155\left(0.4375\text{in}\right)+0.65625\text{in}-\frac{7}{16}\text{in}\right)\left(0.65625\text{in}+\frac{7}{16}\text{in}\right)}{\left(1.155\left(0.4375\text{in}\right)+0.65625\text{in}+\frac{7}{16}\text{in}\right)\left(0.65625\text{in}-\frac{7}{16}\text{in}\right)}}\\ =\frac{0.5774\left(3.14\right)\left(14.5\text{Mpsi}\right)\left(\frac{1\text{Mlbf}/{\text{in}}^{\text{2}}}{1\text{Mpsi}}\right)\left(0.4375\text{in}\right)}{\ln \frac{\left(1.155\left(0.4375\text{in}\right)+0.65625\text{in}-0.4375\text{in}\right)\left(0.65625\text{in}+0.4375\text{in}\right)}{\left(1.155\left(0.4375\text{in}\right)+0.65625\text{in}+0.4375\text{in}\right)\left(0.65625\text{in}-0.4375\text{in}\right)}}\\ =\frac{11.50144\text{Mlbf}/\text{in}}{\ln \frac{0.79194}{0.34979}}\\ =\frac{11.50144}{0.81713}\text{Mlbf}/\text{in}\end{array}$

$\begin{array}{c}{k}_3=\frac{11.50144}{0.81713}\text{Mlbf}/\text{in}\\ =14.075\text{Mlbf}/\text{in}\\ =14.08\text{Mlbf}/\text{in}\end{array}$

Substitute $31.4\text{Mlbf}/\text{in}$ for $k_1$, $195.4\text{Mlbf}/\text{in}$ for $k_2$ and $14.08\text{Mlbf}/\text{in}$ for $k_3$ in the Equation (XIV).

$\begin{array}{c}{k}_m={\left[\left(\frac{1}{31.4\text{Mlbf}/\text{in}\text{Mlbf}/\text{in}}\right)+\left(\frac{1}{195.4\text{Mlbf}/\text{in}}\right)+\left(\frac{1}{14.08\text{Mlbf}/\text{in}}\right)\right]}^{-1}\\ =\left[0.031847+5.1177\times {10}^{-3}+0.0710\right]\text{Mlbf}/\text{in}\\ ={\left(0.10798\right)}^{-1}\text{Mlbf}/\text{in}\\ \approx 9.2603\text{Mlbf}/\text{in}\end{array}$

Substitute $3.804\text{Mlbf}/\text{in}$ for $k_b$ and $9.2603\text{Mlbf}/\text{in}$ for $k_m$ in Equation (XV).

$\begin{array}{c}C=\frac{3.804\text{Mlbf}/\text{in}}{3.804\text{Mlbf}/\text{in}+9.2603\text{Mlbf}/\text{in}}\\ =\frac{3.804\text{Mlbf}/\text{in}}{13.0643\text{Mlbf}/\text{in}}\\ =0.291\end{array}$

Refer to Table $8-9$ “SAE specification for steel bolts” to obtain the value of $S_p$ as $120\text{kpsi}$.

Substitute $120\text{kpsi}$ for $S_p$, $0.1063{\text{in}}^{\text{2}}$ for $A_t$ in the Equation (XVI).

$\begin{array}{c}{F}_i=0.75\left(0.1063{\text{in}}^2\right)\left(120\text{kpsi}\right)\\ =0.75\left(0.1063{\text{in}}^2\right)\left(120\text{kpsi}\right)\left(\frac{1\text{kips}/{\text{in}}^{\text{2}}}{1\text{kpsi}}\right)\\ =9.567\text{kips}\\ \approx \text{9}\text{.57}\text{kips}\end{array}$

Substitute $3.25\text{in}$ for $C$ in Equation (XVII).

$\begin{array}{c}{A}_g=\frac{\pi }{4}{\left(3.25\text{in}\text{in}\right)}^2\\ =\left(0.785\right)\left(10.5625\right){\text{in}}^{\text{2}}\\ =9.61625{\text{in}}^{\text{2}}\\ \approx 8.29{\text{in}}^{\text{2}}\end{array}$

Substitute $8.29{\text{in}}^{\text{2}}$ for $A_g$ and $1200\text{psi}$ for $p_g$ in Equation (XVIII).

$\begin{array}{c}{P}_{total}=\left(8.29{\text{in}}^{\text{2}}\right)\left(1200\text{psi}\right)\\ =\left(8.29{\text{in}}^{\text{2}}\right)\left(1200\text{psi}\right)\left(\frac{{\text{10}}^{-\text{3}}\text{kips}/{\text{in}}^{\text{2}}}{\text{1}\text{psi}}\right)\\ =9.948\text{kips}\end{array}$

Substitute $9.948\text{kips}$ for $P_{total}$ and $8$ for $N$ in Equation (XIX).

$\begin{array}{c}P=\frac{9.948\text{kips}}{8}\\ =1.2435\text{kips}/\text{bolt}\\ \approx 1.244\text{kips}/\text{bolt}\end{array}$

Substitute $120\text{kpsi}$ for $S_p$, $0.291$ for $C$, $0.1063{\text{in}}^{\text{2}}$ for $A_t$, $1.244\text{kips}/\text{bolt}$ for $P$ and $9.57\text{kips}$ for $F_i$ in Equation (XX).

$\begin{array}{c}{n}_p=\frac{\left(120\text{kpsi}\right)\left(0.1063{\text{in}}^{\text{2}}\right)}{\left(0.291\right)\left(1.244\text{kips}\right)+9.57\text{kips}}\\ =\frac{12.756}{9.932}\\ =1.2843\\ \approx 1.28\end{array}$

Thus, the yield factor of safety is $1.28$.

Substitute $120\text{kpsi}$ for $S_p$, $0.291$ for $C$, $0.1063{\text{in}}^{\text{2}}$ for $A_t$, $1.244\text{kips}/\text{bolt}$ for $P$ and $9.57\text{kips}$ for $F_i$ in Equation (XXI).

$\begin{array}{c}{n}_L=\frac{\left(120\text{kpsi}\right)\left(0.1063{\text{in}}^2\right)-\left(9.57\text{kips}\right)}{\left(0.291\right)\left(1.244\text{kips}\right)}\\ =\frac{\left(120\text{kpsi}\right)\left(\frac{\text{1}\text{kips}/{\text{in}}^{\text{2}}}{\text{kpsi}}\right)\left(0.1063{\text{in}}^2\right)-\left(9.57\text{kips}\right)}{\left(0.291\right)\left(1.244\text{kips}\right)}\\ =\frac{3.186}{0.36200}\\ =8.80\end{array}$

Thus, the load factor of safety is $8.80$.

Substitute $0.291$ for $C$, $1.244\text{kips}/\text{bolt}$ for $P$ and $9.57\text{kips}$ for $F_i$ in Equation (XXII).

$\begin{array}{c}{n}_o=\frac{9.57\text{kips}}{\left(1.244\text{kips}/\text{bolt}\right)\left(1-0.291\right)}\\ =\frac{9.57}{0.88199}\\ =10.85\\ =10.9\end{array}$

Thus, the joint separation factor is $10.9$.