Chapter 7.5, Problem 75E

A.)

To determine

Match the system of inequalities with the graph of its solution.

Answer to Problem 75E

The system of inequalities matches to graph (iv)

Explanation of Solution

Given:

The following inequalities

  $\begin{array}{l}{x}_2+y_2\le 16\hfill \\ x+y\ge 4\hfill \end{array}$

Consider the following inequalities

  $\begin{array}{l}{x}_2+y_2\le 16\hfill \\ x+y\ge 4\hfill \end{array}$

First use maple to draw the graph of above inequalities

  

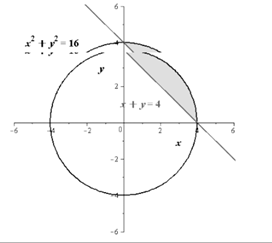
From the above graph it shows that the shaded region is inside the circle $x_2+y_2=16$ and above the line $x+y=4$

Therefore, the system of inequalities matches to graph (iv)

B.)

To determine

Match the system of inequalities with the graph of its solution.

Answer to Problem 75E

The system of inequalities matches to graph (ii).

Explanation of Solution

Given:

The following inequalities

  $\begin{array}{l}{x}_2+y_2\le 16\hfill \\ x+y \le 4\hfill \end{array}$

Consider the following inequalities

  $\begin{array}{l}{x}_2+y_2\le 16\hfill \\ x+y \le 4\hfill \end{array}$

First use maple to draw the graph of above inequalities

  

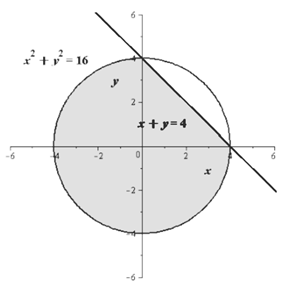
From the above graph it shows that the shaded region is inside the circle $x_2+y_2=16$ and below the line $x+y=4$ .

Therefore, the system of inequalities matches to graph (ii).

C.)

To determine

Match the system of inequalities with the graph of its solution.

Answer to Problem 75E

The system of inequalities matches to graph(iii).

Explanation of Solution

Given:

The following inequalities

  $\begin{array}{l}{x}_2+y_2\ge 16\hfill \\ x+y\ge 4\hfill \end{array}$

Consider the following inequalities

  $\begin{array}{l}{x}_2+y_2\ge 16\hfill \\ x+y\ge 4\hfill \end{array}$

First use maple to draw the graph of above inequalities

  

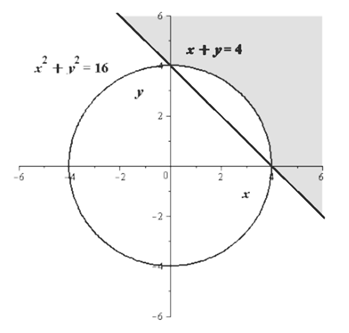
The shaded region is outside the circle $x_2+y_2=16$ and above the line $x+y=4$ .

Therefore, the system of inequalities matches to graph(iii).

D.)

To determine

Match the system of inequalities with the graph of its solution.

Answer to Problem 75E

The system of inequalities matches to graph (i).

Explanation of Solution

Given:

The following inequalities

  $\begin{array}{l}{x}_2+y_2\ge 16\hfill \\ x+y\le 4\hfill \end{array}$

Consider the following inequalities

  $\begin{array}{l}{x}_2+y_2\ge 16\hfill \\ x+y\le 4\hfill \end{array}$

First use maple to draw the graph of above inequalities

  

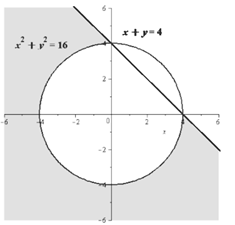
The shaded region is outside the circle $x_2+y_2=16$ and below the line $x+y=4$

Therefore, the system of inequalities matches to graph (i).