Chapter 6.5, Problem 30PPE

To determine

To find: To set and graph a linear inequality on modeling given situation and then finding three possible combinations of each type of wood that can be bought.

Answer to Problem 30PPE

Required linear inequality is

  $2.5x+1.75y\le 200$ and its graph is,

  

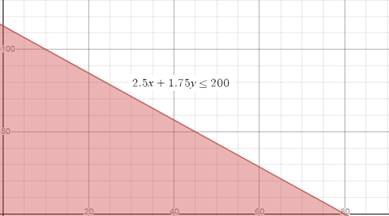
Three possible combinations of both type of woods are,

1. 40 feet of cedar wood and 50 feet of pine wood.
2. 45 feet of cedar wood and 50 feet of pine wood.
3. 60 feet of cedar wood and 25 feet of pine wood.

Explanation of Solution

Given information: Cedar costs $2.5 per foot and pine costs $1.75 per foot. Both type of woods to be bought in a budget $200.

Concept used: First set the linear inequality, representing given situation using following statement,

  $\text{Amount of cedar wood }\times \text{its cost+amount of pine wood}\times \text{its cost}\le 200$

And then find three values of unknown variables, satisfying this inequality.

Calculation: Let x foot of cedar wood and y foot of pine wood are bought. So, use above statement to form linear inequality as,

  $2.5x+1.75y\le 200$

Calculate its two points of related equation $2.5x+1.75y=200$ in ordered pair form as follows,

x	$2.5x+1.75y=200$	$(x,y)$
80	$\begin{array}{l}2.5\times 80+1.75y=200\\ 200+1.75y=200\\ 1.75y=200-200=0\\ y=\frac{0}{1.75}=0\end{array}$	$(80,0)$
45	$\begin{array}{l}2.5\times 45+1.75y=200\\ 112.5+1.75y=200\\ 1.75y=200-112.5=87.5\\ y=\frac{87.5}{1.75}=50\end{array}$	$(45,50)$

Plot these points in XY plane and get the given inequality satisfied by point (0,0), so that to find its solution region as shown in below graph,

  

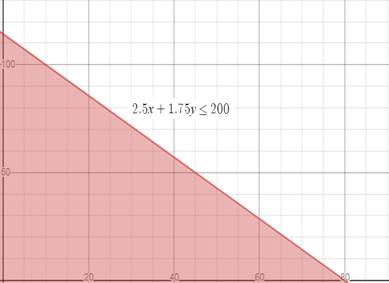
Three possible amounts of woods, as shown in above shaded area, are,

  $\begin{array}{l}(i)x=40,y=50\\ (ii)x=45,y=50\\ (iii)x=60,y=25\end{array}$

Conclusion: So, different possibility of buying may be,

1. 40 feet of cedar wood and 50 feet of pine wood.
2. 45 feet of cedar wood and 50 feet of pine wood.
3. 60 feet of cedar wood and 25 feet of pine wood.