
Concept explainers
The uniform bars AB and BC each weigh 4 lb/ft. Calculate the tension in cable DE, and the magnitudes of the ball-and-socket reactions at A, B, and C.
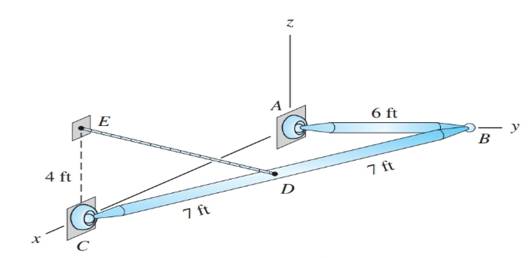
Tension in cable DE and magnitude of reactions at A, B and C.
Answer to Problem 5.56RP
Explanation of Solution
Given Information:
Weight of the uniform bars AB and BC is 4 lb/ft.

Calculation:
Draw free body diagram of as shown in following figure,
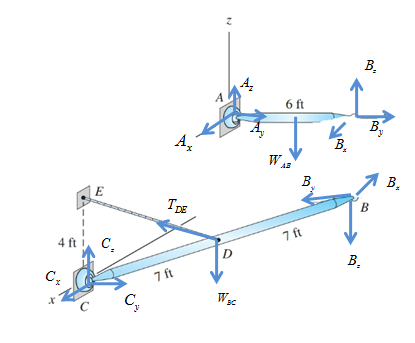
Take equilibrium of moments about x-axis passing through A in free body diagram of AB as,
Take equilibrium of moments about z-axis passing through A in free body diagram of AB as,
Take equilibrium of forces in x-direction in free body diagram of AB as,
Take equilibrium of forces in y-direction in free body diagram of AB as,
Take equilibrium of forces in z-direction in free body diagram of AB as,
Take equilibrium of moments about C in free body diagram of BC as,
Above vector equation yields following scalar equations,
Solve equations 6 and 7 to get
From equation (4)
Take equilibrium of forces in free body diagram of BC as,
Above vector equation yields following scalar equations,
Substitute known parameters and solve equations (8-10) to get,
Magnitude of reaction at A:
Magnitude of reaction at B:
Magnitude of reaction at C:
Conclusion:
Therefore,
Want to see more full solutions like this?
Chapter 5 Solutions
International Edition---engineering Mechanics: Statics, 4th Edition
- Can I get help on this question?arrow_forwardDuring some actual expansion and compression processes in piston–cylinder devices, the gases have been observed to satisfy the relationship PVn = C, where n and C are constants. Calculate the work done when a gas expands from 350 kPa and 0.03 m3 to a final volume of 0.2 m3 for the case of n = 1.5. The work done in this case is kJ.arrow_forwardCarbon dioxide contained in a piston–cylinder device is compressed from 0.3 to 0.1 m3. During the process, the pressure and volume are related by P = aV–2, where a = 6 kPa·m6. Calculate the work done on carbon dioxide during this process. The work done on carbon dioxide during this process is kJ.arrow_forward
- The volume of 1 kg of helium in a piston–cylinder device is initially 5 m3. Now helium is compressed to 3 m3 while its pressure is maintained constant at 130 kPa. Determine the initial and final temperatures of helium as well as the work required to compress it, in kJ. The gas constant of helium is R = 2.0769 kJ/kg·K. The initial temperature of helium is K. The final temperature of helium is K. The work required to compress helium is kJ.arrow_forwardA piston-cylinder device initially contains 0.4 kg of nitrogen gas at 160 kPa and 140°C. Nitrogen is now expanded isothermally to a pressure of 80 kPa. Determine the boundary work done during this process. The properties of nitrogen are R= 0.2968 kJ/kg-K and k= 1.4. N₂ 160 kPa 140°C The boundary work done during this process is KJ.arrow_forward! Required information An abrasive cutoff wheel has a diameter of 5 in, is 1/16 in thick, and has a 3/4-in bore. The wheel weighs 4.80 oz and runs at 11,700 rev/min. The wheel material is isotropic, with a Poisson's ratio of 0.20, and has an ultimate strength of 12 kpsi. Choose the correct equation from the following options: Multiple Choice о σmax= (314) (4r2 — r²) - о σmax = p² (3+) (4r² + r²) 16 σmax = (314) (4r² + r²) σmax = (314) (4² - r²)arrow_forward
- I don't know how to solve thisarrow_forwardI am not able to solve this question. Each part doesn't make sense to me.arrow_forwardExercises Find the solution of the following Differential Equations 1) y" + y = 3x² 3) "+2y+3y=27x 5) y"+y=6sin(x) 7) y"+4y+4y = 18 cosh(x) 9) (4)-5y"+4y = 10 cos(x) 11) y"+y=x²+x 13) y"-2y+y=e* 15) y+2y"-y'-2y=1-4x³ 2) y"+2y' + y = x² 4) "+y=-30 sin(4x) 6) y"+4y+3y=sin(x)+2 cos(x) 8) y"-2y+2y= 2e* cos(x) 10) y+y-2y=3e* 12) y"-y=e* 14) y"+y+y=x+4x³ +12x² 16) y"-2y+2y=2e* cos(x)arrow_forward
 International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
