Calculus Early Transcendentals, Binder Ready Version
Calculus Early Transcendentals, Binder Ready Version
11th Edition
ISBN: 9781118883822
Author: Howard Anton, Irl C. Bivens, Stephen Davis
Publisher: WILEY
bartleby

Concept explainers

Minimization
In mathematics, traditional optimization problems are typically expressed in terms of minimization. When we talk about minimizing or maximizing a function, we refer to the maximum and minimum possible values of that function. This can be expressed in ter…
Maxima and Minima
The extreme points of a function are the maximum and the minimum points of the function. A maximum is attained when the function takes the maximum value and a minimum is attained when the function takes the minimum value.
Derivatives
A derivative means a change. Geometrically it can be represented as a line with some steepness. Imagine climbing a mountain which is very steep and 500 meters high. Is it easier to climb? Definitely not! Suppose walking on the road for 500 meters. Which …
Concavity
In calculus, concavity is a descriptor of mathematics that tells about the shape of the graph. It is the parameter that helps to estimate the maximum and minimum value of any of the functions and the concave nature using the graphical method. We use the …
bartleby

Videos

Textbook Question
Book Icon
Chapter 4.6, Problem 3ES

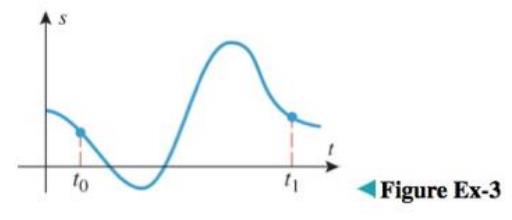
The graph of the position function of a particle moving on a horizontal line is shown in the accompanying figure.

(a) Is the particle moving left or right at time t 0 ?

(b) Is the acceleration positive or negative at time t 0 ?

(c) Is the particle speeding up or slowing down at time r 0 ?

(d) Is the particle speeding up or slowing down at time t 1 ?

Chapter 4.6, Problem 3ES, The graph of the position function of a particle moving on a horizontal line is shown in the

Expert Solution & Answer
Check Mark

Want to see the full answer?

Check out a sample textbook solution
Blurred answer
Previous
Chapter 4.6, Problem 2ES
Next
Chapter 4.6, Problem 4ES
Students have asked these similar questions
A4) The position of a hummingbird flying along a straight line in t seconds is given by S(t) = 2t - 20t meters. [ 1 mark ] (a) Determine the velocity of the bird at t = 3 sec. [0.25 mark] (b) Determine the acceleration of the bird at t = 3 sec. [0.25 mark] (c) Determine the acceleration of the bird when the velocity equals 0. [0.5 mark]
(10) The position function for a particle's motion on a line is s(t) = t³-t²-t+1, t 20. s is in meters and t in seconds. At what value(s) of t is the particle at rest? (A) t = 0 sec. (B) t = sec. (C) t = 1 sec. (D) it has no rest
Question #14 An object is launched into the air. Its path is calculated using the function s(t) = -0.25t² +10t +5 where s(t) is the position of the object in feet and the variable "t" time in seconds. Find each of the following... ( A) What is the position of the object at t = 4 seconds? B) What is the velocity of the object at t = 4 seconds? C) What is the acceleration of the object at t = 4 seconds? D) When does the object reach its maximum height? E) What is the maximum height of the object?

Chapter 4 Solutions

Calculus Early Transcendentals, Binder Ready Version

Ch. 4.1 - (a) A function f is increasing on a,b if whenever...Ch. 4.1 - Let fx=0.1x33x29x . Then...Ch. 4.1 - Suppose that fx has derivative fx=x42ex/2 . Then...Ch. 4.1 - Consider the statement “The rise in the cost of...Ch. 4.1 - In each part, sketch the graph of a function f...Ch. 4.1 - In each part, sketch the graph of a function f...Ch. 4.1 - Use the graph of the equation y=fx in the...Ch. 4.1 - Use the graph of the equation y=fx in the...Ch. 4.1 - Use the graph of y=fx in the accompanying figure...Ch. 4.1 - Use the graph of y=fx in the accompanying figure...
Ch. 4.1 - In each part, use the graph of y=fx in the...Ch. 4.1 - Prob. 8ESCh. 4.1 - A sign chart is presented for the first and second...Ch. 4.1 - A sign chart is presented for the first and second...Ch. 4.1 - Assume that f is differentiable everywhere....Ch. 4.1 - Assume that f is differentiable everywhere....Ch. 4.1 - Assume that f is differentiable everywhere....Ch. 4.1 - Assume that f is differentiable everywhere....Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Find: (a) the intervals on which f is increasing,...Ch. 4.1 - Analyze the trigonometric function f over the...Ch. 4.1 - Analyze the trigonometric function f over the...Ch. 4.1 - Analyze the trigonometric function f over the...Ch. 4.1 - Analyze the trigonometric function f over the...Ch. 4.1 - Analyze the trigonometric function f over the...Ch. 4.1 - Analyze the trigonometric function f over the...Ch. 4.1 - In parts (a)-(c), sketch a continuous curve y=fx...Ch. 4.1 - In each part sketch a continuous curve y=fx with...Ch. 4.1 - If f is increasing on an interval 0,b, then it...Ch. 4.1 - If f is increasing on an interval 0,b, then it...Ch. 4.1 - If f is increasing on an interval 0,b, then it...Ch. 4.1 - If f is increasing on an interval 0,b, then it...Ch. 4.1 - If f is increasing on an interval 0,b, then it...Ch. 4.1 - If f is increasing on an interval 0,b, then it...Ch. 4.1 - Use a graphing utility to generate the graphs of f...Ch. 4.1 - Use a graphing utility to generate the graphs of f...Ch. 4.1 - Use a CAS to find f and to approximate the...Ch. 4.1 - Use a CAS to find f and to approximate the...Ch. 4.1 - Use Definition to prove that is increasing on . Ch. 4.1 - Use Definition to prove that is increasing on . Ch. 4.1 - Determine whether the statements are true or...Ch. 4.1 - Determine whether the statements are true or...Ch. 4.1 - In each part, find functions f and g that are...Ch. 4.1 - In each part, find functions f and g that are...Ch. 4.1 - (a) Prove that a general cubic polynomial (b)...Ch. 4.1 - From Exercise 57, the polynomial fx=x3+bx2+1 has...Ch. 4.1 - Use Definition 4.1.1 to prove: (a) If f is...Ch. 4.1 - Use part (a) of Exercise 59 to show that fx=x+sinx...Ch. 4.1 - Use part (b) of Exercise 59 to show that fx=cosxx...Ch. 4.1 - Let y=1/1+x2 . Find the value of x for which y is...Ch. 4.1 - Prob. 63ESCh. 4.1 - Prob. 64ESCh. 4.1 - Prob. 65ESCh. 4.1 - Prob. 66ESCh. 4.1 - Suppose that a population y grows according to the...Ch. 4.1 - Suppose that the number of individuals at time t...Ch. 4.1 - Suppose that the spread of a flu virus on a...Ch. 4.1 - The logistic growth model given in Formula (1) is...Ch. 4.1 - Assuming that and are positive constants, verify...Ch. 4.1 - Explain what the sign analyses of and tell us...Ch. 4.2 - A function f has a relative maximum at x0 if there...Ch. 4.2 - Suppose that f is defined everywhere and x=2,3,5,7...Ch. 4.2 - Suppose that f id defined everywhere and x=2 and...Ch. 4.2 - Let fx=x242 . Then fx=4xx24 and fx=43x24 ....Ch. 4.2 - In each part, sketch the graph of a continuous...Ch. 4.2 - In each part, sketch the graph of a continuous...Ch. 4.2 - (a) Use both the first and second derivative tests...Ch. 4.2 - (a) Use both the first and second derivative tests...Ch. 4.2 - (a) Show that both of the functions fx=x14 and...Ch. 4.2 - (a) Show that fx=1x5 and gx=3x48x3 both have...Ch. 4.2 - Locate the critical points and identify which...Ch. 4.2 - Locate the critical points and identify which...Ch. 4.2 - Locate the critical points and identify which...Ch. 4.2 - Locate the critical points and identify which...Ch. 4.2 - Locate the critical points and identify which...Ch. 4.2 - Locate the critical points and identify which...Ch. 4.2 - Locate the critical points and identify which...Ch. 4.2 - Locate the critical points and identify which...Ch. 4.2 - Assume that is continuous everywhere. Determine...Ch. 4.2 - Assume that f is continuous everywhere. Determine...Ch. 4.2 - Assume that f is continuous everywhere. Determine...Ch. 4.2 - Assume that f is continuous everywhere. Determine...Ch. 4.2 - The graph of a function fx is given. Sketch graphs...Ch. 4.2 - The graph of a function fx is given. Sketch graphs...Ch. 4.2 - Use the graph of f shown in the figure to estimate...Ch. 4.2 - Use the graph of f shown in the figure to estimate...Ch. 4.2 - Use the graph of f shown in the figure to estimate...Ch. 4.2 - Use the graph of f shown in the figure to estimate...Ch. 4.2 - Use the given derivative to find all critical...Ch. 4.2 - Use the given derivative to find all critical...Ch. 4.2 - Use the given derivative to find all critical...Ch. 4.2 - Use the given derivative to find all critical...Ch. 4.2 - Use the given derivative to find all critical...Ch. 4.2 - Use the given derivative to find all critical...Ch. 4.2 - Use the given derivative to find all critical...Ch. 4.2 - Use the given derivative to find all critical...Ch. 4.2 - Find the relative extrema using both first and...Ch. 4.2 - Find the relative extrema using both first and...Ch. 4.2 - Find the relative extrema using both first and...Ch. 4.2 - Find the relative extrema using both first and...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Use any method to find the relative extrema of the...Ch. 4.2 - Give a graph of the polynomial and label the...Ch. 4.2 - Give a graph of the polynomial and label the...Ch. 4.2 - Give a graph of the polynomial and label the...Ch. 4.2 - Give a graph of the polynomial and label the...Ch. 4.2 - Give a graph of the polynomial and label the...Ch. 4.2 - Give a graph of the polynomial and label the...Ch. 4.2 - Give a graph of the polynomial and label the...Ch. 4.2 - Give a graph of the polynomial and label the...Ch. 4.2 - Give a graph of the polynomial and label the...Ch. 4.2 - Give a graph of the polynomial and label the...Ch. 4.2 - In each part: (i) Make a conjecture about the...Ch. 4.2 - Sketch the graph of y=xamxbn for the stated values...Ch. 4.2 - Find the relative extrema in the interval 0x2, and...Ch. 4.2 - Find the relative extrema in the interval 0x2, and...Ch. 4.2 - Find the relative extrema in the interval 0x2, and...Ch. 4.2 - Find the relative extrema in the interval 0x2, and...Ch. 4.2 - Use a graphing utility to make a conjecture about...Ch. 4.2 - Use a graphing utility to make a conjecture about...Ch. 4.2 - Use a graphing utility to make a conjecture about...Ch. 4.2 - Use a graphing utility to make a conjecture about...Ch. 4.2 - Use a graphing utility to generate the graphs of f...Ch. 4.2 - Use a graphing utility to generate the graphs of f...Ch. 4.2 - Use a CAS to graph f and f, and then use those...Ch. 4.2 - Use a CAS to graph f and f, and then use those...Ch. 4.2 - Use a CAS to graph f and f, and then use those...Ch. 4.2 - Use a CAS to graph f and f, and then use those...Ch. 4.2 - In each part, find k so that f has a relative...Ch. 4.2 - (a) Use a CAS to graph the function fx=x4+1x2+1...Ch. 4.2 - Function similar to fx=12ex2/2 arise in a wide...Ch. 4.2 - Functions of the form fx=xnexn!,x0 where n is a...Ch. 4.2 - Let h and g have relative maxima at x0 . Prove or...Ch. 4.3 - Let fx=3x+1x3x+2x4 . Given that...Ch. 4.3 - Let fx=x24x8/3 . Given that...Ch. 4.3 - Let fx=x22ex/2 . Given that...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - Give a graph of the rational function and label...Ch. 4.3 - In each part, make a rough sketch of the graph...Ch. 4.3 - In each part, make a rough sketch of the graph...Ch. 4.3 - Show that y=x+3 is an oblique asymptote of the...Ch. 4.3 - Show that y=3x2 is a curvilinear asymptote of the...Ch. 4.3 - Sketch a graph of the rational function and label...Ch. 4.3 - Sketch a graph of the rational function and label...Ch. 4.3 - Sketch a graph of the rational function and label...Ch. 4.3 - Sketch a graph of the rational function and label...Ch. 4.3 - Sketch a graph of the rational function and label...Ch. 4.3 - Sketch a graph of the rational function and label...Ch. 4.3 - In each part, match the function with graphs I-VI....Ch. 4.3 - Sketch the general shape of the graph of y=x1/n,...Ch. 4.3 - Determine whether the statement is true or false....Ch. 4.3 - Determine whether the statement is true or false....Ch. 4.3 - Determine whether the statement is true or false....Ch. 4.3 - Determine whether the statement is true or false....Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Give a graph of the function and identify the...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Using L'Ho pital's rule (Section 3.6) one can...Ch. 4.3 - Consider the family of curves y=xebxb0 . (a) Use a...Ch. 4.3 - Consider the family of curves y=ebx2b0 . (a) Use a...Ch. 4.3 - (a) Determine whether the following limits exist,...Ch. 4.3 - Consider the family of curves y=xnex2/n, where n...Ch. 4.3 - The accompanying figure shows the graph of the...Ch. 4.3 - Let fx=12xhx, where hx is as given in Exercise 65....Ch. 4.3 - A rectangular plot of land is to be fenced off so...Ch. 4.3 - A box with a square base and open top is to be...Ch. 4.4 - Use the accompanying graph to find the...Ch. 4.4 - Suppose that a function f is continuous on 4,4 and...Ch. 4.4 - Let fx=x33x29x+25 . Use the derivative fx=3x+1x3...Ch. 4.4 - Use the graph to find x-coordinates of the...Ch. 4.4 - Use the graph to find x-coordinates of the...Ch. 4.4 - In each part, sketch the graph of a continuous...Ch. 4.4 - In each part, sketch the graph of a continuous...Ch. 4.4 - Let fx11x,0x10,x=1 Explain why f has a minimum...Ch. 4.4 - Let fxx,0x112,x=0,1 Explain why f has a minimum...Ch. 4.4 - Find the absolute maximum and minimum values of f...Ch. 4.4 - Find the absolute maximum and minimum values of f...Ch. 4.4 - Find the absolute maximum and minimum values of f...Ch. 4.4 - Find the absolute maximum and minimum values of f...Ch. 4.4 - Find the absolute maximum and minimum values of f...Ch. 4.4 - Find the absolute maximum and minimum values of f...Ch. 4.4 - Find the absolute maximum and minimum values of f...Ch. 4.4 - Find the absolute maximum and minimum values of f...Ch. 4.4 - Find the absolute maximum and minimum values of f...Ch. 4.4 - Find the absolute maximum and minimum values of f...Ch. 4.4 - Determine whether the statement is true or false....Ch. 4.4 - Determine whether the statement is true or false....Ch. 4.4 - Determine whether the statement is true or false....Ch. 4.4 - Determine whether the statement is true or false....Ch. 4.4 - Find the absolute maximum and minimum values of f,...Ch. 4.4 - Find the absolute maximum and minimum values of f,...Ch. 4.4 - Find the absolute maximum and minimum values of f,...Ch. 4.4 - Find the absolute maximum and minimum values of f,...Ch. 4.4 - Find the absolute maximum and minimum values of f,...Ch. 4.4 - Find the absolute maximum and minimum values of f,...Ch. 4.4 - Find the absolute maximum and minimum values of f,...Ch. 4.4 - Find the absolute maximum and minimum values of f,...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Use a graphing utility to estimate the absolute...Ch. 4.4 - Find the absolute maximum and minimum values of...Ch. 4.4 - Let fx=x2+px+q . Find the values of p and q such...Ch. 4.4 - If f is a periodic function, then the locations of...Ch. 4.4 - If f is a periodic function, then the locations of...Ch. 4.4 - One way of proving that fxgx for all x in a given...Ch. 4.4 - One way of proving that fxgx for all x in a given...Ch. 4.4 - What is the smallest possible slope for a tangent...Ch. 4.4 - (a) Show that fx=secx+cscx has a minimum value but...Ch. 4.4 - Show that the absolute minimum value of...Ch. 4.4 - The concentration Ct of a drug in the bloodstream...Ch. 4.4 - A rectangular poster has one side of length x...Ch. 4.4 - A small soft drink can is designed to have volume...Ch. 4.4 - At a construction site, a worker raises an 8-foot...Ch. 4.4 - In the context of Exercise 55, suppose that 0AB...Ch. 4.4 - Suppose that the equations of motion of a paper...Ch. 4.4 - The accompanying figure on the next page shows the...Ch. 4.4 - Let fx=ax2+bx+c, where a0 . Prove that fx0 for all...Ch. 4.4 - Prove Theorem 4.4.3 in the case where the extreme...Ch. 4.4 - Suppose that f is continuous and positive-valued...Ch. 4.5 - A positive number x and its reciprocal are added...Ch. 4.5 - Two nonnegative numbers, x and y, have a sum equal...Ch. 4.5 - A rectangle in the xy-plane has one comer at the...Ch. 4.5 - An open box is to be made from a 20-inch by...Ch. 4.5 - Find a number in the closed interval 12,32 such...Ch. 4.5 - How should two nonnegative numbers be chosen so...Ch. 4.5 - A rectangular field is to be bounded by a fence on...Ch. 4.5 - The boundary of a field is a right triangle with a...Ch. 4.5 - A rectangular plot of land is to be fenced in...Ch. 4.5 - A rectangle is to be inscribed in a right triangle...Ch. 4.5 - Solve the problem in Exercise 6 assuming the...Ch. 4.5 - A rectangle has its two lower comers on the x-axis...Ch. 4.5 - Find the dimensions of the rectangle with maximum...Ch. 4.5 - Find the point P in the first quadrant on the...Ch. 4.5 - A rectangular area of 3200ft2 is to be fenced off....Ch. 4.5 - Show that among all rectangles with perimeter p,...Ch. 4.5 - Show that among all rectangles with area A, the...Ch. 4.5 - A wire of length 12in can be bent into a circle,...Ch. 4.5 - A rectangle R in the plane has comers at 8,12, and...Ch. 4.5 - Solve the problem in Exercise 15 if S is a 16 by...Ch. 4.5 - Solve the problem in Exercise 15 if S is...Ch. 4.5 - A rectangular page is to contain 42 square inches...Ch. 4.5 - A box with a square base is taller than it is...Ch. 4.5 - A box with a square base is wider than it is tall....Ch. 4.5 - An open box is to be made from a 3ft by 8ft...Ch. 4.5 - A closed rectangular container with a square base...Ch. 4.5 - A closed rectangular container with a square base...Ch. 4.5 - A container with square base, vertical sides, and...Ch. 4.5 - A rectangular container with two square sides and...Ch. 4.5 - A church window consisting of a rectangle topped...Ch. 4.5 - Find the dimensions of the right circular cylinder...Ch. 4.5 - Find the dimensions of the right circular cylinder...Ch. 4.5 - A closed, cylindrical can is to have a volume of V...Ch. 4.5 - A closed cylindrical can is to have a surface area...Ch. 4.5 - A cylindrical can, open at the top, is to hold...Ch. 4.5 - A soup can in the shape of a right circular...Ch. 4.5 - A box-shaped wire frame consists of two identical...Ch. 4.5 - Suppose that the sum of the surface areas of a...Ch. 4.5 - Find the height and radius of the cone of slant...Ch. 4.5 - A cone is made from a circular sheet of radius R...Ch. 4.5 - A cone-shaped paper drinking cup is to hold 100cm3...Ch. 4.5 - Find the dimensions of the isosceles triangle of...Ch. 4.5 - Find the height and radius of the right circular...Ch. 4.5 - A commercial cattle ranch currently allows 20...Ch. 4.5 - Prob. 41ESCh. 4.5 - A fertilizer producer finds that it can sell its...Ch. 4.5 - (a) A chemical manufacturer sells sulfuric acid in...Ch. 4.5 - A firm determines that x units of its product can...Ch. 4.5 - In a certain chemical manufacturing process, the...Ch. 4.5 - An independent truck driver charges a client $15...Ch. 4.5 - A trapezoid is inscribed in a semicircle of radius...Ch. 4.5 - A drainage channel is to be made so that its cross...Ch. 4.5 - A lamp is suspended above the center of a round...Ch. 4.5 - A plank is used to reach over a fence 8ft high to...Ch. 4.5 - Two particles, A and B, are in motion in the...Ch. 4.5 - Follow the directions of Exercise 51, with...Ch. 4.5 - Find the coordinates of the point P on the curve...Ch. 4.5 - Find the x-coordinate of the point P on the...Ch. 4.5 - Where on the curve y=1+x21 does the tangent line...Ch. 4.5 - Suppose that the number of bacteria in a culture...Ch. 4.5 - The shoreline of Circle Lake is a circle with...Ch. 4.5 - A man is floating in a rowboat 1 mile from the...Ch. 4.5 - A pipe of negligible diameter is to be carried...Ch. 4.5 - A concrete barrier whose cross section is an...Ch. 4.5 - Suppose that the intensity of a point light source...Ch. 4.5 - Given points A2,1 and B5,4, find the point P in...Ch. 4.5 - The lower edge of a painting, 10ft in height, is...Ch. 4.5 - Fermat’s principle (biography on p.213 ) in...Ch. 4.5 - Fermat’s principle (Exercise 64) also explains...Ch. 4.5 - A farmer wants to walk at a constant rate from her...Ch. 4.5 - Prob. 67ESCh. 4.5 - Prove: If fx0 on an interval and if fx has a...Ch. 4.6 - For a particle in rectilinear motion, the velocity...Ch. 4.6 - Suppose that a particle moving along the s-axis...Ch. 4.6 - A particle in rectilinear motion is speeding up if...Ch. 4.6 - Suppose that a particle moving along the s-axis...Ch. 4.6 - The graphs of three position functions are shown...Ch. 4.6 - The graphs of three velocity functions are shown...Ch. 4.6 - The graph of the position function of a particle...Ch. 4.6 - For the graphs in the accompanying figure, match...Ch. 4.6 - Sketch a reasonable graph of s versus t for a...Ch. 4.6 - The accompanying figure shows the position versus...Ch. 4.6 - The accompanying figure shows the graph of...Ch. 4.6 - The accompanying figure (on the next page) shows...Ch. 4.6 - Determine whether the statement is true or false....Ch. 4.6 - Prob. 10ESCh. 4.6 - Determine whether the statement is true or false....Ch. 4.6 - Determine whether the statement is true or false....Ch. 4.6 - The accompanying figure shows the velocity versus...Ch. 4.6 - The accompanying figure shows the velocity versus...Ch. 4.6 - The function st describes the position of a...Ch. 4.6 - The function st describes the position of a...Ch. 4.6 - The function st describes the position of a...Ch. 4.6 - The function st describes the position of a...Ch. 4.6 - The function st describes the position of a...Ch. 4.6 - The function st describes the position of a...Ch. 4.6 - The function st describes the position of a...Ch. 4.6 - The function st describes the position of a...Ch. 4.6 - Let st=t/t2+5 be the position function of a...Ch. 4.6 - Prob. 24ESCh. 4.6 - A position function of a particle moving along a...Ch. 4.6 - A position function of a particle moving along a...Ch. 4.6 - A position function of a particle moving along a...Ch. 4.6 - A position function of a particle moving along a...Ch. 4.6 - A position function of a particle moving along a...Ch. 4.6 - A position function of a particle moving along a...Ch. 4.6 - A position function of a particle moving along a...Ch. 4.6 - A position function of a particle moving along a...Ch. 4.6 - Let st=5t222t be the position function of a...Ch. 4.6 - Let s=100/t2+12 be the position function of a...Ch. 4.6 - A position function of a particle moving along a...Ch. 4.6 - A position function of a particle moving along a...Ch. 4.6 - Let s=2t2+1 be the position function of a particle...Ch. 4.6 - (a) Use the chain rule to show that for a particle...Ch. 4.6 - Suppose that the position functions of two...Ch. 4.6 - Let sA=15t2+10t+20 and sB=5t2+40t,t0, be the...Ch. 4.6 - Prove that a particle is speeding up if the...Ch. 4.7 - Use the accompanying graph to estimate x2 and x3...Ch. 4.7 - Suppose that f1=1 and f1=4 . If Newton’s Method...Ch. 4.7 - Suppose we are given that f0=3 and that x2=3 when...Ch. 4.7 - If Newton’s Method is applied to y=ex1 with...Ch. 4.7 - In this exercise set, express your answers with as...Ch. 4.7 - In this exercise set, express your answers with as...Ch. 4.7 - In this exercise set, express your answers with as...Ch. 4.7 - In this exercise set, express your answers with as...Ch. 4.7 - The given equation has one real solution....Ch. 4.7 - The given equation has one real solution....Ch. 4.7 - The given equation has one real solution....Ch. 4.7 - The given equation has one real solution....Ch. 4.7 - Use a graphing utility to determine how many...Ch. 4.7 - Use a graphing utility to determine how many...Ch. 4.7 - Use a graphing utility to determine how many...Ch. 4.7 - Use a graphing utility to determine how many...Ch. 4.7 - Use a graphing utility to determine how many...Ch. 4.7 - Use a graphing utility to determine how many...Ch. 4.7 - Use a graphing utility to determine the number of...Ch. 4.7 - Use a graphing utility to determine the number of...Ch. 4.7 - Use a graphing utility to determine the number of...Ch. 4.7 - Use a graphing utility to determine the number of...Ch. 4.7 - Use a graphing utility to determine the number of...Ch. 4.7 - Use a graphing utility to determine the number of...Ch. 4.7 - Determine whether the statement is true or false....Ch. 4.7 - Determine whether the statement is true or false....Ch. 4.7 - Determine whether the statement is true or false....Ch. 4.7 - Determine whether the statement is true or false....Ch. 4.7 - The mechanic’s rule for approximating square...Ch. 4.7 - Many calculators compute reciprocals using the...Ch. 4.7 - Use Newton’s Method to approximate the absolute...Ch. 4.7 - Use Newton’s Method to approximate the absolute...Ch. 4.7 - For the function fx=ex1+x2 use Newton’s Method...Ch. 4.7 - Use Newton’s Method to approximate the absolute...Ch. 4.7 - Use Newton’s Method to approximate the...Ch. 4.7 - Use Newton’s Method to approximate the...Ch. 4.7 - (a) Show that on a circle of radius r, the central...Ch. 4.7 - A segment of a circle is the region enclosed by an...Ch. 4.7 - A walker exercises on an elliptical track given by...Ch. 4.7 - A 6-inch tall plastic “bubble� sits on a flat...Ch. 4.7 - Use Newton’s Method to approximate all real...Ch. 4.7 - Use Newton’s Method to approximate all real...Ch. 4.7 - An annuity is a sequence of equal payments that...Ch. 4.7 - (a) Use a graphing utility to generate the graph...Ch. 4.7 - (a) Apply Newton’s Method to fx=x2+1 with a...Ch. 4.7 - In each part, explain what happens if you apply...Ch. 4.7 - Compare Newton’s Method and the...Ch. 4.8 - Let fx=x2x . (a) An interval on which f satisfies...Ch. 4.8 - Use the accompanying graph of f to find an...Ch. 4.8 - Let fx=x2x . (a) Find a point b such that the...Ch. 4.8 - Use the graph of f in the accompanying figure to...Ch. 4.8 - Find a function f such that the graph of f...Ch. 4.8 - Verify that the hypotheses of Rolle’s Theorem...Ch. 4.8 - Verify that the hypotheses of Rolle’s Theorem...Ch. 4.8 - Verify that the hypotheses of Rolle’s Theorem...Ch. 4.8 - Prob. 4ESCh. 4.8 - Verify that the hypotheses of the Mean-Value...Ch. 4.8 - Verify that the hypotheses of the Mean-Value...Ch. 4.8 - Verify that the hypotheses of the Mean-Value...Ch. 4.8 - Verify that the hypotheses of the Mean-Value...Ch. 4.8 - Prob. 9ESCh. 4.8 - Let fx=x34x . (a) Find the equation of the secant...Ch. 4.8 - Determine whether the statement is true or false....Ch. 4.8 - Determine whether the statement is true or false....Ch. 4.8 - Determine whether the statement is true or false....Ch. 4.8 - Determine whether the statement is true or false....Ch. 4.8 - Let fx=tanx . (a) Show that there is no point c in...Ch. 4.8 - Let fx=x2/3,a=1, and b=8 . (a) Show that there is...Ch. 4.8 - (a) Show that if f is differentiable on ,+, and if...Ch. 4.8 - Review Formulas (6) and (7) in Section 2.1 and use...Ch. 4.8 - Use the result of Exercise 18 in these exercises....Ch. 4.8 - Use the result of Exercise 18 in these exercises....Ch. 4.8 - Suppose that two runners in a 100m dash finish in...Ch. 4.8 - Use the fact that ddxxln2x=ln2xx2x to show that...Ch. 4.8 - (a) Use the Constant Difference Theorem (4.8.3) to...Ch. 4.8 - (a) Use the Constant Difference Theorem (4.8.3) to...Ch. 4.8 - Let gx=xexex . Find fx so that fx=gx and f1=2 .Ch. 4.8 - Let gx=tan1x . Find fx so that fx=gx and f1=2 .Ch. 4.8 - (a) Use the Mean-Value Theorem to show that if f...Ch. 4.8 - (a) Use the Mean-Value Theorem to show that if f...Ch. 4.8 - (a) Use the Mean-Value Theorem to show that yxyx2x...Ch. 4.8 - Show that if f is differentiable on an open...Ch. 4.8 - Use the result in Exercise 30 to show the...Ch. 4.8 - Use the inequality 31.8 to prove that 1.731.75Ch. 4.8 - Use the Mean-Value Theorem to prove that...Ch. 4.8 - (a) Show that if f and g are functions for which...Ch. 4.8 - (a) Show that if f and g are functions for which...Ch. 4.8 - Let f and g be continuous on a,b and...Ch. 4.8 - Illustrate the result in Exercise 36 by drawing an...Ch. 4.8 - (a) Prove that if fx0 for all x in a,b, then fx=0...Ch. 4.8 - (a) Prove part b of Theorem 4.1.2. (b) Prove part...Ch. 4.8 - Use the Mean-Value Theorem to prove the following...Ch. 4.8 - Let fx=3x2,x1ax+b,x1 Find the values of a and b so...Ch. 4.8 - (a) Let fx=x2,x0x2+1,00 Show that limx0fx=limx0+fx...Ch. 4.8 - Use the Mean-Value Theorem to prove the following...Ch. 4 - (a) If x1x2, what relationship must hold between...Ch. 4 - (a) What condition on f ensures that f is concave...Ch. 4 - Find: (a) the intervals on which f is increasing,...Ch. 4 - Find: (a) the intervals on which f is increasing,...Ch. 4 - Find: (a) the intervals on which f is increasing,...Ch. 4 - Find: (a) the intervals on which f is increasing,...Ch. 4 - Find: (a) the intervals on which f is increasing,...Ch. 4 - Find: (a) the intervals on which f is increasing,...Ch. 4 - Find: (a) the intervals on which f is increasing,...Ch. 4 - Find: (a) the intervals on which f is increasing,...Ch. 4 - Analyze the trigonometric function f over the...Ch. 4 - Analyze the trigonometric function f over the...Ch. 4 - Analyze the trigonometric function f over the...Ch. 4 - Analyze the trigonometric function f over the...Ch. 4 - In each part, sketch a continuous curve y=fx with...Ch. 4 - In parts (a)-(d), the graph of a polynomial with...Ch. 4 - For a general quadratic polynomial fx=ax2+bx+ca0...Ch. 4 - For the general cubic polynomial fxax3+bx2+cx+da0...Ch. 4 - Use a graphing utility to estimate the value of x...Ch. 4 - Prove that for any positive constants a and k, the...Ch. 4 - (a) Where on the graph of y=fx would you expect y...Ch. 4 - Determine whether the statement is true or false....Ch. 4 - (a) According to the first derivative test, what...Ch. 4 - Locate the critical points and identify which...Ch. 4 - Locate the critical points and identify which...Ch. 4 - Locate the critical points and identify which...Ch. 4 - In each part, find all critical points, and use...Ch. 4 - In each part, find all critical points, and use...Ch. 4 - Give a graph of the function f, and identify the...Ch. 4 - Give a graph of the function f, and identify the...Ch. 4 - Give a graph of the function f, and identify the...Ch. 4 - Give a graph of the function f, and identify the...Ch. 4 - Give a graph of the function f, and identify the...Ch. 4 - Give a graph of the function f, and identify the...Ch. 4 - Give a graph of the function f, and identify the...Ch. 4 - Give a graph of the function f, and identify the...Ch. 4 - Use any method to find the relative extrema of the...Ch. 4 - Use any method to find the relative extrema of the...Ch. 4 - Use any method to find the relative extrema of the...Ch. 4 - Use any method to find the relative extrema of the...Ch. 4 - Use any method to find the relative extrema of the...Ch. 4 - Use any method to find the relative extrema of the...Ch. 4 - Use any method to find the relative extrema of the...Ch. 4 - Use any method to find the relative extrema of the...Ch. 4 - (a) Use a graphing utility to generate the graphs...Ch. 4 - Use implicit differentiation to show that a...Ch. 4 - Let fx=2x3+x215x+72x13x2+x1 Graph y=fx, and find...Ch. 4 - Let fx=x5x43x3+2x+4x72x63x5+6x4+4x8 (a) Use a CAS...Ch. 4 - (a) What inequality must fx satisfy for the...Ch. 4 - According to the Extreme-Value Theorem, what...Ch. 4 - In each part, determine whether the statement is...Ch. 4 - In each part, find the absolute minimum m and the...Ch. 4 - In each part, find the absolute minimum m and the...Ch. 4 - In each part, find the absolute minimum m and the...Ch. 4 - In each part, use a graphing utility to estimate...Ch. 4 - Prob. 58RECh. 4 - Let fx=x3+2x4+1 (a) Generate the graph of y=fx,...Ch. 4 - A church window consists of a blue semicircular...Ch. 4 - Find the dimensions of the rectangle of maximum...Ch. 4 - As shown in the accompanying figure on the next...Ch. 4 - A sheet of cardboard 12in square is used to make...Ch. 4 - Is it true or false that a particle in rectilinear...Ch. 4 - (a) Can an object in rectilinear motion reverse...Ch. 4 - Suppose that the position function of a particle...Ch. 4 - For parts (a)-(f), suppose that the position...Ch. 4 - Use Newton’s Method to approximate all three...Ch. 4 - Use Newton’s Method to approximate the smallest...Ch. 4 - Use a graphing utility to determine the number of...Ch. 4 - According to Kepler’s law, the planets in our...Ch. 4 - Using the formulas in Exercise 72, find the...Ch. 4 - Suppose that f is continuous on the closed...Ch. 4 - Prob. 75RECh. 4 - In each part, determine whether all of the...Ch. 4 - Use the fact that ddxx62x2+x=6x54x+1 to show that...Ch. 4 - Let fx=x34x+6 . Find fx so that fx=gx and f1=2 .

Additional Math Textbook Solutions

Find more solutions based on key concepts
Find the exact value of expression.

Precalculus Enhanced with Graphing Utilities

Length of a Guy Wire A communications tower is located at the top of a steep hill, as shown. The angle of incli...

Precalculus: Mathematics for Calculus - 6th Edition

The amplitude, period and frequency.

Precalculus

Continuity and limits with transcendental functions Determine the interval(s) on which the following functions ...

Single Variable Calculus: Early Transcendentals (2nd Edition) - Standalone book

The intercepts of the equation 9 x 2 +4y=36 are ______. (pp.18-19)

Precalculus Enhanced with Graphing Utilities (7th Edition)

The domain of the function hx=log2x2+4 in interval notation.

Precalculus

Knowledge Booster
Background pattern image
Calculus
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.
Similar questions
Recommended textbooks for you
Text book image
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781305652224
Author:Charles P. McKeague, Mark D. Turner
Publisher:Cengage Learning
Text book image
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Text book image
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Text book image
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
SEE MORE TEXTBOOKS
Finding Local Maxima and Minima by Differentiation; Author: Professor Dave Explains;https://www.youtube.com/watch?v=pvLj1s7SOtk;License: Standard YouTube License, CC-BY