Chapter 4.5, Problem 39P

4.39 and 4.40 A copper strip (E_c = 105 GPa) and an aluminum strip (E_a = 75 GPa) are bonded together to form the composite beam shown. Knowing that the beam is bent about a horizontal axis by a couple of moment M = 35 N·m, determine the maximum stress in (a) the aluminum strip, (b) the copper strip.

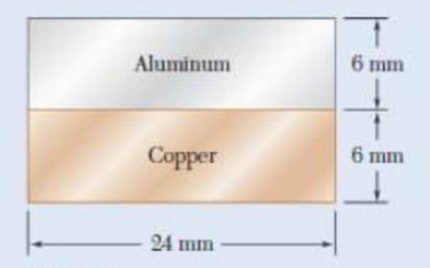
Fig. P4.39

To determine

Find the maximum stress in the aluminum strip.

Answer to Problem 39P

The maximum stress in the aluminum strip is $\underset{\_}{-56\text{MPa}}$.

Explanation of Solution

Given information:

The modulus of elasticity $\left(E_a\right)$ of Aluminum is $\text{105}\text{GPa}$.

The modulus of elasticity $\left(E_c\right)$ of Copper is $\text{75}\text{GPa}$.

The moment (M) in the beam is $35\text{N}\cdot \text{m}$.

Calculation:

Show the cross-section of the composite bar as shown in Figure 1.

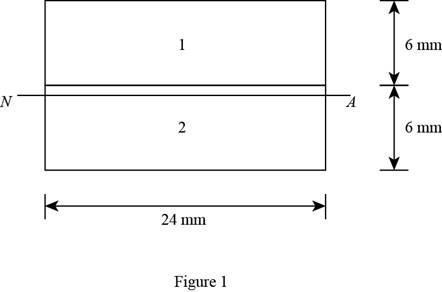
Refer Figure 1.

Consider the Copper and Aluminum is represented by rectangle 2 and 1.

The width and depth of the rectangle 1 are $b_1=24\text{ mm}$ and $d_1=6\text{mm}$.

The width and depth of the rectangle 2 are $b_2=24\text{mm}$ and $d_1=6\text{mm}$.

Consider Aluminum as the reference material, then the value of $n_1=1$.

Calculate the ratio $n_1$ for Copper using the relation:

$n_1=\frac{E_c}{E_a}$ (1)

Substitute $\text{105}\text{GPa}$ for $E_c$ and $\text{75}\text{GPa}$ for $E_a$ in Equation (1).

$\begin{array}{c}{n}_1=\frac{\text{105}\text{GPa}}{\text{75}\text{GPa}}\\ =1.4\end{array}$

Calculate the distance ($Y_0$) between the neutral axis and the bottom of the beam using the relation:

${\overline{Y}}_0=\left(\frac{n_1{A}_1{y}_1+n_2{A}_2{y}_2}{n_1{A}_1+n_2{A}_2}\right)$ (2)

Here, $y_1$ and $y_2$ are the distance between the centroid of the rectangle 1 and 2 and the neutral axis.

The value of $y_1$ and $y_2$ are $9\text{mm}$ and $3\text{mm}$.

Substitute $\left(24\text{ mm}\times \text{6}\text{mm}\right)$ for $A_1$, $\left(24\text{ mm}\times \text{6}\text{mm}\right)$ for $A_2$, 1.4 for $n_1$, 1 for $n_2$, $9\text{mm}$ for $y_1$, $3\text{mm}$ for $y_2$ in Equation (2).

$\begin{array}{c}{\overline{Y}}_0=\left(\frac{1\times \left(24\times \text{6}\right)\times 9+1.4\times \left(24\times \text{6}\right)\times 3}{1.4\times \left(24\times \text{6}\right)+1\times \left(24\times \text{6}\right)}\right)\\ =\frac{1296+604.8}{201.6+144}\\ =\frac{1900.8}{345.6}\\ =5.5\text{mm}\end{array}$

Consider the entire cross-section of the composite bar is transformed into Aluminum.

Calculate the moment of inertia $\left(I_1\right)$ of the rectangle 1 using the relation:

$I_1=\frac{n_1}{12}{b}_1{d}_1^3+n_1{A}_1{\left(y_1-{\overline{Y}}_0\right)}^2$ (3)

Substitute $24\text{mm}$ for $b_1$, $6\text{mm}$ for $d_1$, $1$ for $n_1$, $5.5\text{mm}$ for ${\overline{Y}}_0$, and $9\text{mm}$ for $y_1$ in Equation (3).

$\begin{array}{c}{I}_1=\frac{1}{12}\times 24\times 6^3+24\times 6\times {\left(9-5.5\right)}^2\\ =432+1,764\\ =2196{\text{mm}}^4\end{array}$

Calculate the moment of inertia $\left(I_2\right)$ of the rectangle 2 using the relation:

$I_2=\frac{n_2}{12}{b}_2{d}_2^3+n_2{A}_2{\left({\overline{Y}}_0-y_2\right)}^2$ (4)

Substitute $24\text{mm}$ for $b_1$, $6\text{mm}$ for $d_1$, $1$ for $n_1$, $5.5\text{mm}$ for ${\overline{Y}}_0$, and $3\text{mm}$ for $y_2$ in Equation (4).

$\begin{array}{c}{I}_2=\frac{1.4}{12}\times 24\times 6^3+1.4\times 24\times 6\times {\left(5.5-3\right)}^2\\ =604.8+1,260\\ =1,864.8{\text{mm}}^4\end{array}$

Calculate the moment of inertia of the transformed cross-section using the relation:

$I=I_1+I_2$ (5)

Substitute $2,196{\text{mm}}^4$ for $I_1$, and $1,864.8{\text{mm}}^4$ for $I_2$ in Equation (5).

$\begin{array}{c}I=2,196{\text{mm}}^4+1,864.8{\text{mm}}^4\\ =4,060.8{\text{mm}}^4\end{array}$

Calculate the maximum stress ($\sigma$) in the aluminum strip using the relation:

$\sigma =-\frac{nMy}{I}$ (6)

Calculate the maximum stress for Aluminum strip as follows:

Substitute $35\text{N}\cdot \text{m}$ for $M$, $4,060.8{\text{mm}}^4$ for I, 1 for $n$ and $6.5\text{mm}$ for y in Equation (6).

$\begin{array}{c}\sigma =-\frac{1\times 35\text{N}\cdot \text{m}\times 6.5\text{mm}\times \left(\frac{1\text{m}}{1,000\text{mm}}\right)}{4,060.8{\text{mm}}^4\times \left(\frac{1{\text{m}}^4}{{10}^{-12}{\text{mm}}^4}\right)}\\ =-\frac{1\times 35\text{N}\cdot \text{m}\times 6.5\text{mm}\times \left(\frac{1\text{m}}{1,000\text{mm}}\right)}{4,060.8{\text{mm}}^4\times \left(\frac{1{\text{m}}^4}{{10}^{12}{\text{mm}}^4}\right)}\\ =-\left(\frac{35\times 6.5\times {10}^{-3}}{4,060.8\times {10}^{-12}}\right)\end{array}$

$\begin{array}{c}\sigma =-56\times {10}^6\frac{\text{N}}{{\text{mm}}^2}\times \left(\frac{1\text{MPa}}{{10}^6\frac{\text{N}}{{\text{mm}}^2}}\right)\\ =-56\text{MPa}\end{array}$

Thus, the maximum stress in the aluminum strip is $\underset{\_}{-56\text{MPa}}$.

To determine

Find the maximum stress in the aluminum strip.

Answer to Problem 39P

The maximum stress in the aluminum strip is $\underset{\_}{66.4\text{MPa}}$.

Explanation of Solution

Given information:

The modulus of elasticity $\left(E_a\right)$ of Aluminum is $\text{105}\text{GPa}$.

The modulus of elasticity $\left(E_c\right)$ of Copper is $\text{75}\text{GPa}$.

The moment (M) in the beam is $35\text{N}\cdot \text{m}$.

Calculation:

Calculate the maximum stress for Copper strip as follows:

Substitute $35\text{N}\cdot \text{m}$ for $M$, $4,060.8{\text{mm}}^4$ for I, 1.4 for $n$ and $-5.5\text{mm}$ for y in Equation (6).

$\begin{array}{c}\sigma =-\frac{1.4\times 35\text{N}\cdot \text{m}\times \left(-5.5\text{mm}\right)\times \left(\frac{1\text{m}}{1,000\text{mm}}\right)}{4,060.8{\text{mm}}^4\times \left(\frac{1{\text{m}}^4}{{10}^{-12}{\text{mm}}^4}\right)}\\ =\frac{1.4\times 35\text{N}\cdot \text{m}\times 5.5\text{mm}\times \left(\frac{1\text{m}}{1,000\text{mm}}\right)}{4,060.8{\text{mm}}^4\times \left(\frac{1{\text{m}}^4}{{10}^{12}{\text{mm}}^4}\right)}\\ =\left(\frac{1.4\times 35\times 5.5\times {10}^{-3}}{4,060.8\times {10}^{-12}}\right)\end{array}$

$\begin{array}{c}\sigma =66.4\times {10}^6\frac{\text{N}}{{\text{mm}}^2}\times \left(\frac{1\text{MPa}}{{10}^6\frac{\text{N}}{{\text{mm}}^2}}\right)\\ =66.4\text{MPa}\end{array}$

Thus, the maximum stress in the copper strip is $\underset{\_}{66.4\text{MPa}}$.