EP ENGR.MECH.:DYNAMICS-REV.MOD.MAS.ACC.
14th Edition
ISBN: 9780133976588
Author: HIBBELER
Publisher: PEARSON CO
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 22.6, Problem 41P
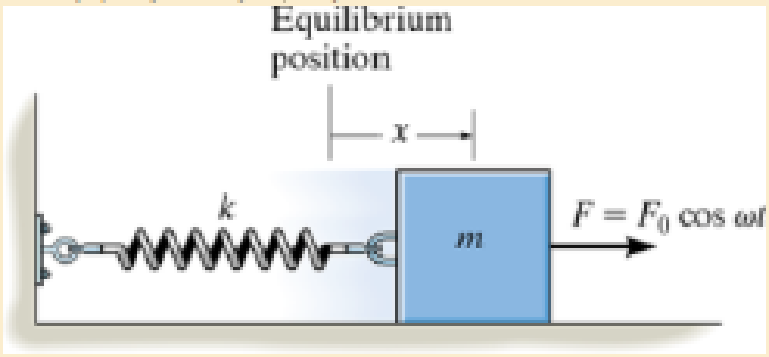
If the block-and-spring model is subjected to the periodic force F=F0 cos ωt, show that the differential equation of motion is ẍ + (k/m)x = (F0/m) cos ωt , where x is measured from the equilibrium position of the block. What is the general solution of this equation?
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
۳/۱
العنوان
O
не
شكا
+91x PU + 96852
A heavy car plunges into a lake during an accident and lands at the bottom of the lake
on its wheels as shown in figure. The door is 1.2 m high and I m wide, and the top edge of
Deine the hadrostatic force on the
Plot the displacement diagram for a cam with roller follower of diameter 10 mm. The required
motion is as follows;
1- Rising 60 mm in 135° with uniform acceleration and retardation motion.
2- Dwell 90°
3- Falling 60 mm for 135° with Uniform acceleration-retardation motion.
Then design the cam profile to give the above displacement diagram if the minimum circle
diameter of the cam is 50 mm.
=
-20125
750 x2.01
Plot the displacement diagram for a cam with roller follower of diameter 10 mm. The required
motion is as follows;
1- Rising 60 mm in 135° with uniform acceleration and retardation motion.
2- Dwell 90°
3- Falling 60 mm for 135° with Uniform acceleration-retardation motion.
Then design the cam profile to give the above displacement diagram if the minimum circle
diameter of the cam is 50 mm.
Q1/ A vertical, circular gate with water on one side as shown. Determine
the total resultant force acting on the gate and the location of the center of
pressure, use water specific weight 9.81 kN/m³
1 m
4 m
Chapter 22 Solutions
EP ENGR.MECH.:DYNAMICS-REV.MOD.MAS.ACC.
Ch. 22.1 - A spring is stretched 175 mm by an 8-kg block. If...Ch. 22.1 - Prob. 2PCh. 22.1 - A spring is stretched 200 mm by a 15-kg block. If...Ch. 22.1 - When a 20-lb weight is suspended from a spring,...Ch. 22.1 - Prob. 5PCh. 22.1 - Prob. 6PCh. 22.1 - Prob. 7PCh. 22.1 - Prob. 8PCh. 22.1 - A 3-kg block is suspended from a spring having a...Ch. 22.1 - Prob. 10P
Ch. 22.1 - Prob. 11PCh. 22.1 - 22-12. Determine the natural period of vibration...Ch. 22.1 - The body of arbitrary shape has a mass m, mass...Ch. 22.1 - Determine the torsional stiffness k, measured in...Ch. 22.1 - Prob. 15PCh. 22.1 - Prob. 16PCh. 22.1 - If the natural periods of oscillation of the...Ch. 22.1 - Prob. 18PCh. 22.1 - Prob. 19PCh. 22.1 - A uniform board is supported on two wheels which...Ch. 22.1 - If the wire AB is subjected to a tension of 20 lb,...Ch. 22.1 - The bar has a length l and mass m. It is supported...Ch. 22.1 - The 20-kg disk, is pinned at its mass center O and...Ch. 22.1 - Prob. 24PCh. 22.1 - If the disk in Prob. 22-24 has a mass of 10 kg,...Ch. 22.1 - Prob. 26PCh. 22.1 - Prob. 27PCh. 22.1 - Prob. 28PCh. 22.1 - Prob. 29PCh. 22.2 - Determine the differential equation of motion of...Ch. 22.2 - Determine the natural period of vibration of the...Ch. 22.2 - Determine the natural period of vibration of the...Ch. 22.2 - Prob. 33PCh. 22.2 - Determine the differential equation of motion of...Ch. 22.2 - Prob. 35PCh. 22.2 - Prob. 36PCh. 22.2 - Prob. 37PCh. 22.2 - Prob. 38PCh. 22.2 - Prob. 39PCh. 22.2 - If the slender rod has a weight of 5 lb, determine...Ch. 22.6 - If the block-and-spring model is subjected to the...Ch. 22.6 - Prob. 42PCh. 22.6 - A 4-lb weight is attached to a spring having a...Ch. 22.6 - Prob. 44PCh. 22.6 - Prob. 45PCh. 22.6 - Prob. 46PCh. 22.6 - Prob. 47PCh. 22.6 - Prob. 48PCh. 22.6 - Prob. 49PCh. 22.6 - Prob. 50PCh. 22.6 - The 40-kg block is attached to a spring having a...Ch. 22.6 - The 5kg circular disk is mounted off center on a...Ch. 22.6 - Prob. 53PCh. 22.6 - Prob. 54PCh. 22.6 - Prob. 55PCh. 22.6 - Prob. 56PCh. 22.6 - Prob. 57PCh. 22.6 - Prob. 58PCh. 22.6 - Prob. 59PCh. 22.6 - The 450-kg trailer is pulled with a constant speed...Ch. 22.6 - Prob. 61PCh. 22.6 - Prob. 62PCh. 22.6 - Prob. 63PCh. 22.6 - The spring system is connected to a crosshead that...Ch. 22.6 - Prob. 65PCh. 22.6 - Prob. 66PCh. 22.6 - Prob. 67PCh. 22.6 - The 200-lb electric motor is fastened to the...Ch. 22.6 - Prob. 69PCh. 22.6 - If two of these maximum displacements can be...Ch. 22.6 - Prob. 71PCh. 22.6 - Prob. 72PCh. 22.6 - Prob. 73PCh. 22.6 - Prob. 74PCh. 22.6 - Prob. 75PCh. 22.6 - Prob. 76PCh. 22.6 - Prob. 77PCh. 22.6 - Prob. 78PCh. 22.6 - Prob. 79P
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- I need handwritten solution with sketches for eacharrow_forwardGiven answers to be: i) 14.65 kN; 6.16 kN; 8.46 kN ii) 8.63 kN; 9.88 kN iii) Bearing 6315 for B1 & B2, or Bearing 6215 for B1arrow_forward(b) A steel 'hot rolled structural hollow section' column of length 5.75 m, has the cross-section shown in Figure Q.5(b) and supports a load of 750 kN. During service, it is subjected to axial compression loading where one end of the column is effectively restrained in position and direction (fixed) and the other is effectively held in position but not in direction (pinned). i) Given that the steel has a design strength of 275 MN/m², determine the load factor for the structural member based upon the BS5950 design approach using Datasheet Q.5(b). [11] ii) Determine the axial load that can be supported by the column using the Rankine-Gordon formula, given that the yield strength of the material is 280 MN/m² and the constant *a* is 1/30000. [6] 300 600 2-300 mm wide x 5 mm thick plates. Figure Q.5(b) L=5.75m Pinned Fixedarrow_forward
- Q1: For the following force system, find the moments with respect to axes x, y, and zarrow_forwardQ10) Body A weighs 600 lb contact with smooth surfaces at D and E. Determine the tension in the cord and the forces acting on C on member BD, also calculate the reaction at B and F. Cable 6' 3' wwwarrow_forwardHelp ارجو مساعدتي في حل هذا السؤالarrow_forward
- Q3: Find the resultant of the force system.arrow_forwardQuestion 1 A three-blade propeller of a diameter of 2 m has an activity factor AF of 200 and its ratio of static thrust coefficient to static torque coefficient is 10. The propeller's integrated lift coefficient is 0.3.arrow_forward(L=6847 mm, q = 5331 N/mm, M = 1408549 N.mm, and El = 8.6 x 1014 N. mm²) X A ΕΙ B L Y Marrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
International Edition---engineering Mechanics: St...
Mechanical Engineering
ISBN:9781305501607
Author:Andrew Pytel And Jaan Kiusalaas
Publisher:CENGAGE L
Ch 2 - 2.2.2 Forced Undamped Oscillation; Author: Benjamin Drew;https://www.youtube.com/watch?v=6Tb7Rx-bCWE;License: Standard youtube license