Chapter 2.2, Problem 22E

To determine

To calculate: The $\underset{x\to 0}{\lim }\frac{\tan 3x}{\tan 5x}$ . Also confirm the result graphically.

Answer to Problem 22E

  $\underset{x\to 0}{\lim }\frac{\tan 3x}{\tan 5x}=\frac{3}{5}$ .

Explanation of Solution

Given information:

Given limit as $\underset{x\to 0}{\lim }\frac{\tan 3x}{\tan 5x}$

Consider the limits as, $\underset{x\to 0}{\lim }\frac{\tan 3x}{\tan 5x}$

Since, $\tan x=x+\frac{1}{3}{x}^3+\frac{2}{15}{x}^5+\mathrm{...}$

Hence, $\underset{x\to 0}{\lim }\frac{\tan 3x}{\tan 5x}=\underset{x\to 0}{\lim }\frac{\left(3x\right)+\frac{1}{3}{\left(3x\right)}^3+\frac{2}{15}{\left(3x\right)}^5+\mathrm{...}}{\left(5x\right)+\frac{1}{3}{\left(5x\right)}^3+\frac{2}{15}{\left(5x\right)}^5+\mathrm{...}}$

Then,

  $\begin{array}{c}\underset{x\to 0}{\lim }\frac{\tan 3x}{\tan 5x}=\underset{x\to 0}{\lim }\frac{3x+9x^3+\frac{162}{5}{x}^5+\mathrm{...}}{5x+\frac{125}{3}{x}^3+\frac{1250}{3}{x}^5+\mathrm{...}}\\ =\underset{x\to 0}{\lim }\frac{x\left(3+9x^2+\frac{162}{5}{x}^4+\mathrm{...}\right)}{x\left(5+\frac{125}{3}{x}^2+\frac{1250}{3}{x}^4+\mathrm{...}\right)}\\ =\underset{x\to 0}{\lim }\frac{3+9x^2+\frac{162}{5}{x}^4+\mathrm{...}}{5+\frac{125}{3}{x}^2+\frac{1250}{3}{x}^4+\mathrm{...}}\\ =\frac{3+0+0+\mathrm{...}}{5+0+0+\mathrm{...}}\\ =\frac{3}{5}\\ =0.6\end{array}$

Graph of the function is,

  

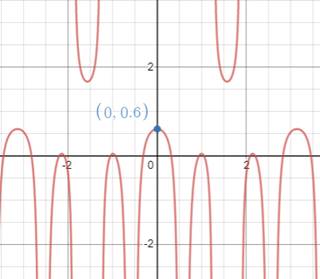
Therefore, $\underset{x\to 0}{\lim }\frac{\tan 3x}{\tan 5x}=\frac{3}{5}$