
Concept explainers
Repeal Problem 59 for the case where the charge density in the slab is given by ρ = ρ0|x/d|, where ρ0 is a constant.
59. A charged slab extends infinitely in two dimensions and has thickness d in the third dimension, as shown in Fig. 21.36. The slab carries a uniform volume charge density ρ. Find expressions for the electric field (a) inside and (b) outside the slab, as functions of the distance x from the center plane. (Although the infinite slab is impossible, your answer is a good approximation to the field of a finite slab whose width is much greater than its thickness.)
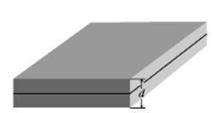
FIGURE 21.36 Problems 59 and 75
Want to see the full answer?
Check out a sample textbook solution
Chapter 21 Solutions
Essential University Physics (3rd Edition)
Additional Science Textbook Solutions
Campbell Biology (11th Edition)
Campbell Essential Biology (7th Edition)
Biology: Life on Earth (11th Edition)
Chemistry & Chemical Reactivity
Human Biology: Concepts and Current Issues (8th Edition)
Anatomy & Physiology (6th Edition)
- The magnitude of the force between a pair of point charges is proportional to the product of the magnitudes of their charges and inversely proportional to the square of their separation distance. Four distinct charge-pair arrangements are presented. All charges are multiples of a common positive charge, q. All charge separations are multiples of a common length, L. Rank the four arrangements from smallest to greatest magnitude of the electric force.arrow_forwardA number of electric charges has been placed at distinct points along a line with separations as indicated. Two charges share a common magnitude, q (lower case), and another charge has magnitude Q (upper case). The signs of the charges are indicated explicitly such that ∣∣+q∣∣∣∣+Q∣∣=∣∣−q∣∣==∣∣−Q∣∣=qQ Four different configurations of charges are shown. For each, express the net electric force on the charge with magnitude Q (upper case) as F⃗E=FE,xî where the positive x direction is towards the right. By repositioning the figures to the area on the right, rank the configurations from the most negative value to the most positive value of FE,x.arrow_forwardA collection of electric charges that share a common magnitude q (lower case) has been placed at the corners of a square, and an additional charge with magnitude Q (upper case) is located at the center of that square. The signs of the charges are indicated explicitly such that ∣∣+q∣∣∣∣+Q∣∣=∣∣−q∣∣==∣∣−Q∣∣=qQ Four unique setups of charges are displayed. By moving one of the direction drawings from near the bottom to the bucket beside each of the setups, indicate the direction of the net electric force on the charge with magnitude Q, located near the center, else indicate that the magnitude of the net electric force is zero, if appropriate.arrow_forward
- In Dark Souls 3 you can kill the Ancient Wyvern by dropping on its head from above it. Let’s say you jump off the ledge with an initial velocity of 3.86 mph and spend 1.72 s in the air before hitting the wyvern’s head. Assume the gravity is the same as that of Earth and upwards is the positive direction. Also, 1 mile = 1609 m. A) How high up is the the ledge you jumped from as measured from the wyvern’s head? B) What is your velocity when you hit the wyvern?arrow_forwardA conducting sphere is mounted on an insulating stand, and initially it is electrically neutral. A student wishes to induce a charge distribution similar to what is shown here. The student may connect the sphere to ground or leave it electrically isolated. The student may also place a charged insulated rod near to the sphere without touching it. Q. The diagrams below indicate different choices for whether or not to include a ground connection as well as the sign of the charge on and the placement of an insulating rod. Choose a diagram that would produce the desired charge distribution. (If there are multiple correct answers, you need to select only one of them.)arrow_forwardA person is making pancakes and tries to flip one in the pan. The person is holding the pan a distance y0 = 1.10 m above the ground when they launch the pancake. The pancake just barely touches the ceiling, which is at a height y = 2.47 m above the ground. A) What must be the initial velocity of the pancake to reach that height? B) This person, shocked that they almost hit the ceiling, does not catch it on the way down and the pancake hits the floor. Assuming up as the positive direction, what is the velocity of the pancake when it hits the floor, ruining breakfast and this person’s day?arrow_forward
- One of Spider-Man’s less talked about powers is that he can jump really high. In the comics Spider-Man can jump upwards 3 stories. A) If Spider-Man leaves the ground at 14.3 m/s, how high can he get? y= B) If Spider-Man jumps directly upwards with the initial velocity used above and then returns to the ground, what total amount of time does he spend airborn? t=arrow_forwardAn insulating rod is positively charged, and an electrically neutral conducting sphere is mounted on an insulating stand. The rod is brought near to the sphere on the right, but they never actually touch. Q. Select the image that best represents the resulting charge distribution on the conducting sphere.arrow_forwardThis is a multi-part problem. For each part make sure to include sign to represent direction, with up being positive and down being negative. A ball is thrown vertically upward with a speed of 30.5 m/s. A) How high does it rise? y= B) How long does it take to reach its highest point? t= C) How long does it take the ball return to its starting point after it reaches its highest point? t= D) What is its velocity when it returns to the level from which it started? v=arrow_forward
- Blue light has a wavelength of 485 nm. What is the frequency of a photon of blue light? Question 13 Question 13 What is the wavelength of radiofrequency broadcast of 104 MHz? Question 14 Question 14 1 Point 3. The output intensity from an x-ray exposure is 4 mGy at 90 cm. What will the intensity of the exposure be at 180 cm? Question 15 Question 15 1 Point What is the frequency of an 80 keV x-ray?arrow_forwardUnder what condition is IA - BI = A + B? Vectors À and B are in the same direction. Vectors À and B are in opposite directions. The magnitude of vector Vectors À and 官 B is zero. are in perpendicular directions.arrow_forwardFor the vectors shown in the figure, express vector 3 in terms of vectors M and N. M S =-M+ Ň == S=м- Ñ S = M +Ñ +Narrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
 Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





