Chapter 2, Problem 6RE

House freshmen: Newly elected members of the U.S. House of Representatives are referred to as “freshmen.” The following frequency distribution presents the number of freshmen elected in each of the past 53 elections, from 1912 to 2016.

1. How many classes are there?
2. What is the class width?
3. What are the class limits?
4. Construct a frequency histogram.
5. Construct a relative frequency distribution.
6. Construct a relative frequency histogram.
7. In what percentage of elections were loo or more freshmen elected?
8. In what percentage of elections were fewer than 60 freshmen elected?

To determine

To find:Number of classes.

Answer to Problem 6RE

The number of classes is 8.

Explanation of Solution

Given information:The following frequency distribution presents the numberof freshmen elected in each of the past 53 elections from 1912 to 2016.

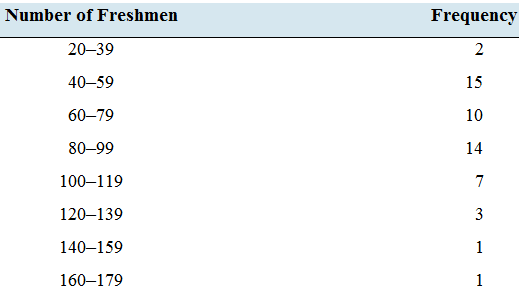
Number of Freshmen	Frequency
20-39	2
40-59	15
60-79	10
80-99	14
100-119	7
120-139	3
140-159	1
160-179	1

Calculation:

The classes from the given table are 20-39, 40-59, 60-79, 80-99, 100-119, 120-139, 140-159 and 160-179.

There are eight classes are there.

Hence, the number of classes is 8.

To determine

To find: The class width.

Answer to Problem 6RE

The class width is 19.

Explanation of Solution

Given information: The following frequency distribution presents the number of freshmen elected in each of the past 53 elections from 1912 to 2016.

Number of Freshmen	Frequency
20-39	2
40-59	15
60-79	10
80-99	14
100-119	7
120-139	3
140-159	1
160-179	1

Formula used: $classwidth=\frac{largestdatavalue–smallestdatavalue}{Numberofclasses}$

Calculation:

From the given table, the largest value is 179 and the smallest value is 20.

The number of classes is 8.

Then the class width is given by

  $\begin{array}{c}classwidth=\frac{largestdatavalue–smallestdatavalue}{Numberofclasses}\\ classwidth=\frac{179-20}{8}\\ =\frac{159}{8}\\ =19.875\\ \simeq 19\end{array}$

Hence, the class width is 19.

To determine

To find: The class limits.

Answer to Problem 6RE

The lower class limits are 20, 40, 60, 80, 100, 120, 140 and 160.

The upper class limits are 39, 59, 79, 99, 119, 139, 159 and 179.

Explanation of Solution

Given information: The following frequency distribution presents the number of freshmen elected in each of the past 53 elections from 1912 to 2016.

Number of Freshmen	Frequency
20-39	2
40-59	15
60-79	10
80-99	14
100-119	7
120-139	3
140-159	1
160-179	1

Definition used:

The lower class limits of a class are the smallest value that can appear in that class.

The upper class limits of a class are the largest value that can appear in that class.

Calculation:

From the given table we can say that

The lower class limits are 20, 40, 60, 80, 100, 120, 140 and 160.

The upper class limits are 39,59, 79, 99, 119, 139, 159 and 179.

To determine

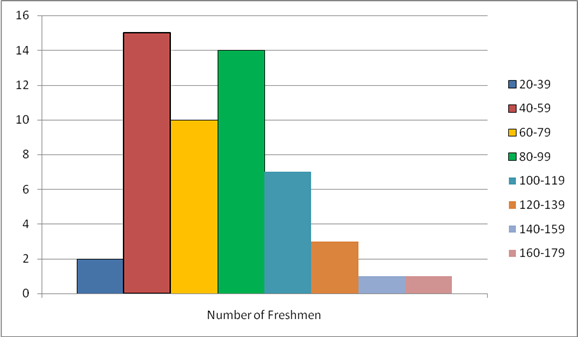
To construct: A frequency histogram.

Explanation of Solution

Given information: The following frequency distribution presents the number of freshmen elected in each of the past 53 elections from 1912 to 2016.

Number of Freshmen	Frequency
20-39	2
40-59	15
60-79	10
80-99	14
100-119	7
120-139	3
140-159	1
160-179	1

Definition used:

Histogram based on frequency is called frequency histogram.

Solution:

From the given table, the frequency histogram is given by

  

To determine

To construct: The relative frequency distribution.

Explanation of Solution

Given information: The following frequency distribution presents the number of freshmen elected in each of the past 53 elections from 1912 to 2016.

Number of Freshmen	Frequency
20-39	2
40-59	15
60-79	10
80-99	14
100-119	7
120-139	3
140-159	1
160-179	1

Formula used: $Relativefrequency=\frac{Frequency}{}$

  $Relativefrequency=\frac{Frequency}{ Sum\text{ of all }Frequency}$

Calculation:

From the given table,

The sum of all frequency is $2+15+10+14+7+3+1+1=53$

The table of relative frequency is given by

Number of Freshmen	Frequency	Relative Frequency
20-39	2	$\frac{2}{53}=0.0377$
40-59	15	$\frac{15}{53}=0.2830$
60-79	10	$\frac{10}{53}=0.1887$
80-99	14	$\frac{14}{53}=0.2642$
100-119	7	$\frac{7}{53}=0.1321$
120-139	3	$\frac{3}{53}=0.0566$
140-159	1	$\frac{1}{53}=0.0189$
160-179	1	$\frac{1}{53}=0.0189$

To determine

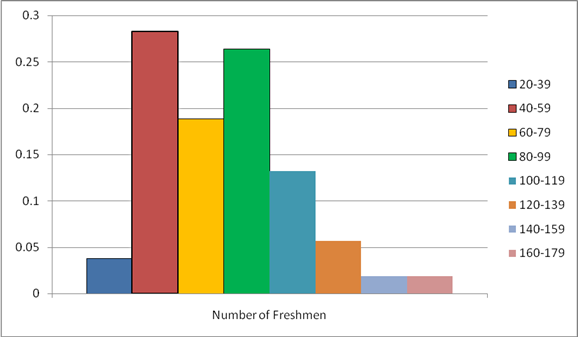
To construct: A relative frequency histogram.

Explanation of Solution

Given information: The following frequency distribution presents the number of freshmen elected in each of the past 53 elections from 1912 to 2016.

Number of Freshmen	Frequency
20-39	2
40-59	15
60-79	10
80-99	14
100-119	7
120-139	3
140-159	1
160-179	1

Definition used:

Histogram based on relative frequency is called relative frequency histogram.

Solution:

Number of Freshmen	Frequency	Relative Frequency
20-39	2	$0.0377$
40-59	15	$0.2830$
60-79	10	$0.1887$
80-99	14	$0.2642$
100-119	7	$0.1321$
120-139	3	$0.0566$
140-159	1	$0.0189$
160-179	1	$0.0189$

From the above table, the relative frequency histogram is given by

  

To determine

To find: The percentage of elections was 100 or more freshmen elected.

Answer to Problem 6RE

The percentage of elections was 100 or more freshmen elected is $22.6\%$ .

Explanation of Solution

Given information: The following frequency distribution presents the number of freshmen elected in each of the past 53 elections from 1912 to 2016.

Number of Freshmen	Frequency
20-39	2
40-59	15
60-79	10
80-99	14
100-119	7
120-139	3
140-159	1
160-179	1

Definition used:

Percentage for a frequency is given by

  $Percentage=\frac{Frequency}{ Sum\text{ of all }Frequency}\times 100\%$

Calculation:

From the given table we can say that

The frequency for 100 or more freshmen elected is 7, 3, 1 and 1.

The sum of all frequencies of 100 or more freshmen were elected is $7+3+1+1=12$

The sum of all frequency is 53.

Hence, the percentage is given by

  $\begin{array}{c} Percentage=\frac{Frequency}{ Sum\text{ of all }Frequency}\times 100\%\\ =\frac{12}{53}\times 100\%\\ =0.226\times 100\%\\ =22.6\%\end{array}$

Hence, the percentage of elections was 100 or more freshmen electedis $22.6\%$ .

To determine

To find: The percentage of elections was fewer than 60 freshmen elected.

Answer to Problem 6RE

Thepercentage of elections was fewer than 60 freshmen elected is $32\%$ .

Explanation of Solution

Given information: The following frequency distribution presents the number of freshmen elected in each of the past 53 elections from 1912 to 2016.

Number of Freshmen	Frequency
20-39	2
40-59	15
60-79	10
80-99	14
100-119	7
120-139	3
140-159	1
160-179	1

Definition used:

Percentage for a frequency is given by

  $Percentage=\frac{Frequency}{ Sum\text{ of all }Frequency}\times 100\%$

Calculation:

From the given table we can say that

The frequency for fewer than 60 freshmen elected is 2 and 15.

The sum of all frequencies of fewer than 60 freshmen were elected is $2+15=17$

The sum of all frequency is 53.

Hence, the percentage is given by

  $\begin{array}{c} Percentage=\frac{Frequency}{ Sum\text{ of all }Frequency}\times 100\%\\ =\frac{17}{53}\times 100\%\\ =0.32\times 100\%\\ =32\%\end{array}$

Hence, the percentage of elections was fewer than 60 freshmen elected is $32\%$ .