Chapter 1.7, Problem 69E

To determine

To solve: the nonlinear inequality. Express the solution using interval notation and graph the solution set.

Answer to Problem 69E

  $x\in \left(-3,-\frac{1}{2}\right)\cup \left(2,\infty \right)$ .

Explanation of Solution

Given:

The given inequality is $\frac{x+2}{x+3}<\frac{x-1}{x-2}$ .

Concept used:

Guidelines for solving nonlinear inequality:

1. Move all terms to one side.
2. Factor the non-zero side of the inequality.
3. Find the value for which each factor is zero. The number will divide the real lines into interval. List the interval determined by these numbers.
4. Make a table or diagram by using test values of the signs of each factor on each interval. In the last row of the table determining the sign of the product of these factors.
5. Determine the solution of the inequality from the last row of the sign table.

Calculation:

The given inequality can be expressed as

  $\begin{array}{l}\frac{x+2}{x+3}-\frac{x-1}{x-2}<0\left\{\text{subtract both sides from }\frac{x-1}{x-2}\right\}\\ \Rightarrow \frac{\left(x+2\right)\left(x-2\right)-\left(x+3\right)\left(x-1\right)}{\left(x+3\right)\left(x-2\right)}<0\left\{\text{simplify}\right\}\\ \Rightarrow \frac{x^2-4-\left(x^2+2x-3\right)}{\left(x+3\right)\left(x-2\right)}<0\\ \Rightarrow \frac{x^2-4-x^2-2x+3}{\left(x+3\right)\left(x-2\right)}<0\\ \therefore \frac{-2x-1}{\left(x+3\right)\left(x-2\right)}<0\end{array}$ .

Multiply both sides by $-1$ and reverse the inequality symbol.

  $\frac{2x+1}{\left(x+3\right)\left(x-2\right)}>0$

Firstto find the zeros of the expression in the numerator and demniminator, then

  $\begin{array}{l}2x+1=0\Rightarrow x=-\frac{1}{2}\\ x+3=0\Rightarrow x=-3\\ x-2=0\Rightarrow x=2\end{array}$

From the three zeros above, it extracts the following intervals:

  $\left(-\infty ,-3\right)\left(-3,-\frac{1}{2}\right)\left(-\frac{1}{2},2\right)\left(2,\infty \right)$

Now, make a table by using test values of the signs of each factor on each interval.

	$\left(-\infty ,-3\right)$	$\left(-3,-\frac{1}{2}\right)$	$\left(-\frac{1}{2},2\right)$	$\left(2,\infty \right)$
$2x+1$	$-$	$-$	+	+
$\left(x+3\right)$	$-$	+	+	+
$\left(x-2\right)$	$-$	$-$	$-$	+
quotient	$-$	+	$-$	+

As it is seen that the grater than or equal to 0 in the interval $\left(-3,-\frac{1}{2}\right)$ and $\left(2,\infty \right)$ .

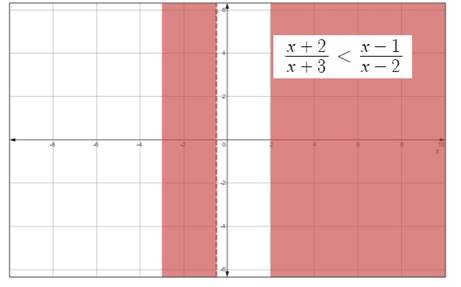
Hence,the solution set is $x\in \left(-3,-\frac{1}{2}\right)\cup \left(2,\infty \right)$ .

The solution set of the inequality graphed on the number line.

  

The graph of the non-linear inequality $\frac{x+2}{x+3}<\frac{x-1}{x-2}$ is: