Chapter 1.5, Problem 32E

(a)

To determine

To find: The amount remaining isotope of sodium after 60 hours.

Answer to Problem 32E

The amount remaining isotope of sodium after 60 hours is 0.125g.

Explanation of Solution

Given:

The half-life of sodium is 15 hours.

The mass of sample sodium = 2g.

Calculation:

Let $m_0$ be the initial mass of sodium.

That is $m_0=2\text{g}$.

After 15 hours the amount of sodium is $m_1=\frac{1}{2}{m}_0$.

Substitute 2g for $m_0$ in $m_1$,

$\begin{array}{l}{m}_1=\frac{1}{2}\left(2\right)\\ m_1=1\text{g}\end{array}$

After 30 hours the amount of sodium $m_2=\frac{1}{2}{m}_1$

Substitute 1g for $m_1$ in $m_2$,

$\begin{array}{l}{m}_2=\frac{1}{2}\left(1\right)\\ m_2=0.5\text{g}\end{array}$

After 45 hours the amount of sodium is $m_3=\frac{1}{2}{m}_2$

Substitute 0.5g for $m_2$ in $m_3$,

$\begin{array}{l}{m}_3=\frac{1}{2}\left(0.5\right)\\ m_3=0.25\text{g}\end{array}$

After 60 hours the amount of sodium is $m_4=\frac{1}{2}{m}_3$

Substitute 0.25g for $m_3$ in $m_4$,

$\begin{array}{l}{m}_4=\frac{1}{2}\left(0.25\right)\\ m_4=0.125\text{g}\end{array}$

Therefore, the amount sodium after 60 hours is 0.125g.

(b)

To determine

To find: The amount of sodium after t hours.

Answer to Problem 32E

After t hours amount of sodium is $m_t=2^{\frac{-t}{15}}\left(2\right)$.

Explanation of Solution

Calculation:

Consider half –life sodium amount in 15 hours = $\frac{1}{2}{m}_0$.

Where $m_0=2\text{g}$.

After $t=15x\text{hours}$.where x be variable.

Therefore after t hours amount of sodium as follows,

$m_t=\frac{1}{2^x}\left(m_0\right)$ (1)

Substitute $\frac{t}{15}$ for x and 2 for $m_0$ in equation (1),

$\begin{array}{l}{m}_t=\frac{1}{2^{\frac{t}{15}}}\left(2\right)\\ m_t=2^{\frac{-t}{15}}\left(2\right)\end{array}$

Therefore, after t hours amount of sodium is $m_t=2^{\frac{-t}{15}}\left(2\right)$.

(c)

To determine

To estimate: The remaining amount of sodium after 4 days.

Answer to Problem 32E

After 4days the amount of sodium is $m_{96}=0.0236\text{g}$.

Explanation of Solution

Given:

Consider after 15 hours the amount of sodium = 1g.

$m_0=2\text{g}$.

Calculation:

After 15 hours the amount of sodium is $m_1=\frac{1}{2}{m}_0$.

By using part (b) solution $m_t=2^{\frac{-t}{15}}\left(2\right)$ for t hours.

Obtain the amount after 4 days that means 96 hours.

Substitute 96 for t in $m_t=2^{\frac{-t}{15}}\left(2\right)$,

$\begin{array}{l}{m}_{96}=2^{\frac{-96}{15}}\left(2\right)\\ m_{96}=2^{-6.4}\left(2\right)\\ m_{96}=0.0118\left(2\right)\\ m_{96}=0.0236\text{g}\end{array}$

Therefore, after 4 days the amount of sodium is $m_{96}=0.0236\text{g}$.

(d)

To determine

To estimate: The amount of bismuth reduced to 0.01g.

Answer to Problem 32E

The amount of sodium amount reduced to 0.01g for 38.2215 days.

Explanation of Solution

Given:

The reduced amount of sodium = 0.01g.

Graph:

Let x axis for hours and y axis for sodium (g).

Use the online graphing calculator and draw the graphs for sodium level and hours as shown below Figure 1.

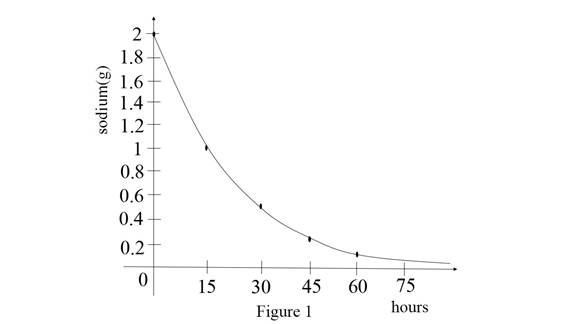
Observe the graph an initial stage sodium amount is 2g.

Then after 15 hours the mass is reducing to 1g.

After 30 hours the mass is reducing to 0.5g.

After 45 hours mass is reducing to 0.25g.

After 60 hours mass is reducing to 0.125g.

Therefore, the mass of sodium is reducing when the hours are increasing.

Calculation:

From the graph, the general form of sodium after t hours $m_t=2^{\frac{-t}{15}}\left(2\right)$.

Substitute 0.01 for $m_t$ in $m_t=2^{\frac{-t}{15}}\left(2\right)$,

$\begin{array}{l}0.01=2^{\frac{-t}{15}}\left(2\right)\\ \frac{0.01}{2}=2^{\frac{-t}{15}}\\ 0.005=2^{\frac{-t}{15}}\end{array}$

Take naturel logarithm on both sides,

$\begin{array}{l}\ln \left(0.005\right)=\ln \left(2^{\frac{-t}{15}}\right)\\ \ln \left(0.005\right)=\frac{-t}{15}\ln \left(2\right)\\ \frac{\ln \left(0.005\right)}{\ln \left(2\right)}=\frac{-t}{15}\\ t=-15\left(\frac{\ln \left(0.005\right)}{\ln \left(2\right)}\right)\end{array}$

Simplify further,

$\begin{array}{l}t=-15\left(\frac{-5.2983}{0.6931}\right)\\ t=-15\left(-7.6443\right)\\ t=114.6645\end{array}$

Therefore, the amount of sodium reduced to 0.01g for 114.6645 hours.