Chapter 1.3, Problem 58E

The Heaviside function defined in Exercise 59 can also be used to define the ramp function y = ctH(t), which represents a gradual increase in voltage or current in a circuit.

(a) Sketch the graph of the ramp function y = tH(t).

(b) Sketch the graph of the voltage V(t) in a circuit if the switch is turned on at time t = 0 and the voltage is gradually increased to 120 volts over a 60-second time interval. Write a formula for V(t) in terms of H(t) for t ≤ 60.

(c) Sketch the graph of the voltage V(t) in a circuit if the switch is turned on at time t = 7 seconds and the voltage is gradually increased to 100 volts over a period of 25 seconds.  Write a formula for V(t) in terms of H(t) for t ≤ 32.

To determine

To sketch: The graph of the ramp function $y=tH\left(t\right)$.

Explanation of Solution

Heaviside function is, $H\left(t\right)=\{\begin{array}{l}0\text{ if }t<0\\ 1\text{if }t\ge 0\end{array}$.

So, the ramp function is, $R\left(t\right)=tH\left(t\right)=\{\begin{array}{l}0\text{ if }t<0\\ t\text{if }t\ge 0\end{array}$.

Use online graph calculator and draw the graph of the ramp function as shown below in Figure 1.

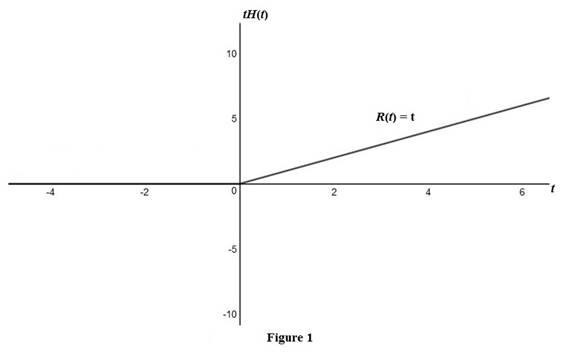
From Figure1, it is noted that the graph is a ray with starting point 0.

To determine

To sketch: The graph of circuit if the switch is turned at t = 0 and the voltage gradually increased to 120 volts in 60 seconds; write the formula for $V\left(t\right)$ in terms of $H\left(t\right)$ for

$t\le 60$.

Answer to Problem 58E

The formula for $V\left(t\right)$ in terms of $H\left(t\right)$ is $V\left(t\right)=2tH\left(t\right),t\le 60$.

Explanation of Solution

Given:

The voltage $V\left(t\right)$ switched in time $t=0$ and 120 volts are gradually increased over a period $t\le 60$.

Calculation:

Let the ramp function be $y=V\left(t\right)=ctH\left(t\right)$.

Find the value of c.

Since $t=60$, then the ramp function becomes, $V\left(60\right)=c\left(60\right)H\left(t\right)$ (1)

It is known that $H\left(t\right)=1$.

Therefore, equation(1) becomes,

$\begin{array}{c}120=c\left(60\right)\left(1\right)\\ c=\frac{120}{60}\\ c=2\end{array}$

Thus, the ramp function is $y=V\left(t\right)=2tH\left(t\right)$.

Therefore, $V\left(t\right)$ can be written in form of $H\left(t\right)$ as, $2tH\left(t\right)=\{\begin{array}{l}0\text{ if }t<0\\ 2t\text{if }t\ge 0\end{array}$.

Use online graph calculator and draw the graph of $V\left(t\right)=\{\begin{array}{l}0\text{ if }t<0\\ 2t\text{if }t\ge 0\end{array}$ as shown below in Figure 2.

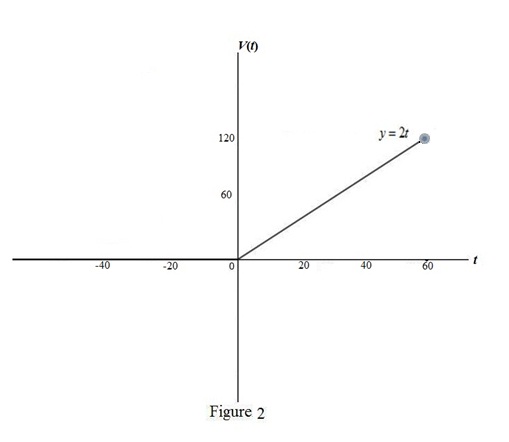
From Figure1, it is noted that the graph is a ray with starting point 0.

To determine

To sketch: The graph of the voltage in a circuit if the switch is turned at t = 7 seconds and gradually increased t0 100 volts over a period of 25 seconds; write the formula for $V\left(t\right)$ in terms of $H\left(t\right)$ for $t\le 32$.

Answer to Problem 58E

The formula for $V\left(t\right)$ in terms of $H\left(t\right)$ after $t=7$ is $V\left(t\right)=4\left(t-7\right)H\left(t-7\right),t\le 32$

Explanation of Solution

The voltage varies from 0 to 100 in 25 seconds.

Therefore,

$\begin{array}{c}c=\frac{100-0}{25}\\ =\frac{100}{25}\\ =4\end{array}$

Therefore, the ramp function becomes, $V\left(t\right)=4tH\left(t\right)$

The switch is turned at t = 7 seconds

That is, the time $t-7=0$

Therefore, the formula for $V\left(t\right)$ in terms of $H\left(t\right)$ after $t=7$ is,. $V\left(t\right)=4\left(t-7\right)H\left(t-7\right)$.

Thus, $V\left(t\right)$ is transferred to the 7 units to the right of the ramp function.

The function, $V\left(t\right)$ can be written as, $V\left(t\right)=\{\begin{array}{l}0\text{ if }t<7\\ 4\left(t-7\right)\text{if 7}\le t\le 32\end{array}$.

Use online graph calculator and draw the graph of $V\left(t\right)=\{\begin{array}{l}0\text{ if }t<7\\ 4\left(t-7\right)\text{if 7}\le t\le 32\end{array}$ as shown below in Figure 3.

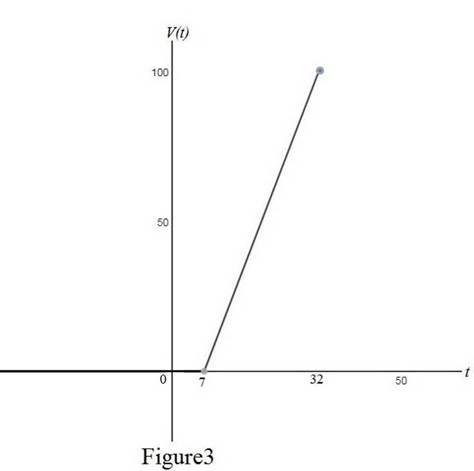
From Figure 3, it is noted that the voltage reaches to 100 in 25 seconds.