
To solve: The function is defined on the interval ,
a. Graph .
In (b)–(e), approximate the area under from 0 to 6 as follows:
Answer to Problem 9AYU
Solution:
a.
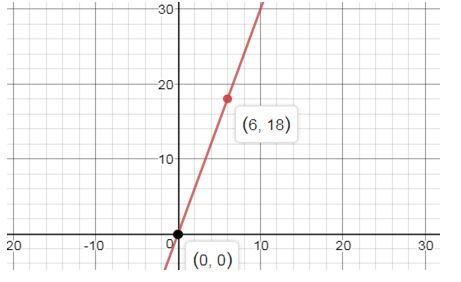
Explanation of Solution
Given:
The function is defined on the interval .
Calculation:
; ; ; ; ; ;
a. Graph .

To solve: The function is defined on the interval ,
b. Partition into three subintervals of equal length and choose as the left endpoint of each subinterval.
Answer to Problem 9AYU
Solution:
b. 36
Explanation of Solution
Given:
The function is defined on the interval .
Calculation:
; ; ; ; ; ;
b. Partition into three subintervals of equal length 2 and choose as the left endpoint of each subinterval.
The area is approximated as
To solve: The function is defined on the interval ,
c. Partition into three subintervals of equal length and choose as the right endpoint of each subinterval.
Answer to Problem 9AYU
Solution:
c. 72
Explanation of Solution
Given:
The function is defined on the interval .
Calculation:
; ; ; ; ; ;
c. Partition into three subintervals of equal length 2 and choose as the right endpoint of each subinterval.
The area is approximated as
To solve: The function is defined on the interval ,
d. Partition into six subintervals of equal length and choose as the left endpoint of each subinterval.
Answer to Problem 9AYU
Solution:
d. 45
Explanation of Solution
Given:
The function is defined on the interval .
Calculation:
; ; ; ; ; ;
d. Partition into six subintervals of equal length 1 and choose as the left endpoint of each subinterval.
The area is approximated as
To solve: The function is defined on the interval ,
e. Partition into six subintervals of equal length and choose as the right endpoint of each subinterval.
Answer to Problem 9AYU
Solution:
e. 63
Explanation of Solution
Given:
The function is defined on the interval .
Calculation:
; ; ; ; ; ;
e. Partition into six subintervals of equal length 1 and choose as the right endpoint of each subinterval.
The area is approximated as
To solve: The function is defined on the interval ,
f. What is the actual area ?
Answer to Problem 9AYU
Solution:
f. 54
Explanation of Solution
Given:
The function is defined on the interval .
Calculation:
; ; ; ; ; ;
f. The actual area under the graph of from 0 to 6 is the area of a right triangle whose base is of length 6 and whose height is 18. The actual area is
Therefore
Chapter 14 Solutions
Precalculus Enhanced with Graphing Utilities
Additional Math Textbook Solutions
Elementary Statistics: Picturing the World (7th Edition)
Thinking Mathematically (6th Edition)
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Algebra and Trigonometry (6th Edition)
University Calculus: Early Transcendentals (4th Edition)
- what is the horizonal asymptote of question d?arrow_forward2 3 Polar axis The graph of the polar function r = = f(0) is given in the polar coordinate system. Which of the following defines f(0) for 0 ≤ 0 ≤ 2πT? A 3+ cos(30) B 3 cos(30) C 3+ sin(30) D 3 sin (30)arrow_forwardSolve by superposition method the following DE: y^(4) - y = xe^(x) sen(2x), conditions: y(0) = y'(0) = y''(0) = y'''(0) =0arrow_forward
- Use the annulus method to find the solution of the DE: y''' + 8y = e^(3x) sen(3x) cos(3x)arrow_forward3:59 m s ☑ D'Aniello Boutique | Fashion VOLTE danielloboutique.it/asia SUBSCRIBE NOW: 10% OFF TO USE ANYTIME YOU WANT d'aniello NEW IN WOMEN NEW IN MEN WINTER SALE: 50% OFF on FW24 SHOP WOMEN SHOP MENarrow_forwardJOB UPDATE EMERSON GRAD ENGINEER (FRESHERS) SOFTWARE ENGG NEW RELIC BROWSERSTACK (FRESHERS) SOFTWARE ENGG FULL STACK DATA ENGINEER GENPACT + PYTHON CARS24 WORK FROM HOME #vinkjobs TELE PERFORMANCE Vinkjobs.com CUSTOMER SUPPORT Search "Vinkjobs.com" on Googlearrow_forward
- do question 2 pleasearrow_forwardquestion 10 pleasearrow_forward00 (a) Starting with the geometric series Σ X^, find the sum of the series n = 0 00 Σηχη - 1, |x| < 1. n = 1 (b) Find the sum of each of the following series. 00 Σnx", n = 1 |x| < 1 (ii) n = 1 sin (c) Find the sum of each of the following series. (i) 00 Σn(n-1)x^, |x| <1 n = 2 (ii) 00 n = 2 n² - n 4n (iii) M8 n = 1 շոarrow_forward
- (a) Use differentiation to find a power series representation for 1 f(x) = (4 + x)²* f(x) = 00 Σ n = 0 What is the radius of convergence, R? R = (b) Use part (a) to find a power series for f(x) = 1 (4 + x)³° f(x) = 00 Σ n = 0 What is the radius of convergence, R? R = (c) Use part (b) to find a power series for f(x) = x² (4 + x)³* 00 f(x) = Σ n = 2 What is the radius of convergence, R? R = Need Help? Read It Watch It SUBMIT ANSWERarrow_forwardanswer for question 4 pleasearrow_forward(3) (20 points) Let F(x, y, z) = (y, z, x²z). Define E = {(x, y, z) | x² + y² ≤ z ≤ 1, x ≤ 0}. (a) (2 points) Calculate the divergence V. F. (b) (4 points) Let D = {(x, y) | x² + y² ≤ 1, x ≤ 0} Without calculation, show that the triple integral √ (V · F) dV = √ 2²(1. = x²(1 − x² - y²) dA. Earrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





