Chapter 13, Problem 29P

To determine

Calculate the coupling coefficient of the circuit that make the $10\Omega$ resistor dissipate 1.28 kW and calculate the stored energy in the coupled coils at $t=1.5\text{s}$.

Answer to Problem 29P

The required coupling coefficient is $\underset{\_}{0.9845}$ and the stored energy in the coupled coils at $t=1.5\text{s}$ is $\underset{\_}{522\text{mJ}}$.

Explanation of Solution

Given data:

Refer to Figure 13.98 in the textbook for the circuit with coupled coils.

In Figure 13.98, consider that the primary and secondary loops contain the currents $I_1$ and $I_2$ respectively.

The value of $L_1\text{and}{L}_2$ are 30 mH and 50 mH respectively.

The value of $\omega$ is 1000 rad/s.

Calculation:

Calculate the inductors in frequency domain.

Write the expression for the inductive reactance.

$j{X}_L=j\omega L$        (1)

Substitute 30 mH for $L$ and $1,000\text{rad/s}$ for $\omega$ in Equation (1).

$\begin{array}{c}j{X}_L=j\left(30\text{mH}\right)\left(1,000\text{rad/s}\right)\\ =j\left(30\times {10}^{-3}\right)\left(1,000\right)\\ =j30\Omega \end{array}$

Substitute 50 mH for $L$ and $1,000\text{rad/s}$ for $\omega$ in Equation (1).

$\begin{array}{c}j{X}_L=j\left(50\text{mH}\right)\left(1,000\text{rad/s}\right)\\ =j\left(50\times {10}^{-3}\right)\left(1,000\right)\\ =j50\Omega \end{array}$

Consider that the value of X.

$X=\omega M$

Substitute $1,000\text{rad/s}$ for $\omega$.

$X=1,000M$        (2)

Consider that the second side reflect on the primary side. Consider the expression for the input impedance.

$Z_{\text{in}}=\left(10+j30\right)+\frac{X^2}{20+j50}$        (3)

Write the expression for the current $I_1$ using Figure 13.98.

$I_1=\frac{V}{Z_{\text{in}}}$        (4)

Substitute 330 V for V and Equation (3) in (4).

$I_1=\frac{330}{\left(10+j30\right)+\frac{X^2}{20+j50}}$        (5)

Consider the expression for the power dissipated in the $10\Omega$ resistor.

$p=0.5{\left|I_1\right|}^2\left(10\Omega \right)$

Substitute 1.288 kW for p.

$\begin{array}{l}1.28\text{kW}=0.5{\left|I_1\right|}^2\left(10\Omega \right)\\ {\left|I_1\right|}^2=\frac{1,280}{0.5\times 10}\\ {\left|I_1\right|}^2=256\\ \left|I_1\right|=16\text{A}\end{array}$

Substitute 16 A for $I_1$ in equation (5).

$\begin{array}{l}16=\frac{330}{\left(10+j30\right)+\frac{X^2}{20+j50}}\\ 16=\frac{330\left(20+j50\right)}{\left(10+j30\right)\left(20+j50\right)+X^2}\\ 16=\frac{330\left(20+j50\right)}{X^2-1300+j1100}\end{array}$

Square on both sides of the equation.

$\begin{array}{l}256=\frac{108,900\left(400+2500\right)}{{\left(X^2-1300\right)}^2+1,210,000}\\ {\left(X^2-1300\right)}^2+1,210,000=1,233,632.8\\ {\left(X^2-1300\right)}^2=23,632.8\\ X^2-1300=\pm 153.73\end{array}$

Simplify the equation as follows.

$X^2=\pm 153.73+1300$

By solving the above equation, there are two positive values of X and they are,

$X=33.857\text{and}38.128$.

Consider that the value of X as 38.128.

Substitute 38.128 for X in Equation (2).

$\begin{array}{l}38.128=1,000M\\ M=\frac{38.128}{1,000}\\ M=38.128\text{mH}\end{array}$

Consider the expression for the coefficient of coupling in the coupled coils.

$k=\frac{M}{\sqrt{L_1{L}_2}}$

Substitute 38.128 mH for M, 30 mH for $L_1$, and 50 mH for $L_2$.

$\begin{array}{c}k=\frac{38.128\text{mH}}{\sqrt{\left(30\text{mH}\right)\left(50\text{mH}\right)}}\\ =\frac{38.128}{\sqrt{30\times 50}}\\ =0.9845\end{array}$

Modify the Figure 13.98 by transforming the time-domain circuit with coupled-coils to frequency domain of the circuit with coupled-coils. The frequency domain equivalent circuit is shown in Figure 1.

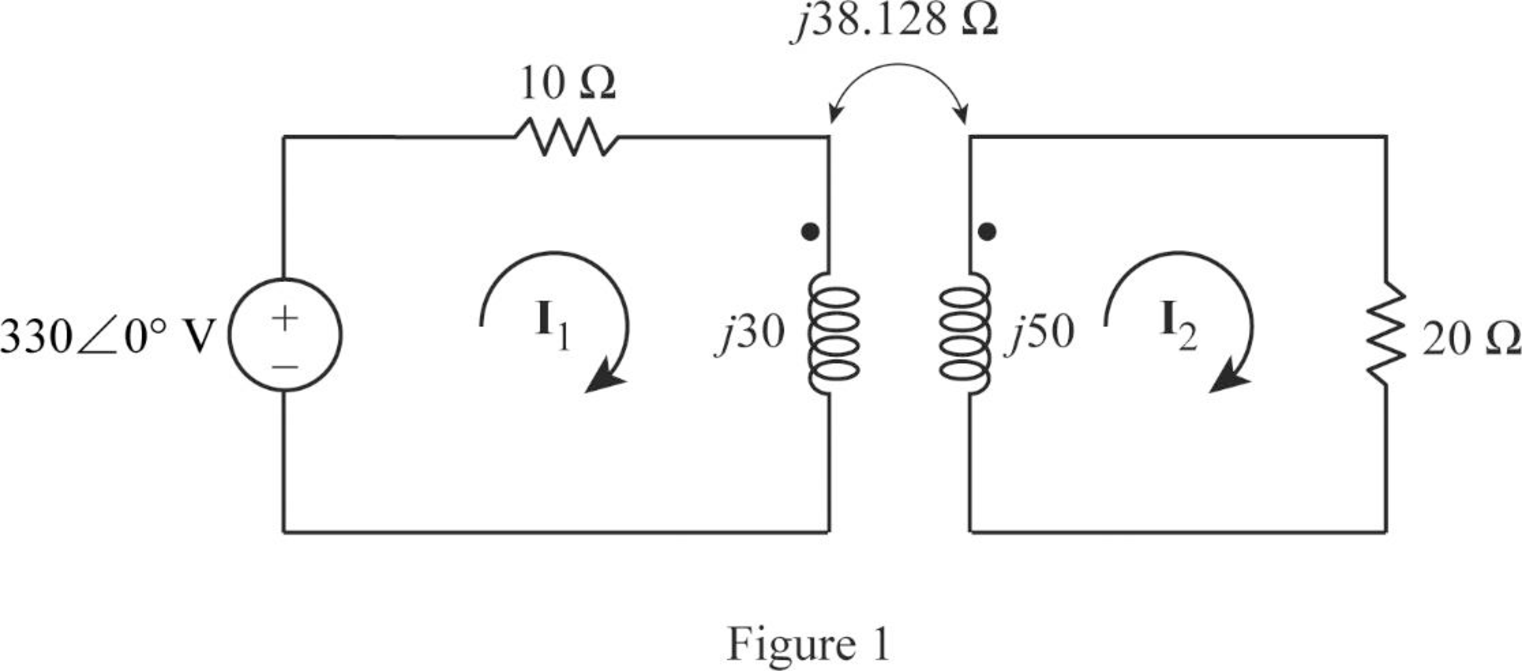
From Figure 1, consider that the loops 1 and 2 contain the currents $I_1$ and $I_2$ respectively.

Apply Kirchhoff's voltage law to the loop 1 in Figure 1.

$\left(10+j30\right)I_1-j38.128I_2=330$        (6)

Apply Kirchhoff's voltage law to the loop 2 in Figure 1.

$-j38.128I_1+\left(20+j50\right)I_2=0$        (7)

Write equations (6) and (7) in matrix form as follows.

$\left[\begin{array}{cc}\left(10+j30\right)& -j38.128\\ -j38.128& \left(20+j50\right)\end{array}\right]\left[\begin{array}{c}{I}_1\\ I_2\end{array}\right]=\left[\begin{array}{c}330\\ 0\end{array}\right]$        (8)

Write the MATLAB code to solve the equation (8).

A = [(10+j*30) j*(-38.128); j*(-38.128) (20+j*50)];

B = [330; 0];

I = inv(A)*B

The output in command window:

I =

   15.535 - 3.829i

   11.219 + 1.568i

From the MATLAB output, the currents $I_1$ and $I_2$ are,

$\begin{array}{c}{I}_1=\left(15.535-j3.829\right)\text{A}\\ =16\angle -13.81°\text{A}\end{array}$

And

$\begin{array}{c}{I}_2=\left(11.219+j1.568\right)\text{A}\\ =11.328\angle 7.97°\text{A}\end{array}$

Write the currents $I_1$ and $I_2$ in time-domain.

$i_1=16\cos \left(1000t-13.81°\right)\text{A}$        (9)

$i_2=11.328\cos \left(1000t+7.97°\right)\text{A}$        (10)

Substitute 1.5 ms for t in Equation (9).

$\begin{array}{c}{i}_1=16\cos \left(1000\left(1.5\text{ms}\right)-13.83°\right)\text{A}\\ =16\cos \left(\left(1.5\text{rad}\right)-13.83°\right)\text{A}\\ =16\cos \left(85.94°-13.83°\right)\text{A}\left\{\begin{array}{l}\because 1.5\text{rad}=1.5\times \frac{180}{\pi }\mathrm{deg}\\ =85.94°\end{array}\right\}\\ =4.915\text{A}\end{array}$

Substitute 1.5 ms for t in Equation (10).

$\begin{array}{c}{i}_2=11.328\cos \left(1000\left(1.5\text{ms}\right)+7.97°\right)\text{A}\\ =11.328\cos \left(\left(1.5\text{rad}\right)+7.97°\right)\text{A}\\ =11.328\cos \left(85.94°°+7.97°\right)\text{A}\left\{\begin{array}{l}\because 1.5\text{rad}=1.5\times \frac{180}{\pi }\mathrm{deg}\\ =85.94°\end{array}\right\}\\ =-0.77245\text{A}\end{array}$

Write the expression for the total energy stored in the coupled coils.

$w=0.5L_1{i}_1^2+0.5L_2{i}_2^2-M{i}_1{i}_2$

Substitute 30 mH for $L_1$, 50 mH for $L_2$, 38.128 mH for M, $4.915\text{A}$ for $i_1$, and $-0.77245\text{A}$ for $i_2$.

$\begin{array}{c}w=\left[\begin{array}{l}0.5\left(30\text{mH}\right){\left(4.915\text{A}\right)}^2+0.5\left(50\text{mH}\right){\left(-0.77245\text{A}\right)}^2-\\ \left(38.128\text{mH}\right)\left(4.915\text{A}\right)\left(-0.77245\text{A}\right)\end{array}\right]\\ =0.36236+0.014917+0.144756\\ =0.522\text{J}\\ =522\text{mJ}\end{array}$

Conclusion:

Thus, the required coupling coefficient is $\underset{\_}{0.9845}$ and the stored energy in the coupled coils at $t=1.5\text{s}$ is $\underset{\_}{522\text{mJ}}$.