Chapter 13, Problem 20P

Determine currents I₁, I₂, and I₃ in the circuit of Fig. 13.89. Find the energy stored in the coupled coils at t = 2 ms. Take ω = 1,000 rad/s.

To determine

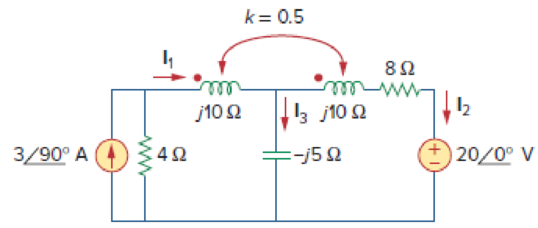
Calculate the currents $I_1,I_2,\text{and}{I}_3$ in the coupled coils circuit in Figure 13.89 and calculate the stored energy in the coupled coils at $t=2\text{ms}$.

Answer to Problem 20P

The currents $I_1,I_2,\text{and}{I}_3$ are $\underset{\_}{2.462\angle 72.18°\text{A}}$, $\underset{\_}{0.878\angle -97.48°\text{A}}$, and $\underset{\_}{3.329\angle 74.89°\text{A}}$ respectively. The energy stored in the coupled coils is $\underset{\_}{43.67\text{mJ}}$.

Explanation of Solution

Given data:

Refer to Figure 13.89 in the textbook for the circuit with coupled coils.

The value of $\omega$ is $1,000\text{rad/s}$. The coupling coefficient is 0.5.

Calculation:

Calculate the mutual inductance in frequency domain.

$\begin{array}{c}jM=j\frac{k}{\sqrt{\left(10\right)\left(10\right)}}\\ =j\frac{0.5}{\sqrt{100}}\left\{\because k=0.5\right\}\\ =j5\Omega \end{array}$

Modify the Figure 13.89 by transforming the current source $\left(3\angle 90°\text{A}=j\text{3}\text{A}\right)$ with parallel resistor $\left(4\Omega \right)$ is converted into the voltage source $\left(j3\text{A}\times 4\Omega =j12\text{V}\right)$ with series resistor $\left(4\Omega \right)$. The modified circuit as shown in Figure 1.

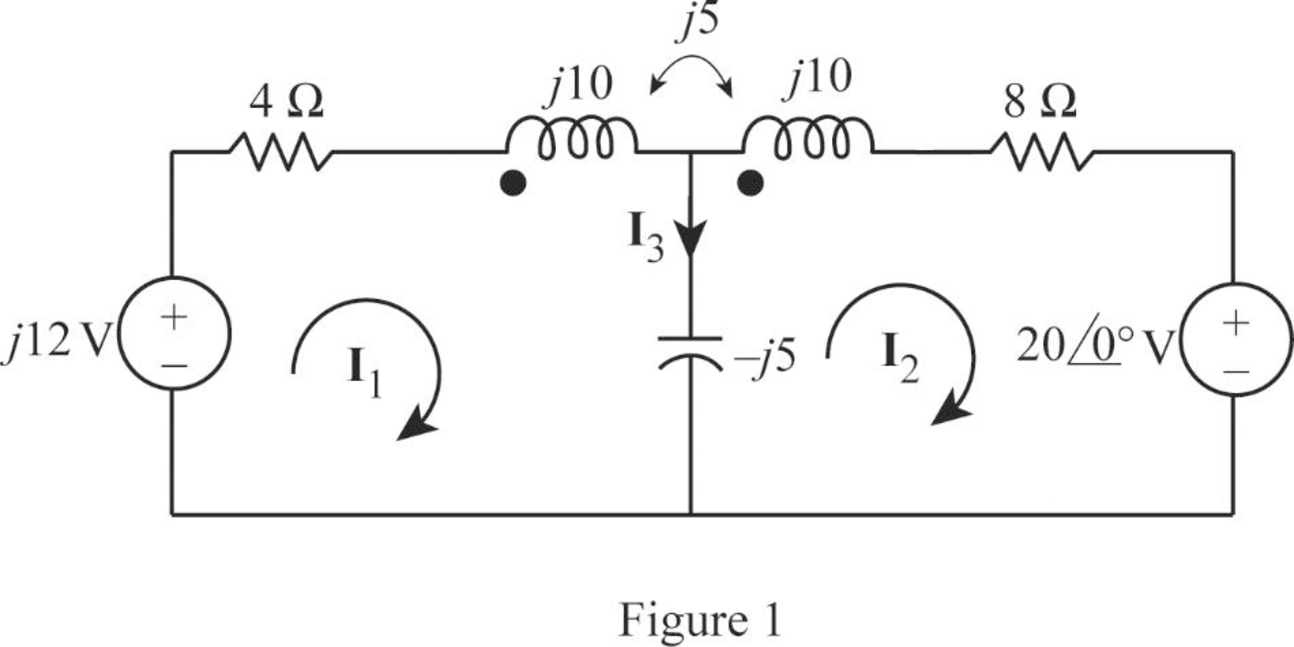
From Figure 1, consider that the loops 1 and 2 contain the currents $I_1$ and $I_2$ respectively.

Apply Kirchhoff's voltage law to the loop 1 in Figure 1.

$\left(4+j10-j5\right)I_1+j5I_2+j5I_2=j12$

$\left(4+j5\right)I_1+j10I_2=j12$        (1)

Apply Kirchhoff's voltage law to the loop 2 in Figure 1.

$20+\left(8+j10-j5\right)I_2+j5I_1+j5I_1=0$

$j10I_1+\left(8+j5\right)I_2=-20$        (2)

Write equations (1) and (2) in matrix form as follows.

$\left[\begin{array}{cc}\left(4+j5\right)& j10\\ j10& \left(8+j5\right)\end{array}\right]\left[\begin{array}{c}{I}_1\\ I_2\end{array}\right]=\left[\begin{array}{c}j12\\ -20\end{array}\right]$        (3)

Write the MATLAB code to solve the equation (3).

A = [(4+j*5) j*10;j*10 (8+j*5)];

B = [j*12; -20];

C = inv(A)*B

The output in command window:

C =

   0.75354 + 2.34381i

  -0.11429 - 0.87049i

From the MATLAB output, the currents $I_1$ and $I_2$ are,

$\begin{array}{c}{I}_1=\left(0.75354+j2.34381\right)\text{A}\\ =2.462\angle 72.18°\text{A}\end{array}$

And

$\begin{array}{c}{I}_2=\left(-0.11429-j0.87049\right)\text{A}\\ =0.878\angle -97.48°\text{A}\end{array}$

From Figure 1, consider the expression for the current $I_3$.

$I_3=I_1-I_2$

Substitute $\left(0.75354+j2.34381\right)\text{A}$ for $I_1$ and $\left(-0.11429-j0.87049\right)\text{A}$ for $I_2$.

$\begin{array}{c}{I}_3=\left(0.75354+j2.34381\right)\text{A}-\left(-0.11429-j0.87049\right)\text{A}\\ =0.86783+j3.2143\\ =3.329\angle 74.89°\text{A}\end{array}$

Write the current $I_1$ and $I_2$ in time-domain.

$i_1=2.462\cos \left(1000t+72.18°\right)\text{A}$        (4)

$i_2=0.878\cos \left(1000t-97.48°\right)\text{A}$        (5)

Substitute 2 ms for t in Equation (4).

$\begin{array}{c}{i}_1=2.462\cos \left(1000\left(2\text{ms}\right)+72.18°\right)\text{A}\\ \text{=}2.462\cos \left(\left(2\text{rad}\right)+72.18°\right)\text{A}\\ \text{=}2.462\cos \left(114.6°+72.18°\right)\text{A}\left\{\begin{array}{l}\because 2\text{rad}=2\times \frac{180}{\pi }\mathrm{deg}\\ =114.6°\end{array}\right\}\\ =-2.445\text{A}\end{array}$

Substitute 2 ms for t in Equation (5).

$\begin{array}{c}{i}_2=0.878\cos \left(1000\left(2\text{ms}\right)-97.48°\right)\text{A}\\ =0.878\cos \left(\left(2\text{rad}\right)-97.48°\right)\text{A}\\ =0.878\cos \left(114.6°-97.48°\right)\text{A}\left\{\begin{array}{l}\because 2\text{rad}=2\times \frac{180}{\pi }\mathrm{deg}\\ =114.6°\end{array}\right\}\\ =-0.8391\text{A}\end{array}$

From Figure 13.81, find the inductor values.

Calculate the inductor $L_1$.

$\omega L_1=10$

Substitute 1000 for $\omega$.

$\begin{array}{l}\left(1000\right)L_1=10\\ L_1=0.01\text{H}\end{array}$

Calculate the inductor $L_2$.

$\omega L_2=10$

Substitute 1000 for $\omega$.

$\begin{array}{l}\left(1000\right)L_2=10\\ L_2=0.01\text{H}\end{array}$

And

Calculate the mutual inductance.

$M=0.5L_1$

Substitute 0.01 H for $L_1$.

$\begin{array}{c}M=0.5\left(0.01\text{H}\right)\\ =0.005\text{H}\end{array}$

Write the expression for the total energy stored in the coupled coils.

$w=0.5L_1{i}_1^2+0.5L_2{i}_2^2+M{i}_1{i}_2$

Substitute 0.01 H for $L_1$, 0.01 H for $L_2$, 0.005 H for M, $-2.445\text{A}$ for $i_1$, $-0.8391\text{A}$ for $i_2$

$\begin{array}{c}w=0.5\left(0.01\right){\left(-2.445\right)}^2+0.5\left(0.01\right){\left(-0.8391\right)}^2+0.005\left(-2.445\right)\left(-0.8391\right)\\ =\left(29.89\times {10}^{-3}\right)+\left(3.5204\times {10}^{-3}\right)+\left(10.258\times {10}^{-3}\right)\\ =43.67\times {10}^{-3}\text{J}\\ =43.67\text{mJ}\end{array}$

Conclusion:

Thus, the currents $I_1,I_2,\text{and}{I}_3$ are $\underset{\_}{2.462\angle 72.18°\text{A}}$, $\underset{\_}{0.878\angle -97.48°\text{A}}$, and $\underset{\_}{3.329\angle 74.89°\text{A}}$ respectively. The energy stored in the coupled coils is $\underset{\_}{43.67\text{mJ}}$.