Finite Mathematics for Business, Economics, Life Sciences and Social Sciences
14th Edition
ISBN: 9780134677972
Author: Barnett
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 1.3, Problem 17E
Freezing temperature. Ethylene glycol and propylene glycol are liquids used in antifreeze and deicing solutions. Ethylene glycol is listed as a hazardous chemical by the Environmental Protection Agency, while propylene glycol is generally regarded as safe. Table 11 lists the freezing temperature for various concentrations (as a percentage of total weight) of each chemical in a solution used to deice airplanes. A linear regression model for the ethylene glycol data in Table 11 is
where
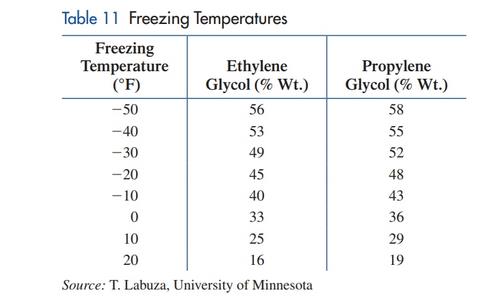
(A) Draw a
(B) Use the model to estimate the freezing temperature to the nearest degree of a solution that
is 30% ethylene glycol.
(C) Use the model to estimate the percentage of ethylene glycol in a solution that freezes at
15°F.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
We have data on Lung Capacity of persons and we wish
to build a multiple linear regression model that predicts
Lung Capacity based on the predictors Age and
Smoking Status. Age is a numeric variable whereas
Smoke is a categorical variable (0 if non-smoker, 1 if
smoker). Here is the partial result from STATISTICA.
b*
Std.Err.
of b*
Std.Err.
N=725
of b
Intercept
Age
Smoke
0.835543
-0.075120
1.085725
0.555396
0.182989
0.014378
0.021631
0.021631
-0.648588
0.186761
Which of the following statements is absolutely false?
A. The expected lung capacity of a smoker is expected
to be 0.648588 lower than that of a non-smoker.
B. The predictor variables Age and Smoker both
contribute significantly to the model.
C. For every one year that a person gets older, the lung
capacity is expected to increase by 0.555396 units,
holding smoker status constant.
D. For every one unit increase in smoker status, lung
capacity is expected to decrease by 0.648588 units,
holding age constant.
A study was done in diesel-powered light-duty pickup truck to see if humidity, air temperature, and barometric
pressure influence emission of nitrous oxide (in ppm). Emission measurements were taken at different times,
with varying experimental conditions.
a. Fit the multiple linear regression model.
b. Estimate the amount of nitrous oxide emitted for the conditions where humidity is 50%,
temperature is 76 deg F, and barometric pressure is 29.30.
Nitrous
Humidity, Temp.,
Pressure.
Nitrous
Humidity, Temp.,
Pressure,
Oxide, y
Oxide, y
0.90
72.4
76.3
29.18
1.07
23.2
76.8
29.38
0.91
41.6
70.3
29.35
0.94
47.4
86.6
29.35
0.96
34.3
77.1
29.2
1.10
31.5
76.9
29.63
0.89
35.1
68.0
29.27
1.10
10.6
86.3
29.56
1.00
10.7
79.0
29.78
1.10
86.0
76.3
11.2
29.48
1.10
12.9
67.4
29.39
0.91
73.3
29.40
1.15
8.3
66.8
29.69
0.87
75.4
77.9
29.28
1.03
20.1
76.9
29.48
0.78
96.6
78.7
29.29
0.77
72.2
77.7
29.09
0.82
107.4
86.8
29.03
1.07
24.0
67.7
29.60
0.95
54.9
70.9
29.37
The production of wine is a multibillion-dollar worldwide industry. In an attempt to develop a model of wine quality as judged by wine experts, data was collected from red wine variants of a certain type of wine. A sample of 12 wines is given. Develop a simple linear regression model to predict wine quality, measured on a scale from 0 (very bad) to 10 (excellent), based on alcohol content (%).
b. For these data, b0=4.4 and b1=0.2. Interpret the meaning of the slope,
b1, in this problem. Choose the correct answer below.
A.
The slope, b1, implies that for each increase of 1 wine quality rating, the alcohol content is expected to increase by the value of b1, in percentages.
B.
The slope, b1, implies that the alcohol content is equal to the value of b1,in percentages.
C.
The slope, b1, implies that for each increase of alcohol percentage of 1.0, the wine quality rating is expected to increase by the value of b1.
D.
The slope, b1, implies that…
Chapter 1 Solutions
Finite Mathematics for Business, Economics, Life Sciences and Social Sciences
Ch. 1.1 - According to equality property 2, multiplying both...Ch. 1.1 - Replace ? with < or > in each of the following:...Ch. 1.1 - The solution to Example 5B shows the graph of the...Ch. 1.1 - Solve and check: 3x2(2x5)=2(x+3)8Ch. 1.1 - Solve and check: x+13x4=12Ch. 1.1 - Matched Problem 3 If a cardboard box has length L,...Ch. 1.1 - Replace each question mark with either < or >....Ch. 1.1 - (A) Write 7,4 as a double inequality and graph....Ch. 1.1 - Solve and graph: 3(x1)5(x+2)5Ch. 1.1 - Solve and graph: 83x57
Ch. 1.1 - Mary paid 8.5 sales tax and a 190 title and...Ch. 1.1 - How many bike computers would a company have to...Ch. 1.1 - What net annual salary in 1973 would have had the...Ch. 1.1 - In Problems 1-6, solve for x. 5x+3=x+23Ch. 1.1 - In Problems 1-6, solve for x. 7x6=5x24Ch. 1.1 - In Problems 1-6, solve for x. 9(4x)=2(x+7)Ch. 1.1 - In Problems 1-6, solve for x. 3(x+6)=52(x+1)Ch. 1.1 - In Problems 1-6, solve for x. x+14=x2+5Ch. 1.1 - In Problems 1-6, solve for x. 2x+135x2=4Ch. 1.1 - In Problems 7-12, write the interval as an...Ch. 1.1 - In Problems 7-12, write the interval as an...Ch. 1.1 - In Problems 7-12, write the interval as an...Ch. 1.1 - In Problems 7-12, write the interval as an...Ch. 1.1 - In Problems 7-12, write the interval as an...Ch. 1.1 - In Problems 7-12, write the interval as an...Ch. 1.1 - In Problems 13-18, write the solution set using...Ch. 1.1 - In Problems 13-18, write the solution set using...Ch. 1.1 - In Problems 13-18, write the solution set using...Ch. 1.1 - In Problems 13-18, write the solution set using...Ch. 1.1 - In Problems 13-18, write the solution set using...Ch. 1.1 - In Problems 13-18, write the solution set using...Ch. 1.1 - In Problems 19-32, find the solution set. x4+12=18Ch. 1.1 - In Problems 19-32, find the solution set. m34=23Ch. 1.1 - In Problems 19-32, find the solution set. y532Ch. 1.1 - In Problems 19-32, find the solution set. x456Ch. 1.1 - In Problems 19-32, find the solution set....Ch. 1.1 - In Problems 19-32, find the solution set....Ch. 1.1 - In Problems 19-32, find the solution set....Ch. 1.1 - In Problems 19-32, find the solution set. 34x=5x+1Ch. 1.1 - In Problems 19-32, find the solution set. 3y4y3Ch. 1.1 - In Problems 19-32, find the solution set. x22x5Ch. 1.1 - In Problems 19-32, find the solution set. x5x6=65Ch. 1.1 - In Problems 19-32, find the solution set. y4y3=12Ch. 1.1 - In Problems 19-32, find the solution set. m5335m2Ch. 1.1 - In Problems 19-32, find the solution set. u223u3+2Ch. 1.1 - In Problems 33-36, solve and graph. 23x714Ch. 1.1 - In Problems 33-36, solve and graph. 45x+621Ch. 1.1 - In Problems 33-36, solve and graph. 495C+3268Ch. 1.1 - In Problems 33-36, solve and graph. 123t+511Ch. 1.1 - In Problems 37-42, solve for the indicated...Ch. 1.1 - In Problems 37-42, solve for the indicated...Ch. 1.1 - In Problems 37-42, solve for the indicated...Ch. 1.1 - In Problems 37-42, solve for the indicated...Ch. 1.1 - In Problems 37-42, solve for the indicated...Ch. 1.1 - In Problems 37-42, solve for the indicated...Ch. 1.1 - In Problems 43 and 44, solve and graph. 347x18Ch. 1.1 - In Problems 43 and 44, solve and graph. 1083u6Ch. 1.1 - If both a and b are positive numbers and b/a. is...Ch. 1.1 - If both a and b are negative numbers and b/a is...Ch. 1.1 - Ticket sales. A rock concert brought in $432,500...Ch. 1.1 - Parking meter coins. An all-day parking meter...Ch. 1.1 - IRA. You have $500,000 in an IRA (Individual...Ch. 1.1 - IRA. Refer to Problem 49. How should you divide...Ch. 1.1 - Car prices. If the price change of cars parallels...Ch. 1.1 - Home values. If the price change in houses...Ch. 1.1 - Retail and wholesale prices. Retail prices in a...Ch. 1.1 - Retail and sale prices. Sale prices in a...Ch. 1.1 - Equipment rental. A golf course charges $52 for a...Ch. 1.1 - Equipment rental. The local supermarket rents...Ch. 1.1 - Sales commissions. One employee of a computer...Ch. 1.1 - Sales commissions. A second employee of the...Ch. 1.1 - Break-even analysis. A publisher for a promising...Ch. 1.1 - Break-even analysis. The publisher of a new book...Ch. 1.1 - Break-even analysis. The publisher in Problem 59...Ch. 1.1 - Break-even analysis. The publisher in Problem 60...Ch. 1.1 - Wildlife management. A naturalist estimated the...Ch. 1.1 - Temperature conversion. If the temperature for a...Ch. 1.1 - Psychology. The IQ (intelligence quotient) is...Ch. 1.1 - Psychology. Refer to Problem 65. If the IQ of a...Ch. 1.2 - (A) As noted earlier, (4,3) is a solution of the...Ch. 1.2 - (A) Graph y=x+b for b=5,3,0,3 and 5 simultaneously...Ch. 1.2 - Graph:4x3y=12Ch. 1.2 - Graph 4x-3y=12 on a graphing calculator and find...Ch. 1.2 - (A) Graph x=5andy=3 simultaneously in the same...Ch. 1.2 - Find the slope of the line through each pair of...Ch. 1.2 - Write the equation of the line with slope 12 and y...Ch. 1.2 - (A) Find an equation for the line that has slope...Ch. 1.2 - Answer parts (A) and (B) in Example 7 for fixed...Ch. 1.2 - At a price of $12.59 per box of grapefruit, the...Ch. 1.2 - \ Problems 1-4 refer to graphs (A)-(D). Identify...Ch. 1.2 - Problems 1-4 refer to graphs (A)-(D). Identify the...Ch. 1.2 - Problems 1-4 refer to graphs (A)-(D). Identify the...Ch. 1.2 - Problems 1-4 refer to graphs (A)-(D). Identify the...Ch. 1.2 - In Problems 5-8, sketch a graph of each equation...Ch. 1.2 - In Problems 5-8, sketch a graph of each equation...Ch. 1.2 - In Problems 5-8, sketch a graph of each equation...Ch. 1.2 - In Problems 5-8, sketch a graph of each equation...Ch. 1.2 - In Problems 9-14, find the slope and y intercept...Ch. 1.2 - In Problems 9-14, find the slope and y intercept...Ch. 1.2 - In Problems 9-14, find the slope and y intercept...Ch. 1.2 - In Problems 9-14, find the slope and y intercept...Ch. 1.2 - In Problems 9-14, find the slope and y intercept...Ch. 1.2 - In Problems 9-14, find the slope and y intercept...Ch. 1.2 - In Problems 15-20, find the slope and x intercept...Ch. 1.2 - In Problems 15-20, find the slope and x intercept...Ch. 1.2 - In Problems 15-20, find the slope and x intercept...Ch. 1.2 - In Problems 15-20, find the slope and x intercept...Ch. 1.2 - In Problems 15-20, find the slope and x intercept...Ch. 1.2 - In Problems 15-20, find the slope and x intercept...Ch. 1.2 - In Problems 21-24, write an equation of the line...Ch. 1.2 - In Problems 21-24, write an equation of the line...Ch. 1.2 - In Problems 21-24, write an equation of the line...Ch. 1.2 - In Problems 21-24, write an equation of the line...Ch. 1.2 - In Problems 25-28, use the graph of each line to...Ch. 1.2 - In Problems 25-28, use the graph of each line to...Ch. 1.2 - In Problems 25-28, use the graph of each line to...Ch. 1.2 - In Problems 25-28, use the graph of each line to...Ch. 1.2 - In Problems 29-34, sketch a graph of each equation...Ch. 1.2 - In Problems 29-34, sketch a graph of each equation...Ch. 1.2 - In Problems 29-34, sketch a graph of each equation...Ch. 1.2 - In Problems 29-34, sketch a graph of each equation...Ch. 1.2 - In Problems 29-34, sketch a graph of each equation...Ch. 1.2 - In Problems 29-34, sketch a graph of each equation...Ch. 1.2 - In Problems 35-40, find the slope of the graph of...Ch. 1.2 - In Problems 35-40, find the slope of the graph of...Ch. 1.2 - In Problems 35-40, find the slope of the graph of...Ch. 1.2 - In Problems 35-40, find the slope of the graph of...Ch. 1.2 - In Problems 35-40, find the slope of the graph of...Ch. 1.2 - In Problems 35-40, find the slope of the graph of...Ch. 1.2 - Given Ax+By=12, graph each of the following three...Ch. 1.2 - Given Ax+By=24, graph each of the following three...Ch. 1.2 - Graph y=25x+200,x0.Ch. 1.2 - Graph y=40x+160,x0.Ch. 1.2 - (A) Graph y=1.2x4.2 in a rectangular coordinate...Ch. 1.2 - (A) Graph y=0.8x+5.2 in a rectangular coordinate...Ch. 1.2 - In Problems 47-50, write the equations of the...Ch. 1.2 - In Problems 47-50, write the equations of the...Ch. 1.2 - In Problems 47-50, write the equations of the...Ch. 1.2 - In Problems 47-50, write the equations of the...Ch. 1.2 - In Problems 51-58, write the slope-intercept form...Ch. 1.2 - In Problems 51-58, write the slope-intercept form...Ch. 1.2 - In Problems 51-58, write the slope-intercept form...Ch. 1.2 - In Problems 51-58, write the slope-intercept form...Ch. 1.2 - In Problems 51-58, write the slope-intercept form...Ch. 1.2 - In Problems 51-58, write the slope-intercept form...Ch. 1.2 - In Problems 51-58, write the slope-intercept form...Ch. 1.2 - In Problems 51-58, write the slope-intercept form...Ch. 1.2 - In Problems 59-66, (A) Find the slope of the line...Ch. 1.2 - In Problems 59-66, (A) Find the slope of the line...Ch. 1.2 - In Problems 59-66, (A) Find the slope of the line...Ch. 1.2 - In Problems 59-66, (A) Find the slope of the line...Ch. 1.2 - In Problems 59-66, (A) Find the slope of the line...Ch. 1.2 - In Problems 59-66, (A) Find the slope of the line...Ch. 1.2 - In Problems 59-66, (A) Find the slope of the line...Ch. 1.2 - In Problems 59-66, (A) Find the slope of the line...Ch. 1.2 - Discuss the relationship among the graphs of the...Ch. 1.2 - Discuss the relationship among the graphs of the...Ch. 1.2 - Cost analysis. A donut shop has a fixed cost of...Ch. 1.2 - Cost analysis. A small company manufactures picnic...Ch. 1.2 - Cost analysis. A plant can manufacture 80 golf...Ch. 1.2 - Cost analysis. A plant can manufacture 50 tennis...Ch. 1.2 - Business-Markup policy. A drugstore sells a drug...Ch. 1.2 - Business-Markup policy. A clothing store sells a...Ch. 1.2 - Business-Depreciation. A farmer buys a new tractor...Ch. 1.2 - Business-Depreciation. A charter fishing company...Ch. 1.2 - Boiling point. The temperature at which water...Ch. 1.2 - Boiling point. The temperature at which water...Ch. 1.2 - Flight conditions. In stable air, the air...Ch. 1.2 - Flight navigation. The airspeed indicator on some...Ch. 1.2 - Demographics. The average number of persons per...Ch. 1.2 - Demographics. The median household income divides...Ch. 1.2 - Cigarette smoking. The percentage of female...Ch. 1.2 - Cigarette smoking. The percentage of male...Ch. 1.2 - Supply and demand. At a price of $9.00 per bushel,...Ch. 1.2 - Supply and demand. At a price of $3.20 per bushel,...Ch. 1.2 - Physics. Hooke’s law states that the relationship...Ch. 1.2 - Physics. The distance d between a fixed spring and...Ch. 1.3 - As illustrated in Example 1A, the slope m of a...Ch. 1.3 - As stated previously, we used linear regression to...Ch. 1.3 - The equation a=28.55w+118.7 expresses BSA for...Ch. 1.3 - A 400-pound load of grain is dropped from an...Ch. 1.3 - Prices for emerald-shaped diamonds from an online...Ch. 1.3 - Using the model of Example 4, estimate the...Ch. 1.3 - Figure 5 shows the scatter plot for white spruce...Ch. 1.3 - Ideal weight. Dr. J. D. Robinson published the...Ch. 1.3 - Ideal weight. Dr. J. D. Robinson also published...Ch. 1.3 - Underwater pressure. At sea level, the weight of...Ch. 1.3 - Underwater pressure. Refer to Problem 3. In fresh...Ch. 1.3 - Rate of descent Parachutes. At low altitudes, the...Ch. 1.3 - Rate of descent Parachutes. The U.S Army is...Ch. 1.3 - Speed of sound. The speed of sound through air is...Ch. 1.3 - Speed of sound. The speed of sound through sea...Ch. 1.3 - Energy production. Table 5 lists U.S. fossil fuel...Ch. 1.3 - Energy consumption. Table 6 lists U.S. fossil fuel...Ch. 1.3 - Cigarette smoking. The data in Table 7 shows that...Ch. 1.3 - Cigarette smoking. The data in Table 7 shows that...Ch. 1.3 - Licensed drivers. Table 8 contains the state...Ch. 1.3 - Licensed drivers. Table 9 contains the state...Ch. 1.3 - Net sales. A linear regression model for the net...Ch. 1.3 - Operating income. A linear regression model for...Ch. 1.3 - Freezing temperature. Ethylene glycol and...Ch. 1.3 - Freezing temperature. A linear regression model...Ch. 1.3 - Forestry. The figure contains a scatter plot of...Ch. 1.3 - Forestry. The figure contains a scatter plot of...Ch. 1.3 - Undergraduate enrollment. Table 12 lists fall...Ch. 1.3 - Graduate enrollment. Table 13 lists fall graduate...Ch. 1.3 - Climate. Find a linear regression model for the...Ch. 1.3 - Climate. Find a linear regression model for the...Ch. 1.3 - Olympic Games. Find a linear regression model for...Ch. 1.3 - Olympic Games. Find a linear regression model for...Ch. 1.3 - Supply and demand. Table 16 contains price supply...Ch. 1.3 - Supply and demand. Table 17 contains price supply...Ch. 1 - Solve2x+3=7x11.Ch. 1 - Solvex12x33=12.Ch. 1 - Solve2x+5y=9fory.Ch. 1 - Solve3x4y=7forx.Ch. 1 - Solve Problems 5-7 and graph on a real number...Ch. 1 - Solve Problems 5-7 and graph on a real number...Ch. 1 - Solve Problems 5-7 and graph on a real number...Ch. 1 - Sketch a graph of 3x+2y=9.Ch. 1 - Write an equation of a line with x intercept 6 and...Ch. 1 - Sketch a graph of 2x3y=18. What are the intercepts...Ch. 1 - Write an equation in the form y=mx+b for a line...Ch. 1 - Write the equations of the vertical line and the...Ch. 1 - Write the equation of a line through each...Ch. 1 - Write the equation of the line through the two...Ch. 1 - Solve Problems 15-19. 3x+25=5xCh. 1 - Solve Problems 15-19. u5=u6+65Ch. 1 - Solve Problems 15-19. 5x34+x2=x24+1Ch. 1 - Solve Problems 15-19. 0.05x+0.2530x=3.3Ch. 1 - Solve Problems 15-19. 0.2x3+0.05x=0.4Ch. 1 - Solve Problems 20-24 and graph on a real number...Ch. 1 - Solve Problems 20-24 and graph on a real number...Ch. 1 - Solve Problems 20-24 and graph on a real number...Ch. 1 - Solve Problems 20-24 and graph on a real number...Ch. 1 - Solve Problems 20-24 and graph on a real number...Ch. 1 - Given Ax+By=30, graph each of the following cases...Ch. 1 - Describe the graphs of x=3 and y=2 Graph both...Ch. 1 - Describe the lines defined by the following...Ch. 1 - Solve Problems 28 and 29 for the indicated...Ch. 1 - Solve Problems 28 and 29 for the indicated...Ch. 1 - For what values of a and b is the inequality a+bba...Ch. 1 - If a and b are negative numbers and ab, then is...Ch. 1 - Graph y=mx+bandy=1mx+b simultaneously in the same...Ch. 1 - Investing. An investor has $300,000 to invest. If...Ch. 1 - Break-even analysis. A producer of educational...Ch. 1 - Sports medicine. A simple rule of thumb for...Ch. 1 - Linear depreciation. A bulldozer was purchased by...Ch. 1 - Business Pricing. A sporting goods store sells...Ch. 1 - Income. A salesperson receives a base salary of...Ch. 1 - Price demand. The weekly demand for mouthwash in a...Ch. 1 - Freezing temperature. Methanol, also known as wood...Ch. 1 - High school dropout rates. Table 2 gives U.S. high...Ch. 1 - Consumer Price Index. The U.S. Consumer Price...Ch. 1 - Forestry. The figure contains a scatter plot of 20...
Additional Math Textbook Solutions
Find more solutions based on key concepts
The equivalent expression of x(y+z) by using the commutative property.
Calculus for Business, Economics, Life Sciences, and Social Sciences (13th Edition)
The equation dydx=4y2−3y+1 is separable or not.
Fundamentals of Differential Equations and Boundary Value Problems
Percentiles. The pth percentile of a sorted data set is a number xp such that p of the data fall at or below xp...
Excursions in Modern Mathematics (9th Edition)
In the following exercises, identify the mats 0f the integrand 10 remove absolute values, then evaluate using t...
Calculus Volume 2
CHECK POINT 1 Find a counterexample to show that the statement The product of two two-digit numbers is a three-...
Thinking Mathematically (6th Edition)
69. Get Started Early! Mitch and Bill are both age 75. When Mitch was 25 years old, he began depositing $1000 p...
Using and Understanding Mathematics: A Quantitative Reasoning Approach (6th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- Life Expectancy The following table shows the average life expectancy, in years, of a child born in the given year42 Life expectancy 2005 77.6 2007 78.1 2009 78.5 2011 78.7 2013 78.8 a. Find the equation of the regression line, and explain the meaning of its slope. b. Plot the data points and the regression line. c. Explain in practical terms the meaning of the slope of the regression line. d. Based on the trend of the regression line, what do you predict as the life expectancy of a child born in 2019? e. Based on the trend of the regression line, what do you predict as the life expectancy of a child born in 1580?2300arrow_forwardDemand for Candy Bars In this problem you will determine a linear demand equation that describes the demand for candy bars in your class. Survey your classmates to determine what price they would be willing to pay for a candy bar. Your survey form might look like the sample to the left. a Make a table of the number of respondents who answered yes at each price level. b Make a scatter plot of your data. c Find and graph the regression line y=mp+b, which gives the number of respondents y who would buy a candy bar if the price were p cents. This is the demand equation. Why is the slope m negative? d What is the p-intercept of the demand equation? What does this intercept tell you about pricing candy bars? Would you buy a candy bar from the vending machine in the hallway if the price is as indicated. Price Yes or No 50 75 1.00 1.25 1.50 1.75 2.00arrow_forwardA study was conducted to see if a person's income will affect their well-being. We want to create a model with Y = well-being index, and X = income in $1,000. The dependent variable in this study is: Income in $1,000 Well-being index Neitherarrow_forward
- Turbid water is muddy or cloudy water. Sunlight is necessary for most life forms; thus turbid water is considered a threat to wetland ecosystems. Passive filtration systems are commonly used to reduce turbidity in wetlands. Suspended solids are measured in mg/l. Is there a relation between input and output turbidity for a passive filtration system and, if so, is it statistically significant? At a wetlands environment in Illinois, the inlet and outlet turbidity of a passive filtration system have been measured. A random sample of measurements are shown below. (Reference: EPA Wetland Case Studies.) Reading Inlet (mg/l) Outlet (mg/l) 3 4 5 6 7 8 9 10 11 12 31.6 86.9 87.0 12.0 45.4 43.3 32.3 77.7 71.7 85.9 64.9 80.1 3.3 14.2 16.8 5.7 4.6 6.2 3.4 7.7 8.0 7.4 8.7 14.3 Use a 1% level of significance to test the claim that there is a monotone relationship (either way) between the ranks of the inlet readings and outlet readings. (a) Rank-order the inlet readings using 1 as the largest data…arrow_forwardTurbid water is muddy or cloudy water. Sunlight is necessary for most life forms; thus turbid water is considered a threat to wetland ecosystems. Passive filtration systems are commonly used to reduce turbidity in wetlands. Suspended solids are measured in mg/l. Is there a relation between input and output turbidity for a passive filtration system and, if so, is it statistically significant? At a wetlands environment in Illinois, the inlet and outlet turbidity of a passive filtration system have been measured. A random sample of measurements are shown below. (Reference: EPA Wetland Case Studies.) Reading 1 2 3 4 5 6 7 8 9 10 11 12 Inlet (mg/l) 59.1 25.7 70.5 71.0 37.6 43.5 13.1 24.2 16.7 49.1 67.6 31.7 Outlet (mg/l) 18.2 14.3 15.3 17.5 13.1 8.0 4.1 4.4 4.3 5.8 16.3 7.1 Use a 1% level of significance to test the claim that there is a monotone relationship (either way) between the ranks of the inlet readings and outlet readings. (a) Rank-order the inlet readings using 1 as…arrow_forwardTurbid water is muddy or cloudy water. Sunlight is necessary for most life forms; thus turbid water is considered a threat to wetland ecosystems. Passive filtration systems are commonly used to reduce turbidity in wetlands. Suspended solids are measured in mg/l. Is there a relation between input and output turbidity for a passive filtration system and, if so, is it statistically significant? At a wetlands environment in Illinois, the inlet and outlet turbidity of a passive filtration system have been measured. A random sample of measurements are shown below. (Reference: EPA Wetland Case Studies.) Reading 1 2 3 4 5 6 7 8 9 10 11 12 Inlet (mg/l) 62.1 13.8 63.5 30.6 91.1 11.5 67.2 75.6 79.3 29.5 72.9 21.3 Outlet (mg/l) 11.3 2.7 7.5 12.3 12.9 3.8 13.3 13.2 15.1 6.0 7.0 4.7 Use a 1% level of significance to test the claim that there is a monotone relationship (either way) between the ranks of the inlet readings and outlet readings. (a) Rank-order the inlet readings using 1 as…arrow_forward
- Turbid water is muddy or cloudy water. Sunlight is necessary for most life forms; thus turbid water is considered a threat to wetland ecosystems. Passive filtration systems are commonly used to reduce turbidity in wetlands. Suspended solids are measured in mg/l. Is there a relation between input and output turbidity for a passive filtration system and, if so, is it statistically significant? At a wetlands environment in Illinois, the inlet and outlet turbidity of a passive filtration system have been measured. A random sample of measurements are shown below. (Reference: EPA Wetland Case Studies.) Use a 1% level of significance to test the claim that there is a monotone relationship (either way) between the ranks of the inlet readings and outlet readings. (a) Rank-order the inlet readings using 1 as the largest data value. Also rank-order the outlet readings using 1 as the largest data value. Then construct a table of ranks to be used for a Spearman rank correlation test. (c) Compute…arrow_forwardAssume we have data demonstrating a strong linear link between the amount of fertilizer applied to certain plants and their yield. Which is the independent variable in this research question?arrow_forwardThe table contains price-supply data and price-demand data for com. Find a linear regression model for the price-supply data where x is supply (in billions of bushels) and y is price (in dollars) Do the same for the price-demand data. Find the equilibrium price for com Price ($/bu) 2.15 2.25 2.35 2.48 2.44 2.52 Supply (billion bu) 6.46 7.41 7:51 7.86 8.09 8.35 Price ($/bu) 2.06 2.12 2.22 2.39 2.32 2.42 Demand (billion bu) 9.92 9.28 8:34 8.04 7.86 6.93 4 Find a linear regression model for the price-supply data where x is supply (in billions of bushels) and y is price (in dollars) Y-0 (Type an equation using x as the variable Round to two decimal places as needed)arrow_forward
- A study examined the eating habits of 20 children at a nursery school. The variables measured for each child included: calories (the number of calories eaten at lunch), time (the time in minutes spent eating lunch), and sex (male=1, female=0). A multiple linear regression model using Y = calories, X1 = time, and X2 = sex led to the following model: y=547.65-2.85x1+10.67x2 For two children who spend the same amount of time eating, one male and one female, which child is predicted to consume more calories and by how much?arrow_forwardThe production of wine is a multibillion-dollar worldwide industry. In an attempt to develop a model of wine quality as judged by wine experts, data was collected from red wine variants of a certain type of wine. A sample of 12 wines is given. Develop a simple linear regression model to predict wine quality, measured on a scale from 0 (very bad) to 10 (excellent), based on alcohol content (%). Complete parts (a) through (d) below. Click the icon to view the data of wine quality and alcohol content. a. Construct a scatter plot. Choose the correct graph below. A. O D. A 0 15 0 15 0 40 Alcohol Content (%) Alcohol Content (%) Alcohol Content (%) b. For these data, bo = 4.9 and b₁ = 0.2. Interpret the meaning of the slope, b₁, in this problem. Choose the correct answer below. X A. The slope, b₁, implies that for each increase of 1 wine quality rating, the alcohol content is expected to increase by the value of b₁, in percentages. B. The slope, b₁, implies that for each increase of alcohol…arrow_forwardThe production of wine is a multibillion-dollar worldwide industry. In an attempt to develop a model of wine quality as judged by wine experts, data was collected from red wine variants of a certain type of wine. A sample of 12 wines is given. Develop a simple linear regression model to predict wine quality, measured on a scale from 0 (very bad) to 10 (excellent), based on alcohol content (%). Complete parts (a) through (d) below. Click the icon to view the data of wine quality and alcohol content. a. Construct a scatter plot. Choose the correct graph below. 10- SPA 15 0 15 40 Alcohol Content (%) Alcohol Content (%) Alcohol Content (%) b. For these data, b = 4.9 and b₁ = 0.2. Interpret the meaning of the slope, b₁, in this problem. Choose the correct answer below. A. The slope, b₁, implies that for each increase of 1 wine quality rating, the alcohol content is expected to increase by the value of b₁, in percentages. B. The slope, b₁, implies that for each increase of alcohol percentage…arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...
Algebra
ISBN:9781337111348
Author:Bruce Crauder, Benny Evans, Alan Noell
Publisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Correlation Vs Regression: Difference Between them with definition & Comparison Chart; Author: Key Differences;https://www.youtube.com/watch?v=Ou2QGSJVd0U;License: Standard YouTube License, CC-BY
Correlation and Regression: Concepts with Illustrative examples; Author: LEARN & APPLY : Lean and Six Sigma;https://www.youtube.com/watch?v=xTpHD5WLuoA;License: Standard YouTube License, CC-BY