Chapter 12.7, Problem 4CYU

To determine

To find: the surface area of the glass on the Luxor.

Answer to Problem 4CYU

The surface area of the square pyramid is $1032656.84{\text{ft}}^2$ .

Explanation of Solution

Given:

  

Formula used:

The lateral area L of a square pyramid is $L=\frac{1}{2}Pl$

The surface area S of a square pyramid is S = L + B

Calculation:

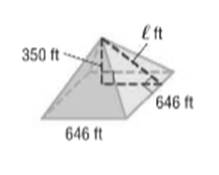
The given figure is a square pyramid.

The objective is to find the lateral and surface area of the square pyramid.

Find the slant of the square pyramid.

Use Pythagorean theorem to find the slant height.

  $\begin{array}{c}{l}^2={350}^2+{\left(\frac{646}{2}\right)}^2\left[\text{Since the base of the triangle is half the side of the square}\right]\\ ={350}^2+{323}^2\\ =122500+104329\\ =226829\\ l\approx 476.27\end{array}$

The side of the square is 646 ft.

The perimeter P of the square is P = 4(side).

Substitute side = 646 in the perimeter formula to find the perimeter of the square.

Thus,

  $\begin{array}{c}P=4\cdot 646\\ =2584\end{array}$

The slant height of the square pyramid is 2584 ft.

Substitute P = 2584 and $l\approx 476.27$ in $L=\frac{1}{2}Pl$

Thus,

  $\begin{array}{c}L=\frac{1}{2}Pl\\ =\frac{1}{\cancel{2}}\cdot \cancel{2}\cancel{5}\cancel{8}{\cancel{4}}^{1292}\left(476.27\right)\\ =1292\left(476.27\right)\\ =615340.84\end{array}$

Therefore, the lateral area of the square pyramid is $615340.84{\text{ft}}^2$

Find the surface area of the square pyramid.

The area B of the square pyramid is $B={\left(side\right)}^2$

  $\begin{array}{c}B={\left(side\right)}^2\\ ={646}^2\\ =417316\end{array}$

Substitute B = 417316 and L = 615340.84 in S = L + B

  $\begin{array}{c}S=L+B\\ \approx 615340.84+417316\\ =1032656.84\end{array}$

Therefore, the surface area of the square pyramid is approximately $1032656.84{\text{ft}}^2$ .

Conclusion:

Therefore, the surface area of the square pyramid is $1032656.84{\text{ft}}^2$ .