Fundamentals of Physics Extended
10th Edition
ISBN: 9781118230725
Author: David Halliday, Robert Resnick, Jearl Walker
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 11, Problem 81P
SSM A uniform wheel of mass 10.0 kg and radius 0.400 m is mounted rigidly on a massless axle through its center (Fig. 11-62). The radius of the axle is 0.200 m, and the rotational inertia of the wheel–axle combination about its central axis is 0.600 kg·m2. The wheel is initially at rest at the top of a surface that is inclined at angle θ = 30.0° with the horizontal; the axle rests on the surface while the wheel extends into a groove in the surface without touching the surface. Once released, the axle rolls down along the surface smoothly and without slipping. When the wheel–axle combination has moved down the surface by 2.00 m, what are (a) its rotational kinetic energy and (b) its translational kinetic energy?
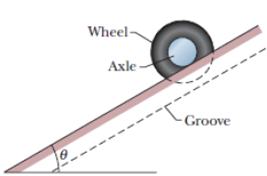
Figure 11-62 Problem 81.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
43 SSM ww The uniform solid Rotation
block in Fig. 10-38 has mass 0.172 kg axis
and edge lengths a = 3.5 cm, b= 8.4
cm, and c = 1.4 cm. Calculate its rota-
tional inertia about an axis through
one corner and perpendicular to the
large faces.
31 In Fig. 11-42, a 0.400 kg ball is
shot directly upward at initial speed 40.0
m/s. What is its angular momentum
about P, 2.00 m horizontally from the
launch point, when the ball is (a) at Figure 11-42 Problem 31.
maximum height and (b) halfway back
to the ground? What is the torque on the ball about P due to the
gravitational force when the ball is (c) at maximum height and
(d) halfway back to the ground?
Ball
In Fig. 10-34, two particles, each with mass m = 0.85 kg, are fastened to each other, and to a
rotation axis at O, by two thin rods, each with length d= 5.6 cm and mass M = 1.2 kg. The
combination rotates around the rotation axis with the angular speed = 0.30 rad/s. Measured
about O, what are the combination's (a) rotational inertia and (b) kinetic energy?
(1)
M
Rotation axis
2M
m
4
10) The rigid body consists of three particles connected by massless rods. It is to be
rotated about an axis perpendicular to its plane through point P. If M = 0.40 kg, a =
30 cm, and b = 50 cm, how much work is required to take the body from rest to an
angular speed of 5.0 rad/s?
M
4
m
2M
Chapter 11 Solutions
Fundamentals of Physics Extended
Ch. 11 - Figure 11-23 shows three particles of the same...Ch. 11 - Figure 11-24 shows two particles A and B at xyz...Ch. 11 - What happens to the initially stationary yo-yo in...Ch. 11 - The position vector r of a particle relative to a...Ch. 11 - In Fig. 11-26, three forces of the same magnitude...Ch. 11 - The angular momenta t of a particle in four...Ch. 11 - A rhinoceros beetle rides the rim of a horizontal...Ch. 11 - Figure 11-27 shows an overhead view of a...Ch. 11 - Figure 11-38 gives the angular momentum magnitude...Ch. 11 - Figure 11-29 shows a particle moving at constant...
Ch. 11 - A cannonball and a marble roll smoothly from rest...Ch. 11 - Prob. 12QCh. 11 - A car travels at 80 km/h on a level road in the...Ch. 11 - An automobile traveling at 80.0 km/h has tires of...Ch. 11 - Prob. 3PCh. 11 - A uniform solid sphere rolls down an incline. a...Ch. 11 - ILW A 1000 kg car has four 10 kg wheels. When the...Ch. 11 - Figure 11-30 gives the speed v versus time t for a...Ch. 11 - ILW In Fig. 11-31, a solid cylinder of radius 10cm...Ch. 11 - Figure 11-32 shows the potential energy Ux of a...Ch. 11 - GO In Fig. 11-33, a solid ball rolls smoothly from...Ch. 11 - A hollow sphere of radius 0.15 m, with rotational...Ch. 11 - In Fig. 11-34, a constant horizontal force Fapp of...Ch. 11 - GO In Fig. 11-35, a solid brass ball of mass 0.280...Ch. 11 - GO Nonuniform ball. In Fig. 11-36, a ball of mass...Ch. 11 - In Fig. 11-37, a small, solid, uniform ball is to...Ch. 11 - GO A bowler throws a bowling ball of radius R = 11...Ch. 11 - GO Nonuniform cylindrical object. In Fig. 11-39, a...Ch. 11 - SSM A yo-yo has a rotational inertia of 950 gcm2...Ch. 11 - Prob. 18PCh. 11 - In unit-vector notation, what is the net torque...Ch. 11 - A plum is located at coordinates 2.0 m, 0, 4.0 m....Ch. 11 - In unit-vector notation, what is the torque about...Ch. 11 - A particle moves through an xyz coordinate system...Ch. 11 - Force F=(2.0N)i(3.0N)k acts on a pebble with...Ch. 11 - In unit-vector notation, what is the torque about...Ch. 11 - SSM Force F=(8.0N)i+(6.0N)j acts on a particle...Ch. 11 - At the instant of Fig. 11-40, a 2.0 kg particle P...Ch. 11 - SSM At one instant, force F=4.0N acts on a 0.25 kg...Ch. 11 - A 2.0 kg particle-like object moves in a plant...Ch. 11 - ILW In the instant of Fig, 11-41, two particles...Ch. 11 - At the instant the displacement of a 2.00 kg...Ch. 11 - In Fig. 11-42, a 0.400 kg ball is shot directly...Ch. 11 - A particle is acted on by two torques about the...Ch. 11 - SSM WWW ILW At time t = 0, a 3.0 kg particle with...Ch. 11 - A particle is to move in an xy plane, clockwise...Ch. 11 - At time t, the vector r=4.0t2i(2.0t+6.0t2)j gives...Ch. 11 - Prob. 36PCh. 11 - GO In Fig. 11-44, three particles of mass m = 23 g...Ch. 11 - A sanding disk with rotational inertia 1.2 103...Ch. 11 - SSM The angular momentum of a flywheel having a...Ch. 11 - A disk with a rotational inertia of 7.00 kgm2...Ch. 11 - GO Figure 11-45 shows a rigid structure consisting...Ch. 11 - Figure 11-46 gives the torque that acts on an...Ch. 11 - Prob. 43PCh. 11 - A Texas cockroach of mass 0.17 kg runs...Ch. 11 - SSM WWW A man stands on a platform that is...Ch. 11 - The rotational inertia of a collapsing spinning...Ch. 11 - SSM A track is mounted on a large wheel that is...Ch. 11 - A Texas cockroach walks from the center of a...Ch. 11 - Two disks are mounted like a merry-go-round on...Ch. 11 - The rotor of an electric motor has rotational...Ch. 11 - SSM ILW A wheel is rotating freely at angular...Ch. 11 - GO A cockroach of mass m lies on the rim of a...Ch. 11 - GO In Fig. 11-50 an overhead view, a uniform thin...Ch. 11 - GO Figure 11-51 shows an overhead view of a ring...Ch. 11 - A horizontal vinyl record of mass 0.10 kg and...Ch. 11 - In a long jump, an athlete leaves the ground with...Ch. 11 - A uniform disk of mass 10m and radius 3.0r can...Ch. 11 - A horizontal platform in the shape of a circular...Ch. 11 - Figure 11-52 is an overhead view of a thin uniform...Ch. 11 - In Fig. 11-53, a 1.0 g bullet is tired into a 0.50...Ch. 11 - The uniform rod length 0.60 m, mass 1.0 kg in Fig....Ch. 11 - GO During a jump to his partner, an aerialist is...Ch. 11 - GO In Fig. 11-56, a 30 kg child stands on the edge...Ch. 11 - A ballerina begins a tour jet Fig. 11-19a with...Ch. 11 - SSM WWW Two 2.00 kg balls are attached to the ends...Ch. 11 - Prob. 66PCh. 11 - Prob. 67PCh. 11 - Prob. 68PCh. 11 - A certain gyroscope consists of a uniform disk...Ch. 11 - A uniform solid ball rolls smoothly along a floor,...Ch. 11 - SSM In Fig. 11-60, a constant horizontal force...Ch. 11 - A thin-walled pipe rolls along the floor. What is...Ch. 11 - Prob. 73PCh. 11 - Prob. 74PCh. 11 - Prob. 75PCh. 11 - A uniform block of granite in the shape of a book...Ch. 11 - SSM Two particles, each of mass 2.90 104 kg and...Ch. 11 - A wheel of radius 0.250 m, moving initially al...Ch. 11 - Wheels A and B in Fig. 11-61 are connected by a...Ch. 11 - Prob. 80PCh. 11 - SSM A uniform wheel of mass 10.0 kg and radius...Ch. 11 - A uniform rod rotates in a horizontal plane about...Ch. 11 - A solid sphere of weight 36.0 N rolls up an...Ch. 11 - Suppose that the yo-yo in Problem 17, instead of...Ch. 11 - A girl of mass M stands on the rim of a...Ch. 11 - Prob. 86P
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- A mountain biker tries to start pedalling in the mud. The total mass (80 kg, bike + biker) is distributed equally between the front and rear wheels. There is a loss of 10% of moment of force between pedal and the rear wheel. The diameter of wheels is 70 cm, and the static friction coefficient between tyre and the mud is 0.3. The pedal angle is 25° from the vertical and has a length of 20 cm. The force exerted by the cyclist is vertical. What is the maximal force that the biker can produce without skidding?Hint 1: ImageHint 2: the value of Mp, the moment around the pedals, is about 50 Nm.arrow_forwardasaparrow_forwardFigure 10-33 gives angular speed versus time for a thin rod that rotates around one end. The scale on the w axis is set by ws = 6.0 rad/s. (a) What is the magnitude of the rod's angular acceleration? (b) At t = 4.0 s, the rod has rotational kinetic energy of 1.60 J. What is its kinetic energy at t = 0? | t (s) 4 Figure 10-33 Problem 34.arrow_forward
- A solid, uniform disk of radius 0.250 m and mass 61.2 kg rolls down a ramp of length 5.10 m that makes an angle of 18.0° with the horizontal. The disk starts from rest from the top of the ramp. (a) Find the speed of the disk's center of mass when it reaches the bottom of the ramp. |m/s (b) Find the angular speed of the disk at the bottom of the ramp. rad/sarrow_forwardA cord is wrapped around the rim of a solid uniform wheel 0.250 m in radius and of mass 9.20 kg. A steady horizontal pull of 40.0 N to the right is exerted on the cord, pulling it off tangentially from the wheel. The wheel is mounted on frictionless bearings on a horizontal axle through its center. (a) Compute the angular acceleration of the wheel and the acceleration of the part of the cord that has already been pulled off the wheel. (b) Find the magnitude and direction of the force that the axle exerts on the wheel. (c) Which of the answers in parts (a) and (b) would change if the pull were upward instead of horizontal?arrow_forward*12-168. A particle travels along the portion of the "four- leaf rose" defined by the equation r= (5 cos 20) m. If the angular velocity of the radial coordinate line is 0=(31²) rad/s, where t is in seconds, determine the radial and transverse components of the particle's velocity and acceleration at the instant = 30°. When t=0,0=0. ① r = (5 cos 20) Prob. 12-168arrow_forward
- You are trying to raise a bicycle wheel of mass m and radius R up over a curb of height h. To do this, you apply a horizontal force F S . What is the smallest magnitude of the force F S that will succeed in raising the wheel onto the curb when the force is applied (a) at the center of the wheel and (b) at the top of the wheel? (c) In which case is less force required?arrow_forwardThe left-hand end of a uniform rod of length L and mass m is attached to a vertical wall by a frictionless hinge. The rod is held at an angle u above the horizontal by a horizontal wire that runs between the wall and the right-hand end of the rod. (a) If the tension in the wire is T, what is the magnitude of the angle u that the rod makes with the horizontal? (b) The wire breaks and the rod rotates about the hinge. What is the angular speed of the rod as the rod passes through a horizontal position?arrow_forwardA cylinder of mass M and radius R is pulled by a constant force of magnitude Fapplied at the top horizontally. The cylinder rolls smoothly on the horizontal surface. Assume that M=10 kg, R=0.10 m, F=12 N, and note that the rotational inertia of the cylinder about its axis is 1/2MR^2 (a) Find the magnitude of the acceleration of thic center of mass of the cylinder (please input the numerical value in unit of m/s) (b) Find the magnitude of the angular acceleration of the contact point between the cylinder and the surface about the axis of the cylinder (please input the numerical value in unit of rad/s). (c) Find the magnitude of the frictional force acting on the cylinder (please input the numerical value in unit of N).arrow_forward
- acts on a pebble with position vector 7 = (1.92 m ) – (5.98 m )ê, re ), relative to the origin. What is the resulting torque acting on the pebble about (a) the origin and (b) a point with coordinates (8.32 m, 0, -7.80 m)? Force F = (4.70 N ) - (5.35 Nac (a) Number (b) Number i + i + k Units k Unitsarrow_forwardForce F = (5.58 N)î – (6.71 N )k acts on a pebble with position vector 7 = (2.79 m ) – (7.59 m ) k, relative to the origin. - What is the resulting torque acting on the pebble about (a) the origin and (b) a point with coordinates (3.43 m, 0, -3.30 m)? (a) Number i i k Units (b) Number i i i k Unitsarrow_forwardAs shown in Fig. 10-3, a mass m = 400 g hangs from the rim of a wheel of radius r = 15 cm. When released from rest, the mass falls 2.0 m in 6.5 s. Find the moment of inertia of the wheel. B 400 g mg FT FTarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
Moment of Inertia; Author: Physics with Professor Matt Anderson;https://www.youtube.com/watch?v=ZrGhUTeIlWs;License: Standard Youtube License