Chapter 11, Problem 11.50PAE

Interpretation Introduction

Interpretation: Given the decomposition of $S{O}_{2}C{l}_2$ and the table of total pressures at different time in the reaction vessel, determine how to convert the total pressures to partial pressures of $S{O}_{2}C{l}_2$, the order of the reaction and the rate constant.

Concept Introduction:

The integrated rate law equation explains how the concentrations of reactants change with time.

Consider a first order chemical reaction

$A\to B+C$.

The concentration of the reactant A at time t is given by the below equation

${\left[A\right]}_t ={\left[A\right]}_o \times e^{–kt}$

Where, ${\left[A\right]}_o$ is its initial concentration, k is the first-order rate constant and the "e" in the exponential term is of course the base of the natural logarithms. The negative sign in the power of e means that the value of this term diminishes as t increases, which means it is a decaying process.

The integrated rate law for this first order reaction is obtained by taking the natural logarithm of both sides of ${\left[A\right]}_t ={\left[A\right]}_o \times e^{–kt}$

That is,

$ln{\left[A\right]}_t=–kt +ln{\left[A\right]}_o$

Using Dalton's law, the partial pressure of formic acid is given by

$P_i=x_{i}P$

Where, $P_i$ is Partial pressure of gas i in a mixture of gases

$x_i$ is the mole fraction of gas i in a mixture of gases

The order of the reaction can be determined from a plot of concentration against time.

If we plot concentration against time, and if the curve is linear, the reaction is a zero order reaction.

If we plot log of concentration against time and if the curve is linear, the reaction is a first order reaction.

If we plot concentration inverse against time and if the curve is linear, the reaction is a second order reaction.

Answer to Problem 11.50PAE

Solution: The rate constant is $7.1\times {10}^{-4}{s}^{-1}$ and the order of decomposition of $S{O}_{2}C{l}_2$ is first order reaction

Explanation of Solution

Given Information: The table containing the total pressures in the reaction vessel during the decomposition of $S{O}_{2}C{l}_2$

Decomposition of $S{O}_{2}C{l}_2$ in the atmosphere is shown in the following reaction:

$S{O}_{2}C{l}_2(g)\to S{O}_2(g)+C{l}_2(g)$

The initial partial pressure of $S{O}_{2}C{l}_2$ is 491.7 torr.

Calculate the partial pressure of $S{O}_{2}C{l}_2$ at any given time using Dalton's law

Partial pressure of $S{O}_{2}C{l}_2$ = $(491.7-x)torr$

Partial pressure of $S{O}_2$ = $(x)torr$

Partial pressure of $C{l}_2$ = $(x)torr$

Therefore, the total pressure at any given time t is given as

$\begin{array}{l}{P}_{total}=P(S{O}_{2}C{l}_2)+P(C{l}_2)+P(S{O}_2)\\ P_{total}=[(491.7-x)+x+x]=[491.7+x]\\ P(S{O}_{2}C{l}_2)=491.7-x=491.7-(P_{total}-491.7)=983.4-P_{total}\end{array}$

Time(t)	Total pressure $P_{total}$	$P(S{O}_{2}C{l}_2)=983.4-P_{total}$
0	491.7	491.7
185.3	549.6	434.0
242.8	566.6	417.0
304.5	584.1	399.5
362.7	599.9	383.7
429.5	617.2	366.4
509.7	637.0	346.6
606.3	659.5	324.1

We need to plot these values of partial pressure with time to see if the reaction is zero order or not

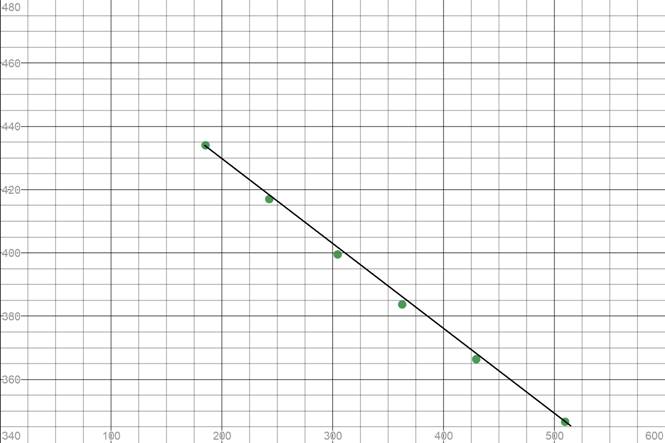
As some points do not lie on the straight line, the curve is not linear. Thus, it is not a zero order reaction.

Time(t)	$P(S{O}_{2}C{l}_2)=983.4-P_{total}$	$\ln P(S{O}_{2}C{l}_2)$
0	491.7	6.1978687744
185.3	434.0	6.0730445341
242.8	417.0	6.0330862218
304.5	399.5	5.9902137652
362.7	383.7	5.9498609973
429.5	366.4	5.9037256328
509.7	346.6	5.8481713773
606.3	324.1	5.7810521101

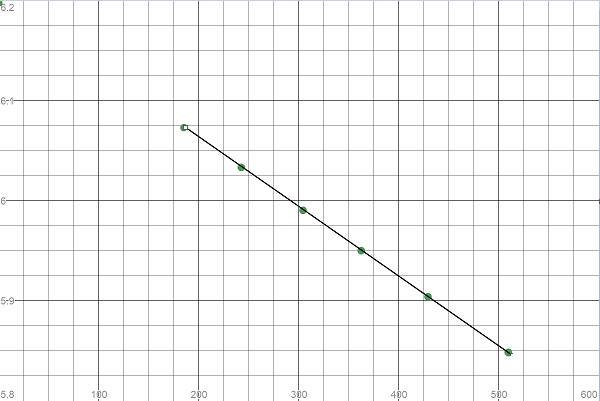
Here we see the curve is linear and thus the reaction is a first order reaction.

To calculate the rate constant, we need the negative slope of the line in the plot

$Slope=\frac{(6.21)-(5.78)}{0-606.3}=-7.1\times {10}^{-4}{s}^{-1}$

Hence, the rate constant is the negative of the slope obtained. It is equal to $7.1\times {10}^{-4}{s}^{-1}$

Conclusion

The concept of integrated rate law and the manipulation the data into a plot helps in determining the order of the decomposition of $S{O}_{2}C{l}_2$ and the rate constant with the help of total pressure given at various time t.