
Concept explainers
(a)
The principal mass moment of inertias at the origin.
Answer to Problem B.74P
The principal mass moment of inertias at the origin are
Explanation of Solution
Given information:
The mass per unit length of the steel is
Draw the diagram for the different section of the body.
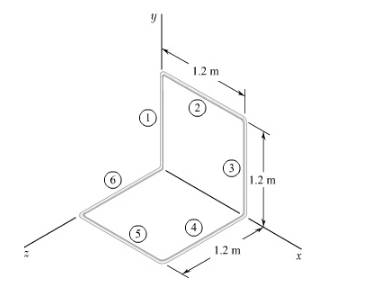
Figure-(1)
Concept used:
Write the expression for the mass of each section.
Here, the mass per unit length is
Write the expression of mass moment of inertia of section 1 about
Write the expression of mass moment of inertia of section 2 about
Write the expression of total mass moment of inertia about
Here, the mass moment of inertia for section 3 about x- axis is
The mass moment of inertia for section 1 is equal to the mass moment of inertia for section 3.
The mass moment of inertia for section 1 is equal to the mass moment of inertia for section 4.
The mass moment of inertia for section 1 is equal to the mass moment of inertia for section 6.
The mass moment of inertia for section 2 is equal to the mass moment of inertia for section 5.
Substitute
Write the expression of total mass moment of inertia about
Here, the mass moment of inertia for section 3 about y- axis is
The mass moment of inertia for section 1 is equal to zero.
The mass moment of inertia for section 4 is equal to the mass moment of inertia for section 5.
The mass moment of inertia for section 2 is equal to the mass moment of inertia for section 6.
Substitute
Write the expression of mass moment of inertia of section 2 about
Write the expression of mass moment of inertia of section 3 about
Write the expression of mass moment of inertia of section 4 about
The figure below illustrates the centroidal axis of a component.
Figure-(2)
From the symmetry in above figure about
Here, the product mass moment of inertia in
From the symmetry in the above figure about
Here, the product mass moment of inertia in
From the symmetry in the above figure about
Here, the product mass moment of inertia in
Write the expression for product of mass moment of inertia in
Here, the product mass moment of inertia is
Write the expression for product mass moment of inertia in
Here, the product mass moment of inertia is
Write the expression for product mass moment of inertia in
Here, the product mass moment of inertia in
Write the expression of mass moment of inertia with respect o origin along the unit vector
Here, the mass moment of inertia with respect to origin along unit vector
Calculation:
Substitute
Substitute
Substitute
Substitute
Substitute
Substitute
Substitute
Substitute
The mass moment of inertia about z- axis and the mass moment of inertia about y- axis is equal due to symmetry.
Hence,
Substitute
Substitute
Substitute
After solving the above equation,
Conclusion:
The principal mass moment of inertias at the origin are
(b)
The principal axis about the origin.
Answer to Problem B.74P
The principal axis about the origin are
Explanation of Solution
Given information:
The mass per unit length of the steel is
Draw the diagram for the different section of the body.
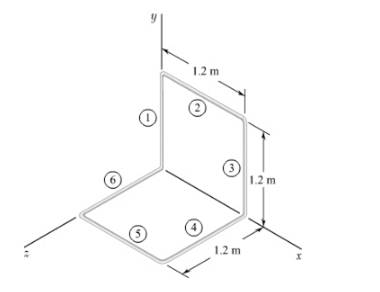
Figure-(1)
Calculation:
The direction cosine is calculated as follows:
Substitute the values from the sub-part (a) in above equations as follows:
After solving above equations,
Direction cosine in x direction is calculated as follows:
Direction cosine in y and z direction are,
So, the direction is calculated as follows:
Again,
The direction cosine is calculated as follows:
Substitute the values from the sub-part (a) in above equations as follows:
After solving above equations,
Direction cosine in x direction is calculated as follows:
Direction cosine in z direction is,
So, the direction is calculated as follows:
Similarly,
The direction cosine is calculated as follows:
Substitute the values from the sub-part (a) in above equations as follows:
After solving above equations,
Direction cosine in x direction is calculated as follows:
Direction cosine in y and z direction are,
So, the direction is calculated as follows:
The sketch is shown below:
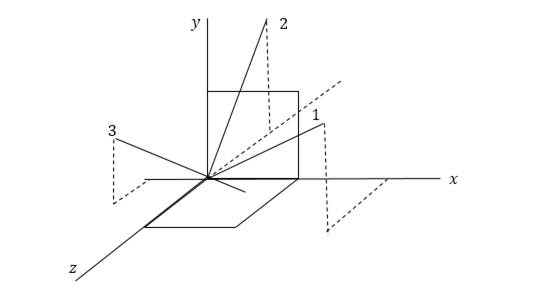
Conclusion:
So, the principal axis about the origin are
Want to see more full solutions like this?
Chapter B Solutions
Vector Mechanics For Engineers
- The flow rate is 12.275 Liters/s and the diameter is 6.266 cm.arrow_forwardAn experimental setup is being built to study the flow in a large water main (i.e., a large pipe). The water main is expected to convey a discharge (Qp). The experimental tube will be built at a length scale of 1/20 of the actual water main. After building the experimental setup, the pressure drop per unit length in the model tube (APm/Lm) is measured. Problem (20): Given the value of APm/Lm [kPa/m], and assuming pressure coefficient similitude, calculate the drop in the pressure per unit length of the water main (APP/Lp) in [Pa/m]. Givens: AP M/L m = 590.637 kPa/m meen Answers: ( 1 ) 59.369 Pa/m ( 2 ) 73.83 Pa/m (3) 95.443 Pa/m ( 4 ) 44.444 Pa/m *******arrow_forwardFind the reaction force in y if Ain = 0.169 m^2, Aout = 0.143 m^2, p_in = 0.552 atm, Q = 0.367 m^3/s, α = 31.72 degrees. The pipe is flat on the ground so do not factor in weight of the pipe and fluid.arrow_forward
- Find the reaction force in x if Ain = 0.301 m^2, Aout = 0.177 m^2, p_in = 1.338 atm, Q = 0.669 m^3/s, and α = 37.183 degreesarrow_forwardProblem 5: Three-Force Equilibrium A structural connection at point O is in equilibrium under the action of three forces. • • . Member A applies a force of 9 kN vertically upward along the y-axis. Member B applies an unknown force F at the angle shown. Member C applies an unknown force T along its length at an angle shown. Determine the magnitudes of forces F and T required for equilibrium, assuming 0 = 90° y 9 kN Aarrow_forwardProblem 19: Determine the force in members HG, HE, and DE of the truss, and state if the members are in tension or compression. 4 ft K J I H G B C D E F -3 ft -3 ft 3 ft 3 ft 3 ft- 1500 lb 1500 lb 1500 lb 1500 lb 1500 lbarrow_forward
- Problem 14: Determine the reactions at the pin A, and the tension in cord. Neglect the thickness of the beam. F1=26kN F2 13 12 80° -2m 3marrow_forwardProblem 22: Determine the force in members GF, FC, and CD of the bridge truss and state if the members are in tension or compression. F 15 ft B D -40 ft 40 ft -40 ft 40 ft- 5 k 10 k 15 k 30 ft Earrow_forwardProblem 20: Determine the force in members BC, HC, and HG. After the truss is sectioned use a single equation of equilibrium for the calculation of each force. State if the members are in tension or compression. 5 kN 4 kN 4 kN 3 kN 2 kN B D E F 3 m -5 m- -5 m- 5 m 5 m-arrow_forward
- An experimental setup is being built to study the flow in a large water main (i.e., a large pipe). The water main is expected to convey a discharge (Qp). The experimental tube will be built at a length scale of 1/20 of the actual water main. After building the experimental setup, the pressure drop per unit length in the model tube (APm/Lm) is measured. Problem (19): Given the value of Qp [m³/s], and assuming Reynolds number similitude between the water main and experimental tube, calculate the flow rate in the model tube (Qm) in [lit/s]. = 30.015 m^3/sarrow_forwardProblem 11: The lamp has a weight of 15 lb and is supported by the six cords connected together as shown. Determine the tension in each cord and the angle 0 for equilibrium. Cord BC is horizontal. E 30° B 60° Aarrow_forwardProblem 10: If the bucket weighs 50 lb, determine the tension developed in each of the wires. B $30° 5 E D 130°arrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





