Vector Mechanics for Engineers: Statics and Dynamics
Vector Mechanics for Engineers: Statics and Dynamics
11th Edition
ISBN: 9780073398242
Author: Ferdinand P. Beer, E. Russell Johnston Jr., David Mazurek, Phillip J. Cornwell, Brian Self
Publisher: McGraw-Hill Education
bartleby

Concept explainers

Moment of Inertia
Inertia can be termed as a resistance offered by a body to change its state. A rigid body initially at rest will apply resistance when acted by an external force that tends to change its state of rest or velocity, or a body initially at motion will provi…
bartleby

Videos

Question
Book Icon
Chapter 9.5, Problem 9.139P
To determine

Find the mass moment of inertia of the anchor with respect to each of the coordinate axes.

Expert Solution & Answer
Check Mark

Answer to Problem 9.139P

The mass moment of inertia (Ix) of the anchor with respect to x axis is 344×10−6 lb⋅ft⋅s2_.

The mass moment of inertia (Iy) of the anchor with respect to y axis is 132.1×10−6 lb⋅ft⋅s2_.

The mass moment of inertia (Iz) of the anchor with respect to z axis is 453×10−6 lb⋅ft⋅s2_.

Explanation of Solution

Given information:

The thickness (t) of the anchor is 0.05 in.

The specific weight (γ) of the galvanized steel is 470 lb/ft3.

Assume the acceleration due to gravity (g) as 32.2 ft/s2

Calculation:

Show the section 1, section 2 and section 3 of the framing anchor as in Figure 1.

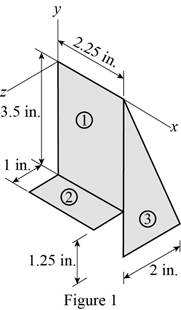
Vector Mechanics for Engineers: Statics and Dynamics, Chapter 9.5, Problem 9.139P

Calculate the density (ρ) of the galvanized steel using the relation:

ρ=γg

Substitute 470 lb/ft3 for γ and 32.2 ft/s2 for g.

ρ=47032.2=14.596 lb⋅s2/ft4

Calculate the volume of the section 1 (V1) using the relation:

V1=A1t=3.5×2.25×t

Substitute 0.05 in. for t.

V1=3.5×2.25×0.05=0.39375 in.3×(1 ft12 in.)3=2.2786×10−4ft3

Calculate the volume of the section 2 (V2) as below:

V2=A2t=1×2.25×t

Substitute 0.05 in. for t.

V2=1×2.25×0.05=0.1125 in.3×(1 ft12 in.)3=6.5104×10−5ft3

Calculate the volume (V3) of the section 3 as below:

V3=A3t=12(3.5+1.25)×2×t

Substitute 0.05 in. for t.

V3=12×(3.5+1.25)×2×0.05=0.2375 in.3×(1 ft12 in.)3=1.3744×10−4ft3

Calculate the mass of section 1 (m1) using the relation:

m1=ρV1

Substitute 2.2786×10−4ft3 for V1 and 14.596 lb⋅s2/ft4 for ρ.

m1=14.596 ×2.2786×10−4=3325.845×10−6 lb⋅s2/ft

Calculate the mass of section 2 (m2) using the relation:

m2=ρV2

Substitute 6.5104×10−5ft3 for V2 and 14.596 lb⋅s2/ft4 for ρ.

m2=14.596 ×6.5104×10−5=950.26×10−6 lb⋅s2/ft

Calculate the mass of section 3 (m3) using the relation:

m3=ρV3

Substitute 1.3744×10−4ft3 for V3 and 14.596 lb⋅s2/ft4 for ρ.

m3=14.596 ×1.3744×10−4=2006.14×10−6 lb⋅s2/ft

Calculate the mass moment of inertia with respect to x axis for section 1 (Ix)1 as below:

(Ix)1=112(m1)(3.5)2+(m1×(3.52)2)

Substitute 3325.845×10−6 lb⋅s2/ft for m1.

(Ix)1=112(3325.845×10−6 lb⋅s2/ft)(3.5 in.×1 ft12 in.)2+(3325.845×10−6 lb⋅s2/ft×((3.5 in.)×1 ft12 in.2)2)=2.3577×10−5+7.073×10−5=9.4307 ×10−5 lb⋅ft⋅s2

Calculate the mass moment of inertia with respect to x axis for  section 2 (Ix)2 as below:

(Ix)2=112(m2)(1)2+(m2×(3.52+(12)2))

Substitute 950.26×10−6 lb⋅s2/ft for m2.

(Ix)2=112(950.26×10−6 lb⋅s2/ft)(1in.×1 ft12 in.)2+(950.26×10−6 lb⋅s2/ft× ((3.5 in.×1 ft12 in.)2+((1in.×1 ft12 in.)2)2))=5.4992×10−7+8.2488×10−5=83.037×10−6 lb⋅ft⋅s2

Calculate the mass moment of inertia with respect to x axis for section 3 (Ix)3 as below:

(Ix)3=118(m3)((3.5+1.25)2+22)+(m3×((23(3.5+1.25))2+(13×2)2))

Substitute 2006.14×10−6 lb⋅s2/ft for m3.

(Ix)3=118(2006.14×10−6 lb⋅s2/ft)(((3.5+1.25)in.×1 ft12 in.)2+(2in.×1 ft12 in.)2)+ +(2006.14×10−6 lb⋅s2/ft×((23((3.5+1.25)in.×1 ft12 in.))2+(13×2in.×1 ft12 in.)2))=2.0558×10−5+1.4589×10−4=166.452×10−6 lb⋅ft⋅s2

Calculate the mass moment of inertia with respect to x axis for anchor (Ix) using the parallel axis theorem as below:

Ix=(Ix)1+(Ix)2+(Ix)3

Substitute 9.4307 ×10−5 lb⋅ft⋅s2 for (Ix)1, 83.037×10−6 lb⋅ft⋅s2 for (Ix)2 and 166.452×10−6 lb⋅ft⋅s2 for (Ix)3.

Ix=9.4307 ×10−5 +83.037×10−6  +166.452×10−6=3.437×10−4 lb⋅ft⋅s2≈344×10−6 lb⋅ft⋅s2

Calculate the mass moment of inertia with respect to y axis for section 1 (Iy)1 as below:

(Iy)1=112m1(2.25)2+m1(2.252)2

Substitute 3325.845×10−6 lb⋅s2/ft for m1.

(Iy)1={112×3325.845×10−6 lb⋅s2/ft×(2.25 in.×1 ft12 in.)2 +3325.845×10−6 lb⋅s2/ft×((2.25in.×1 ft12 in.)2)2}=9.7436×10−6+2.923×10−5=38.975×10−6 lb⋅ft⋅s2

Calculate the mass moment of inertia with respect to y axis for section 2 (Iy)2 as below:

(Iy)2=112m2((2.25)2+(1)2)+m2[(2.252)2+(12)2]

Substitute 950.26×10−6 lb⋅s2/ft for m2.

(Iy)2={112(950.26×10−6 lb⋅s2/ft)((2.25in.×1 ft12 in.)2+(1in.×1 ft12 in.)2) +950.26×10−6 lb⋅s2/ft[((2.25in.×1 ft12 in.)2)2+(1in.×1 ft12 in.2)2]}=3.334×10−6+1.00017×10−5=13.336×10−6 lb⋅ft⋅s2

Calculate the mass moment of inertia with respect to y axis for section 3 (Iy)3 as below:

(Iy)3=118m3(2)2+m3[2.252+(132)2]

Substitute 2006.14×10−6 lb⋅s2/ft for m3.

(Iy)3={118×2006.14×10−6 lb⋅s2/ft(2in.×1 ft12 in.)2 +2006.14×10−6 lb⋅s2/ft[(2.25in.×1 ft12 in.)2+(13×(2in.×1 ft12 in.))2]}=3.096×10−6+7.672×10−5=79.816×10−6 lb⋅ft⋅s2

Calculate the mass moment of inertia with respect to y axis for anchor (Iy) using the parallel axis theorem as below:

Iy=(Iy)1+(Iy)2+(Iy)3

Substitute 38.975×10−6lb⋅ft⋅s2 for (Iy)1 13.336×10−6 lb⋅ft⋅s2 for (Iy)2 and 79.816×10−6lb⋅ft⋅s2 for (Iy)3.

Iy=38.975×10−6 +13.336×10−6+79.816×10−6=132.127×10−6 lb⋅ft⋅s2≈132.1×10−6 lb⋅ft⋅s2

Calculate the mass moment of inertia with respect to z axis for section 1 (Iz)1 as below:

(Iz)1=112m1[(2.25)2+(3.5)2]+m1[(2.252)2+(3.52)2]

Substitute 3325.845×10−6 lb⋅s2/ft for m1.

(Iz)1={1123325.845×10−6 lb⋅s2/ft[(2.25in.×1 ft12 in.)2+(3.5in.×1 ft12 in.)2] +3325.845×10−6 lb⋅s2/ft[(2.25in.×1 ft12 in.2)2+(3.5in.×1 ft12 in.2)2]}=3.332×10−5+9.996×10−5=133.283×10−6lb⋅ft⋅s2

Calculate the mass moment of inertia with respect to z axis for section 2 (Ix)2 as below:

(Iz)2=112m2(2.25)2+m2[(2.252)2+(3.5)2]

Substitute 950.26×10−6 lb⋅s2/ft for m2.

(Iz)2={112×950.26×10−6 lb⋅s2/ft×(2.25in.×1 ft12 in.)2 +950.26×10−6 lb⋅s2/ft×[(2.25in.×1 ft12 in.2)2+(3.5in.×1 ft12 in.)2]}=2.784×10−6+8.918×10−5=91.974×10−6 lb⋅ft⋅s2

Calculate the mass moment of inertia with respect to z axis for section 3 (Iy)3 as below:

(Iz)3=118m3(3.5+1.25)2+m3[(2.25)2+(23(3.5+1.25))2]

Substitute 2006.14×10−6 lb⋅s2/ft for m3.

(Iz)3={118×2006.14×10−6 lb⋅s2/ft((3.5+1.25)in.×1 ft12 in.)2 +2006.14×10−6 lb⋅s2/ft×[(2.25in.×1 ft12 in.)2+(23((3.5+1.25)in.×1 ft12 in.))2]}=1.746×10−6+2.102×10−4=227.694×10−6 lb⋅ft⋅s2

Calculate the mass moment of inertia with respect to z axis for anchor (Ix) using the parallel axis theorem as below:

Iz=(Iz)1+(Iz)2+(Iz)3

Substitute 133.283×10−6lb⋅ft⋅s2 for (Iz)1 91.974×10−6 lb⋅ft⋅s2 for (Iz)2 and 227.694×10−5 lb⋅ft⋅s2 for (Iz)3.

Iz=133.283×10−6+91.974×10−6 +2.27.694×10−6 =452.951×10−6 lb⋅ft⋅s2≈453×10−6 lb⋅ft⋅s2

Therefore, the mass moment of inertia (Ix) of the anchor with respect to x axis is 344×10−6 lb⋅ft⋅s2_.

Therefore, the mass moment of inertia (Iy) of the anchor with respect to y axis is 132.1×10−6 lb⋅ft⋅s2_.

Therefore, the mass moment of inertia (Iz) of the anchor with respect to z axis is 453×10−6 lb⋅ft⋅s2_.

Want to see more full solutions like this?

Subscribe now to access step-by-step solutions to millions of textbook problems written by subject matter experts!
Previous
Chapter 9.5, Problem 9.138P
Next
Chapter 9.5, Problem 9.140P
Students have asked these similar questions
-6- 8 من 8 Mechanical vibration HW-prob-1 lecture 8 By: Lecturer Mohammed O. attea The 8-lb body is released from rest a distance xo to the right of the equilibrium position. Determine the displacement x as a function of time t, where t = 0 is the time of release. c=2.5 lb-sec/ft wwwww k-3 lb/in. 8 lb Prob. -2 Find the value of (c) if the system is critically damping. Prob-3 Find Meq and Ceq at point B, Drive eq. of motion for the system below. Ш H -7~ + 目 T T & T тт +
Q For the following plan of building foundation, Determine immediate settlement at points (A) and (B) knowing that: E,-25MPa, u=0.3, Depth of foundation (D) =1m, Depth of layer below base level of foundation (H)=10m. 3m 2m 100kPa A 2m 150kPa 5m 200kPa B
W PE 2 43 R² 80 + 10 + kr³ Ø8=0 +0 R²+J+ kr200 R² + J-) + k r² = 0 kr20 kr20 8+ W₁ = = 0 R²+1) R²+J+) 4 lec 8.pdf Mechanical vibration lecture 6 By: Lecturer Mohammed C. Attea HW1 (Energy method) Find equation of motion and natural frequency for the system shown in fig. by energy method. m. Jo 000 HW2// For the system Fig below find 1-F.B.D 2Eq.of motion 8 wn 4-0 (1) -5- m

Chapter 9 Solutions

Vector Mechanics for Engineers: Statics and Dynamics

Ch. 9.1 - 9.1 through 9.4 Determine by direct integration...Ch. 9.1 - 9.1 through 9.4 Determine by direct integration...Ch. 9.1 - Prob. 9.3PCh. 9.1 - Prob. 9.4PCh. 9.1 - 9.5 through 9.8 Determine by direct integration...Ch. 9.1 - 9.5 through 9.8 Determine by direct integration...Ch. 9.1 - Prob. 9.7PCh. 9.1 - Prob. 9.8PCh. 9.1 - 9.9 through 9.11 Determine by direct integration...Ch. 9.1 - Prob. 9.10P
Ch. 9.1 - Prob. 9.11PCh. 9.1 - Prob. 9.12PCh. 9.1 - Prob. 9.13PCh. 9.1 - 9.12 through 9.14 Determine by direct integration...Ch. 9.1 - Prob. 9.15PCh. 9.1 - Prob. 9.16PCh. 9.1 - Prob. 9.17PCh. 9.1 - Prob. 9.18PCh. 9.1 - Prob. 9.19PCh. 9.1 - Prob. 9.20PCh. 9.1 - Prob. 9.21PCh. 9.1 - Prob. 9.22PCh. 9.1 - Prob. 9.23PCh. 9.1 - 9.23 and 9.24 Determine the polar moment of...Ch. 9.1 - Prob. 9.25PCh. 9.1 - Prob. 9.26PCh. 9.1 - Prob. 9.27PCh. 9.1 - Prob. 9.28PCh. 9.1 - Prob. 9.29PCh. 9.1 - Prove that the centroidal polar moment of inertia...Ch. 9.2 - Prob. 9.31PCh. 9.2 - Prob. 9.32PCh. 9.2 - Prob. 9.33PCh. 9.2 - Prob. 9.34PCh. 9.2 - Prob. 9.35PCh. 9.2 - Prob. 9.36PCh. 9.2 - Prob. 9.37PCh. 9.2 - Prob. 9.38PCh. 9.2 - Prob. 9.39PCh. 9.2 - Prob. 9.40PCh. 9.2 - Prob. 9.41PCh. 9.2 - 9.41 through 9.44 Determine the moments of inertia...Ch. 9.2 - Prob. 9.43PCh. 9.2 - Prob. 9.44PCh. 9.2 - 9.45 and 9.46 Determine the polar moment of...Ch. 9.2 - Prob. 9.46PCh. 9.2 - Prob. 9.47PCh. 9.2 - 9.47 and 9.48 Determine the polar moment of...Ch. 9.2 - 9.49 Two channels and two plates are used to form...Ch. 9.2 - Prob. 9.50PCh. 9.2 - Prob. 9.51PCh. 9.2 - Two 20-mm steel plates are welded to a rolled S...Ch. 9.2 - A channel and a plate are welded together as shown...Ch. 9.2 - Prob. 9.54PCh. 9.2 - Two L76 76 6.4-mm angles are welded to a C250 ...Ch. 9.2 - Prob. 9.56PCh. 9.2 - Prob. 9.57PCh. 9.2 - 9.57 and 9.58 The panel shown forms the end of a...Ch. 9.2 - Prob. 9.59PCh. 9.2 - 9.60 The panel shown forms the end of a trough...Ch. 9.2 - Prob. 9.61PCh. 9.2 - Prob. 9.62PCh. 9.2 - Prob. 9.63PCh. 9.2 - Prob. 9.64PCh. 9.2 - Prob. 9.65PCh. 9.2 - Prob. 9.66PCh. 9.3 - 9.67 through 9.70 Determine by direct integration...Ch. 9.3 - Prob. 9.68PCh. 9.3 - Prob. 9.69PCh. 9.3 - Prob. 9.70PCh. 9.3 - Prob. 9.71PCh. 9.3 - Prob. 9.72PCh. 9.3 - Prob. 9.73PCh. 9.3 - 9.71 through 9.74 Using the parallel-axis theorem,...Ch. 9.3 - Prob. 9.75PCh. 9.3 - 9.75 through 9.78 Using the parallel-axis theorem,...Ch. 9.3 - Prob. 9.77PCh. 9.3 - Prob. 9.78PCh. 9.3 - Determine for the quarter ellipse of Prob. 9.67...Ch. 9.3 - Determine the moments of inertia and the product...Ch. 9.3 - Determine the moments of inertia and the product...Ch. 9.3 - 9.75 through 9.78 Using the parallel-axis theorem,...Ch. 9.3 - Determine the moments of inertia and the product...Ch. 9.3 - Determine the moments of inertia and the product...Ch. 9.3 - Prob. 9.85PCh. 9.3 - 9.86 through 9.88 For the area indicated,...Ch. 9.3 - Prob. 9.87PCh. 9.3 - Prob. 9.88PCh. 9.3 - Prob. 9.89PCh. 9.3 - 9.89 and 9.90 For the angle cross section...Ch. 9.4 - Using Mohrs circle, determine for the quarter...Ch. 9.4 - Using Mohrs circle, determine the moments of...Ch. 9.4 - Prob. 9.93PCh. 9.4 - Using Mohrs circle, determine the moments of...Ch. 9.4 - Using Mohrs circle, determine the moments of...Ch. 9.4 - Using Mohrs circle, determine the moments of...Ch. 9.4 - For the quarter ellipse of Prob. 9.67, use Mohrs...Ch. 9.4 - 9.98 though 9.102 Using Mohrs circle, determine...Ch. 9.4 - Prob. 9.99PCh. 9.4 - 9.98 though 9.102 Using Mohrs circle, determine...Ch. 9.4 - Prob. 9.101PCh. 9.4 - Prob. 9.102PCh. 9.4 - Prob. 9.103PCh. 9.4 - 9.104 and 9.105 Using Mohrs circle, determine the...Ch. 9.4 - 9.104 and 9.105 Using Mohrs circle, determine the...Ch. 9.4 - For a given area, the moments of inertia with...Ch. 9.4 - it is known that for a given area Iy = 48 106 mm4...Ch. 9.4 - Prob. 9.108PCh. 9.4 - Prob. 9.109PCh. 9.4 - Prob. 9.110PCh. 9.5 - A thin plate with a mass m is cut in the shape of...Ch. 9.5 - A ring with a mass m is cut from a thin uniform...Ch. 9.5 - Prob. 9.113PCh. 9.5 - The parabolic spandrel shown was cut from a thin,...Ch. 9.5 - Prob. 9.115PCh. 9.5 - Fig. P9.115 and P9.116 9.116 A piece of thin,...Ch. 9.5 - 9.117 A thin plate with a mass m has the...Ch. 9.5 - Prob. 9.118PCh. 9.5 - 9.119 Determine by direct integration the mass...Ch. 9.5 - The area shown is revolved about the x axis to...Ch. 9.5 - 9.121 The area shown is revolved about the x axis...Ch. 9.5 - Prob. 9.122PCh. 9.5 - Prob. 9.123PCh. 9.5 - Prob. 9.124PCh. 9.5 - Prob. 9.125PCh. 9.5 - Prob. 9.126PCh. 9.5 - Prob. 9.127PCh. 9.5 - Prob. 9.128PCh. 9.5 - Prob. 9.129PCh. 9.5 - Prob. 9.130PCh. 9.5 - Prob. 9.131PCh. 9.5 - The cups and the arms of an anemometer are...Ch. 9.5 - Prob. 9.133PCh. 9.5 - Determine the mass moment of inertia of the 0.9-lb...Ch. 9.5 - Prob. 9.135PCh. 9.5 - Prob. 9.136PCh. 9.5 - Prob. 9.137PCh. 9.5 - A section of sheet steel 0.03 in. thick is cut and...Ch. 9.5 - Prob. 9.139PCh. 9.5 - A farmer constructs a trough by welding a...Ch. 9.5 - The machine element shown is fabricated from...Ch. 9.5 - Determine the mass moments of inertia and the...Ch. 9.5 - Determine the mass moment of inertia of the steel...Ch. 9.5 - Prob. 9.144PCh. 9.5 - Determine the mass moment of inertia of the steel...Ch. 9.5 - Aluminum wire with a weight per unit length of...Ch. 9.5 - The figure shown is formed of 18-in.-diameter...Ch. 9.5 - A homogeneous wire with a mass per unit length of...Ch. 9.6 - Determine the mass products of inertia Ixy, Iyz,...Ch. 9.6 - Determine the mass products of inertia Ixy, Iyz,...Ch. 9.6 - Prob. 9.151PCh. 9.6 - Determine the mass products of inertia Ixy, Iyz,...Ch. 9.6 - 9.153 through 9.156 A section of sheet steel 2 mm...Ch. 9.6 - Prob. 9.154PCh. 9.6 - Prob. 9.155PCh. 9.6 - 9.153 through 9.156 A section of sheet steel 2 mm...Ch. 9.6 - Prob. 9.157PCh. 9.6 - Prob. 9.158PCh. 9.6 - Prob. 9.159PCh. 9.6 - Prob. 9.160PCh. 9.6 - Prob. 9.161PCh. 9.6 - For the homogeneous tetrahedron of mass m shown,...Ch. 9.6 - Prob. 9.163PCh. 9.6 - Prob. 9.164PCh. 9.6 - Prob. 9.165PCh. 9.6 - Determine the mass moment of inertia of the steel...Ch. 9.6 - Prob. 9.167PCh. 9.6 - Prob. 9.168PCh. 9.6 - Prob. 9.169PCh. 9.6 - 9.170 through 9.172 For the wire figure of the...Ch. 9.6 - Prob. 9.171PCh. 9.6 - Prob. 9.172PCh. 9.6 - Prob. 9.173PCh. 9.6 - Prob. 9.174PCh. 9.6 - Prob. 9.175PCh. 9.6 - Prob. 9.176PCh. 9.6 - Prob. 9.177PCh. 9.6 - Prob. 9.178PCh. 9.6 - Prob. 9.179PCh. 9.6 - Prob. 9.180PCh. 9.6 - Prob. 9.181PCh. 9.6 - Prob. 9.182PCh. 9.6 - Prob. 9.183PCh. 9.6 - Prob. 9.184PCh. 9 - Determine by direct integration the moments of...Ch. 9 - Determine the moment of inertia and the radius of...Ch. 9 - Prob. 9.187RPCh. 9 - Prob. 9.188RPCh. 9 - Prob. 9.189RPCh. 9 - Two L4 4 12-in. angles are welded to a steel...Ch. 9 - Prob. 9.191RPCh. 9 - Prob. 9.192RPCh. 9 - Prob. 9.193RPCh. 9 - Prob. 9.194RPCh. 9 - Prob. 9.195RPCh. 9 - Determine the mass moment of inertia of the steel...
Knowledge Booster
Background pattern image
Mechanical Engineering
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
Text book image
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Text book image
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Text book image
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Text book image
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Text book image
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Text book image
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY
SEE MORE TEXTBOOKS
moment of inertia; Author: NCERT OFFICIAL;https://www.youtube.com/watch?v=A4KhJYrt4-s;License: Standard YouTube License, CC-BY