
Concept explainers
The moment of inertia of a system of four particles, about an axis passing through its center of mass and parallel to the z axis.
Answer to Problem 42P
The moment of inertia of a system of four particles, one each at the corners of a square of edge
Explanation of Solution
Given info:
The masses of the balls:
The length of the edge of the cube
The moment of inertia of the system about the z axis
Formula used:
The moment of inertia of a particle of mass
Where,
The moment of inertia of a system of particles about an axis is equal to the sum of moments of inertia of the individual particles about the axis of rotation.
The coordinates
Here,
According to parallel axis theorem,
Here,
Calculation:
Four particles of masses
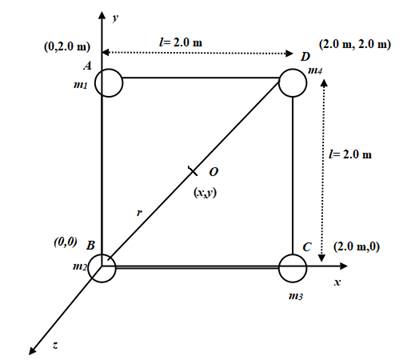
Figure 1
The mass
Use equations (3) and (4) to determine the coordinates
The center of mass of the system of 4 particles lies at
Determine the distance
Substitute the values of
Determine the value of the mass
Rewrite equation (5) for
Substitute the values of the variables in equation (8) and calculate the moment of inertia of the system of particles about the axis passing through the center of gravity and parallel to the z axis.
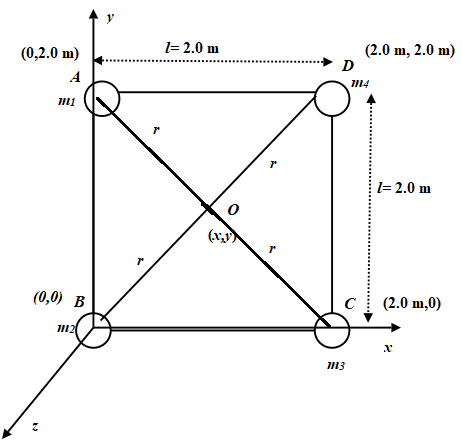
Figure 2
The particles of masses
Substitute the values of the given quantities in equation (9) and determine the value of
The calculated value agrees with the value calculated using the parallel axis theorem.
Conclusion:
Thus, the moment of inertia of a system of four particles, one each at the corners of a square of edge
Want to see more full solutions like this?
Chapter 9 Solutions
Physics for Scientists and Engineers
- The human eye is most sensitive to light having a frequency of about 5.5 × 1014 Hz, which is in the yellow-green region of the electromagnetic spectrum. How many wavelengths of this light can fit across a distance of 2.2 cm?arrow_forwardA one-dimensional harmonic oscillator of mass m and angular frequency w is in a heat bath of temperature T. What is the root mean square of the displacement of the oscillator? (In the expressions below k is the Boltzmann constant.) Select one: ○ (KT/mw²)1/2 ○ (KT/mw²)-1/2 ○ kT/w O (KT/mw²) 1/2In(2)arrow_forwardTwo polarizers are placed on top of each other so that their transmission axes coincide. If unpolarized light falls on the system, the transmitted intensity is lo. What is the transmitted intensity if one of the polarizers is rotated by 30 degrees? Select one: ○ 10/4 ○ 0.866 lo ○ 310/4 01/2 10/2arrow_forward
- Before attempting this problem, review Conceptual Example 7. The intensity of the light that reaches the photocell in the drawing is 160 W/m², when 0 = 18°. What would be the intensity reaching the photocell if the analyzer were removed from the setup, everything else remaining the same? Light Photocell Polarizer Insert Analyzerarrow_forwardThe lifetime of a muon in its rest frame is 2.2 microseconds. What is the lifetime of the muon measured in the laboratory frame, where the muon's kinetic energy is 53 MeV? It is known that the rest energy of the muon is 106 MeV. Select one: O 4.4 microseconds O 6.6 microseconds O 3.3 microseconds O 1.1 microsecondsarrow_forwardThe Lagrangian of a particle performing harmonic oscil- lations is written in the form L = ax² - Bx² - yx, where a, and are constants. What is the angular frequency of oscillations? A) √2/a B) √(+2a)/B C) √√Ba D) B/αarrow_forward
- The mean temperature of the Earth is T=287 K. What would the new mean temperature T' be if the mean distance between the Earth and the Sun was increased by 2%? Select one: ○ 293 K O 281 K ○ 273 K 284 Karrow_forwardTwo concentric current-carrying wire loops of radius 3 cm and 9 cm lie in the same plane. The currents in the loops flow in the same direction and are equal in magnitude. The magnetic field at the common center of the loops is 50 mT. What would be the value of magnetic field at the center if the direction of the two currents was opposite to each other (but their value is kept constant)? Select one: ○ 20 mT ○ 10 mT O 15 mT ○ 25 mTarrow_forwardAn ideal coil of inductivity 50 mH is connected in series with a resistor of 50 ohm. This system is connected to a 4.5 V battery for a long time. What is the current in the circuit? Select one: O 45 mA ○ 90 mA 00 mA O 150 mAarrow_forward
- There are two thin-walled spherical shells made from the same material, the radius of the smaller shell is half of the radius of the larger one. The thickness of the walls is the same. Denote the moment of inertia (with respect to the center) of the larger shell by I₁, and that of the smaller one by 12. What is the ratio I₁/12? Select one: ○ 8 O 16 O 4 ○ 32arrow_forwardA swimming pool has dimensions 20.0 m X 20.0 m and a flat bottom. The pool is filled to a depth of 3.00 m with fresh water. By what force does the water push each of the sidewalls? Density of water is 1000 kg/m³. Select one: ○ ~ 900 KN о ~ 2 ~ 1800 kN 600 kN 1500 kNarrow_forwardFrom one corner of a thin homogeneous square metal sheet with sides of L = 20 cm is cut an L/2 square sheet as shown in the figure. Approximately how far away is the centre of mass of the resulting shape from the centre P of the original square? P ○ 24 mm ○ 42 mm ○ 32 mm ○ 16 mmarrow_forward
 Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning
Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





