Chapter 8.5, Problem 49AYU

To determine

To find: Describe the motion of the object. Be sure to give the mass and damping factor.

b. What is the initial displacement of the bob? That is, what is the displacement at $\text{t} = 0$?

c. Graph the motion using a graphing utility.

d. What is the displacement of the bob at the start of the second oscillation?

e. What happens to the displacement of the bob as time increases without bound?

Answer to Problem 49AYU

a. It is damped motion with a bob of mass 35kg and a damping factor of $0.5\text{kg}/\text{sec}$

b. Initial displacement is $-30$ meters leftward

c. Graph is plotted

d. Displacement of the bob at the second oscillation is $29.155$ meters leftwards.

e. Hence $t$ increases displacement tends to become 0 or the bob comes to rest.

Explanation of Solution

Given:

The distance $\text{d}$ (in meters) of the bob of a pendulum of mass $\text{m}$ (in kilograms) from its rest position at time $\text{t}$ (in seconds) is given. The bob is released from the left of its rest position and represents a negative direction.

$d\left(t\right) = - 15e^{ - \frac{0.9t}{30}}\text{cos}\sqrt{\left(\frac{\pi }{3}\right)² - \frac{.81}{900}}t$

Formula used:

The displacement $\text{d}$ of an oscillating object from its at–rest position at time $\text{t}$ is given by $\text{d}\left(\text{t}\right) = {\text{ ae}}^{ - \frac{\text{bt}}{2\text{m}}}\text{cos}\sqrt{{\text{ω}}^2 - \frac{{\text{b}}^2}{4{\text{m}}^2}}\text{t}$,

where $\text{b}$ is the damping factor or damping coefficient and $\text{m}$ is the mass of the oscillating object. Here $\left|a\right|$ is the displacement at $\text{t} = 0$, and $\frac{2\text{π}}{\text{w}}$ is the period under simple harmonic motion (no damping).

Calculation:

a. $d\left(t\right) = - 15e^{ - \frac{0.9t}{30}}\text{cos}\sqrt{\left(\frac{\pi }{3}\right)² - \frac{.81}{900}}t$

$\text{d}\left(\text{t}\right) = {\text{ ae}}^{ - \frac{\text{bt}}{2\text{m}}}\text{cos}\sqrt{{\text{ω}}^2 - \frac{{\text{b}}^2}{4{\text{m}}^2}}\text{t}$,

From the given equation

b the damping factor is $\text{m} = 0.9\text{kg}/\text{sec}$ and

mass of the bob is ${\text{m}}^2$

$4{\text{m}}^2 = 900, \text{m} = 15 \text{kg}$

It is damped motion with a bob of mass 15kg and a damping factor of $0.9\text{kg}/\text{sec}$

b. To find the initial displacement let us substitute $\text{t} = 0$ in the given equation.

$d\left(0\right) = - 15e^{ - \frac{0.9 * 0}{30}}\text{cos}\sqrt{\left(\frac{\pi }{3}\right)² - \frac{.81}{900}} * 0$

Initial displacement is $-15$ meters leftward

c. The graph of $d\left(t\right) = - 15e^{ - \frac{0.9 * t}{30}}\text{cos}\sqrt{\left(\frac{\pi }{3}\right)² - \frac{.81}{900}} * t$

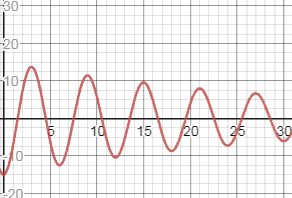
d. From the graph below we see that the displacement of the bob at the second oscillation is $- 12.533$, that is $12.533$ meters leftwards.

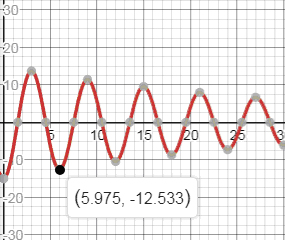
e. Displacement of the bob as t increases $e^{ - \frac{0.9 * 0}{30}}$ tends to 0. Hence $\text{t}$ increases displacement tends to become 0 or the bob comes to rest.