Chapter 8, Problem 76PP

BIO The Flight of the Dragonflies

Of all the animals you’re likely to see on a summer day, the most ancient is the dragonfly. In fact, the fossil record for dragonflies extends back over 250 million years, more than twice as long as for birds. Ancient dragonflies could be as large as a hawk, and were surely buzzing around the heads of both T. Rex and Triceratops.

Dragonflies belong to the order Odonata (“toothed jaws”) and the suborder Anisoptera (“different wings”), a reference to the fact that their hindwings are wider front-to-back than their forewings. (Damselflies. in contrast, have forewings and hindwings that are the same width ) Although ancient in their lineage, dragonflies are among the fastest flying and most acrobatic of all insects; some of their maneuvers subject them to accelerations as great as 20g.

The properties of dragonfly wings, and how they account for such speed and mobility, have been of great interest to biologists Figure 8-47 (a) shows an experimental setup designed to measure the force constant of Plexiglas models of wings, which are used in wind tunnel tests A downward force is applied to the model wing at the tip (1 for hindwing, 2 for forewing) or at two-thirds the distance to the tip (3 for hindwing, 4 for forewing). As the force is varied in magnitude, the resulting deflection of the wing is measured The results are shown in Figure 8-47 (b) Notice that significant differences are seen between the hindwings and forewings, as one might expect from their different shapes.

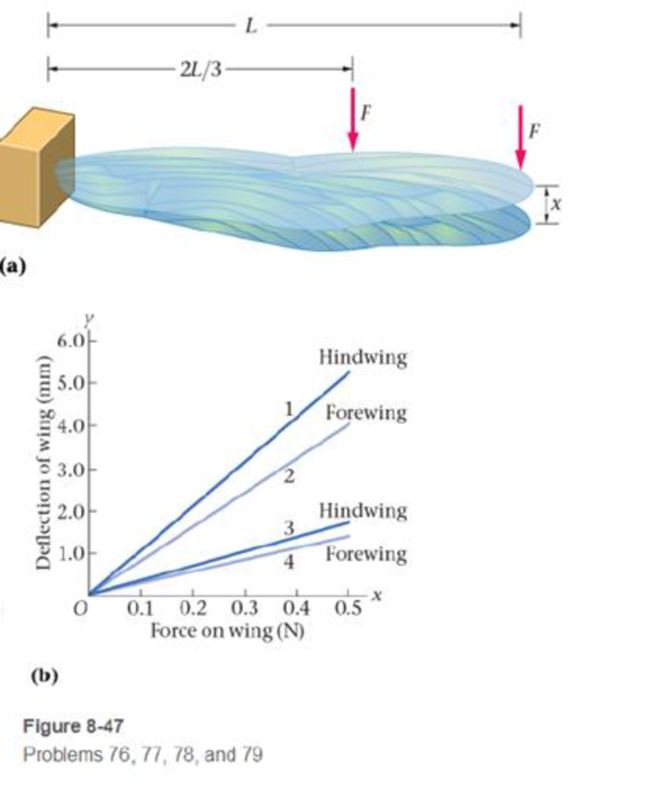
76. • Treating the model wing as an ideal spring what is the force constant of the hindwing when a force is applied to its tip?

1. A.    94 N/m
2. B.     130 N/m
3. C.     290 N/m
4. D.    330 N/m