
Concept explainers
Refer to the circuit of Fig. 8.95, which contains a voltage-controlled dependent voltage source in addition to two resistors. (a) Compute the circuit time constant. (b) Obtain an expression for vx valid for all t. (c) Plot the power dissipated in the 4 Ω resistor over the range of six time constants. (d) Repeat parts (a) to (c) if the dependent source is installed in the circuit upside down. (e) Are both circuit configurations “stable”? Explain.
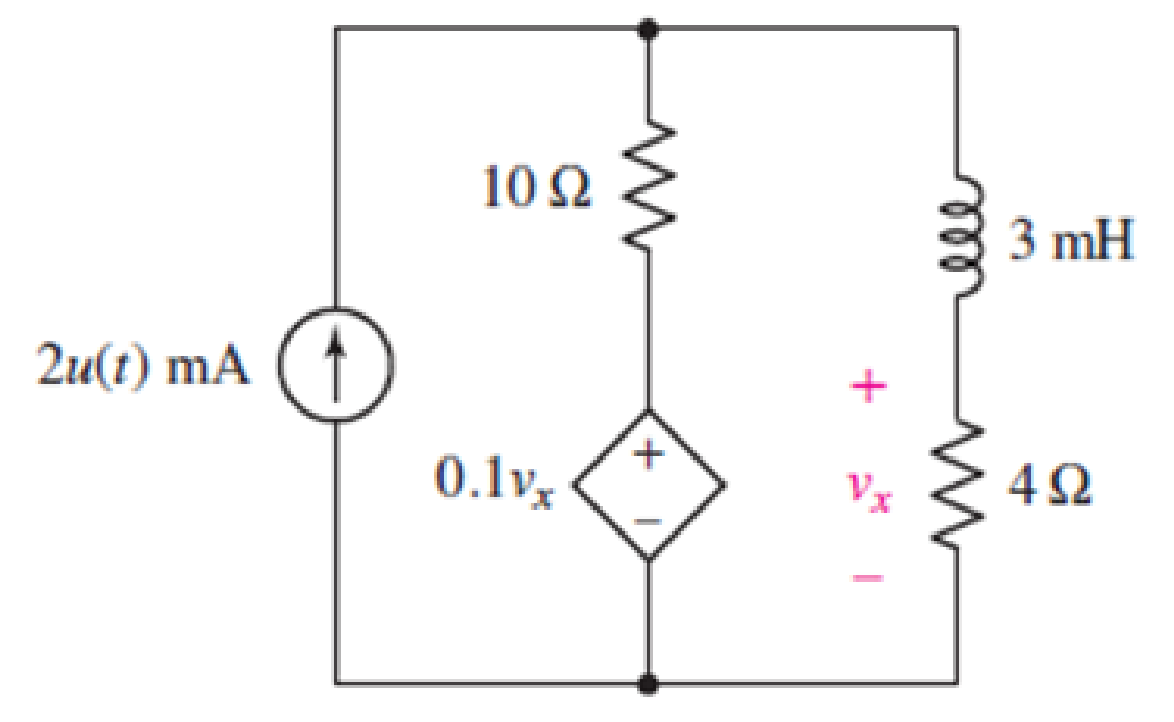
Figures 8.95
(a)
Find the circuit time constant.
Answer to Problem 76E
The time constant of the circuit is
Explanation of Solution
Formula used:
The expression for the resistance of the circuit is as follows:
Here,
The expression for the time constant of circuit is as follows:
Here,
Calculation:
To find equivalent resistance of a circuit the independent current source is replaced by open circuit and
The circuit diagram is redrawn as shown in Figure 1.
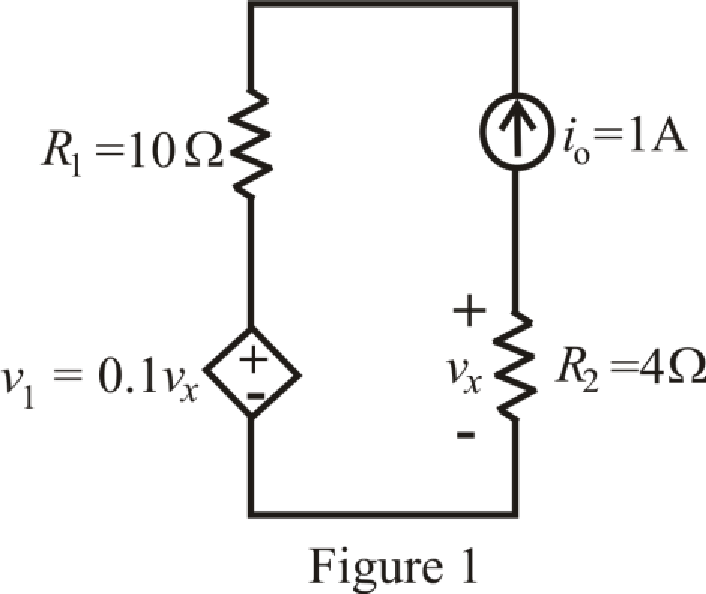
Refer to the redrawn Figure 1:
Apply KVL in mesh 1:
Here,
Substitute
The expression for voltage across the
Here,
Substitute
Substitute
Rearrange for
Substitute
So, the equivalent resistance across inductor is
Substitute
So the time constant of the circuit is
Conclusion:
Thus, the time constant of the circuit is
(b)
Obtain an expression for
Answer to Problem 76E
The expression for the voltage
Explanation of Solution
Formula used:
The expression for the final response of the circuit valid for all
Here,
Calculation:
The unit-step forcing function as a function of time which is zero for all values of its argument less than zero and which is unity for all positive values of its argument.
Here,
The independent current source is:
Substitute
The current through
The
So, the value of the current flowing through the inductor for
The inductor does not allow sudden change in the current.
So,
Therefore, the current flowing in the circuit for
Substitute
So, the current flowing through the
The circuit diagram is redrawn as shown in Figure 2 for
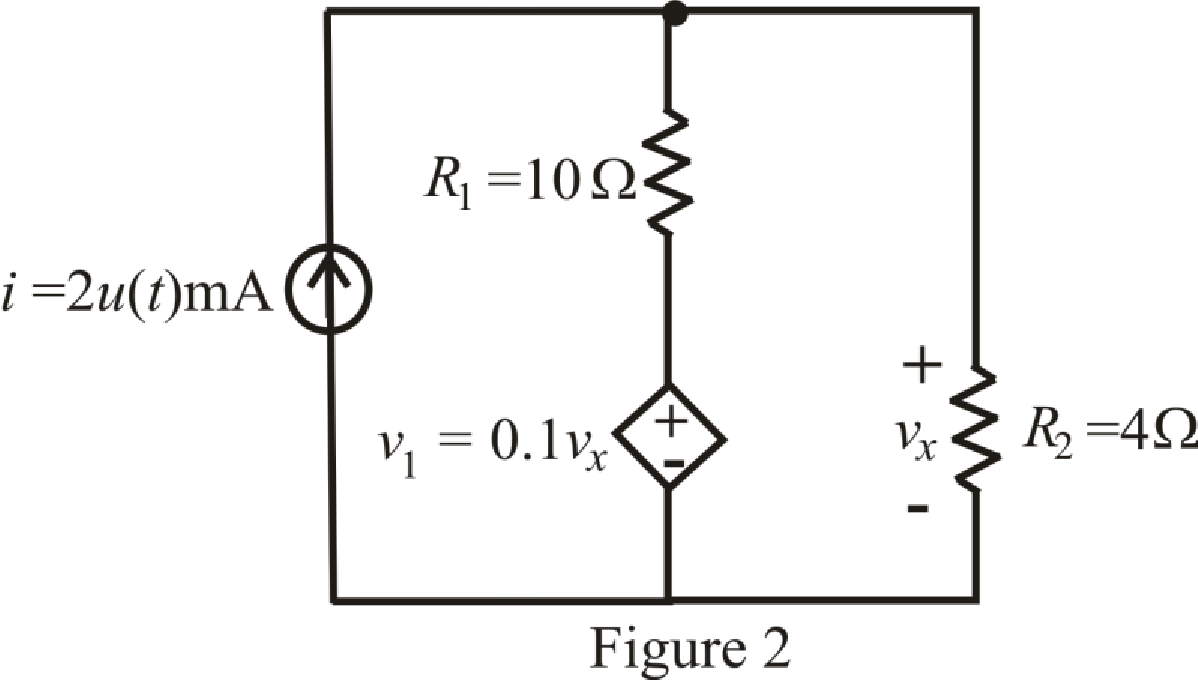
Refer to the redrawn Figure 2:
Apply KCL in the circuit:
Substitute
Rearrange for
The expression for the current flowing through
Here,
Substitute
So, the current flowing through
Substitute
The expression for the voltage across the
Substitute
Conclusion:
Thus, the expression for the voltage
(c)
Plot the power dissipated in the
Explanation of Solution
Given data:
The range of the time is six time constant.
Formula used:
The expression for the power dissipated in the
Here,
Calculation:
Substitute
The time constant of the circuit is
The different value for the power dissipated in the
The graph for power dissipated in the
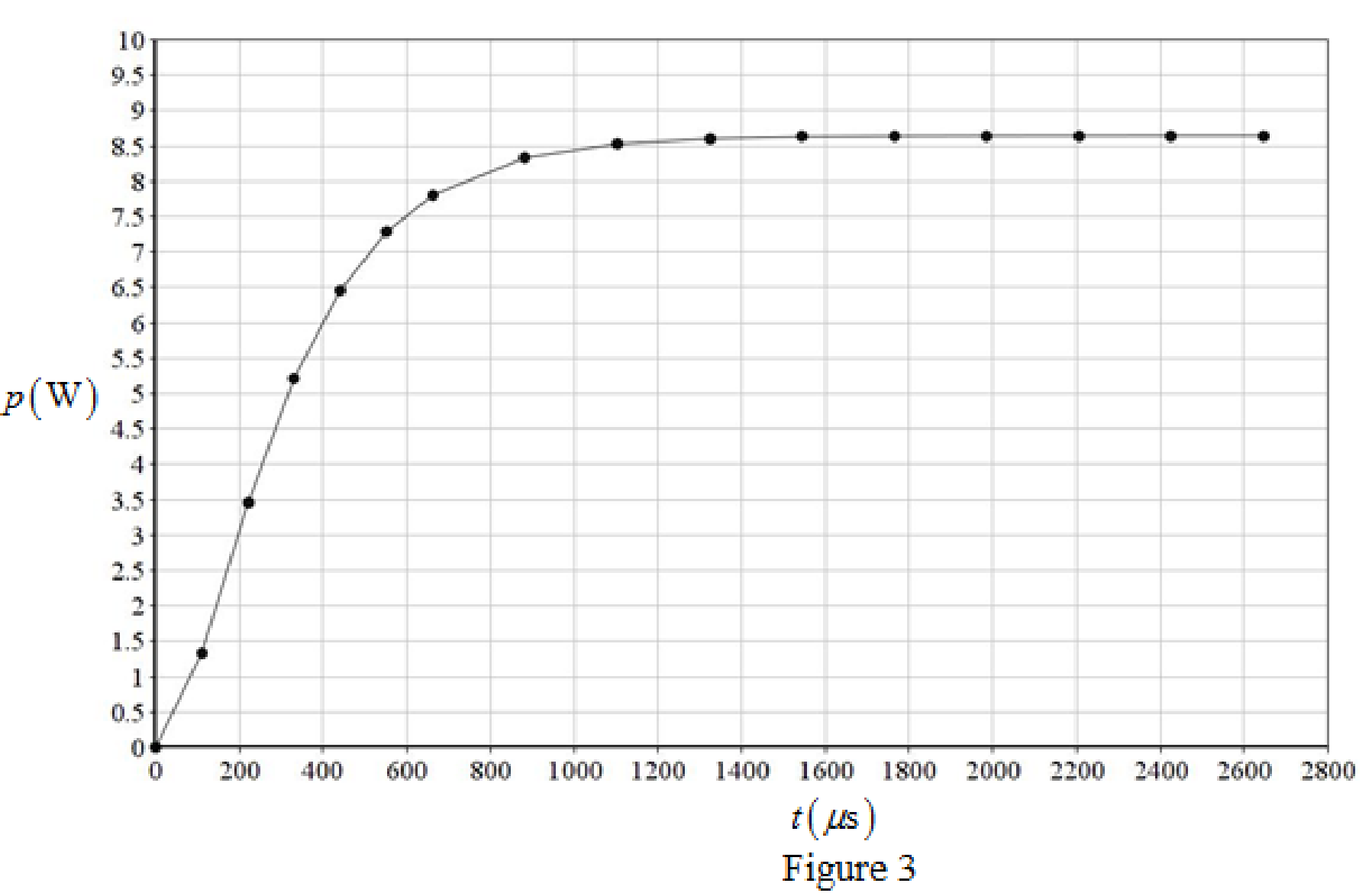
Calculation:
Thus, the graph for power dissipated in the
Conclusion:
(d)
Repeat parts (a) to (c) if the dependent source is installed in the circuit upside down.
Explanation of Solution
Calculation:
To find equivalent resistance of a circuit the independent current source is replaced by open circuit and
The circuit diagram is redrawn as shown in Figure 4:
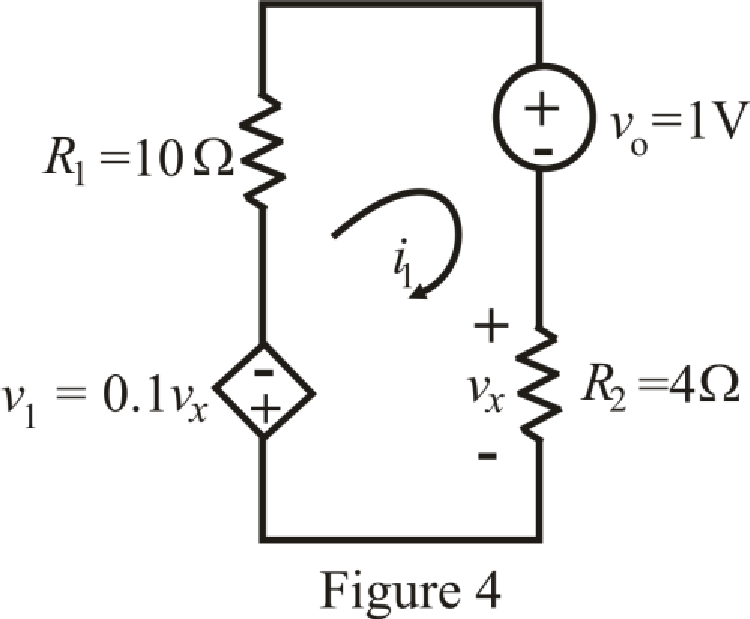
Refer to the redrawn Figure 4:
Apply KVL in mesh 1:
Here,
Substitute
The expression for voltage across the
Here,
Substitute
Substitute
Rearrange for
Substitute
So, the equivalent resistance across inductor is
Substitute
So, the time constant of the circuit is
The unit-step forcing function as a function of time which is zero for all values of its argument less than zero and which is unity for all positive values of its argument.
Here,
The independent voltage source is:
Substitute
The current through
The
So, the value of the current flowing through the inductor for
The inductor does not allow sudden change in the current.
So,
Therefore, the current flowing in the circuit for
Substitute
So, the current flowing through the
The circuit diagram is redrawn as shown in Figure 5 for
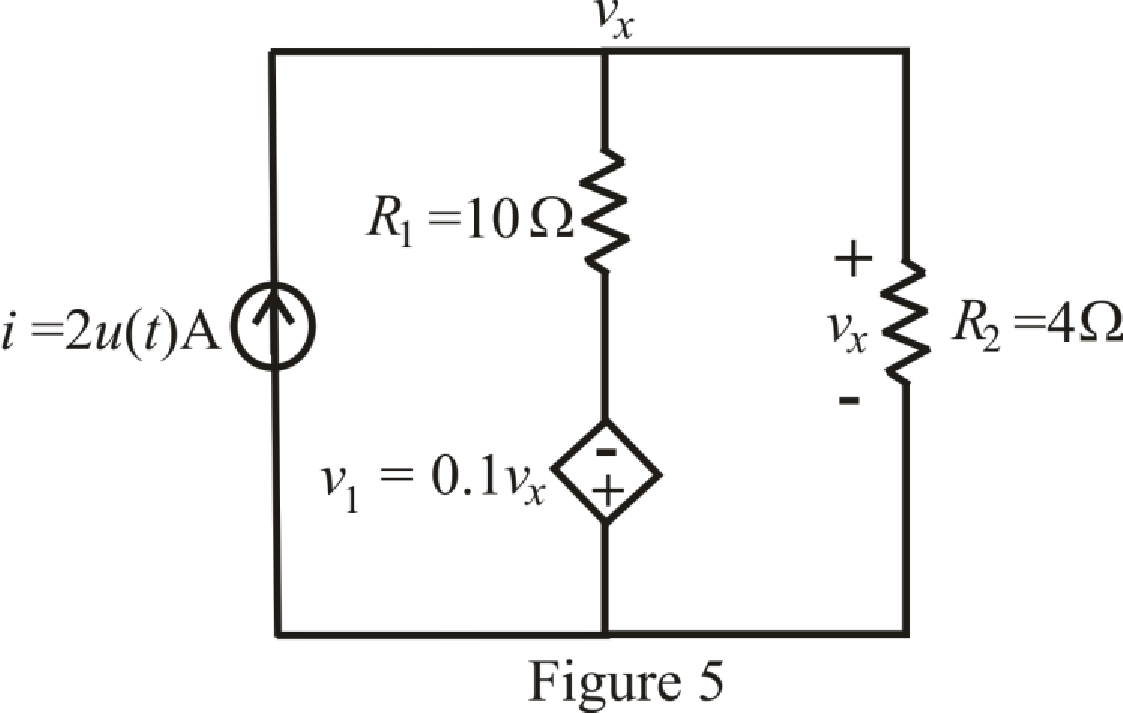
Refer to the redrawn Figure 5:
Apply KCL in the circuit:
Substitute
Rearrange for
The expression for the current flowing through
Here,
Substitute
So, the current flowing through
Substitute
The expression for the voltage across the
Substitute
So, the expression for the voltage
Substitute
The time constant of the circuit is
The different value for the power dissipated in the
The graph for power dissipated in the
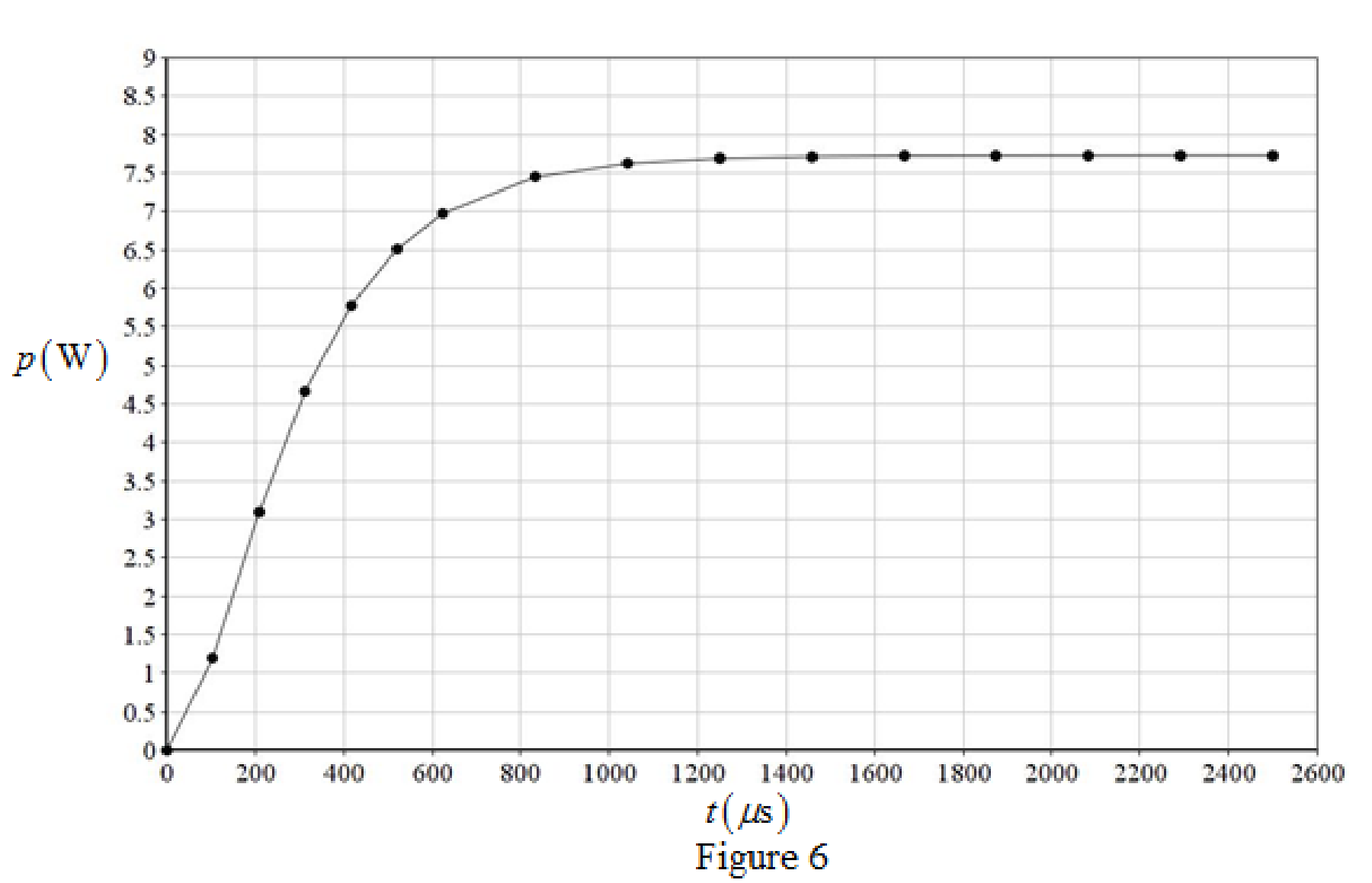
Conclusion:
Thus, the time constant of the circuit is
(e)
Are both circuit configurations “stable”? Explain.
Answer to Problem 76E
Both circuit configurations are “stable”.
Explanation of Solution
Refer to Figure 3 and Figure 6:
The response (output power dissipated in the
So, both circuit configurations “stable”.
Conclusion:
Thus, both circuit configurations are “stable”.
Want to see more full solutions like this?
Chapter 8 Solutions
Loose Leaf for Engineering Circuit Analysis Format: Loose-leaf
Additional Engineering Textbook Solutions
Thinking Like an Engineer: An Active Learning Approach (4th Edition)
Fluid Mechanics: Fundamentals and Applications
INTERNATIONAL EDITION---Engineering Mechanics: Statics, 14th edition (SI unit)
Vector Mechanics for Engineers: Statics and Dynamics
Elementary Surveying: An Introduction To Geomatics (15th Edition)
Starting Out with Programming Logic and Design (5th Edition) (What's New in Computer Science)
- They are one quearrow_forwardO Draw the four possible negative feedback contigurations of an op-amp. Write the input and output impedances of these configurations in ideal cases. 5arrow_forwardE9.6 Determine the average power absorbed by the 4-2 and 3-2 resistors in Fig. E9.6. 302 j20 Figure E9.3 4Ω ww Figure E9.6 12/0° V j30 -j2 N 13/10° A (+60° V (OEFarrow_forward
- -160 For the P-channel JFET given in the following figure, the IDSS = 2MA a) Determine IDQ and VSDQ b) Determine the source-follower circuit transistor parameters are: Vp = +1,75 V, and λ=0. Small-signal voltage gain, Av = So VDD = 10V R₁ = 90kr Rs =5k CC1 WW R₂ = 110kn 50 C02 BL = 10 kr GNDarrow_forwardNeed a solarrow_forwardI need a drawing on how to connect the function generator, oscilliscope, and both multimeters. It is hard for me to follow text instructions. The function generator has a postive,common and negative. The oscilliscope has chanell A and B, both channels have a postive and a negative. I know you can provide text instruction but a little sketch would be very helpful thank you.arrow_forward
- Don't use ai to answer I will report you answerarrow_forwardQ1/ A three phase, 500 kVA, 6600 V, 50 Hz, 6 pole, star connected synchronous motor has synchronous impedance of J 70 ohm per phase at its normal rating, the motor is excited to give unity power factor at the input terminals. Find a) The rated current and power factor. b) The emf behind the synchronous impedance. c) The developed torque. d) The pull out torque. e) The increase in excitation which will just permit an increase of 30% of rated torque before pulling out of synchronism. (45 M.)arrow_forwardcan you fin Vds and Vgs of all transistors and specify te operating region off all transistors and prove it. 58V 5.8 V 1.8V M2 0.9V 22222 と A 4852 m 3 01 A Voy = 0.2 V V4)=0.SV λ=0.1 V-1arrow_forward
 Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON
Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning
Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education
Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education
Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON
Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,
Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,





