Chapter 8, Problem 28E

(a)

To determine

Find the value of $i_L$, $v_L$ and $v_R$ at $t=0^{-}$.

Answer to Problem 28E

At $t=0^{-}$ the value of $i_L$ is $\text{240 μA}$, $v_L$ is $0\text{ V}$ and $v_R$ is $4\text{80 mV}$.

Explanation of Solution

Formula used:

The expression for the current flowing through the resistor is as follows.

$i_L=\frac{v_1}{R_3}$ (1)

Here,

$i_L$ is the current flowing through the resistor,

$v_1$ is the voltage and

$R_3$ is the resistance.

Calculation:

The redrawn circuit diagram is given in Figure 1.

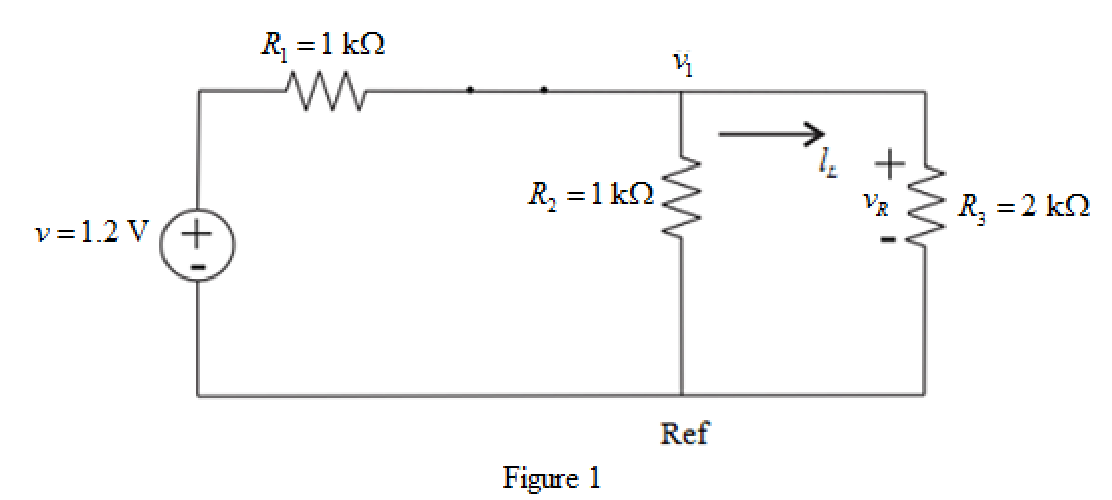
Refer to the redrawn Figure 1.

The given circuit inductor is connected for 6 years prior to being flipped open at $t=0$. So, it is connected for long time and inductor behaves as short circuit. Hence, the voltage across the inductor is $0\text{ V}$.

Apply KCL at node 1.

$\frac{v_1-v}{R_1}+\frac{v_1}{R_2}+\frac{v_1}{R_3}=0\text{ A}$ (2)

Here,

$R_1$ is the resistance of the $\text{1 k}\Omega$ resistor and

$R_2$ is the resistance of the $2\text{ k}\Omega$ resistor.

$R_3$ is the resistance of the $2\text{ k}\Omega$ resistor.

Substitute $1.2\text{ V}$ for $v$, $1\text{ k}\Omega$ for $R_1$, $1\text{ k}\Omega$ for $R_2$ and $2\text{ k}\Omega$ for $R_3$ in equation (2).

$\begin{array}{l}\frac{v_1-\left(1.2\text{ V}\right)}{1\text{ k}\Omega }+\frac{v_1}{1\text{ k}\Omega }+\frac{v_1}{\text{2 k}\Omega }=0\text{ A}\\ \frac{v_1-\left(1.2\text{ V}\right)}{1\times {10}^3\Omega }+\frac{v_1}{1\times {10}^3\Omega }+\frac{v_1}{\text{2}\times {10}^3\Omega }=0\text{ A }\left\{\because 1\text{ k}\Omega ={10}^3\Omega \right\}\\ \frac{2v_1-\left(\text{2}\text{.4 V}\right)+2v_1+v_1}{\text{2}\times {10}^3\Omega }=0\text{ A }\\ 5v_1-\left(\text{2}\text{.4 V}\right)=0\text{ A}\end{array}$

Rearrange the above equation for $v_1$.

$\begin{array}{l}5v_1=\text{2}\text{.4 V}\\ v_1=0.48\text{ V}\end{array}$

The voltage across $2\text{ k}\Omega$ resistor $v_R$ is equal to $v_1$.

$\begin{array}{c}{v}_R=0.48\text{ V}\\ =\text{480}\times {\text{10}}^{-3}\text{ V}\\ =4\text{80 mV }\left\{\because 1\text{ mV}={10}^{-3}\text{ V}\right\}\end{array}$

Substitute $0.48\text{ V}$ for $v_1$ and $2\text{ k}\Omega$ for $R_3$ in the equation (1).

$\begin{array}{c}{i}_L=\frac{0.48\text{ V}}{2\text{ k}\Omega }\\ =\frac{0.48\text{ V}}{2\times {10}^3\Omega }\left\{\because 1\text{ k}\Omega ={10}^3\Omega \right\}\\ =0.24\times {10}^{-3}\text{ A}\\ =\text{240}\times {10}^{-6}\text{ A }\end{array}$

$i_L=\text{240 }\mu \text{A }\left\{\because 1\mu \text{A}={10}^{-6}\text{ A}\right\}$

Conclusion:

Thus, at $t=0^{-}$ the value of $i_L$ is $\text{240 }\mu \text{A}$, $v_L$ is $0\text{ V}$ and $v_R$ is $4\text{80 mV}$.

(b)

To determine

Find the value of $i_L$, $v_L$ and $v_R$ at $t$ equal to $0^{+}$.

Answer to Problem 28E

At $t=0^{+}$ the value of $i_L$ is $\text{240 }\mu \text{A}$, $v_L$ is $-0.72\text{ V}$ and $v_R$ is $4\text{80 mV}$.

Explanation of Solution

Calculation:

The redrawn circuit diagram is given in Figure 2.

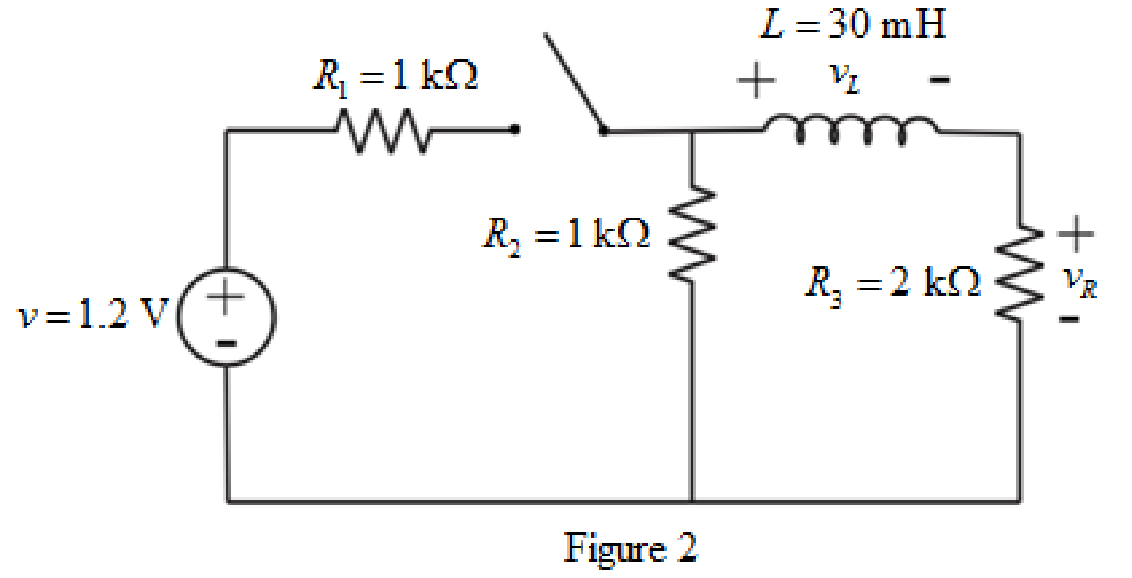
Refer to the redrawn Figure 2.

The expression for the voltage across the $2\text{ k}\Omega$ resistor is as follows.

$v_R=i_L{R}_3$ (3)

Here,

$v_R$ is the voltage across the $2\text{ k}\Omega$ resistor,

$R_3$ is the resistance of the $2\text{ k}\Omega$ resistor and

$i_L$ is the current flowing through the $2\text{ k}\Omega$ resistor.

Refer to the redrawn Figure 2.

The inductor does not allow sudden change in the current.

$i_L\left(0^{+}\right)=i_L\left(0^{-}\right)$

So, the current through inductor at $t=0^{+}$ is $\text{240 }\mu \text{A}$.

Apply KVL in the right side mesh.

$v_L+i_L{R}_2+i_L{R}_3=0\text{ V}$ (4)

Here,

$R_1$ is the resistance of the $1\text{ k}\Omega$ resistor and

$R_2$ is the resistance of the $1\text{ k}\Omega$ resistor.

Substitute $1\text{ k}\Omega$ for $R_2$ and $2\text{ k}\Omega$ for $R_3$ and $\text{240 }\mu \text{A}$ for $i_L$ in equation (4).

$\begin{array}{l}{v}_L+\left(\text{240 }\mu \text{A}\right)\left(1\text{ k}\Omega \right)+\left(\text{240 }\mu \text{A}\right)\left(\text{2 k}\Omega \right)=0\text{ V}\\ v_L+\left(\text{240 }\mu \text{A}\right)\left(1\times {10}^3\Omega \right)+\left(\text{240 }\mu \text{A}\right)\left(\text{2}\times {10}^3\Omega \right)=0\text{ V }\left\{\because 1\text{ k}\Omega ={10}^3\Omega \right\}\\ v_L+\left(\text{240}\times {10}^{-6}\text{ A}\right)\left(1\times {10}^3\Omega \right)+\left(\text{240}\times {10}^{-6}\text{ A}\right)\left(\text{2}\times {10}^3\Omega \right)=0\text{ V }\left\{\because 1\mu \text{A}={10}^{-6}\text{ A}\right\}\\ v_L+0.240\text{ V}+\text{0}.480\text{ V}=0\text{ V}\end{array}$

Rearrange for $v_L$.

$\begin{array}{c}{v}_L=-\left(0.240\text{ V}+\text{0}.480\text{ V}\right)\\ =-0.72\text{ V}\end{array}$

Substitute $2\text{ k}\Omega$ for $R_3$ and $\text{240 }\mu \text{A}$ for $i_L$ in the equation (3).

$\begin{array}{c}{v}_R=\left(\text{240 }\mu \text{A}\right)\left(2\text{ k}\Omega \right)\\ =\left(\text{240 }\mu \text{A}\right)\left(2\times {10}^3\Omega \right)\left\{\because 1\text{ k}\Omega ={10}^3\Omega \right\}\\ =\left(\text{240}\times {10}^{-6}\text{ A}\right)\left(2\times {10}^3\Omega \right)\left\{\because 1\mu \text{A}={10}^{-6}\text{ A}\right\}\\ =480\times {10}^{-3}\text{ V}\end{array}$

$v_R=480\text{ mV }\left\{\because 1\text{ mA}={10}^{-3}\text{ A}\right\}$

Conclusion:

Thus, at $t=0^{+}$ the value of $i_L$ is $\text{240 }\mu \text{A}$, $v_L$ is $-0.72\text{ V}$ and $v_R$ is $4\text{80 mV}$.

(c)

To determine

Find the value of $i_L$, $v_L$ and $v_R$ at $t$ equal to $1\mu \text{s}$.

Answer to Problem 28E

At $t=1\mu \text{s}$ the value of $i_L$ is $217.16\text{}\mu \text{A}$, $v_L$ is $\text{651}\text{.48 mV}$ and $v_R$ is $434.3\text{ mV }$.

Explanation of Solution

Given data:

The time is $1\mu \text{s}$.

Formula used:

The expression for the equivalent resistor connected in series is as follows.

$R_{eq}=R_1+R_2+R_3\mathrm{.....}+R_N$ (5)

Here,

$R_{eq}$ is the equivalent resistance between two points.

$R_1$, $R_2$, $R_3$…, $R_N$ are the resistances.

The expression for the time constant for $RL$ the circuit is as follows.

$\tau =\frac{L}{R_{eq}}$ (6)

Here,

$\tau$ is the time constant,

$R_{eq}$ is the equivalent resistance across the inductor and

$L$ is the inductance.

The expression for the current for $t>0$ is as follows.

$i_L\left(t\right)=i_L\left(0\right)e^{\frac{-t}{\tau }}$ (7)

Here,

$i_L\left(t\right)$ is the current  flowing through the inductor for $t>0$,

$i_L\left(0\right)$ is the current  flowing through the inductor at $t<0$ and

$t$ is the given time.

Calculation:

The redrawn circuit diagram is given in Figure 3.

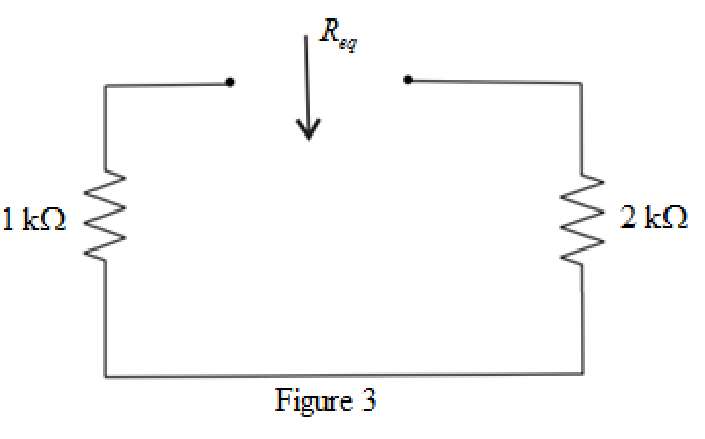
Refer to the redrawn Figure 3.

$2\text{ k}\Omega$ and $1\text{ k}\Omega$ resistors are connected in series. So, the equivalent resistance is,

$\begin{array}{c}{R}_{eq}=2\text{ k}\Omega +1\text{ k}\Omega \\ =3\text{ k}\Omega \end{array}$

The simplified diagram is shown in Figure 4.

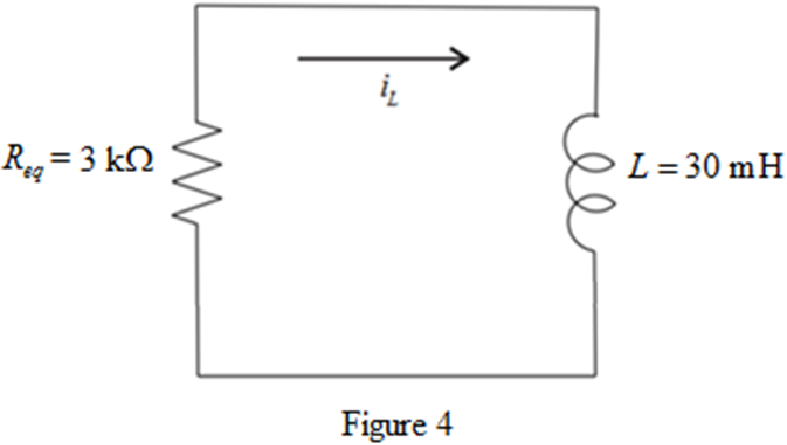
Refer to the redrawn Figure 4.

Substitute $3\text{ k}\Omega$ for $R_{eq}$ and $30\text{ mH}$ for $L$ in the equation (6).

$\begin{array}{c}\tau =\frac{30\text{ mH}}{3\text{ k}\Omega }\\ =\frac{30\times {10}^{-3}\text{ H}}{3\text{ k}\Omega }\left\{\because 1\text{ mH}={10}^{-3}\text{ H}\right\}\\ =\frac{30\times {10}^{-3}\text{ H}}{3\times {10}^3\Omega }\left\{\because 1\text{ k}\Omega ={10}^3\Omega \right\}\\ =10\times {10}^{-6}\text{ s}\end{array}$

$\tau =10\mu \text{s }\left\{\because 1\mu \text{s}={10}^{-6}\text{ s}\right\}$

Substitute $10\mu \text{s}$ for $\tau$ and $\text{240 μA}$ for $i_L\left(0\right)$ and $1\mu \text{s}$ for $t$ in the equation (7).

$\begin{array}{c}{i}_L\left(10\mu \text{s}\right)=\left(\text{240 }\mu \text{A}\right)e^{\frac{-1\mu \text{s}}{10\mu \text{s}}}\\ =\left(\text{240 }\mu \text{A}\right)\left(0.9048\right)\\ =217.16\text{}\mu \text{A}\end{array}$

Substitute $1\text{ k}\Omega$ for $R_2$ and $2\text{ k}\Omega$ for $R_3$ and $217.16\text{}\text{ μA}$ for $i_L$ in the equation (4).

$\begin{array}{l}{v}_L+\left(217.16\text{}\mu \text{A}\right)\left(1\text{ k}\Omega \right)+\left(217.16\text{}\mu \text{A}\right)\left(\text{2 k}\Omega \right)=0\text{ V}\\ v_L+\left(217.16\text{}\mu \text{A}\right)\left(1\times {10}^3\Omega \right)+\left(217.16\text{}\mu \text{A}\right)\left(\text{2}\times {10}^3\Omega \right)=0\text{ V }\left\{\because 1\text{ k}\Omega ={10}^3\Omega \right\}\\ v_L+\left(217.16\times {10}^{-6}\text{ A}\right)\left(1\times {10}^3\Omega \right)+\left(217.16\times {10}^{-6}\text{ A}\right)\left(\text{2}\times {10}^3\Omega \right)=0\text{ V }\left\{\because 1\mu \text{A}={10}^{-6}\text{ A}\right\}\\ v_L+0.21716\text{ V}+\mathrm{0.434.32}\text{ V}=0\text{ V}\end{array}$

Rearrange the above equation for $v_L$.

$\begin{array}{c}{v}_L=-\left(0.21716\text{ V}+\mathrm{0.434.32}\text{ V}\right)\\ =-0.65148\text{ V}\\ =-\text{651}\text{.48 mV }\left\{\because 1\text{ A}={10}^3\text{ mA}\right\}\end{array}$

Substitute $2\text{ k}\Omega$ for $R_3$ and $217.16\text{}\text{ μA}$ for $i_L$ in the equation (3).

$\begin{array}{c}{v}_R=\left(217.16\text{}\mu \text{A}\right)\left(2\text{ k}\Omega \right)\\ =\left(217.16\text{}\mu \text{A}\right)\left(2\times {10}^3\Omega \right)\left\{\because 1\text{ k}\Omega ={10}^3\Omega \right\}\\ =\left(217.16\times {10}^{-6}\text{ A}\right)\left(2\times {10}^3\Omega \right)\left\{\because 1\mu \text{A}={10}^{-6}\text{ A}\right\}\\ =0.4343\text{ V}\end{array}$

$v_R=434.3\text{ mV }\left\{\because 1\text{ A}={10}^3\text{ mA}\right\}$

Conclusion:

Thus, at $t=1\mu \text{s}$ the value of $i_L$ is $217.16\text{}\mu \text{A}$, $v_L$ is $\text{651}\text{.48 mV}$ and $v_R$ is $434.3\text{ mV }$.

(d)

To determine

Find the value of $i_L$, $v_L$ and $v_R$ at $t$ equal to $10\mu \text{s}$.

Answer to Problem 28E

At $10\mu \text{s}$ the value of $i_L$ is $88.291\text{}\mu \text{A}$, $v_L$ is $-\text{264}\text{.9 mV}$ and $v_R$ is $176.6\text{ mV}$.

Explanation of Solution

Given Data:

The time is $10\mu \text{s}$.

Calculation:

Substitute $10\mu \text{s}$ for $\tau$ and $\text{240 }\mu \text{A}$ for $i_L\left(0\right)$ and $10\mu \text{s}$ for $t$ in the equation (7).

$\begin{array}{c}{i}_L\left(10\mu \text{s}\right)=\left(\text{240 }\mu \text{A}\right)e^{\frac{-10\mu \text{s}}{10\mu \text{s}}}\\ =\left(\text{240 }\mu \text{A}\right)\left(0.36788\right)\\ =88.291\text{}\mu \text{A}\end{array}$

Substitute $1\text{ k}\Omega$ for $R_2$ and $2\text{ k}\Omega$ for $R_3$ and $88.291\text{}\text{ μA}$ for $i_L$ in the equation (4).

$\begin{array}{l}{v}_L+\left(88.291\text{}\mu \text{A}\right)\left(1\text{ k}\Omega \right)+\left(88.291\text{}\mu \text{A}\right)\left(\text{2 k}\Omega \right)=0\text{ V}\\ v_L+\left(88.291\text{}\mu \text{A}\right)\left(1\times {10}^3\Omega \right)+\left(88.291\text{}\mu \text{A}\right)\left(\text{2}\times {10}^3\Omega \right)=0\text{ V }\left\{\because 1\text{ k}\Omega ={10}^3\Omega \right\}\\ v_L+\left(88.291\times {10}^{-6}\text{ A}\right)\left(1\times {10}^3\Omega \right)+\left(88.291\times {10}^{-6}\text{ A}\right)\left(\text{2}\times {10}^3\Omega \right)=0\text{ V }\left\{\because 1\mu \text{A}={10}^{-6}\text{ A}\right\}\\ v_L+0.0883\text{ V}+0.1766\text{ V}=0\text{ V}\end{array}$

Rearrange the above equation for $v_L$.

$\begin{array}{c}{v}_L=-\left(0.0883\text{ V}+0.1766\text{ V}\right)\\ =-0.2649\text{ V}\\ =-\text{264}\text{.9 mV }\left\{\because 1\text{ A}={10}^3\text{ mA}\right\}\end{array}$

Substitute $2\text{ k}\Omega$ for $R_3$ and $88.291\text{}\mu \text{A}$ for $i_L$ in the equation (3).

$\begin{array}{c}{v}_R=\left(88.291\text{}\mu \text{A}\right)\left(2\text{ k}\Omega \right)\\ =\left(88.291\text{}\mu \text{A}\right)\left(2\times {10}^3\Omega \right)\left\{\because 1\text{ k}\Omega ={10}^3\Omega \right\}\\ =\left(88.291\times {10}^{-6}\text{ A}\right)\left(2\times {10}^3\Omega \right)\left\{\because 1\mu \text{A}={10}^{-6}\text{ A}\right\}\\ =0.1766\text{ V}\end{array}$

$=176.6\text{ mV }\left\{\because 1\text{ A}={10}^3\text{ mA}\right\}$

Conclusion:

Thus, at $10\mu \text{s}$ the value of $i_L$ is $88.291\text{}\mu \text{A}$, $v_L$ is $-\text{264}\text{.9 mV}$ and $v_R$ is $176.6\text{ mV}$.