Chapter 8, Problem 39P

To determine

Draw the influence lines for the vertical reactions at supports A, B, C and the shear and bending moment at point E.

Explanation of Solution

Calculation:

Apply a 1 kN unit moving load at a distance of x from left end D.

Sketch the free body diagram of frame as shown in Figure 1.

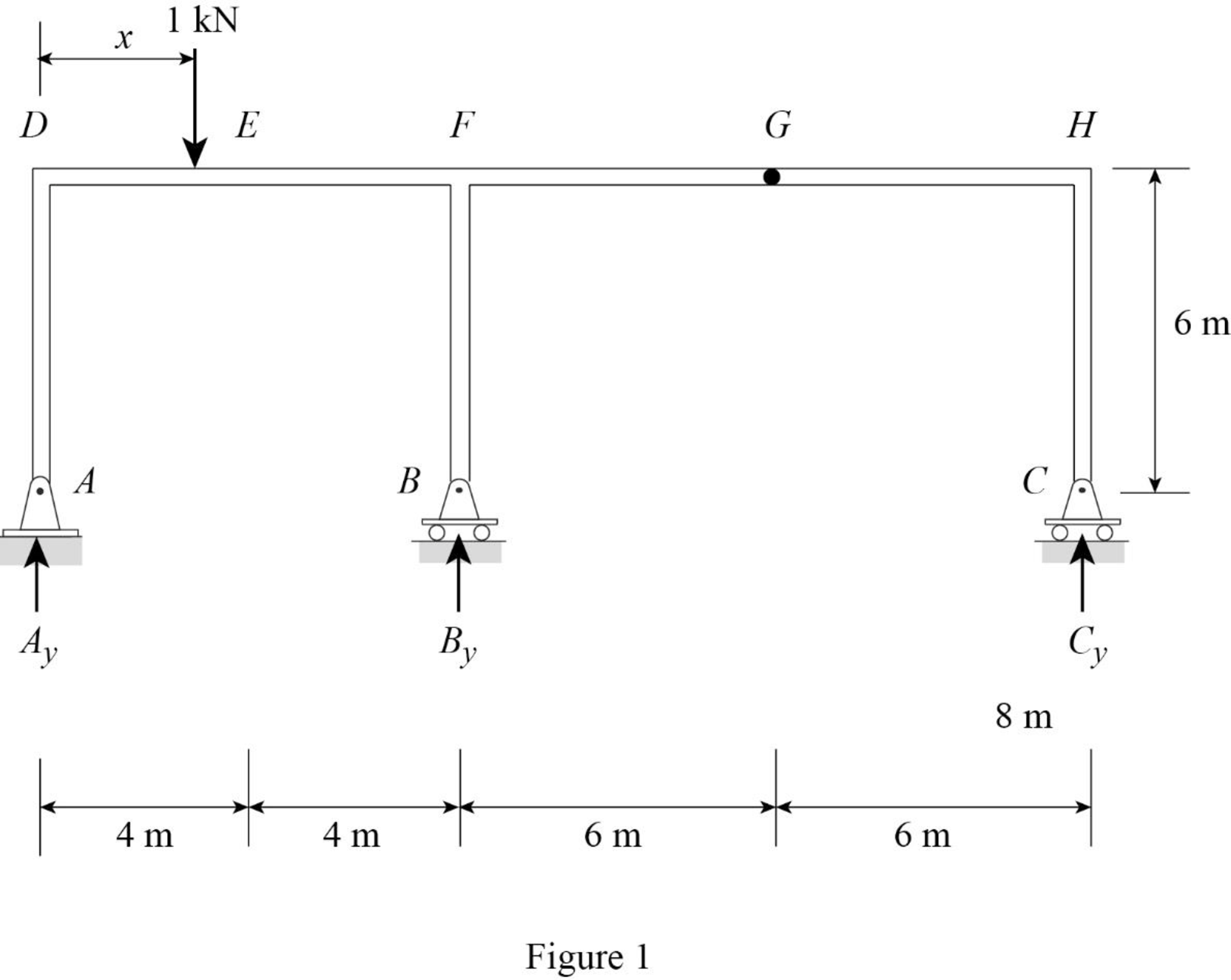
Influence line for vertical reaction at supports C.

Refer Figure 1.

Find the equation of vertical reaction at supports C.

Apply 1 kN load just left of G $\left(0\le x\le 14\text{m}\right)$.

Consider section GH.

Take moment at G from C.

Consider clockwise moment as positive and anticlockwise moment as negative.

$\begin{array}{l}\Sigma M_G=0\\ -C_y\left(6\right)=0\\ C_y=0\end{array}$

Apply 1 kN load just right of G $\left(14\text{m}\le x\le 20\text{m}\right)$.

Consider section GH.

Take moment at G from C.

Consider clockwise moment as positive and anticlockwise moment as negative.

$\begin{array}{l}\Sigma M_G=0\\ -C_y\left(6\right)+1\left(x-14\right)=0\\ 6C_y=x-14\\ C_y=\frac{x}{6}-\frac{7}{3}\end{array}$

Thus, the equation of vertical reaction at supports C as follows,

$C_y=0,\left(0\le x\le 14\text{m}\right)$        (1)

$C_y=\frac{x}{6}-\frac{7}{3},\left(14\text{m}\le x\le \text{20}\text{m}\right)$        (2)

Find the influence line ordinate of $C_y$ at H using Equation (2).

Substitute 20 m for $x$ in Equation (2).

$\begin{array}{c}{C}_y=\frac{20}{6}-\frac{7}{3}\\ =\frac{10}{3}-\frac{7}{3}\\ =\frac{3}{3}\\ =1\text{kN}\end{array}$

Thus, the influence line ordinate of $C_y$ at H is $1\text{kN/kN}$.

Similarly calculate the influence line ordinate of $C_y$ at various points on the frame and summarize the values in Table 1.

x (m)	Points	Influence line ordinate of $C_y$$\left(\text{kN/kN}\right)$
0	D	0
4	E	0
8	F	0
14	G	0
20	H	1

Sketch the influence line diagram for vertical reaction at supports C using Table 1 as shown in Figure 2.

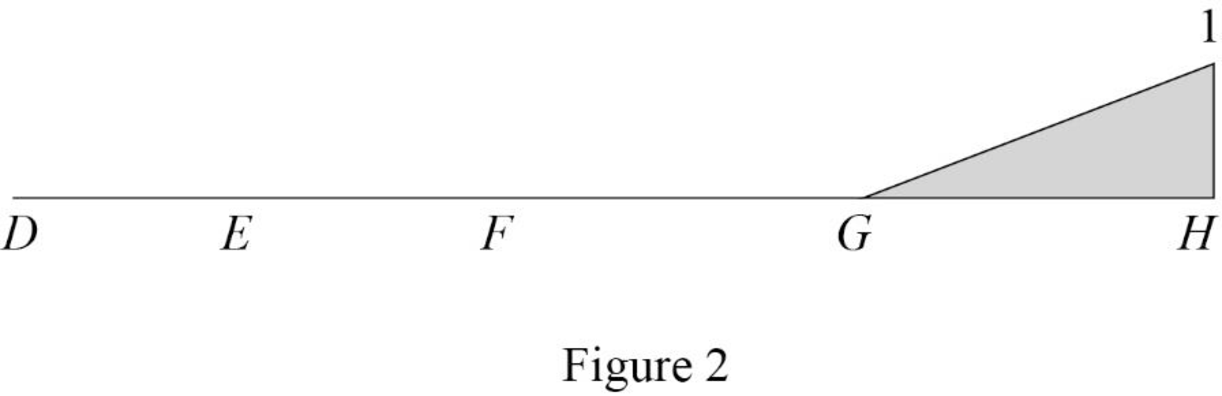
Influence line for vertical reaction at support A.

Apply 1 kN load just left of F $\left(0\le x\le 8\text{m}\right)$.

Refer Figure 1.

Find the equation of vertical reaction at supports C.

Consider section DF.

Take moment at B from A.

Consider clockwise moment as positive and anticlockwise moment as negative.

$\begin{array}{l}\Sigma M_B=0\\ A_y\left(8\right)-1\left(8-x\right)=0\\ 8A_y=8-x\\ A_y=1-\frac{x}{8}\end{array}$

Apply 1 kN load just right of F.

Consider section FH.

Consider moment at B from A is equal to from C.

Consider clockwise moment as positive and anticlockwise moment as negative.

$\begin{array}{l}\Sigma M_B=0\\ A_y\left(8\right)=C_y\left(12\right)-1\left(x-8\right)\\ 8A_y=12C_y-x+8\end{array}$        (3)

Find the equation of vertical reaction at A from F to G $\left(8\text{m}\le x\le 14\text{m}\right)$.

Substitute 0 for $C_y$ in Equation (3).

$\begin{array}{l}8A_y=12\left(0\right)-x+8\\ 8A_y=8-x\\ A_y=1-\frac{x}{8}\end{array}$

Find the equation of vertical reaction at A from G to H $\left(14\text{m}\le x\le 20\text{m}\right)$.

Substitute $\frac{x}{6}-\frac{7}{3}$ for $C_y$ in Equation (3).

$\begin{array}{c}8A_y=12\left(\frac{x}{6}-\frac{7}{3}\right)-x+8\\ =2x-28-x+8\\ =x-20\\ A_y=\frac{x}{8}-\frac{5}{2}\end{array}$

Thus, the equation of vertical reaction at supports A as follows,

$A_y=1-\frac{x}{8},\left(0\le x\le \text{14}\text{m}\right)$        (4)

$A_y=\frac{x}{8}-\frac{5}{2},\left(14\text{m}\le x\le \text{20}\text{m}\right)$        (5)

Find the influence line ordinate of $A_y$ at G using Equation (4).

Substitute 14 m for $x$ in Equation (4).

$\begin{array}{c}{A}_y=1-\frac{14}{8}\\ =-0.75\text{kN}\end{array}$

Thus, the influence line ordinate of $A_y$ at G is $-0.75\text{kN/kN}$.

Similarly calculate the influence line ordinate of $A_y$ at various points on the frame and summarize the values in Table 2.

x (m)	Points	Influence line ordinate of $A_y$$\left(\text{kN/kN}\right)$
0	D	1
4	E	0.5
8	F	0
14	G	$-0.75$
20	H	0

Sketch the influence line diagram for the vertical reaction at support A using Table 2 as shown in Figure 3.

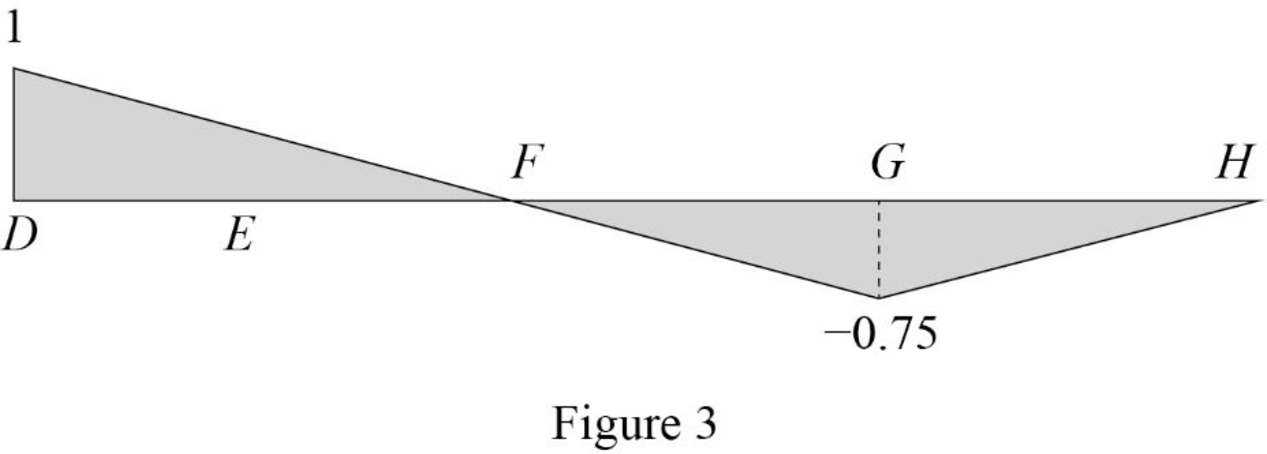
Influence line for vertical reaction at support B.

Apply a 1 kN unit moving load at a distance of x from left end C.

Refer Figure 1.

Apply vertical equilibrium in the system.

Consider upward force as positive and downward force as negative.

$\begin{array}{l}{A}_y+B_y+C_y-1=0\\ B_y=1-A_y-C_y\end{array}$        (6)

Find the equation of vertical support reaction $\left(B_y\right)$ from D to G $\left(0\le x\le 14\text{m}\right)$ using Equation (6).

Substitute $1-\frac{x}{8}$ for $A_y$ and 0 for $C_y$ in Equation (6).

$\begin{array}{c}{B}_y=1-\left(1-\frac{x}{8}\right)-0\\ =1-1+\frac{x}{8}\\ =\frac{x}{8}\end{array}$

Find the equation of vertical support reaction $\left(B_y\right)$ from G to H $\left(14\text{m}\le x\le 20\text{m}\right)$ using Equation (6).

Substitute $\frac{x}{8}-\frac{5}{2}$ for $A_y$ and $\frac{x}{6}-\frac{7}{3}$ for $C_y$ in Equation (6).

$\begin{array}{c}{B}_y=1-\left(\frac{x}{8}-\frac{5}{2}\right)-\left(\frac{x}{6}-\frac{7}{3}\right)\\ =1-\frac{x}{8}+\frac{5}{2}-\frac{x}{6}+\frac{7}{3}\\ =\frac{35}{6}-\frac{7x}{24}\end{array}$

Thus, the equation of vertical support reaction at B as follows,

$B_y=\frac{x}{8},\left(0\le x\le 14\text{m}\right)$        (7)

$B_y=\frac{35}{6}-\frac{7x}{24},\left(14\text{m}\le x\le \text{20}\text{m}\right)$        (8)

Find the influence line ordinate of $B_y$ at F using Equation (7).

Substitute 8 m for $x$ in Equation (7).

$\begin{array}{c}{B}_y=\frac{8}{8}\\ =1\text{kN}\end{array}$

Thus, the influence line ordinate of $B_y$ at F is $1\text{kN/kN}$.

Similarly calculate the influence line ordinate of $B_y$ at various points on the beam and summarize the values in Table 3.

x (m)	Points	Influence line ordinate of $B_y$$\left(\text{kN/kN}\right)$
0	D	0
4	E	0.5
8	F	1
14	G	1.75
20	H	0

Sketch the influence line diagram for the vertical reaction at support B using Table 3 as shown in Figure 4.

Influence line for shear at point E.

Find the equation of shear $\left(S_{E,L}\right)$ at just left of E.

Apply 1 kN just left of E.

Consider section DE.

Sketch the free body diagram of the section AD as shown in Figure 5.

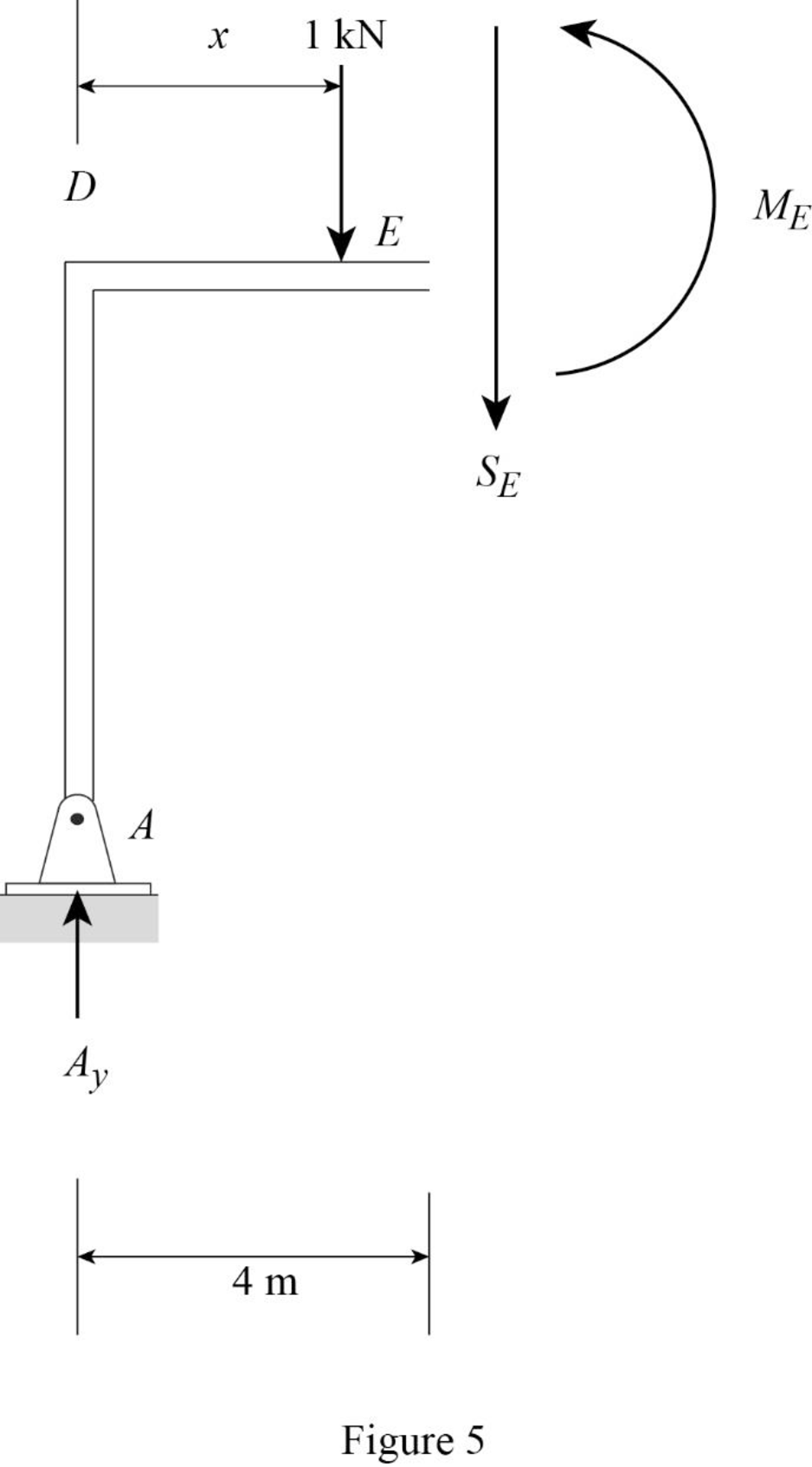
Refer Figure 5.

Apply equilibrium equation of forces.

Consider upward force as positive $\left(\uparrow +\right)$ and downward force as negative $\left(\downarrow -\right)$.

$\begin{array}{l}\Sigma F_y=0\\ A_y-S_{E,L}-1=0\\ S_{E,L}=A_y-1\end{array}$        (9)

Find the equation of shear force at E of portion DE $\left(0\le x\le 4\text{m}\right)$.

Substitute $1-\frac{x}{8}$ for $A_y$ in Equation (9).

$\begin{array}{c}{S}_{E,L}=\left(1-\frac{x}{8}\right)-1\\ =-\frac{x}{8}\end{array}$

Find the equation of shear $\left(S_{E,R}\right)$ at just right of E.

Apply 1 kN just right of E.

Consider section DE.

Sketch the free body diagram of the section DE as shown in Figure 6.

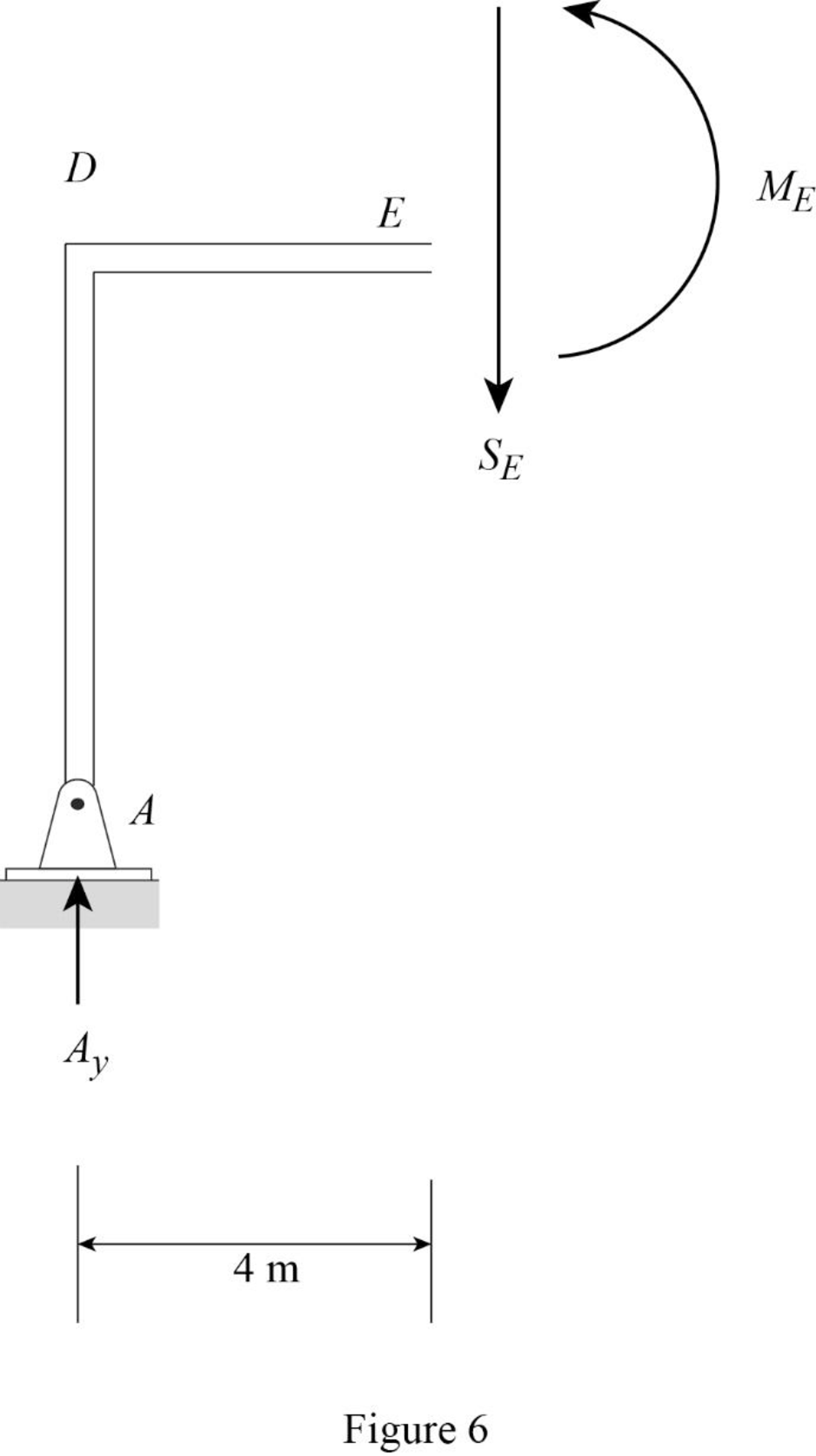
Refer Figure 6.

Apply equilibrium equation of forces.

Consider upward force as positive $\left(\uparrow +\right)$ and downward force as negative $\left(\downarrow -\right)$.

$\begin{array}{l}\Sigma F_y=0\\ S_{E,R}+A_y=0\\ S_{E,R}=A_y\end{array}$        (10)

Find the equation of shear force at E of portion EG $\left(4\text{m}\le x\le 14\text{m}\right)$.

Substitute $1-\frac{x}{8}$ for $A_y$ in Equation (10).

$S_{E,R}=1-\frac{x}{8}$

Find the equation of shear force at E of portion GH $\left(14\text{m}\le x\le 20\text{m}\right)$.

Substitute $\frac{x}{8}-\frac{5}{2}$ for $A_y$ in Equation (10).

$S_{E,R}=\frac{x}{8}-\frac{5}{2}$

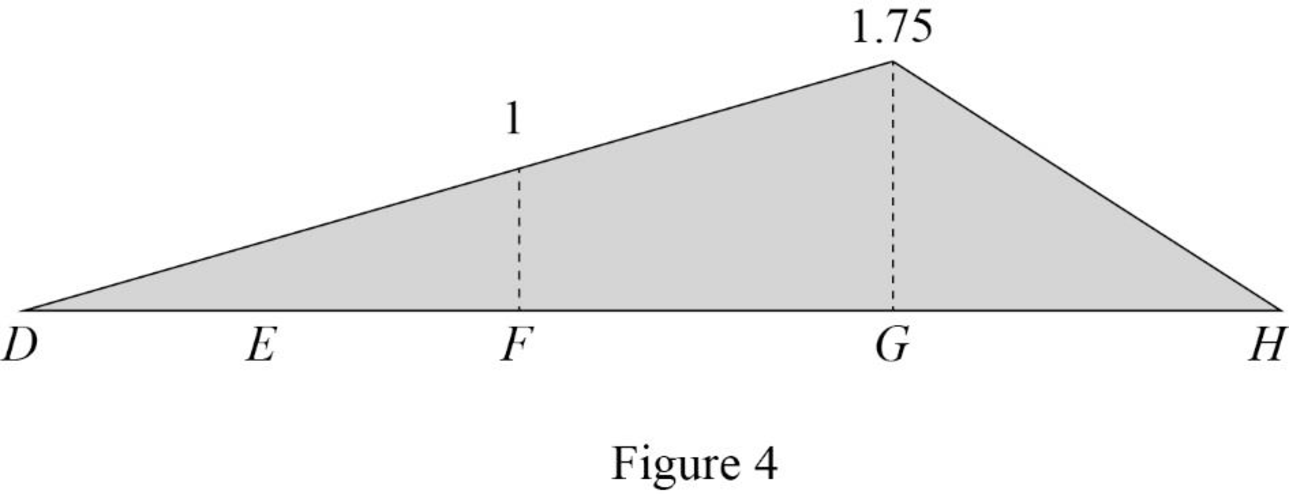
Thus, the equations of the influence line for $S_E$ as follows,

$S_{E,L}=-\frac{x}{8},0\le x<4\text{m}$        (11)

$S_{E,R}=1-\frac{x}{8},4\text{m}\le x<14\text{m}$        (12)

$S_{E,R}=\frac{x}{8}-\frac{5}{2},14\text{m}<x\le 20\text{m}$        (13)

Find the influence line ordinate of $S_{E,L}$ at just left of E using Equation (11).

Substitute 4 m for $x$ in Equation (11).

$\begin{array}{c}{S}_{E,L}=-\frac{4}{8}\\ =-0.5\text{kN}\end{array}$

Thus, the influence line ordinate of $S_E$ at just left of E is $-0.5\text{kN/kN}$.

Find the shear force of $S_E$ at various points of x using the Equations (11), (12), and (13) and summarize the value as in Table 4.

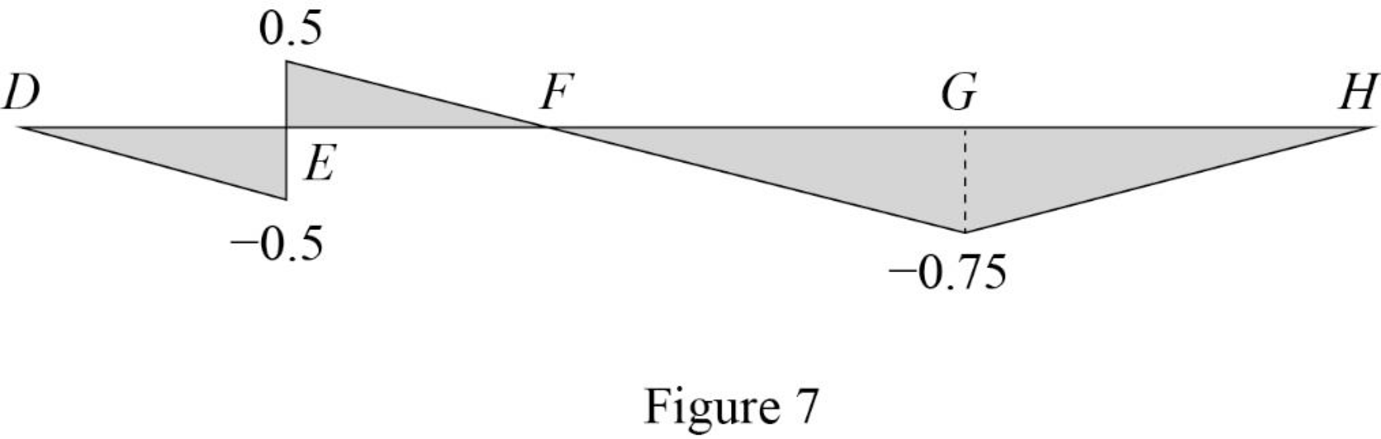
x (m)	Points	Influence line ordinate of $S_E$$\left(\text{kN/kN}\right)$
0	D	0
4	$E^{-}$	$-0.5$
4	$E^{+}$	$0.5$
8	F	0
14	G	$-0.75$
20	H	0

Draw the influence lines for the shear force at point E using Table 4 as shown in Figure 7.

Influence line for moment at point E.

Refer Figure 5.

Consider section DE.

Consider clockwise moment as positive and anticlockwise moment as negative.

Take moment at E.

Find the equation of moment at E of portion DE $\left(0\le x<4\text{m}\right)$.

$M_E=A_y\left(4\right)-\left(1\right)\left(4-x\right)$

Substitute $1-\frac{x}{8}$ for $A_y$.

$\begin{array}{c}{M}_E=\left(1-\frac{x}{8}\right)\left(4\right)-1\left(4-x\right)\\ =4-\frac{x}{2}-4+x\\ =\frac{x}{2}\end{array}$

Refer Figure 6.

Consider section DE.

Find the equation of moment at E of portion EH $\left(4\text{m}\le x<20\text{m}\right)$.

Consider clockwise moment as positive and anticlockwise moment as negative.

Take moment at E.

Find the equation of moment at E of portion EF.

$M_E=A_y\left(4\right)$        (14)

Find the equation of moment at E of portion EG $\left(4\text{m}\le x<14\text{m}\right)$.

Substitute $1-\frac{x}{8}$ for $A_y$ in Equation (14).

$\begin{array}{c}{M}_E=\left(1-\frac{x}{8}\right)\left(4\right)\\ =4-\frac{x}{2}\end{array}$

Find the equation of moment at E of portion GH $\left(14\text{m}\le x<20\text{m}\right)$.

Substitute $\frac{x}{8}-\frac{5}{2}$ for $A_y$ in Equation (14).

$\begin{array}{c}{M}_E=\left(\frac{x}{8}-\frac{5}{2}\right)\left(4\right)\\ =\frac{x}{2}-10\end{array}$

Thus, the equations of the influence line for $M_E$ as follows,

$M_E=\frac{x}{2},0\le x<4\text{m}$        (15)

$M_E=4-\frac{x}{2},4\text{m}\le x<14\text{m}$        (16)

$M_E=\frac{x}{2}-10,14\text{m}<x\le 20\text{m}$        (17)

Find the influence line ordinate of $M_E$ at E using Equation (15).

Substitute 4 m for $x$ in Equation (1).

$\begin{array}{c}{M}_E=\frac{4}{2}\\ =2\text{kN-m}\end{array}$

Thus, the influence line ordinate of $M_E$ at E is $2\text{kN-m/kN}$.

Find the moment at various points of x using the Equations (15), (16), and (17) and summarize the value as in Table 5.

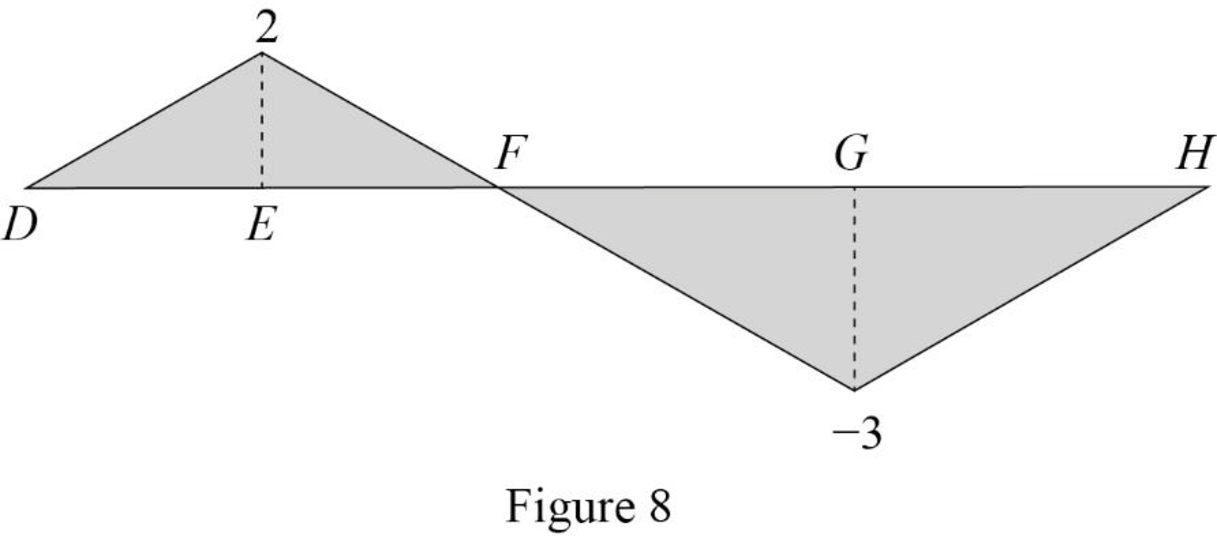
x (m)	Points	Influence line ordinate of $M_E$$\left(\text{kN-m/kN}\right)$
0	D	0
4	E	2
8	F	0
14	G	$-3$
20	H	0

Draw the influence lines for the moment at point E using Table 5 as shown in Figure 8.

Therefore, the influence lines for the vertical reactions at supports A, B, and C and the influence lines for the shear and bending moment at point E are drawn.