
Concept explainers
BIO Microraptor gui: The Biplane Dinosaur
The evolution of flight is a subject of intense interest in paleontology. Some subscribe to the “cursorial” (or ground-up) hypothesis, in which flight began with ground-dwelling animals running and jumping after prey Others favor the “arboreal” (or trees-down) hypothesis, in which tree-dwelling animals, like modern-day flying squirrels, developed flight as an extension of gliding from tree to tree.
A recently discovered fossil from the Cretaceous period in China supports the arboreal hypothesis and adds a new element—it suggests that feathers on both the wings and the lower legs and feet allowed this dinosaur, Microraptor gui, to glide much like a biplane, as shown in Figure 7-31 (a). Researchers have produced a detailed computer simulation of Microraptor, and with its help have obtained the power-versus-speed plot presented in Figure 7-31 (b). This curve shows how much power is required for flight at speeds between 0 and 30 m/s. Notice that the power increases at high speeds, as expected, but it is also high for low speeds, where the dinosaur is almost hovering. A minimum of 8.1 W is needed for flight at 10 m/s. The lower horizontal line shows the estimated 9.8-W power output of Microraptor, indicating the small range of speeds for which flight would be possible. The upper horizontal line shows the wider range of flight speeds that would be available if Microraptor were able to produce 20 W of power.
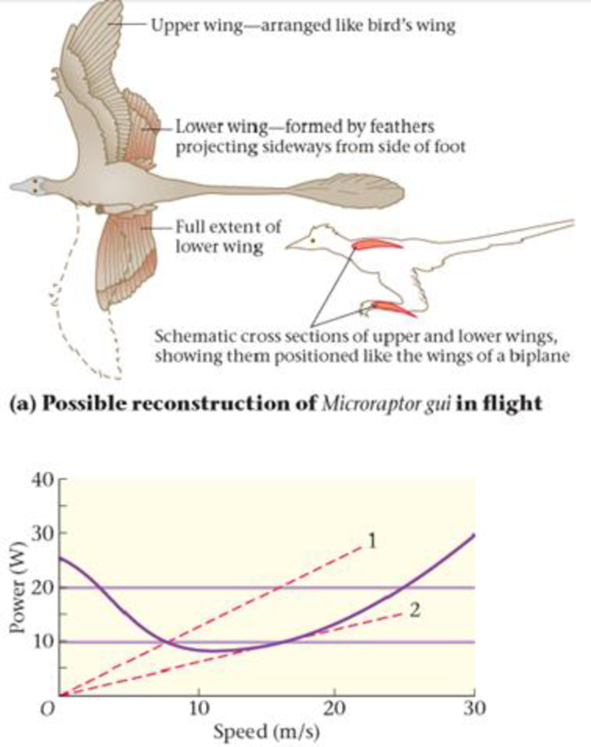
Also of interest are the two dashed, straight lines labeled 1 and 2. These lines represent constant ratios of power to speed—that is, a constant value for P/U Referring to Equation 7-13, we see that
P/v = Fv/v = F
, so lines 1 and 2 correspond to lines of constant force. Line 2 is interesting in that it has the smallest slope that still touches the power-versus-speed curve.
87. Estimate the minimum force that Microraptor must exert to fly
- A. A. 0.65 M
- B. B. 1.3 N
- C. C. 1.0 N
- D. D. 10 N
Want to see the full answer?
Check out a sample textbook solution
Chapter 7 Solutions
EBK PHYSICS
Additional Science Textbook Solutions
Cosmic Perspective Fundamentals
Human Physiology: An Integrated Approach (8th Edition)
Chemistry: An Introduction to General, Organic, and Biological Chemistry (13th Edition)
Campbell Biology in Focus (2nd Edition)
Human Biology: Concepts and Current Issues (8th Edition)
Genetic Analysis: An Integrated Approach (3rd Edition)
- Which of the following laws is true regarding tensile strength? • tensile strength T ①Fbreak = Wtfest Piece thickness rate (mm) ②T = test piece width rabe (mm) Fbreak break watarrow_forwardThe position of a squirrel running in a park is given by = [(0.280 m/s)t + (0.0360 m/s²)t²] + (0.0190 m/s³)ť³ĵj. What is v₂(t), the x-component of the velocity of the squirrel, as a function of time?arrow_forwardNo chatgpt pls will upvotearrow_forward
- You hold a spherical salad bowl 85 cm in front of your face with the bottom of the bowl facing you. The salad bowl is made of polished metal with a 40 cm radius of curvature. Where is the image of your 2.0 cm tall nose located? What is image's size, orientation, and nature. I keep getting the answer -26.2, but it keeps saying it is wrong. I just want to know what i'm doing wrong.arrow_forwardA converging lens with a focal length of 6.70 cm forms an image of a 4.60 mm tall real object that is to the left of the lens. The image is 1.50 cm tall and erect. Where are the object and image located? Is the image real or virtual? Please show all stepsarrow_forwardNo chatgpt pls will upvotearrow_forward
- need help part earrow_forwardCritical damping is the case where the mass never actually crosses over equilibrium position, but reaches equilibrium as fast as possible. Experiment with changing c to find the critical damping constant. Use the same initial conditions as in the last problem. Zoom in a bit to make sure you don't allow any oscillations to take place - even small ones.arrow_forwardNASA's KC-135 Reduced Gravity Research aircraft, affectionately known as the "Vomit Comet," is used in training astronauts and testing equipment for microgravity environments. During a typical mission, the aircraft makes approximately 30 to 40 parabolic arcs. During each arc, the aircraft and objects inside it are in free-fall, and passengers float freely in apparent "weightlessness." The figure below shows the altitude of the aircraft during a typical mission. It climbs from 24,000 ft to 30,850 ft, where it begins a parabolic arc with a velocity of 155 m/s at 45.0° nose-high and exits with velocity 155 m/s at 45.0° nose-low. 31 000 45° nose high 45° nose low 24 000 Zero g 65 Maneuver time (s) (a) What is the aircraft's speed (in m/s) at the top of the parabolic arc? 110.0 m/s (b) What is the aircraft's altitude (in ft) at the top of the parabolic arc? 2.04e+04 What is the initial height at the start of the parabolic arc? What is the initial velocity at this point? What is the final…arrow_forward

 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University





