Chapter 7, Problem 32P

The steel shaft shown in the figure carries a 18-lbf gear on the left and a 32-lbf gear on the right. Estimate the first critical speed due to the loads, the shaft’s critical speed without the loads, and the critical speed of the combination.

Problem 7–32

Dimensions in inches.

To determine

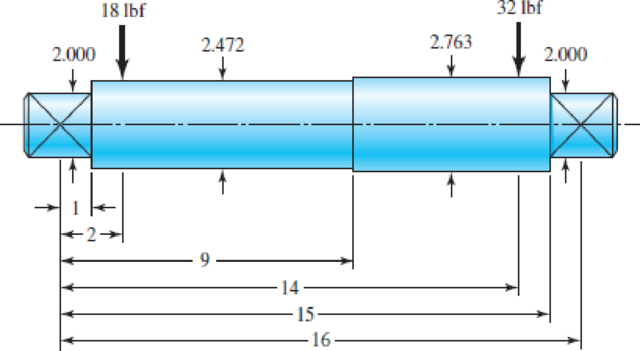
The first critical speed of the shaft due to loads.

The critical speed of the shaft without loads.

The critical speed of the combination.

Answer to Problem 32P

The first critical speed of the shaft due to loads is $2518\text{rad}/\text{s}$.

The critical speed of the shaft without loads is $2095.81\text{rad}/\text{s}$.

The critical speed of the combination is $1610.81\text{rad}/\text{s}$.

Explanation of Solution

Write the expression for the moment of the inertia.

    $I=\frac{\pi d^2}{64}$ (I)

Here, the diameter of the shaft is $d$ and the mathematical constant is $\pi$.

Write the expression for the ratio of the bending moment to inertia.

    $R_m=\frac{M}{I}$ (II)

Here, the bending moment across the shaft is $M$.

Write the expression for the ratio of the bending moment to inertia in terms of the spread sheet cell locations.

    $R_m=\left\{\begin{array}{l}{E}_2\left(x\right)+D_3{\left(x-1\right)}^0+F_3{\left(x-1\right)}^1+F_5{\left(x-2\right)}^1\\ +D_7{\left(x-9\right)}^0+F_7{\left(x-9\right)}^1+D_{11}{\left(x-15\right)}^0+F_{11}{\left(x-15\right)}^1\end{array}\right\}$ (III)

Substitute $E\frac{d^{2}y}{d{x}^2}$ for $R_m$ in Equation (III).

    $\begin{array}{c}{R}_m=\left\{\begin{array}{l}{E}_2\left(x\right)+D_3{\left(x-1\right)}^0+F_3{\left(x-1\right)}^1+F_5{\left(x-2\right)}^1\\ +D_7{\left(x-9\right)}^0+F_7{\left(x-9\right)}^1+D_{11}{\left(x-15\right)}^0+F_{11}{\left(x-15\right)}^1\end{array}\right\}\\ E\frac{d^{2}y}{d{x}^2}=\left\{\begin{array}{l}{E}_2\left(x\right)+D_3{\left(x-1\right)}^0+F_3{\left(x-1\right)}^1+F_5{\left(x-2\right)}^1\\ +D_7{\left(x-9\right)}^0+F_7{\left(x-9\right)}^1+D_{11}{\left(x-15\right)}^0+F_{11}{\left(x-15\right)}^1\end{array}\right\}\end{array}$ (IV)

Here, the young’s modulus of the shaft material is $E$, and the deflection in the shaft is $y$.

Integrate the Equation (IV) with respect to x.

    $\begin{array}{l}E\frac{d^{2}y}{d{x}^2}=\left\{\begin{array}{l}{E}_2\left(\frac{1}{2}\right)\left(x^2\right)+D_3{\left(x-1\right)}^1+F_3\left(\frac{1}{2}\right){\left(x-1\right)}^2+F_5\left(\frac{1}{2}\right){\left(x-2\right)}^2\\ +D_7{\left(x-9\right)}^1+F_7\left(\frac{1}{2}\right){\left(x-9\right)}^2+D_{11}{\left(x-15\right)}^1\\ +F_{11}\left(\frac{1}{2}\right){\left(x-15\right)}^2+C_1\end{array}\right\}\\ Ey=\left\{\begin{array}{l}{E}_2\left(\frac{1}{6}\right)\left(x^3\right)+D_3\left(\frac{1}{2}\right){\left(x-1\right)}^2+F_3\left(\frac{1}{3}\right){\left(x-1\right)}^3+F_5\left(\frac{1}{6}\right){\left(x-2\right)}^3\\ +D_7\left(\frac{1}{2}\right){\left(x-9\right)}^2+F_7\left(\frac{1}{6}\right){\left(x-9\right)}^3+D_{11}\left(\frac{1}{6}\right){\left(x-15\right)}^1\\ +F_{11}\left(\frac{1}{6}\right){\left(x-15\right)}^3+x{C}_1+C_2\end{array}\right\}\\ y=\frac{1}{E}\left\{\begin{array}{c}{E}_2\left(\frac{1}{6}\right)\left(x^3\right)+D_3\left(\frac{1}{2}\right){\left(x-1\right)}^2+F_3\left(\frac{1}{3}\right){\left(x-1\right)}^3+F_5\left(\frac{1}{6}\right){\left(x-2\right)}^3\\ +D_7\left(\frac{1}{2}\right){\left(x-9\right)}^2+F_7\left(\frac{1}{6}\right){\left(x-9\right)}^3+D_{11}\left(\frac{1}{6}\right){\left(x-15\right)}^1\\ +F_{11}\left(\frac{1}{6}\right){\left(x-15\right)}^3+x{C}_1+C_2\end{array}\right\}\end{array}$ (V)

Substitute $1.114085$ for $E_2$, $-0.636727$ for $D_3$, $-0.636727$ for $F_3$, $-0.545552$ for $F_5$, $-0.171504$ for $D_7$, $0.024501$ for $F_7$, $0.115461$ for $D_{11}$, $-0.115461$ for $F_{11}$ in Equation(V).

    $\begin{array}{c}y=\frac{1}{E}\left\{\begin{array}{l}1.114085\left(\frac{1}{6}\right)x^3-0.636727\left(\frac{1}{2}\right){\left(x-1\right)}^2\\ -0.636727\left(\frac{1}{6}\right){\left(x-1\right)}^3-0.545552\left(\frac{1}{6}\right){\left(x-2\right)}^3\\ -0.171504\left(\frac{1}{2}\right){\left(x-9\right)}^2+0.024501\left(\frac{1}{6}\right){\left(x-9\right)}^3\\ +0.115461\left(\frac{1}{2}\right){\left(x-15\right)}^2-0.115461\left(\frac{1}{6}\right){\left(x-15\right)}^3+x{C}_1+C_2\end{array}\right\}\\ =\frac{1}{E}\left\{\begin{array}{l}0.186x^3-0.318{\left(x-1\right)}^2-0.106{\left(x-1\right)}^3\\ -0.091{\left(x-2\right)}^3-0.086{\left(x-9\right)}^2+0.004{\left(x-9\right)}^3\\ +0.058{\left(x-15\right)}^2-0.019{\left(x-15\right)}^3+x{C}_1+C_2\end{array}\right\}\end{array}$ (VI)

Apply the boundary conditions.

Substitute $0$ for $y$, $0\text{in}$ for $x$ and in above Equation.

    $\begin{array}{l}y=\left\{\begin{array}{l}0.186x^3-0.318{\langle x-1\rangle }^2-0.106{\langle x-1\rangle }^3-0.091{\langle x-2\rangle }^3-0.086{\langle x-9\rangle }^2\\ +0.004{\langle x-9\rangle }^3+0.058{\langle x-15\rangle }^2-0.019{\langle x-15\rangle }^3+x{C}_1+C_2\end{array}\right\}\\ 0=\left\{\begin{array}{l}0.186{\langle 0\rangle }^3-0.318{\langle 0-1\rangle }^2-0.106{\langle 0-1\rangle }^3-0.091{\langle 0-1\rangle }^3-0.086{\langle 0-1\rangle }^2\\ +0.004{\langle 0-1\rangle }^3+0.058{\langle 0-1\rangle }^2-0.019{\langle 0-1\rangle }^3+0C_1+C_2\end{array}\right\}\\ C_2=0\end{array}$

Substitute $0$ for $y$, $16\text{in}$ for $x$ and in Equation (VI).

    $\begin{array}{l}y=\left\{\begin{array}{l}0.186x^3-0.318{\langle x-1\rangle }^2-0.106{\langle x-1\rangle }^3\\ -0.091{\langle x-2\rangle }^3-0.086{\langle x-9\rangle }^2+0.004{\langle x-9\rangle }^3\\ +0.058{\langle x-15\rangle }^2-0.019{\langle x-15\rangle }^3+x{C}_1+C_2\end{array}\right\}\\ 0=\left\{\begin{array}{l}0.186{\langle 16\rangle }^3-0.318{\langle 16-1\rangle }^2-0.106{\langle 16-1\rangle }^3\\ -0.091{\langle 16-2\rangle }^3-0.086{\langle 16-9\rangle }^2+0.004{\langle 16-9\rangle }^3\\ +0.058{\langle 16-15\rangle }^2-0.019{\langle 16-15\rangle }^3+16C_1+0\end{array}\right\}\\ 0=\left\{\begin{array}{l}761.85-71.55-357.75-249.704-4.214\\ +1.372+0.058-0.019+16C_1+0\end{array}\right\}\\ 5=C_1\end{array}$

Substitute ${\delta }_{11}$ for $y$, $5$ for $C_1$ and $0$ for $C_2$ in Equation (VI).

    ${\delta }_{11}=\frac{1}{E}\left\{\begin{array}{l}0.186x^3-0.318{\langle x-1\rangle }^2-0.106{\langle x-1\rangle }^3\\ -0.091{\langle x-2\rangle }^3-0.086{\langle x-9\rangle }^2+0.004{\langle x-9\rangle }^3\\ +0.058{\langle x-15\rangle }^2-0.019{\langle x-15\rangle }^3+x\langle 5\rangle \end{array}\right\}$ (VII)

Substitute ${\delta }_{12}$ for $y$, $5$ for $C_1$ and $0$ for $C_2$ in Equation in Equation (VI).

    ${\delta }_{12}=\frac{1}{E}\left\{\begin{array}{l}0.186x^3-0.318{\langle x-1\rangle }^2-0.106{\langle x-1\rangle }^3\\ -0.091{\langle x-2\rangle }^3-0.086{\langle x-9\rangle }^2+0.004{\langle x-9\rangle }^3\\ +0.058{\langle x-15\rangle }^2-0.019{\langle x-15\rangle }^3+x\langle 5\rangle \end{array}\right\}$ (VIII)

Write the expression for the deflection at $0\text{lbf}$ load on the left end.

    ${\delta }_{21}=\frac{1}{E}\left\{\begin{array}{l}0.186x^3-0.318{\langle x-1\rangle }^2-0.106{\langle x-1\rangle }^3\\ -0.091{\langle x-2\rangle }^3-0.086{\langle x-9\rangle }^2+0.004{\langle x-9\rangle }^3\\ +0.058{\langle x-15\rangle }^2-0.019{\langle x-15\rangle }^3+x\langle 5\rangle \end{array}\right\}$ (IX)

Write the expression for the deflection at $1\text{lbf}$ load on the right end.

    ${\delta }_{22}=\frac{1}{E}\left\{\begin{array}{l}0.186x^3-0.318{\langle x-1\rangle }^2-0.106{\langle x-1\rangle }^3\\ -0.091{\langle x-2\rangle }^3-0.086{\langle x-9\rangle }^2+0.004{\langle x-9\rangle }^3\\ +0.058{\langle x-15\rangle }^2-0.019{\langle x-15\rangle }^3+x\langle 5\rangle \end{array}\right\}$ (X)

Write the expression for the deflection of the shaft due to $18\text{lbf}$ load.

    $y_1=F_1{\delta }_{11}+F_2{\delta }_{12}$ (XI)

Write the expression for the deflection of the shaft due to $32\text{lbf}$ load.

    $y_2=F_1{\delta }_{21}+F_2{\delta }_{22}$ (XII)

Write the expression for the critical velocity due to load.

    ${\omega }_1=\sqrt{\frac{g\left(F_1{y}_1+F_2{y}_2\right)}{\left(F_1{y}_1^2+F_2{y}_2^2\right)}}$ (XIII)

Here, the acceleration due to gravity is $g$, the load on the left gear is $F_1$ and the load on the right gear is $F_2$.

Write the expression for the weight of the left gear.

    $w_1=\gamma \left(\frac{\pi }{4}\right)\left(d_1^2{l}_1+d_2^2{l}_2\right)$ (XIV)

Here, the physical constant of the material is $\gamma$, the length of the shaft is $l$ and the diameter is $d$.

Write the expression for the weight of the right gear.

    $w_2=\gamma \left(\frac{\pi }{4}\right)\left(d_3^2{l}_3+d_1^2{l}_1\right)$ (XV)

Write the expression for the critical velocity without load.

    ${\omega }_2=\sqrt{\frac{g\left(w_1{y}_1+w_2{y}_2\right)}{\left(w_1{y}_1^2+w_2{y}_2^2\right)}}$ (XVI)

Here, the acceleration due to gravity is $g$.

Write the expression for the critical speed for the combination using Dunkerley’s equation.

    $\frac{1}{{\omega }^2}={\left(\frac{1}{{\omega }_1^2}\right)}_{withload}+{\left(\frac{1}{{\omega }_2^2}\right)}_{withoutload}$ (XVII)

Conclusion:

Draw the diagram for the shaft.

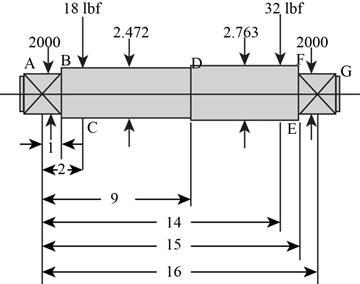
Figure-(1)

The Figure-(1) shows all the dimensions of the shaft.

Calculate the moment of inertia for the part $A$ to $B$:

Substitute $2\text{in}$ for $d$ in Equation (I).

    $\begin{array}{c}{I}_1=\frac{\pi {\left(2\text{in}\right)}^4}{64}\\ =\frac{50.26{\text{in}}^4}{64}\\ =0.58539{\text{in}}^4\\ \approx 0.7854{\text{in}}^4\end{array}$

Calculate the moment of inertia for the part $B$ to $D$:

Substitute $2.472\text{in}$ for $d$ in Equation (I).

    $\begin{array}{c}{I}_2=\frac{\pi {\left(2.472\text{in}\right)}^4}{64}\\ =\frac{117.312{\text{in}}^4}{64}\\ =1.833{\text{in}}^4\end{array}$

Calculate the moment of inertia for the part $D$ to $F$:

Substitute $2.763\text{in}$ for $d$ in Equation (I).

    $\begin{array}{c}{I}_3=\frac{\pi {\left(2.763\text{in}\right)}^4}{64}\\ =\frac{183.0937{\text{in}}^4}{64}\\ =2.860{\text{in}}^4\end{array}$

Since the diameter of the shaft part $A$ to $B$ and $F$ to $G$ is same, so their moment inertia is also same.

Substitute $2\text{in}$ for $x$ and $30\times {10}^6\text{psi}$ for $E$ in Equation (VII).

    $\begin{array}{c}{\delta }_{11}=\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}0.18{\langle 2\text{in}\rangle }^3-0.318{\langle 2\text{in}-\text{1}\rangle }^2-0.106{\langle 2\text{in}-\text{1}\rangle }^3\\ -0.091{\langle 2\text{in}-2\rangle }^3-0.086{\langle 2\text{in}-9\rangle }^2+0.004{\langle 2\text{in}-9\rangle }^3\\ +0.058{\langle 2\text{in}-\text{15}\rangle }^2-0.019{\langle 2\text{in}-\text{15}\rangle }^3-5\langle 2\text{in}\rangle \end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}1.488{\text{in}}^3-0.318{\text{in}}^2-0.106{\text{in}}^3-0-4.214{\text{in}}^2\\ -1.372{\text{in}}^3+9.802{\text{in}}^2+41.743{\text{in}}^3-10\text{in}\end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{37.023\text{in}\right\}\\ =1.234\times {10}^{-6}\text{in}\end{array}$

Substitute $14\text{in}$ for $x$ and $30\times {10}^6\text{psi}$ for $E$ in Equation (VIII).

    $\begin{array}{c}{\delta }_{12}=\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}0.186{\langle 14\text{in}\rangle }^3-0.318{\langle 14\text{in}-\text{1}\rangle }^2-0.106{\langle 14\text{in}-\text{1}\rangle }^3\\ -0.091{\langle 14\text{in}-2\rangle }^3-0.086{\langle 14\text{in}-9\rangle }^2+0.004{\langle 14\text{in}-9\rangle }^3\\ +0.058{\langle 14\text{in}-\text{15}\rangle }^2-0.019{\langle 14\text{in}-\text{15}\rangle }^3-5\langle 14\text{in}\rangle \end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}510.38{\text{in}}^3-53.74{\text{in}}^2-232.88{\text{in}}^3-157.24{\text{in}}^2-2.15{\text{in}}^2\\ -0.5{\text{in}}^3+0.058{\text{in}}^2+0.019{\text{in}}^3-28\text{in}\end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{35.951\text{in}\right\}\\ =1.198\times {10}^{-6}\text{in}\end{array}$

Substitute $14\text{in}$ for $x$ and $30\times {10}^6\text{psi}$ for $E$ in Equation (IX).

    $\begin{array}{c}{\delta }_{21}=\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}0.186{\langle 14\text{in}\rangle }^3-0.318{\langle 14\text{in}-\text{1}\rangle }^2-0.106{\langle 14\text{in}-\text{1}\rangle }^3\\ -0.091{\langle 14\text{in}-2\rangle }^3-0.086{\langle 14\text{in}-9\rangle }^2+0.004{\langle 14\text{in}-9\rangle }^3\\ +0.058{\langle 14\text{in}-\text{15}\rangle }^2-0.019{\langle 14\text{in}-\text{15}\rangle }^3-5\langle 14\text{in}\rangle \end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}510.38{\text{in}}^3-53.74{\text{in}}^2-232.88{\text{in}}^3-157.24{\text{in}}^2-2.15{\text{in}}^2\\ -0.5{\text{in}}^3+0.058{\text{in}}^2+0.019{\text{in}}^3-28\text{in}\end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{35.951\text{in}\right\}\\ =1.198\times {10}^{-6}\text{in}\end{array}$

Substitute $2\text{in}$ for $x$ and $30\times {10}^6\text{psi}$ for $E$ in Equation (X).

    $\begin{array}{c}{\delta }_{22}=\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}0.18{\langle 2\text{in}\rangle }^3-0.318{\langle 2\text{in}-\text{1}\rangle }^2-0.106{\langle 2\text{in}-\text{1}\rangle }^3\\ -0.091{\langle 2\text{in}-2\rangle }^3-0.086{\langle 2\text{in}-9\rangle }^2+0.004{\langle 2\text{in}-9\rangle }^3\\ +0.058{\langle 2\text{in}-\text{15}\rangle }^2-0.019{\langle 2\text{in}-\text{15}\rangle }^3-5\langle 2\text{in}\rangle \end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}1.488{\text{in}}^3-0.318{\text{in}}^2-0.106{\text{in}}^3-0-4.214{\text{in}}^2\\ -1.372{\text{in}}^3+9.802{\text{in}}^2+41.743{\text{in}}^3-10\text{in}\end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{37.023\text{in}\right\}\\ =1.234\times {10}^{-6}\text{in}\end{array}$

Substitute $18\text{lbf}$ for $F_1$, $32\text{lbf}$ for $F_2$, $1.234\times {10}^{-6}\text{in}$ for ${\delta }_{11}$ and $1.198\times {10}^{-6}\text{in}$ for ${\delta }_{12}$ in Equation (XI).

    $\begin{array}{c}{y}_1=\left(18\text{lbf}\right)\left(1.234\times {10}^{-6}\text{in}\right)+\left(32\text{lbf}\right)\left(1.198\times {10}^{-6}\text{in}\right)\\ =\left(22.21+38.336\right)\times {10}^{-6}\text{in}\\ =\text{6}\text{.05}\times {10}^{-5}\text{in}\end{array}$

Substitute $18\text{lbf}$ for $F_1$, $32\text{lbf}$ for $F_2$, $1.234\times {10}^{-6}\text{in}$ for ${\delta }_{22}$ and $1.198\times {10}^{-6}\text{in}$ for ${\delta }_{21}$ in Equation (XII).

    $\begin{array}{c}{y}_2=\left(18\text{lbf}\right)\left(1.198\times {10}^{-6}\text{in}\right)+\left(32\text{lbf}\right)\left(1.234\times {10}^{-6}\text{in}\right)\\ =\left(21.564+39.48\right)\times {10}^{-6}\text{in}\\ =\text{6}\text{.104}\times {10}^{-5}\text{in}\end{array}$

Here, the gravitational constant is $9.81\text{m}/{\text{s}}^{\text{2}}$.

    $\begin{array}{c}g=9.81\text{m}/{\text{s}}^{\text{2}}\\ =9.81\text{m}/{\text{s}}^{\text{2}}\left(\frac{39.37\text{in}/{\text{s}}^{\text{2}}}{1\text{m}/{\text{s}}^{\text{2}}}\right)\\ \approx 386\text{in}/{\text{s}}^{\text{2}}\end{array}$

Substitute $386\text{in}/{\text{s}}^{\text{2}}$ for $g$, $18\text{lbf}$ for $F_1$, $32\text{lbf}$ for $F_2$, $\text{6}\text{.104}\times {10}^{-5}\text{in}$ for $y_2$ and $\text{6}\text{.05}\times {10}^{-5}\text{in}$ for $y_1$ in Equation (XIII).

    $\begin{array}{c}{\omega }_1=\sqrt{\frac{\left(386\text{in}/{\text{s}}^{\text{2}}\right)\left[\left(\text{18}\text{lbf}\right)\left(\text{6}\text{.05}\times {10}^{-5}\text{lbf}\cdot \text{in}\right)+\left(32\text{lbf}\right)\left(\text{6}\text{.104}\times {10}^{-5}\text{lbf}\cdot \text{in}\right)\right]}{\left[\left(\text{18}\text{lbf}\right){\left(\text{6}\text{.05}\times {10}^{-5}\text{in}\right)}^2+\left(32\text{lbf}\right){\left(\text{6}\text{.104}\times {10}^{-5}\text{in}\right)}^2\right]}}\\ =\sqrt{\frac{\left(386\text{in}/{\text{s}}^{\text{2}}\right)\left[\left(108.9\times {10}^{-5}\text{in}\right)+\left(195.328\times {10}^{-5}\text{in}\right)\right]}{\left[\left(658.84\right){\left({10}^{-5}\text{in}\right)}^2+\left(1192.28\right){\left({10}^{-5}\text{in}\right)}^2\right]}}\\ =\sqrt{\frac{\left[\left(117432.008\times {10}^{-5}1/{\text{s}}^2\right)\right]}{\left[\left(1851.12\times {10}^{-10}\right)\right]}}\\ =2518\text{rad}/\text{s}\end{array}$

Thus, he first critical speed of shaft due to loads is $2518\text{rad}/\text{s}$.

Refer to table A-5 “Physical constants of the materials.” to obtain the weight density of the steel as $0.282\text{lbf}/{\text{in}}^{\text{3}}$.

Substitute $0.282\text{lbf}/{\text{in}}^{\text{3}}$ for $\gamma$, $2\text{in}$ for $d_1$, $2.472\text{in}$ for $d_2$, $1\text{in}$ for $l_1$ and $8\text{in}$ for $l_2$ in Equation (XIV).

    $\begin{array}{c}{w}_1=\left(0.282\text{lbf}/{\text{in}}^{\text{3}}\right)\left(\frac{\pi }{4}\right)\left[{\left(2\text{in}\right)}^2\left(1\text{in}\right)+{\left(2.472\text{in}\right)}^2\left(8\text{in}\right)\right]\\ =\left(0.282\text{lbf}/{\text{in}}^{\text{3}}\right)\left(\frac{\pi }{4}\right)\left[\left(52.88{\text{in}}^3\right)\right]\\ =11.71\text{lbf}\end{array}$

Substitute $0.282\text{lbf}/{\text{in}}^{\text{3}}$ for $\gamma$, $2\text{in}$ for $d_1$, $2.763\text{in}$ for $d_2$, $1\text{in}$ for $l_1$ and $6\text{in}$ for $l_2$ in Equation (XV).

    $\begin{array}{c}{w}_2=\left(0.282\text{lbf}/{\text{in}}^{\text{3}}\right)\left(\frac{\pi }{4}\right)\left[{\left(2.763\text{in}\right)}^2\left(6\text{in}\right)+{\left(2\text{in}\right)}^2\left(1\text{in}\right)\right]\\ =\left(0.282\text{lbf}/{\text{in}}^{\text{3}}\right)\left(\frac{\pi }{4}\right)\left[\left(49.80{\text{in}}^3\right)\right]\\ =11.03\text{lbf}\end{array}$

Draw the free body diagram for the calculated weights.

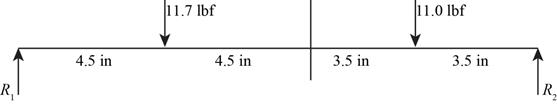
Substitute $4.5\text{in}$ for $x$ and $30\times {10}^6\text{psi}$ for $E$ in Equation (VII).

    $\begin{array}{c}{\delta }_{11}=\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}0.186{\langle 4.5\text{in}\rangle }^3-0.318{\langle 4.5\text{in}-\text{1}\rangle }^2-0.106{\langle 4.5\text{in}-\text{1}\rangle }^3\\ -0.091{\langle 4.5\text{in}-2\rangle }^3-0.086{\langle 4.5\text{in}-9\rangle }^2+0.004{\langle 4.5\text{in}-9\rangle }^3\\ +0.058{\langle 4.5\text{in}-\text{15}\rangle }^2-0.019{\langle 4.5\text{in}-\text{15}\rangle }^3-5\langle 4.5\text{in}\rangle \end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}16.949{\text{in}}^3-6.439{\text{in}}^2-4.544{\text{in}}^3-1.421{\text{in}}^3-1.741{\text{in}}^2\\ -0.3645{\text{in}}^3+6.394{\text{in}}^2+21.99{\text{in}}^3-22.5\text{in}\end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{8.3235\text{in}\right\}\\ =2.775\times {10}^{-5}\text{in}\end{array}$

Substitute $12.5\text{in}$ for $x$ and $30\times {10}^6\text{psi}$ for $E$ in Equation (VIII).

    $\begin{array}{c}{\delta }_{12}=\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}0.186{\langle 12.5\text{in}\rangle }^3-0.318{\langle 12.5\text{in}-\text{1}\rangle }^2-0.106{\langle 12.5\text{in}-\text{1}\rangle }^3\\ -0.091{\langle 12.5\text{in}-2\rangle }^3-0.086{\langle 12.5\text{in}-9\rangle }^2+0.004{\langle 12.5\text{in}-9\rangle }^3\\ +0.058{\langle 12.5\text{in}-\text{15}\rangle }^2-0.019{\langle 12.5\text{in}-\text{15}\rangle }^3-5\langle 12.5\text{in}\rangle \end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}363.28{\text{in}}^3-42.055{\text{in}}^2-161.21{\text{in}}^3-105.34{\text{in}}^3-1.053{\text{in}}^2\\ +0.1715{\text{in}}^3+0.3625{\text{in}}^2+0.2968{\text{in}}^3-62.5\text{in}\end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{8.0472\text{in}\right\}\\ =2.68\times {10}^{-5}\text{in}\end{array}$

Substitute $12.5\text{in}$ for $x$ and $30\times {10}^6\text{psi}$ for $E$ in Equation (IX).

    $\begin{array}{c}{\delta }_{21}=\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}0.186{\langle 12.5\text{in}\rangle }^3-0.318{\langle 12.5\text{in}-\text{1}\rangle }^2\\ -0.106{\langle 12.5\text{in}-\text{1}\rangle }^3-0.091{\langle 12.5\text{in}-2\rangle }^3\\ -0.086{\langle 12.5\text{in}-9\rangle }^2+0.004{\langle 12.5\text{in}-9\rangle }^3\\ +0.058{\langle 12.5\text{in}-\text{15}\rangle }^2-0.019{\langle 12.5\text{in}-\text{15}\rangle }^3-5\langle 12.5\text{in}\rangle \end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}363.28{\text{in}}^3-42.055{\text{in}}^2-161.21{\text{in}}^3-105.34{\text{in}}^3-1.053{\text{in}}^2\\ +0.1715{\text{in}}^3+0.3625{\text{in}}^2+0.2968{\text{in}}^3-62.5\text{in}\end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{8.0472\text{in}\right\}\\ =2.68\times {10}^{-5}\text{in}\end{array}$

Substitute $3.5\text{in}$ for $x$ and $30\times {10}^6\text{psi}$ for $E$ in Equation (X).

    $\begin{array}{c}{\delta }_{22}=\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}0.186{\langle 3.5\text{in}\rangle }^3-0.318{\langle 3.5\text{in}-\text{1}\rangle }^2-0.106{\langle 3.5\text{in}-\text{1}\rangle }^3\\ -0.091{\langle 3.5\text{in}-2\rangle }^3-0.086{\langle 3.5\text{in}-9\rangle }^2+0.004{\langle 3.5\text{in}-9\rangle }^3\\ +0.058{\langle 3.5\text{in}-\text{15}\rangle }^2-0.019{\langle 3.5\text{in}-\text{15}\rangle }^3-5\langle 3.5\text{in}\rangle \end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{\begin{array}{l}7.974{\text{in}}^3-1.987{\text{in}}^2-1.656{\text{in}}^3-0.307{\text{in}}^3-2.601{\text{in}}^2\\ -0.665{\text{in}}^3+7.670{\text{in}}^2+28.896{\text{in}}^3-17.5\text{in}\end{array}\right\}\\ =\frac{1}{\left(30\times {10}^6\text{psi}\right)}\left\{19.824\text{in}\right\}\\ =6.608\times {10}^{-5}\text{in}\end{array}$

Substitute $11.7\text{lbf}$ for $F_1$, $11.03\text{lbf}$ for $F_2$, $2.775\times {10}^{-5}\text{in}$ for ${\delta }_{11}$ and $2.68\times {10}^{-5}\text{in}$ for ${\delta }_{12}$ in Equation (XI).

    $\begin{array}{c}{y}_1=\left(11.7\text{lbf}\right)\left(2.775\times {10}^{-5}\text{in}\right)+\left(11.03\text{lbf}\right)\left(2.68\times {10}^{-5}\text{in}\right)\\ =\left(32.46+29.56\right)\times {10}^{-5}\text{in}\\ =\text{6}\text{.202}\times {10}^{-5}\text{in}\end{array}$

Substitute $11.7\text{lbf}$ for $F_1$, $11.03\text{lbf}$ for $F_2$, $6.608\times {10}^{-5}\text{in}$ for ${\delta }_{22}$ and $2.68\times {10}^{-5}\text{in}$ for ${\delta }_{21}$ in Equation (XII).

    $\begin{array}{c}{y}_2=\left(11.7\text{lbf}\right)\left(2.68\times {10}^{-5}\text{in}\right)+\left(11.03\text{lbf}\right)\left(6.608\times {10}^{-5}\text{in}\right)\\ =\left(31.35+72.88\right)\times {10}^{-5}\text{in}\\ =10.42\times {10}^{-5}\text{in}\end{array}$

Substitute $386\text{in}/{\text{s}}^{\text{2}}$ for $g$, $11.7\text{lbf}$ for $F_1$, $11.03\text{lbf}$ for $F_2$, $10.42\times {10}^{-5}\text{in}$ for $y_2$ and $\text{6}\text{.202}\times {10}^{-5}\text{in}$ for $y_1$ in Equation (XVI).

    $\begin{array}{c}{\omega }_2=\sqrt{\frac{\left(386\text{in}/{\text{s}}^{\text{2}}\right)\left[\left(11.7\text{lbf}\right)\left(\text{6}\text{.202}\times {10}^{-5}\text{in}\right)+\left(11.03\text{lbf}\right)\left(10.42\times {10}^{-5}\text{in}\right)\right]}{\left[\left(11.7\text{lbf}\right){\left(\text{6}\text{.202}\times {10}^{-5}\text{in}\right)}^2+\left(11.03\text{lbf}\right){\left(10.42\times {10}^{-5}\text{in}\right)}^2\right]}}\\ =\sqrt{\frac{\left(386\text{in}/{\text{s}}^{\text{2}}\right)\left[\left(72.56\times {10}^{-5}\text{in}\right)+\left(114.93\times {10}^{-5}\text{in}\right)\right]}{\left[\left(450.03\right){\left({10}^{-5}\text{in}\right)}^2+\left(1197.59\right){\left({10}^{-5}\text{in}\right)}^2\right]}}\\ =\sqrt{\frac{\left[\left(72371.14\times {10}^{-5}1/{\text{s}}^2\right)\right]}{\left[\left(1647.6276\times {10}^{-10}\right)\right]}}\\ =2095.81\text{rad}/\text{s}\end{array}$

Thus, the critical speed of shaft without loads is $2095.81\text{rad}/\text{s}$.

Substitute $2095.81\text{rad}/\text{s}$ for ${\omega }_2$ $2518\text{rad}/\text{s}$ for ${\omega }_1$ in Equation (XVII).

    $\begin{array}{c}\frac{1}{{\omega }^2}=\left(\frac{1}{{\left(2518\text{rad}/\text{s}\right)}^2}\right)+\left(\frac{1}{{\left(2095.81\text{rad}/\text{s}\right)}^2}\right)\\ \frac{1}{{\omega }^2}=\left[\left(1.5772\times {10}^{-7}\right)+\left(2.2766\times {10}^{-7}\right)\right]{\left(\text{rad}/\text{s}\right)}^2\\ {\omega }^2=2594841.455{\left(\text{rad}/\text{s}\right)}^2\\ \omega =1610.81\text{rad}/\text{s}\end{array}$

Thus, the critical speed of the combination is $1610.81\text{rad}/\text{s}$.