Chapter 7, Problem 11CR

(a)

To determine

The real zeros of $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ and their multiplicity.

Answer to Problem 11CR

Solution:

The real zeros of $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ are $-1,\frac{1}{2},1$ .

  $-1$ and $1$ are the zero of the function with multiplicity $2$ whereas $\frac{1}{2}$ is the zero of the function with multiplicity $1$ .

Explanation of Solution

Given information:

The function, $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$

Consider the function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

By rational root theorem,

The divisors of the constant term are $p=\pm 1$ .

The divisors of the leading coefficient are $q=\pm 1,\pm 2$ .

Then, possible rational zeros of the polynomial are, $\frac{p}{q}=\pm 1,\pm \frac{1}{2}$ .

Now, test $x=-1$ using synthetic division.

  $\begin{array}{l}-1\overline{)2-1-422-1}\\ \underset{\_}{-231-31}\\ 2-3-13-10\end{array}$

Here, since the remainder is $0$, $x=-1$ is a zero of $f$ .

After taking $x+1$ as a factor,

  $2x^5-x^4-4x^3+2x^2+2x-1=\left(x+1\right)\left(2x^4-3x^3-x^2+3x-1\right)$

Then, the depressed equation is $2x^4-3x^3-x^2+3x-1$ .

  $2x^4-3x^3-x^2+3x-1$

  $=x^4+x^4-3x^3-x^2+3x-1$

  $=x^4-x^2+x^4-1-3x^3+3x$

  $=x^2\left(x^2-1\right)+\left(x^2-1\right)\left(x^2+1\right)-3x\left(x^2-1\right)$

  $=\left(x^2-1\right)\left(x^2+x^2+1-3x\right)$

  $=\left(x^2-1\right)\left(2x^2+1-3x\right)$

  $=\left(x+1\right)\left(x-1\right)\left(2x^2+1-3x\right)$ .

By quadratic formula, the zeros of the quadratic equation $2x^2+1-3x$ ; $x=\frac{-b\pm \sqrt{b^2-4ac}}{2a}$ .

  $x=\frac{-\left(-3\right)\pm \sqrt{9-8}}{4}=\frac{3\pm 1}{4}=$

  $\Rightarrow x=1\text{or}x=\frac{1}{2}$

The factors of $2x^2+1-3x$ are $\left(x-1\right)\text{and}\left(x-\frac{1}{2}\right)$ .

  $2x^5-x^4-4x^3+2x^2+2x-1=\left(x+1\right)\left(x+1\right)\left(x-1\right)\left(2x^2+1-3x\right)=\left(x+1\right)\left(x+1\right)\left(x-1\right)\left(x-1\right)\left(x-\frac{1}{2}\right)$

The factor form of $f\left(x\right)$ is.

  $2x^5-x^4-4x^3+2x^2+2x-1={\left(x+1\right)}^2{\left(x-1\right)}^2\left(x-\frac{1}{2}\right)$

Hence, the real zeros of $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ are $-1,\frac{1}{2},1$ and the factor form is ${\left(x+1\right)}^2{\left(x-1\right)}^2\left(x-\frac{1}{2}\right)$ .

  $-1$ is the zero of the function with multiplicity $2$ since the exponent of the factor ${\left(x+1\right)}^2$ is $2$ .

  $\frac{1}{2}$ is the zero of the function with multiplicity $1$ since the exponent of the factor $x-\frac{1}{2}$

is $1$ .

  $1$ is the zero of the function with multiplicity $2$ since the exponent of the factor ${\left(x-1\right)}^2$ is $2$ .

(b)

To determine

The $x-$ intercept and $y$ -intercepts of the function, $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

Answer to Problem 11CR

Solution:

The $x-$ intercepts of the function are $\left(0,-1\right),\left(0,\frac{1}{2}\right)$ and, $\left(0,1\right)$ and the $y-$ intercept of the polynomial function is $\left(-1,0\right)$ .

Explanation of Solution

Given information:

The function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

To find $y-$ intercepts substitute $x=0$ in the function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$,

  $f\left(0\right)=2{\left(0\right)}^5-{\left(0\right)}^4-4{\left(0\right)}^3+2{\left(0\right)}^2+2\left(0\right)-1=-1$

Thus the $y-$ intercept of the polynomial function is $(-1,0)$ .

Now to find $x-$ intercept of the function substitute $f\left(x\right)=0$ in the function.

  $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$, it gives

  $0=2x^5-x^4-4x^3+2x^2+2x-1$

  $\Rightarrow {\left(x+1\right)}^2{\left(x-1\right)}^2\left(x-\frac{1}{2}\right)=0$

  $\Rightarrow x+1=0$ or, $x-1=0$ or $x-\frac{1}{2}=0$

  $\Rightarrow x=-1$ or, $x=1$ or $x=\frac{1}{2}$

Hence the $x-$ intercepts of the function are $\left(0,-1\right),\left(0,\frac{1}{2}\right)$ and $\left(0,1\right)$ .

(c)

To determine

The power function that the graph of $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ resembles for large values of $\left|x\right|$ .

Answer to Problem 11CR

Solution:

Thegraph of the function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ resembles like $y=2x^5$ for large values of $\left|x\right|$ .

Explanation of Solution

Given information:

The function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

The polynomial function is $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

Here the degree of the polynomial function $f\left(x\right)$ is $5$ .

The graph of the function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ behaves like $y=2x^5$ for large values of $\left|x\right|$ .

(d)

To determine

To graph: The function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ using a graphing utility.

Explanation of Solution

Given information:

The function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

Graph:

Use the steps below to graph the function using a graphing calculator.

Step I: Press the ON key.

Step II: Now, press [Y=]. Input the right hand side of the function $y=2x^5-x^4-4x^3+2x^2+2x-1$ in $Y_1$

Step III: Press [WINDOW] key and set the viewing window as below,

  $\begin{array}{ll}X\min =-2\hfill & X\max =2X\text{scl}=1\hfill \\ Y\min =-2\hfill & Y\max =1Y\text{scl}=1\hfill \end{array}$

Step IV: Then hit [Graph] key to view the graph.

The graph of the function is as follows:

  

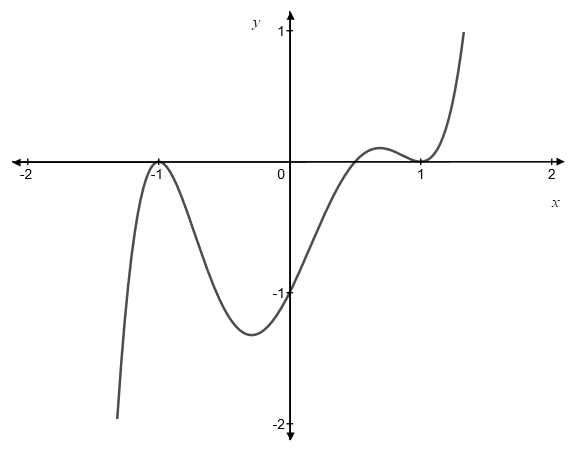
Interpretation:

The graph of the function $f\left(x\right)$ crosses the $x-$ axis at $x=\frac{1}{2}$ since the multiplicity of the $\frac{1}{2}$ is $1$ that is odd multiplicity.

(e)

To determine

The approximation of the turning points, if exists, of the function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

Answer to Problem 11CR

Solution:

The turning points of $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ are $\left(-0.29,-1.325\right),\left(1,0\right)$, $\left(0.69,0.104\right),\left(-1,0\right)$ .

Explanation of Solution

Given information:

The function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

Let, the function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

The maximum number of real zeros is the degree of the polynomial.

Here, the degree of $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ is $5$ .

Since the polynomial function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ has degree $5$ so the maximum number of the turning points on the graph of the function $f\left(x\right)$ is $5-1=4$ .

For the approximation of the turning points find out the maxima and minima using a graphing calculator.

To graph the function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ using graphing utility use the below steps.

Step I: Press the ON key.

Step II: Now, press [Y=]. Input the right hand side of the function $y=2x^5-x^4-4x^3+2x^2+2x-1$ in $Y_1$

Step III: Press [WINDOW] key and set the viewing window as below,

  $\begin{array}{ll}X\min =-2\hfill & X\max =2X\text{scl}=1\hfill \\ Y\min =-2\hfill & Y\max =1Y\text{scl}=1\hfill \end{array}$

Step IV: Then hit [Graph] key to view the graph.

The graph of the function is as follows:

  

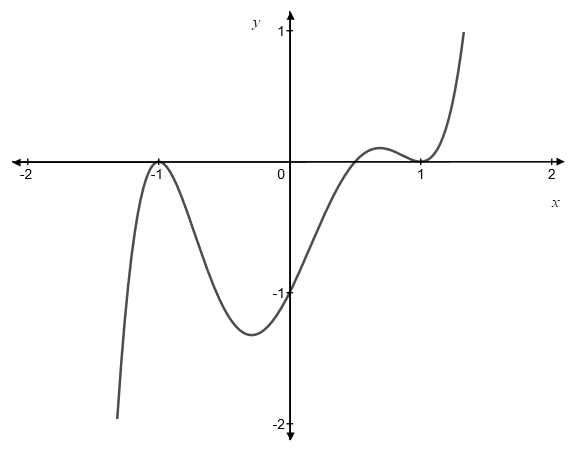
To find local maximum and local minimum on the graph using graphing utility use below steps,

Step IV: Press [2ND] [TRACE] to access the calculate menu

Step V: press [MAXIMUM] and press [ENTER].

Step VI: Set left bound by using the left and right arrow. Click [ENTER].

Step VII: Set right bound by using the left and right arrow. Click [ENTER].

Step VIII: Click [Enter] button twice.

It will give the maximum value $x=0.69,y=0.104$

It will give the maximum value $x=-1,y=0$

Thus, the function have its local maximum value at $\left(0.69,0.104\right),\left(-1,0\right)$ .

To find local minimum value use below steps.

Step IX: Press [2ND] [TRACE] to access the calculate menu

Step X: press [MINIMUM] and press [ENTER].

Step XI: Set left bound by using the left and right arrow. Click [ENTER].

Step XII: Set right bound by using the left and right arrow. Click [ENTER].

Step XIII: Click [Enter] button twice.

It will give the minimum value $x=-0.29,y=-1.325$ .

It will give the minimum value $x=1,y=0$ .

Thus, the function has its local minimum value at $\left(-0.29,-1.325\right),\left(1,0\right)$ .

Therefore, the turning points are $\left(-0.29,-1.325\right),\left(1,0\right)$, $\left(0.69,0.104\right),\left(-1,0\right)$ .

(f)

To determine

To graph: The function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

Explanation of Solution

Given information:

The function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$

Graph:

The polynomial function is $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

From all the above parts, the analysis of the function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ are stated below:

The graph of the function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ behaves like $y=2x^5$ for large values of $\left|x\right|$ .

Thezeros of the function are $-5,-\frac{1}{2}$ and $3$

The $x-$ intercepts of the function are $-1,\frac{1}{2}$ and $1$ and the $y-$ intercept of the polynomial function is $-1$ .

The graph of the function $f\left(x\right)$ crosses the $x-$ axis at $x=\frac{1}{2}$ since the multiplicity of the $\frac{1}{2}$ is $1$ that is odd multiplicity and also the graph of function $f\left(x\right)$ touches the $x-$ axis at $x=1,x=-1$ since the multiplicity of the $-1,1$ are $2$ which is even.

Here the degree of the polynomial function $f\left(x\right)$ is $5$, the maximum number of tuning points are $5-1=4$ which are at $x=-1,x=0.29,x=0.69,x=1$ .

Using all this information, the graph will look alike:

  

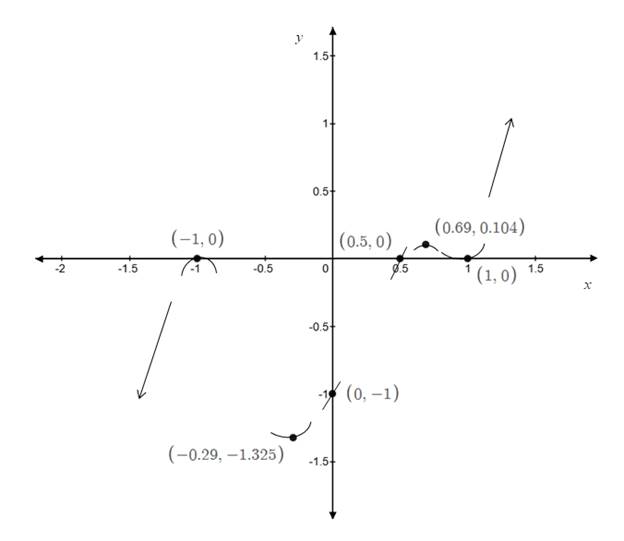
Now find additional points on the graph on each side of $x-$ intercept as follows

For $x=-1.1$ the value of $f\left(x\right)$ at $x=-1.1$ is $f\left(-1.1\right)=2{\left(-1.1\right)}^5-{\left(-1.1\right)}^4-4{\left(-1.1\right)}^3+2{\left(-1.1\right)}^2+2\left(-1.1\right)-1=-0.1411$

For $x=-0.5$ the value of $f\left(x\right)$ at $x=-0.5$ is $f\left(-0.5\right)=2{\left(-0.5\right)}^5-{\left(-0.5\right)}^4-4{\left(-0.5\right)}^3+2{\left(-0.5\right)}^2+2\left(-0.5\right)-1=-1.125$

For $x=-0.25$ the value of $f\left(x\right)$ at $x=-0.25$ is $f\left(-0.25\right)=2{\left(-0.25\right)}^5-{\left(-0.25\right)}^4-4{\left(-0.25\right)}^3+2{\left(-0.25\right)}^2+2\left(-0.25\right)-1=-1.3183$

For $x=0.25$ the value of $f\left(x\right)$ at $x=0.25$ is $f\left(0.25\right)=2{\left(0.25\right)}^5-{\left(0.25\right)}^4-4{\left(0.25\right)}^3+2{\left(0.25\right)}^2+2\left(0.25\right)-1=-0.4395$

For $x=0.55$ the value of $f\left(x\right)$ at $x=0.55$ is $f\left(0.55\right)=2{\left(0.55\right)}^5-{\left(0.55\right)}^4-4{\left(0.55\right)}^3+2{\left(0.55\right)}^2+2\left(0.55\right)-1=0.0487$

For $x=0.75$ the value of $f\left(x\right)$ at $x=0.75$ is $f\left(0.25\right)=2{\left(0.25\right)}^5-{\left(0.75\right)}^4-4{\left(0.75\right)}^3+2{\left(0.75\right)}^2+2\left(0.75\right)-1=0.0957$

For $x=1.1$ the value of $f\left(x\right)$ at $x=1.1$ is $f\left(1.1\right)=2{\left(1.1\right)}^5-{\left(1.1\right)}^4-4{\left(1.1\right)}^3+2{\left(1.1\right)}^2+2\left(1.1\right)-1=0.0529$

Now plot all these coordinates $\left(-1.1,-0.1411\right),\left(-0.5,1.125\right),\left(-0.25,-1.3183\right),\left(0.25,-0.4395\right),(0.55,0.0487),(0.75,0.0957),(1.1,0.0529)$ on the graph and join them.

Therefore, the graph of the function is as follows:

  

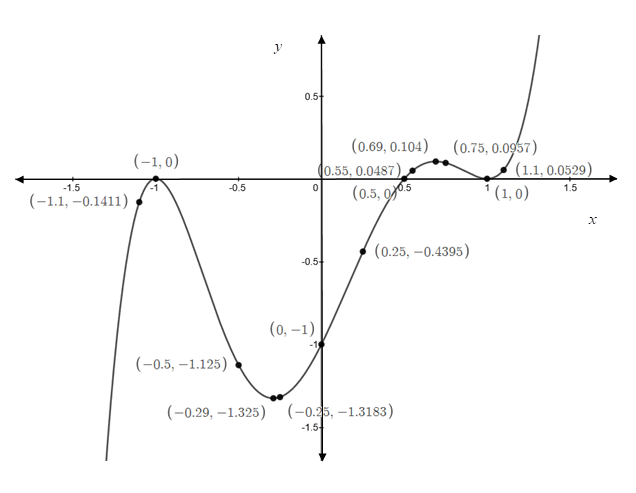
Interpretation:

The graph of the function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ behaves like $y=2x^5$ for large values of $\left|x\right|$ .

Thezeros of the function are $-5,-\frac{1}{2}$ and $3$ .

The $x-$ intercepts of the function are $-1,\frac{1}{2}$ and, $1$ and the $y-$ intercept of the polynomial function is $-1$ .

The graph of the function $f\left(x\right)$ crosses the $x-$ axis at $x=\frac{1}{2}$ since the multiplicity of the $\frac{1}{2}$ is $1$ that is odd multiplicity and also the graph of function $f\left(x\right)$ touches the $x-$ axis at $x=1,x=-1$ since the multiplicity of the $-1,1$ is $2$ which is even.

Here the degree of the polynomial function $f\left(x\right)$ is $5$, the maximum number of tuning points are $5-1=4$ which are at $x=-1,x=0.29,x=0.69,x=1$ .

(g)

To determine

The intervals where the function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ is increasing, or decreasing or constant.

Answer to Problem 11CR

Solution:

The function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ is increasing in the interval $\left(-\infty ,-1\right),\left(-0.29,0.69\right),(1,\infty )$ and decreasing in the intervals $\left(-1,-0.29\right),\left(0.69,1\right)$ and nowhere constant.

Explanation of Solution

Given information:

The function, $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

The polynomial function is $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ .

From parts (d),(e),(f) the graph of the function $f\left(x\right)=2x^5-x^4-4x^3+2x^2+2x-1$ is stated below:

  

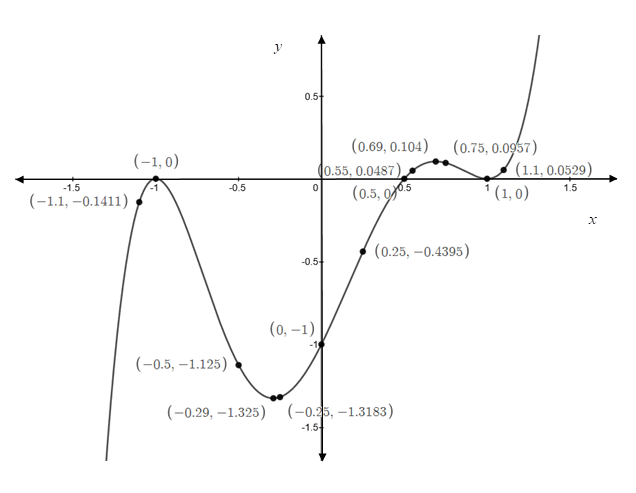
Here the degree of the polynomial function $f\left(x\right)$ is $5$, the maximum number of tuning points are $5-1=4$ which are at $x=-1,x=0.29,x=0.69,x=1$ .

From the graph, it is clearly evident the graph is increasing in the interval $\left(-\infty ,-1\right),\left(-0.29,0.69\right),(1,\infty )$ and decreasing in the intervals $\left(-1,-0.29\right),\left(0.69,1\right)$ and nowhere constant.