
Interpretation:
To plot the nullclines
Concept Introduction:
Nullclines are the curves in the phase portrait where either
Fixed points occur where
The Jacobian matrix at a general point
The Eigenvalue
The solution of the quadratic equation is
The unstable manifold for a fixed point is the set of all points in the plane which tend to the fixed point as time goes to negative infinity.
Answer to Problem 6E
Solution:
a) The nullclines
b) The sign of
c) The Eigenvalues and Eigenvectors of the saddle points at
d) It is proved that the unstable manifold
e) The phase portrait for the given system is plotted.
Explanation of Solution
a) The system is given as
Nullclines are the curves in the phase portrait where either
Substituting
Thus,
Substituting
Therefore, the nullclines of the given system are
The nullclines plot for the given system equation is shown below:
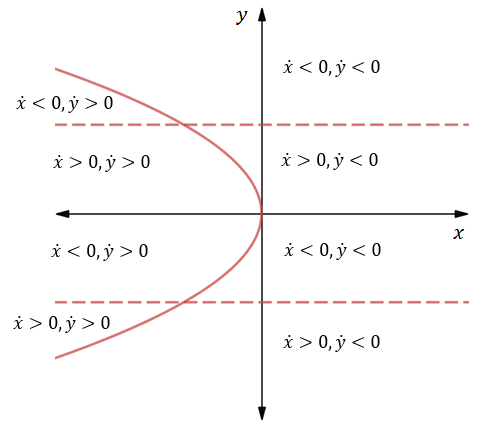
b) The value of the system at
The sign of
c) The fixed points of the system would be where
The fixed points can be obtained by substituting
Therefore, the fixed points are
The Jacobian matrix at a general point
Substituting the given system in the Jacobian matrix,
The value of the Jacobian matrix at the fixed point
Therefore, from the Jacobian matrix, it is clear that the fixed point
The value of the Jacobian matrix at the fixed point
The value of the Jacobian matrix at the fixed point
The Eigenvalue
To find the Eigenvalues and the Eigenvectors of the Jacobian matrix
The determinant of the above matrix is
From the above matrix,
The above quadratic equation can be solved by using
Therefore, the Eigenvalue of the Jacobian matrix
The corresponding Eigenvectors for the above Jacobian matrix
Similarly, to find the Eigenvalues and the Eigenvectors of the Jacobian matrix
The determinant of the above matrix is
From the above matrix,
The above quadratic equation can be solved by using
Therefore, the Eigenvalue of the Jacobian matrix
The corresponding Eigenvectors for the above Jacobian matrix
d) The unstable manifold for a fixed point is the set of all points in the plane which tend to the fixed point as time goes to negative infinity.
Consider the unstable manifold of the saddle point
Since the system is reversible under the transformation
e) Consider the unstable manifold of the saddle point
Since the system is reversible under the transformation
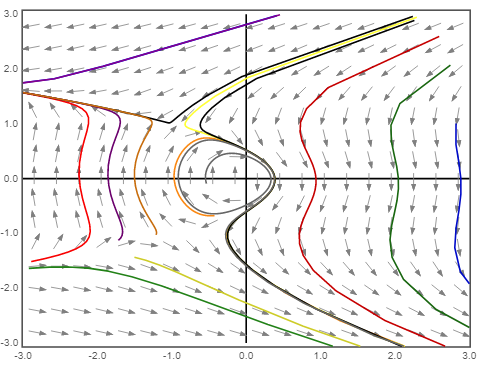
The phase portrait of the given system is shown below:
Want to see more full solutions like this?
Chapter 6 Solutions
Nonlinear Dynamics and Chaos
- Design a PDA recognizing each of the following languages and draw its state diagram. Note that the transition function must be in the format of “a, b →c", namely we can only push/pop one symbol into/from the stack one time upon one input symbol. You will receive 0 point if you push/pop multiple symbols into/from the stack one time upon one input symbol. (1) {w|wa"b", n is odd} = (2) {w|w=w², length of w is odd and Σ = {a,b} } (3) {w|w= = a²b²n, n ≥1 } (4) {w|w= =a^bn+mcm, n≥0, m ≥ 1 } (5) {w|w=a²b³n, n≥0} (6) {w|w= = a¹³, n ≥ 1, m≥ 1 and n‡m } Hint: two cases: n > m and narrow_forward[) Hwk 29 ✗ WHwk 30 (MA 244-03) (SP X - Logout Cengage Learning X MA244-03 Syllabus_Sprin X b Answered: [) Hwk 29 Hwk X https://www.webassign.net/web/Student/Assignment-Responses/last?dep=36606609 4. [-/3 Points] DETAILS MY NOTES LARLINALG8 7.4.013. Solve the system of first-order linear differential equations. (Use C1 and C2 as constants.) Y1' = -4Y1 Y2' = -12 (y1(t), Y2(t)) = ( 3 Need Help? Read It SUBMIT ANSWER 5. [-/3 Points] DETAILS MY NOTES LARLINALG8 7.4.019. Solve the system of first-order linear differential equations. (Use C1, C2, C3, and C4 as constants.) Y1' = 6y1 Y2' = 2y2 Y3' = -643 Y4' = -2y4 = (y1(t), y2(t), y3(t), Y4(t)) = Need Help? Read It SUBMIT ANSWER G Use the Principal Axes The X G cot(0) - Google Search ☑ B 90% + ASK YOUR TEACHER PRACTICE ANOTHER ill ASK YOUR TEACHER PRACTICE ANOTHER 6. [-/4 Points] DETAILS MY NOTES LARLINALG8 7.4.023. Solve the system of first-order linear differential equations. (Use C1 and C2 as constants.) ASK YOUR TEACHER Y1' = Y1 + 5y2 Y2'…arrow_forward[) Hwk 29 SUBMIT ANSWEK Hwk 30 - (MA 244-03) (SP25) || X - Mind Tap Cengage Learning ☑ MA244-03_Syllabus_Spring, 20 × b Answered: [) 90% Hwk 29 Hwk X Rotation of Axes Example - Elimi X + https://www.webassign.net/web/Student/Assignment-Responses/last?dep=36606609 B שי 90% 2. [-/3 Points] DETAILS MY NOTES LARLINALG8 7.4.003. Use the age transition matrix L and age distribution vector X1 to find the age distribution vectors X2 and x3. 0 34 x2 = X3 = L = ↓ ↑ 1 0 0 x1 = 1 0 0 2 20 20 20 Then find a stable age distribution vector. x = t ↓ 1 Need Help? Read It SUBMIT ANSWER 3. [-/3 Points] DETAILS MY NOTES LARLINALG8 7.4.004. Use the age transition matrix L and age distribution vector X1 to find the age distribution vectors x2 and ×3. ill { ASK YOUR TEACHER PRACTICE ANOTHER ASK YOUR TEACHER PRACTICE ANOTHERarrow_forward[) Hwk 29 SUBMIT ANSWER Hwk 29 - (MA 244-03) (SP25) || X - Mind Tap Cengage Learning ☑ MA244-03_Syllabus_Spring, 20 × b Answered: ( Homework#8 | ba X + https://www.webassign.net/web/Student/Assignment-Responses/submit?dep=36606608&tags=autosave#question3706218_2 2. [-/2.85 Points] DETAILS MY NOTES LARLINALG8 7.3.003. Prove that the symmetric matrix is diagonalizable. (Assume that a is real.) 0 0 a A = a 0 a 0 0 Find the eigenvalues of A. (Enter your answers as a comma-separated list. Do not list the same eigenvalue multiple times.) λ= Find an invertible matrix P such that P-1AP is diagonal. P = Which of the following statements is true? (Select all that apply.) ☐ A is diagonalizable because it is a square matrix. A is diagonalizable because it has a determinant of 0. A is diagonalizable because it is an anti-diagonal matrix. A is diagonalizable because it has 3 distinct eigenvalues. A is diagonalizable because it has a nonzero determinant. A is diagonalizable because it is a symmetric…arrow_forwardUse the method of undetermined coefficients to solve the given nonhomogeneous system. x-()*+(5) = 1 3 3 1 X+ t +3 -1 -2t 1 x(t) = º1 1 e +021 e +arrow_forwardFind the general solution of the given system. 6 -(-1)x x' = -6 11 x(t) = x(t) = e5t)*[(c1 + c2(t− 1/6))(c1 + c2t)] Your answer cannoarrow_forward(c) Describe the distribution plan and show the total distribution cost. Optimal Solution Amount Cost $ 2000 Southern-Hamilton 200 Southern-Butler $ Southern-Clermont 300 4500 Northwest-Hamilton 200 $2400 Northwest-Butler 200 $3000 Northwest-Clermont $ Total Cost ક (d) Recent residential and industrial growth in Butler County has the potential for increasing demand by 100 units. (i) Create an updated distribution plan assuming Southern Gas becomes the preferred supplier. Distribution Plan with Southern Gas Amount Southern-Hamilton $ Cost × Southern-Butler x $ Southern-Clermont 300 $ 4500 Northwest-Hamilton 64 x Northwest-Butler $ × Northwest-Clermont 0 $0 Total Cost $ (ii) Create an updated distribution plan assuming Northwest Gas becomes the preferred supplier. Distribution Plan with Northwest Gas Southern-Hamilton Southern-Butler 0 Southern-Clermont Northwest-Hamilton Northwest-Butler Northwest-Clermont Total Cost Amount × x x +7 $0 Cost × $ × $ × +4 $ -/+ $ × ×arrow_forwardThe distribution system for the Herman Company consists of three plants, two warehouses, and four customers. Plant capacities and shipping costs per unit (in $) from each plant to each warehouse are as follows. Warehouse Plant Capacity 1 2 1 4 7 450 2 8 5 600 3 5 6 380 Customer demand and shipping costs per unit (in $) from each warehouse to each customer are as follows. Customer Warehouse 1 2 3 1 6 4 8 2 3 6 7 7 Demand 300 300 300 400 (a) Develop a network representation of this problem. (Submit a file with a maximum size of 1 MB.) Choose File No file chosen This answer has not been graded yet. (b) Formulate a linear programming model of the problem. (Let Plant 1 be node 1, Plant 2 be node 2, Plant 3 be node 3, Warehouse 1 be node 4, Warehouse 2 be node 5, Customer 1 be node 6, Customer 2 be node 7, Customer 3 be node 8, and Customer 4 be node 9. Express your answers in the form x;;, where x,; represents the number of units shipped from node i to node j.) Min 4x14+8x24+5x34+7x15 +5x25…arrow_forwardA linear programming computer package is needed. Hanson Inn is a 96-room hotel located near the airport and convention center in Louisville, Kentucky. When a convention or a special event is in town, Hanson increases its normal room rates and takes reservations based on a revenue management system. A large profesional organization has scheduled its annual convention in Louisville for the first weekend in June. Hanson Inn agreed to make at least 50% of its rooms available for convention attendees at a special convention rate in order to be listed as a recommended hotel for the convention. Although the majority of attendees at the annual meeting typically request a Friday and Saturday two-night package, some attendees may select a Friday night only or a Saturday night only reservation. Customers not attending the convention may also request a Friday and Saturday two-night package, or make a Friday night only or Saturday night only reservation. Thus, six types of reservations are…arrow_forward25.2. Find the Laurent series for the function 1/[z(z-1)] in the follow- ing domains: (a). 0<|z|< 1, (b). 1<|z, (c). 0arrow_forward25.5. Find the Laurent series for the function 1/[(z - 1)(-2)(z - 3)] in the following domains: (a). 0 3. شهریarrow_forward25.1. Expand each of the following functions f(z) in a Laurent series on the indicated domain: (a). z² - 2z+5 (2-2)(z² + 1)' (c). Log za 2 b (z - موجود 11, 29, where b>a> 1 are real, |z| > b.arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_iosRecommended textbooks for you
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
